Finding The Test Statistic For a Hypothesis Test Using StatCrunch
TLDRIn this educational transcript, the speaker introduces the concept of hypothesis testing, focusing on the distinction between a test statistic and a critical value. The speaker uses a problem from a homework set to illustrate the difference, involving a software firm's survey on adults' willingness to erase their personal information online. The example demonstrates how to calculate the test statistic for a proportion using a sample size of 590, where 62% express a desire to erase their data. The speaker emphasizes that the test statistic is the sample's distribution score, which is different from the critical value, a score needed to achieve a certain confidence level. The explanation includes a step-by-step guide on using StatCrunch to obtain the test statistic, clarifying the process for students new to statistical analysis.
Takeaways
- 📚 The speaker is discussing hypothesis testing with students, specifically using a problem from a homework assignment.
- 🔍 The problem involves a claim about the proportion of adults willing to erase their personal information online, with a survey indicating 62% would do so.
- 🧐 The speaker emphasizes the difference between a test statistic and a critical value, which is a common point of confusion among students.
- 📈 A test statistic is the calculated score from a sample that is used to make inferences about a population, while a critical value is the score needed from a distribution to achieve a certain confidence level.
- 📊 The critical value can be determined before conducting a survey and is based on the desired confidence level and the type of distribution (e.g., normal, t-distribution, chi-squared).
- ✅ To find the test statistic, the speaker uses StatCrunch, a statistical software, and demonstrates how to input the number of successes and observations from the sample.
- 🧮 The number of successes is calculated by multiplying the total number of observations by the percentage of interest (in this case, 62% of 590).
- 📝 The speaker rounds the calculated number of successes to the nearest whole number (366) for input into StatCrunch.
- ⚖️ The null hypothesis is set to the default value (proportion equals 50%), and the alternative hypothesis is set to 'greater than' to reflect the claim that most adults would erase their data.
- 🔢 The test statistic, or z-stat, is provided by StatCrunch after performing the proportion hypothesis test with the given sample information.
- 📝 The z-stat is reported to two decimal places, and the speaker demonstrates how to round the third decimal place if necessary.
- 🎓 The speaker encourages students to continue working hard and looks forward to future interactions.
Q & A
What is the main topic of discussion in the provided transcript?
-The main topic of discussion is the concept of hypothesis testing, specifically focusing on the difference between a test statistic and a critical value within the context of a proportion study.
What is the problem number and chapter section the speaker is referring to?
-The speaker is referring to problem number eight from homework number ten, which is found in chapter eight, section one.
What is the claim made about adults in the survey mentioned in the transcript?
-The claim is that most adults, meaning greater than 50 percent, would erase all of their personal information online if they could.
How many adults were surveyed in the study mentioned?
-A total of 590 adults were surveyed.
What percentage of the surveyed adults said they would erase their personal information online?
-62 percent of the surveyed adults said they would erase their personal information online.
What is the difference between a test statistic and a critical value?
-A test statistic is the distribution score obtained from a sample, such as the sample proportion in this case. A critical value, on the other hand, is the score on the distribution that one would need to reach a particular confidence level and does not directly relate to the sample data.
What is the role of the confidence level in determining the critical value?
-The confidence level, such as 95% or 99%, is used to determine the critical value by indicating the level of certainty required for the hypothesis test.
How does one calculate the number of successes for the sample in StatCrunch when only the percentage is given?
-To calculate the number of successes, you multiply the total number of observations by the decimal form of the percentage. In this case, 590 times 0.62 to get 365.8, which is then rounded to 366.
What is the null hypothesis in the context of the problem discussed?
-The null hypothesis is that the proportion of adults who would erase their data is equal to 50%, which is the default assumption before the survey results are considered.
How does the alternative hypothesis in this problem differ from the null hypothesis?
-The alternative hypothesis states that the proportion of adults who would erase their data is greater than 50%, which aligns with the claim that 'most adults' would do so.
What is the test statistic referred to as in StatCrunch for a proportion test?
-In StatCrunch, the test statistic for a proportion test is referred to as the z-stat.
What is the process for performing a proportion hypothesis test in StatCrunch?
-To perform a proportion hypothesis test in StatCrunch, you input the number of successes (calculated if necessary), the number of observations, set the hypothesis test to match the claim, and then compute to obtain the z-stat.
Outlines
📊 Understanding Test Statistic vs. Critical Value
The first paragraph introduces the topic of hypothesis testing, specifically focusing on the test statistic for a population proportion. It clarifies the difference between a test statistic and a critical value. A test statistic is the score obtained from a sample, such as the proportion of people in a study who would erase their personal information online, which in this case is calculated as 62% of 590 individuals. The critical value, on the other hand, is the score needed on the distribution to achieve a certain confidence level and does not directly relate to the sample data. The speaker illustrates how to calculate the actual number of successes from a given percentage using a calculator and emphasizes the importance of understanding these concepts before proceeding with hypothesis testing.
🧮 Using StatCrunch for Hypothesis Testing
The second paragraph demonstrates how to use StatCrunch software to perform a hypothesis test for a population proportion. It outlines the process of entering the number of successes and total observations into the software, setting up the null and alternative hypotheses to reflect the claim that most adults would erase their online data. The null hypothesis is set to a proportion of 50% (p=0.5), with the alternative hypothesis being that the proportion is greater than 50% (p>0.5), indicating 'most' adults. The paragraph concludes with the computation of the test statistic, known as the z-stat in this context, which is a measure of how many standard deviations an observed proportion is from the hypothesized proportion. The speaker shows how to round the z-stat to two decimal places as required and encourages students to continue their hard work.
Mindmap
Keywords
💡Hypothesis Testing
💡Test Statistic
💡Critical Value
💡Population Proportion
💡Sample Proportion
💡Confidence Level
💡Null Hypothesis
💡Alternative Hypothesis
💡Z-Distribution
💡StatCrunch
💡Survey Data
Highlights
Introduction to hypothesis testing with a focus on distinguishing between test statistic and critical value.
Using problem number eight from homework number ten as an example to illustrate the concepts.
The problem involves a software firm survey of 590 adults, with 62% willing to erase their personal information online.
Clarification that the test statistic is different from the critical value, with the latter being a distribution score for a certain confidence level.
Explanation that the critical value can be determined before conducting a survey based on the desired confidence level and sample size.
Demonstration of how to calculate the test statistic using StatCrunch for a proportion distribution.
Conversion of the percentage of respondents (62%) to the actual number of successes (366) for input into StatCrunch.
Setting up the null hypothesis that the proportion is equal to 50% and the alternative hypothesis that the proportion is greater than 50%.
Procedure to input the number of successes and observations into the StatCrunch calculator for a proportion hypothesis test.
The Z-statistic is identified as the test statistic for a Z-distribution in proportion tests.
StatCrunch provides the Z-statistic, which is the distribution score for the sample based on the sample data.
Emphasis on rounding the Z-statistic to two decimal places for reporting.
Encouragement for students to continue working hard and a promise to see them soon.
The importance of understanding the difference between test statistic and critical value in hypothesis testing.
The role of sample size and confidence level in determining the critical value.
How to use the sample data to calculate the number of successes required for the test statistic.
The process of setting up hypothesis tests in StatCrunch and interpreting the results.
The significance of the Z-statistic in determining whether to reject or fail to reject the null hypothesis.
Practical application of hypothesis testing in real-world scenarios, such as surveys about personal information online.
Transcripts
Browse More Related Video
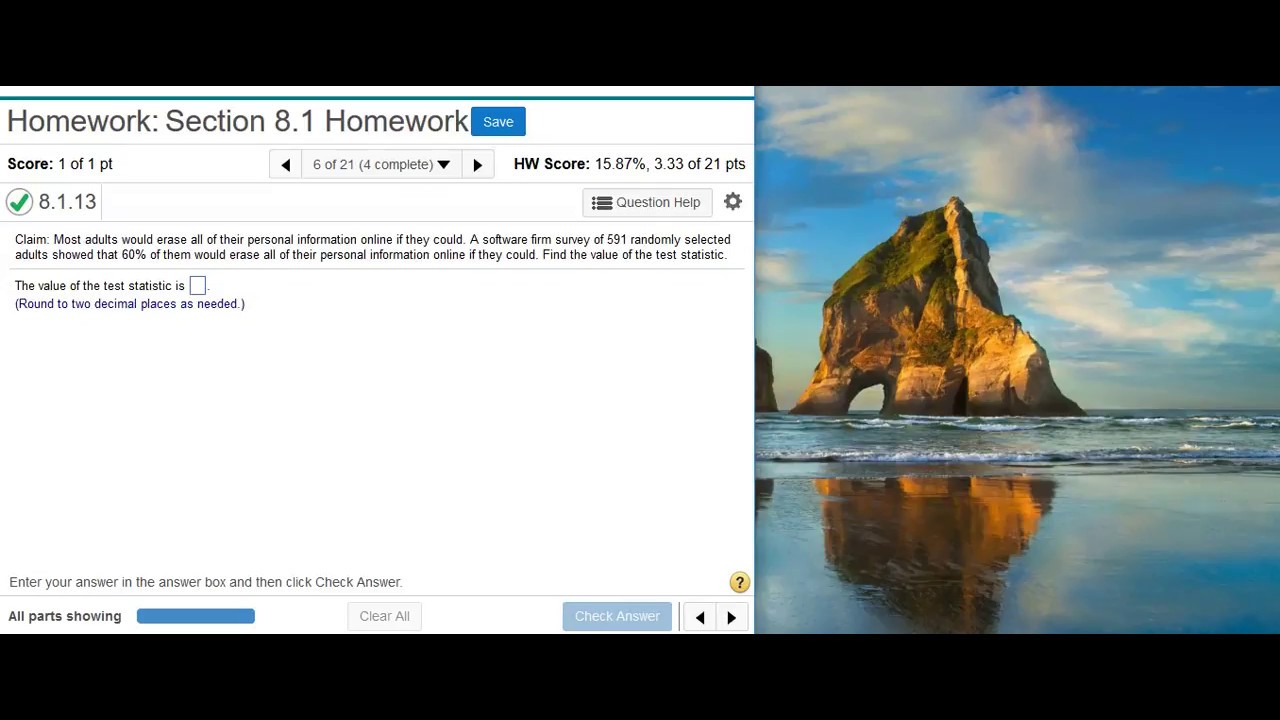
Finding the value of the test statistic for hypothesis testing on proportions
Finding a P-value given the test statistic
9.2.2 Two Means, Independent Samples - The Rationale Behind The Equivalent Methods, P-value Method
8.1.4 Basics of Hypothesis Testing - The Critical Value Method for a Population Proportion

Bootstrap Hypothesis Testing in Statistics with Example |Statistics Tutorial #35 |MarinStatsLectures
Hypothesis Testing With Two Proportions
5.0 / 5 (0 votes)
Thanks for rating: