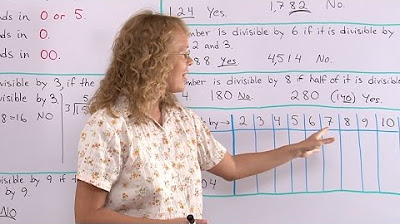This completely changed the way I see numbers | Modular Arithmetic Visually Explained

Zach Star

27 Aug 201920:33

EducationalLearning

32 Likes 10 Comments

TLDRThis video explores fascinating number theory concepts, such as the properties of prime numbers and divisibility rules, using a visual 'wheel math' approach. It demonstrates how to quickly determine if a number is prime by checking divisors less than its square root and explains modular arithmetic through a seven-spoked wheel. The video also touches on the application of these mathematical principles in cryptography, showcasing the Diffie-Hellman protocol for secure communication. Viewers are encouraged to delve deeper into number theory through Brilliant's interactive courses.

Takeaways

🔢 The video introduces number theory concepts with a focus on prime numbers and their properties.
📊 It demonstrates how to determine if a number is prime by checking for divisors less than its square root.
🔑 The script explains the use of modular arithmetic, or 'wheel math,' for visualizing remainders and divisibility.
📐 It shows how to use the properties of exponents and prime numbers to quickly solve divisibility problems.
🎲 The video uses a wheel with a prime number of sections to illustrate Fermat's Little Theorem and other divisibility rules.
📈 The concept of digital roots and their application in determining divisibility by 9 is explained.
🔐 The script touches on the basics of cryptography, mentioning the diffie-hellman protocol for establishing a secret key.
🎯 It highlights the importance of prime numbers in cryptography for secure communication.
📚 The video encourages viewers to explore further with Brilliant's number theory course for a deeper understanding.
🎁 The sponsor, Brilliant, offers a 20% discount for the annual premium subscription to their platform.
📘 The video concludes with a call to action for viewers to like, subscribe, and follow the channel for more content.

Q & A

What is the main topic discussed in the video?
-The main topic of the video is number theory, with a focus on various mathematical properties and theorems related to prime numbers, divisibility, and modular arithmetic.

Outlines

00:00

🔢 Sponsored by Brilliant: Exploring Number Theory

The video is sponsored by Brilliant, introducing intriguing number theory problems. Examples include raising an integer to the 5th power to observe its ones digit, and analyzing prime numbers squared and their divisibility by 24. The speaker discusses the basics of number theory, demonstrating how to determine if a number like 119 is prime by checking divisors up to its square root. The concept of representing composite numbers using primes is explained, emphasizing their unique factorization.

05:01

🧮 Wheel Math and Modular Arithmetic

The concept of modular arithmetic, referred to as 'wheel math', is introduced. A visual representation using a wheel with seven sections illustrates how numbers and their remainders work when divided by seven. The method simplifies addition, multiplication, and exponentiation within this system. The speaker explains that numbers in the same section have congruent remainders, and any number raised to the 6th power on this wheel will have a remainder of one, showcasing Fermat's Little Theorem.

10:02

🔢 Patterns in Prime Numbers and Divisibility

The video explores the properties of numbers, including how raising any number to the fifth power retains its ones digit. Using a wheel with twelve sections, the relationship between prime numbers and their positions is examined. The speaker explains why prime numbers squared minus one are divisible by 24, using the properties of even and odd numbers. The concept of digital roots is introduced, showing how they can determine divisibility by 9 and 3, and their interesting behavior in the Fibonacci sequence.

15:03

🔐 Basics of Cryptography and Secure Communication

The video shifts to cryptography, demonstrating how modular arithmetic can establish secure communication. Using the Diffie-Hellman key exchange protocol, the speaker explains how two parties can create a shared secret key, even with an eavesdropper listening. The method involves selecting a base value and secret numbers, then performing calculations on a wheel with a prime number of sections. This concept underpins modern cryptographic methods, ensuring secure message encryption.

20:03

📚 Brilliant's Courses and Learning Opportunities

The speaker highlights Brilliant's extensive course offerings, including number theory, differential equations, complex analysis, and more. Interactive exercises and practical applications are emphasized to deepen understanding. The platform also provides daily challenges to encourage consistent learning. Viewers are encouraged to sign up for Brilliant to support the channel and gain access to these educational resources.

Mindmap

Keywords

💡Prime Number

A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In the video, prime numbers are central to the discussion of number theory and cryptography, with the script mentioning that any composite number can be uniquely represented as a product of prime numbers. The video also explores properties of prime numbers, such as their relationship with divisibility and their role in Fermat's Little Theorem.

💡Divisibility

Divisibility refers to the property of a number being able to be divided by another number without leaving a remainder. The script discusses various divisibility rules, such as how the sum of the digits of a number can indicate its divisibility by 3 or 9, and how the ones digit can help determine if a number is divisible by 5. The concept is essential for understanding primality tests and modular arithmetic.

💡Modular Arithmetic

Modular arithmetic, or 'wheel math' as colloquially referred to in the script, is a system of arithmetic for integers, where numbers 'wrap around' after they reach a certain value, called the modulus. The video uses a visual wheel to demonstrate how numbers behave under modular arithmetic, particularly with respect to divisibility by 7, and how it can simplify complex problems.

💡Square Root

The square root of a number is a value that, when multiplied by itself, gives the original number. The script mentions the square root in the context of determining whether a number is prime, as one only needs to test for divisibility by prime numbers less than the square root of the number in question. This is because any factor larger than the square root would necessitate a corresponding factor smaller than the square root.

💡Factorial

A factorial of a non-negative integer n, denoted by n!, is the product of all positive integers less than or equal to n. The video uses 18 factorial as an example to illustrate that it cannot be divisible by 23, since 23 is a prime number greater than 18 and is not included in the prime factorization of 18 factorial.

💡Cryptography

Cryptography is the practice and study of secure communication techniques, often involving the use of mathematical algorithms to encrypt and decrypt messages. The script briefly touches on the application of number theory in cryptography, specifically how the properties of prime numbers and modular arithmetic can be used to establish secure communication channels.

💡Fermat's Little Theorem

Fermat's Little Theorem is a result in number theory that states if p is a prime number, then for any integer a, the number a^(p-1) − 1 is an integer multiple of p. The script uses this theorem to explain certain properties of numbers on the 'wheel' with a prime number of sections, such as a number not divisible by 7 raised to the 6th power (7-1) will have a remainder of 1 when divided by 7.

💡Digital Root

The digital root of a number is the recursive sum of its digits until only one digit remains. The script explains how the digital root can indicate the remainder when a number is divided by 9, and how it can be used to quickly determine divisibility by 9. It also mentions that the digital root of numbers in the Fibonacci sequence repeats every 24 numbers.

💡Divisibility Rule

A divisibility rule is a quick way to determine whether a number is divisible by another, without performing the actual division. The script provides the example of the divisibility rule for 9, where the sum of the digits of a number must be divisible by 9 for the number itself to be divisible by 9. This concept is used to simplify the process of checking divisibility.

💡Diffie-Hellman Protocol

The Diffie-Hellman Protocol is a cryptographic method that allows two parties to establish a shared secret key over an insecure channel. The script uses a simplified version of this protocol to illustrate how two individuals can agree on a secret number using modular arithmetic on a wheel with a prime number of sections, which is a fundamental concept in secure communication.

Highlights

Sponsored video by Brilliant exploring number theory and its applications.

Raising any integer to the 5th power retains the same ones digit.

Squaring any prime number greater than 3 and subtracting one results in a number divisible by 24.

Introduction to the basics of number theory in a visual format.

Explanation of how to determine if a number is prime by checking divisors.

Prime numbers are the building blocks of all other numbers.

Composite numbers have a unique prime factorization.

18 factorial is not divisible by 23 due to prime factor composition.

The theorem that a composite number's prime factors must be less than its square root.

Demonstration of using the square root theorem to check for compositeness.

Introduction to 'wheel math' or modular arithmetic for visual problem-solving.

Wheel math demonstrates remainders and divisibility through a spiral pattern.

Properties of numbers on a wheel with a prime number of spokes, like 7.

Application of wheel math to quickly determine divisibility and remainders.

Fermat's Little Theorem explained through the wheel math concept.

Digital root concept and its application to divisibility by 9.

The connection between digital roots and the Fibonacci sequence.

Cryptography application using the diffie-hellman protocol as an example.

Brilliant.org's number theory course and its comprehensive learning approach.

Invitation to subscribe and support the channel for more educational content.

Transcripts

Browse More Related Video

Divisibility Rules

Divisibility rules for 3, 4, 6, 8 and 9

Mathematicians Use Numbers Differently From The Rest of Us

Impossible Squares - Numberphile

Riemann Hypothesis - Numberphile

Every Type of Math Explained in 9 Minutes.

Related Tags

Number Theory Cryptography Prime Numbers Modular Arithmetic Cryptographic Protocols Mathematics Education Fermat's Little Theorem Diffie-Hellman Digital Roots Fibonacci Sequence