Measuring the mass of a metre rule using the Principle of Moments

Simon Lloyd
15 Nov 202006:43
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the host demonstrates a simple experiment to measure the mass of a meter ruler using the principle of moments. The process involves locating the ruler's center of mass, then balancing it with slotted masses at different positions. By adjusting the position of the masses and recording the distances from the pivot, the mass of the ruler is calculated. The actual mass of the ruler is revealed at the end, allowing viewers to compare their calculations.

Takeaways
  • 📏 The objective is to measure the mass of a meter ruler using the principle of moments.
  • 🔨 The equipment needed includes a triangular block of wood as a pivot, 10-gram masses, and some strength.
  • ⚖️ The first step is to locate the center of mass of the ruler by balancing it on the pivot.
  • 🎯 The center of mass is found at the 50.3 cm mark on the ruler.
  • 🔄 The process involves moving the center of mass and hanging the slotted masses on the other side to create a balance.
  • 📐 The position of the slotted masses is kept constant at 950 millimeters from the pivot.
  • 🔢 Three different trials are conducted with varying amounts of mass hung from the slotted masses.
  • 📈 The balance is adjusted by moving the pivot position until the anti-clockwise moment from the ruler equals the clockwise moment from the slotted masses.
  • 📉 The pivot positions and corresponding slotted mass positions are recorded for each trial.
  • 🧮 The values obtained are used to calculate the mass of the ruler, aiming to match the actual mass of 89.62 grams.
  • 📝 The practical demonstrates a simple method to measure mass using principles of physics without a scale.
Q & A

  • What is the main objective of the practical described in the transcript?

    -The main objective of the practical is to measure the mass of a meter ruler using the principle of moments.

  • What materials are required for this practical?

    -The materials required are a triangular block of wood as a pivot, 10-gram masses, and a meter ruler.

  • How is the center of mass of the ruler located in the practical?

    -The center of mass of the ruler is located by balancing the ruler on the pivot until it is stable.

  • What is the approximate position of the center of mass of the ruler as mentioned in the transcript?

    -The approximate position of the center of mass of the ruler is at the 50.3 cm mark.

  • How is the mass of the ruler measured using the principle of moments?

    -The mass of the ruler is measured by hanging different weights (10-gram masses) on one side of the ruler and adjusting the position of the ruler until it balances again, using the principle of moments.

  • What is the significance of keeping the position of the slotted masses constant during the experiment?

    -Keeping the position of the slotted masses constant allows for consistent measurements and accurate calculation of the mass of the ruler based on the principle of moments.

  • What is the initial position of the slotted masses in the experiment?

    -The initial position of the slotted masses is kept at 950 millimeters from the pivot.

  • How many different values of masses and distances from the pivot are measured in the experiment?

    -Three different values of masses and distances from the pivot are measured in the experiment.

  • What is the actual mass of the ruler mentioned in the transcript?

    -The actual mass of the ruler is 89.62 grams.

  • What is the purpose of adding different amounts of mass to the slotted masses?

    -Adding different amounts of mass to the slotted masses helps to find the point of balance at various weights, which is necessary for calculating the mass of the ruler using the principle of moments.

  • How does the experiment use the principle of moments to calculate the mass of the ruler?

    -The experiment uses the principle of moments by balancing the anti-clockwise moment due to the weight of the ruler with the clockwise moment due to the weight of the slotted masses, and then using the measured distances and known masses to calculate the unknown mass of the ruler.

Outlines
00:00
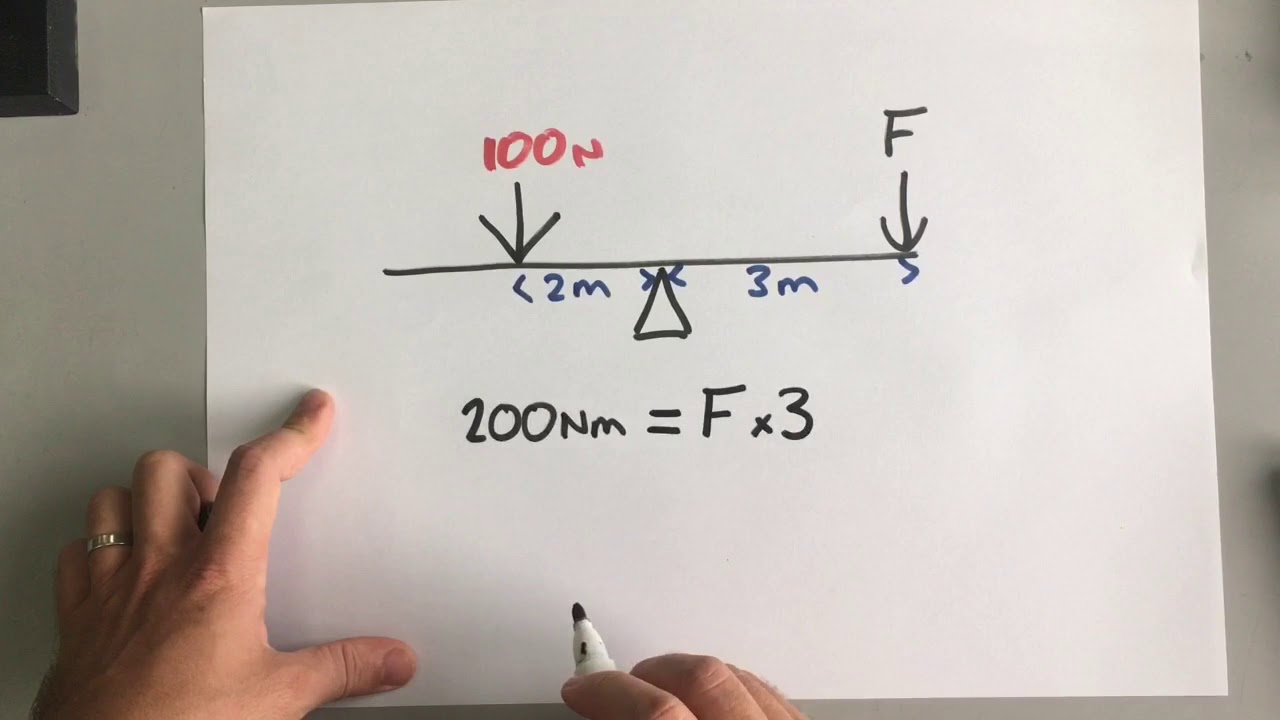
📏 Measuring the Mass of a Meter Ruler Using the Principle of Moments

This paragraph explains a practical experiment to measure the mass of a meter ruler using the principle of moments. The process involves using a triangular block of wood as a pivot, 10-gram masses, and some strength. The first step is to locate the center of mass of the ruler by balancing it on the pivot. Once the center of mass is found at 50.3 centimeters, the experimenter proceeds to move the center of mass to one side and hangs the slotted masses on the other side. The goal is to balance the anti-clockwise moment of the ruler's weight with the clockwise moment of the slotted masses' weight. The experiment is repeated with different amounts of mass to obtain three different values for mass and distance from the pivot.

05:01
🔍 Calculating the Mass of the Ruler and Comparing with Actual Measurement

In this paragraph, the focus shifts to calculating the mass of the meter ruler using the values obtained from the previous experiment. The experimenter keeps the position of the slotted masses constant at 950 millimeters and varies the pivot position to achieve balance with different amounts of mass added. The final values for the pivot positions and the slotted masses are recorded, and these are used to calculate the mass of the ruler. The actual mass of the ruler is revealed to be 89.62 grams, and the paragraph challenges the viewers to compare their calculated values with this actual measurement to see how accurate their moments calculations are.

Mindmap
Keywords
💡Mass
Mass refers to the amount of matter in an object, typically measured in grams or kilograms. In the video, the primary objective is to measure the mass of a meter ruler using the principle of moments. The script describes the process of finding the center of mass and using it to calculate the mass of the ruler, which is a fundamental concept in physics related to balance and equilibrium.
💡Center of Mass
The center of mass is the point at which the mass of an object is concentrated for the purpose of analyzing its motion. In the video, the center of mass of the ruler is determined by balancing it on a pivot, which is crucial for the experiment as it allows for the accurate measurement of the ruler's mass using the principle of moments.
💡Pivot
A pivot is a fixed point around which a body turns or is supported. In the context of the video, a triangular block of wood serves as a pivot to balance the ruler and find its center of mass. The pivot is essential for the experiment as it provides the reference point for measuring the distances and applying the principle of moments.
💡Moments
In physics, moments refer to the turning effect of a force or weight around a pivot point. The principle of moments is used in the video to measure the mass of the ruler by balancing the anti-clockwise moment due to the ruler's weight with the clockwise moment due to the slotted masses.
💡Slotted Masses
Slotted masses are weights with a slot that can be hung at various points to create a moment around a pivot. In the video, the slotted masses are used to apply a known force at different distances from the pivot, which helps in calculating the unknown mass of the ruler by creating a balanced moment.
💡Balance
Balance is the state of being equally distributed or having two or more quantities that are equal or equivalent. The script describes the process of balancing the ruler on the pivot to find the center of mass and then using slotted masses to create a balanced moment, which is necessary for the accurate measurement of mass.
💡Distance from Pivot
The distance from the pivot is a critical measurement in the experiment as it determines the moment arm—the distance over which a force is applied to create a moment. In the video, the distances from the pivot to the center of mass and to the slotted masses are measured to calculate the moments and ultimately the mass of the ruler.
💡Measurement
Measurement is the process of determining the size, amount, or degree of something. In the video, various measurements are taken, such as the distance from the pivot to the center of mass and to the slotted masses, which are essential for applying the principle of moments to calculate the mass of the ruler.
💡Experiment
An experiment is a scientific procedure undertaken to make a discovery, test a hypothesis, or demonstrate a known fact. The video describes a practical experiment aimed at measuring the mass of a meter ruler using the principle of moments, which is a hands-on application of physics principles.
💡Calculation
Calculation refers to the process of computing or reckoning, especially by mathematical methods. In the context of the video, calculations are made using the measured distances and known masses to determine the unknown mass of the ruler through the principle of moments.
💡Accuracy
Accuracy is the quality of being correct or precise. The video emphasizes the importance of accurate measurements and calculations in determining the mass of the ruler, as even small errors can lead to significant deviations from the actual mass.
Highlights

Introduction of a practical experiment to measure the mass of a meter ruler using the principle of moments.

Requirement of a pivot, triangular block of wood, 10 gram masses, and strength for the experiment.

Explanation of locating the center of mass of the ruler by balancing it on the pivot.

Demonstration of finding the center of mass at the 50.3 centimeter mark on the ruler.

Process of moving the center of mass by hanging slotted masses on one side of the ruler.

First trial with 20 grams of slotted masses hung at 950 millimeters from the pivot.

Balancing the ruler to ensure the anti-clockwise and clockwise moments are equal.

Measurement of the new pivot position at 585 millimeters after adding slotted masses.

Second trial with 40 grams of slotted masses, maintaining the position at 950 millimeters.

Adjustment of the pivot to 642 millimeters to achieve balance with the increased mass.

Third trial with 60 grams of slotted masses, keeping the position constant at 950 millimeters.

Final pivot position measurement at 685 millimeters with the 60-gram mass.

Calculation of the mass of the ruler using the measured values of the center of mass, slotted mass position, and pivot position.

Revealing the actual mass of the ruler as 89.62 grams for comparison with calculated results.

Emphasis on the accuracy of the moments calculation method in determining the mass of the ruler.

Encouragement for viewers to compare their calculated mass with the actual mass of the ruler.

Transcripts

Browse More Related Video

Equivalence Principle

AP Physics Workbook 5.L Inelastic Collisions

Center of Mass & Centroid Problems - Calculus

Calculating masses in reactions - p27 (Chem)

GCSE Physics - Forces 5 - Moments and Centre of Mass

Center of Mass | Physics with Professor Matt Anderson | M12-04

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics PracticalCenter of MassBalance MethodMomentum CalculationEducational TutorialWeight MeasurementEngineering BasicsScience ExperimentRuler MassPractical Skills