Introduction to Sum and Difference Formulas in Trigonometry (Precalculus - Trigonometry 25)

Professor Leonard

20 Oct 202151:27

EducationalLearning

32 Likes 10 Comments

TLDRThis video script offers an insightful exploration into the world of trigonometric sum and difference formulas, demonstrating their utility in finding exact solutions for angles not directly available on the unit circle. The presenter breaks down complex concepts, such as representing non-unit circle angles as sums or differences of known angles, and applying formulas for sine, cosine, and tangent. The script is enriched with practical examples, like calculating sine of 105 degrees and tangent of 19 pi over 12, showcasing step-by-step processes that include simplifying expressions and rationalizing denominators. It emphasizes the importance of memorizing these formulas and understanding their derivation from the unit circle. The video also touches on the application of these formulas in reverse, highlighting their role as identities that work both ways, which is crucial for solving more complex trigonometric problems. Overall, the script is an engaging and informative guide for anyone looking to deepen their understanding of trigonometric identities and their applications.

Takeaways

📚 Sum and difference formulas are essential for finding exact solutions for trigonometric functions when dealing with angles not on the unit circle.
🔍 The formulas originate from the unit circle's distance formula and the comparison of sine to cosine, particularly useful in calculus and dealing with multiple angles.
➕ To use the formulas, break down the given angle into a sum or difference of two angles that are on the unit circle, then apply the appropriate sine, cosine, or tangent formula.
📐 Memorize the pattern for sine and cosine: sine terms have the same sign, while cosine terms have opposite signs in the formulas.
🤔 For angles not directly on the unit circle, such as 105 degrees, find two angles (e.g., 60 and 45 degrees) that add up to the given angle and use the sum formula.
🧮 Use the unit circle to find the exact values of sine and cosine for the angles identified, then apply the sum or difference formula to find the exact solution.
😕 For angles like pi/12, which are not on the unit circle, find two angles (e.g., 4pi/12 - 3pi/12) that when subtracted give the desired angle, and use the difference formula.
🤓 Tangent formulas are derived from the sine and cosine ratios and require special attention to signs and the order of angles when applying the sum or difference formula.
📈 Rationalize the denominator when necessary, especially when dealing with square roots, to simplify the final expression and avoid complex numbers in the denominator.
🔢 Memorizing the unit circle and the corresponding values of sine and cosine for common angles is crucial for efficiently applying the formulas.
🔃 Even and odd properties of trigonometric functions can simplify calculations with negative angles or when using functions like secant, which is the reciprocal of cosine.

Q & A

What are sum and difference formulas used for in trigonometry?
-Sum and difference formulas are used in trigonometry to find exact solutions for trigonometric functions when the angles are not on the unit circle. They can also help with identities and are useful in calculus when dealing with multiple angles that need to be combined or separated.
How do sum and difference formulas originate?
-These formulas originate from the distance formula on part of the unit circle. The tangent formula comes from comparing the sine to the cosine function, where tangent of an angle can be represented as sine over cosine.
How can we find the exact solution for an angle like 105 degrees which is not on the unit circle?
-To find the exact solution for an angle like 105 degrees, you can represent the angle as a sum or difference of two angles that are on the unit circle. Then, you can use the appropriate sum or difference formula to break it up and find the exact representation.
What is the sum formula for sine in terms of two angles?
-The sum formula for sine in terms of two angles, alpha and beta, is sine(alpha + beta) = (sine(alpha) * cosine(beta)) + (cosine(alpha) * sine(beta)).
How can you represent 165 degrees using angles on the unit circle?
-You can represent 165 degrees as the sum of 120 degrees and 45 degrees, both of which are on the unit circle. This allows you to use the sum formula for cosine to find the exact representation.
What is the tangent sum formula for two angles?
-The tangent sum formula for two angles, alpha and beta, is (tangent(alpha) + tangent(beta)) / (1 - (tangent(alpha) * tangent(beta))).
Why is it important to rationalize the denominator when dealing with fractions in trigonometry?
-Rationalizing the denominator is important because it simplifies the fraction and eliminates any square roots in the denominator, which can make the expression easier to work with and understand.
How can you find the secant of an angle that is not on the unit circle?
-You can find the secant of an angle not on the unit circle by expressing it as a difference or sum of two known angles on the unit circle and then using the reciprocal relationship between secant and cosine, since secant is the reciprocal of cosine.
What is the relationship between the secant function and the cosine function?
-The secant function is the reciprocal of the cosine function. This means that secant(theta) = 1 / cosine(theta) for any angle theta.
How can you use the properties of even and odd functions to simplify trigonometric expressions?
-For even functions like cosine and secant, if you input opposite angles, you get equal results. For odd functions like sine and tangent, if you input opposite angles, the outputs are opposite in sign. This property can be used to simplify expressions by changing the sign of the angle or finding equivalent angles that are easier to work with.
Why is it essential to maintain the order of angles in sum and difference formulas?
-The order of angles in sum and difference formulas is crucial because these operations are not commutative. This means that the first angle must be subtracted by the second angle, not the other way around, to maintain the correct mathematical relationship.

Outlines

00:00

📚 Introduction to Sum and Difference Formulas

This paragraph introduces the topic of sum and difference formulas in trigonometry. It emphasizes their utility in finding exact solutions for angles not on the unit circle and their application in calculus and identities. The explanation begins with the basic premise that trigonometric functions of two angles (added or subtracted) can be broken down using these formulas, which originate from the unit circle and the relationship between sine and cosine. The paragraph also illustrates how to use these formulas with an example of finding the sine of 105 degrees by expressing it as the sum of angles 60 and 45 degrees, both of which are on the unit circle.

05:03

🔍 Simplifying Trigonometric Expressions

The second paragraph delves into simplifying trigonometric expressions using the unit circle. It explains how to find the exact representation of sine for 105 degrees by using the sum of angles 60 and 45 degrees. The process involves finding the sine and cosine values for each of these angles and then applying the sum formula for sine. The paragraph also touches on the concept of memorization and the utility of the unit circle in these calculations. It concludes with an example of how to represent angles not on the unit circle, such as pi over 12, by using differences of angles that are on the unit circle.

10:04

🤔 Applying Sum Formulas for Cosine and Sine

The third paragraph focuses on applying sum formulas for cosine and sine. It discusses how to represent large angles, such as 165 degrees, as the sum of two angles on the unit circle, like 120 and 45 degrees. The paragraph explains the process of using the sum formula for cosine, which involves pairing cosines and sines and switching signs appropriately. The example provided calculates the cosine of 165 degrees by breaking it down into cosine of 120 degrees and sine of 45 degrees, then simplifying to get the exact x-coordinate on the unit circle for that angle.

15:05

📈 Using Tangent Sum Formulas

This paragraph explores the use of tangent sum formulas for dealing with large angles not on the unit circle. It demonstrates how to express 19 pi over 12 as a sum of two angles, 4 pi over 3 and pi over 4, both of which can be found on the unit circle. The paragraph explains the tangent sum formula, which involves adding the tangents of the two angles and dividing by one minus their product. The example shows the calculation process using the tangent values for the two angles and then rationalizing the denominator to simplify the expression.

20:05

🧮 Secant and Cosine Reciprocity

The sixth paragraph discusses the relationship between secant and cosine, which are reciprocals of each other. It explains how to handle secant of an angle not on the unit circle by using the cosine of the difference of two angles that are on the unit circle. The paragraph details the process of finding the cosine of the difference between pi over 3 and pi over 4, and then taking the reciprocal to find the secant. It also highlights the need to rationalize the denominator to avoid square roots in the final expression.

25:07

🔁 Applying Sum and Difference Formulas in Reverse

The seventh paragraph covers the application of sum and difference formulas in reverse. It explains how to recognize patterns in trigonometric expressions that fit these formulas and rearrange them to find the angles involved. The paragraph emphasizes the importance of the order of angles in subtraction and the use of odd and even properties of trigonometric functions. It provides an example of simplifying an expression involving sine to reveal it as the sine of the difference between two angles.

30:10

🎓 Advanced Applications and Inverses

The final paragraph teases upcoming content that will explore more advanced applications of sum and difference formulas, including dealing with angles not representable on the unit circle. It also mentions plans to discuss proofs related to phase shifting for sines and cosines, and to cover inverses in relation to these formulas. The speaker expresses hope that the viewer has found the introduction to sum and difference formulas useful and ends with a positive note, promising more advanced concepts in subsequent videos.

Mindmap

Keywords

💡Sum and Difference Formulas

Sum and difference formulas are mathematical expressions used in trigonometry to find the exact values of trigonometric functions for angles that are the sum or difference of two known angles. In the video, these formulas are crucial for solving problems where angles are not directly found on the unit circle, such as sine of 105 degrees, which is not directly available but can be expressed as the sum of sine of 60 degrees and cosine of 45 degrees.

💡Unit Circle

The unit circle is a circle with a radius of 1 centered at the origin of a coordinate plane, used in trigonometry to define the sine, cosine, and tangent of an angle. The video emphasizes the importance of the unit circle in finding exact solutions for angles that are not directly on the circle by using sum and difference formulas.

💡Trigonometric Functions

Trigonometric functions, such as sine, cosine, and tangent, are mathematical functions that describe the relationship between the angles and sides of a triangle. In the video, these functions are used to calculate the values for specific angles, especially when dealing with angles that are not directly on the unit circle.

💡Exact Solution

An exact solution in trigonometry refers to a precise value for a trigonometric function without any approximation. The video discusses how sum and difference formulas can be used to find exact solutions for angles that are not directly measurable on the unit circle, providing a way to calculate the y-coordinate of a point on the unit circle for a given angle.

💡Tangent Function

The tangent function, often abbreviated as tan, is a trigonometric function that equals the ratio of the sine to the cosine of an angle. In the context of the video, tangent is used in the sum and difference formulas to find the exact values for angles that are not on the unit circle, such as tangent of 19 pi over 12.

💡Phase Shift

A phase shift in trigonometry refers to a horizontal shift of the graph of a trigonometric function. While not explicitly mentioned in the script, the concept is related to the manipulation of angles in sum and difference formulas, which can be thought of as a way to shift angles to those that are more easily managed on the unit circle.

💡Reciprocal Functions

Reciprocal functions in trigonometry are functions that are the inverse of one another, such as the secant and cosine functions. The video explains that secant of an angle is the reciprocal of cosine of the same angle, which is used to simplify the process of finding the secant of an angle that is not directly on the unit circle.

💡Even and Odd Functions

Even and odd functions are classifications of functions based on their symmetry. An even function is symmetric about the y-axis, meaning that f(x) = f(-x), while an odd function is symmetric about the origin, meaning that f(x) = -f(-x). In the video, the concept is used to simplify the calculation of secant for negative angles by utilizing the even nature of the secant function.

💡Rationalizing the Denominator

Rationalizing the denominator is the process of eliminating a radical (such as a square root) from the denominator of a fraction. In the video, this technique is used to simplify expressions obtained from sum and difference formulas, making them easier to work with and understand.

💡Inverse Functions

Inverse functions are functions that 'reverse' the operation of the original function. While not deeply explored in the script, the concept is alluded to in the context of discussing what will be covered in future videos, including the relationship between sum and difference formulas and inverse trigonometric functions.

💡Trigonometric Identities

Trigonometric identities are equations that hold true for all values of the variables involved, representing fundamental relationships between trigonometric functions. The video script discusses how sum and difference formulas are a type of trigonometric identity that can be used in both directions to simplify and solve trigonometric expressions.

Highlights

Sum and difference formulas are useful for finding exact solutions for angles not on the unit circle.

Trigonometric functions of two angles added or subtracted can be broken down using these formulas.

The origin of sum and difference formulas lies in the unit circle's distance formula and the comparison of sine to cosine.

Tangent can be represented as sine over cosine, which simplifies to the sum or difference of the angles alpha and beta.

For non-unit circle angles, representing the angle as a sum or difference of two known angles allows using sum and difference formulas.

The sum formula for sine is sine(alpha)cosine(beta) + cosine(alpha)sine(beta), and for difference, it's sine(alpha)cosine(beta) - cosine(alpha)sine(beta).

The unit circle is a valuable tool for finding exact representations of angles using sum and difference formulas.

Sine and cosine functions have the same sign in their respective terms within sum and difference formulas.

The process of finding exact solutions involves memorizing patterns and using the unit circle for reference.

For angles like 105 degrees, representing it as a sum of 60 and 45 degrees allows applying the sum formula for sine.

The sum and difference formulas for cosine follow a similar structure but with cosine and sine functions paired differently.

Tangent formulas for the sum or difference of two angles involve the tangents of the individual angles and their product.

Rationalizing the denominator is a common step when dealing with square roots in the denominator of a fraction.

The concept of even and odd functions applies to trigonometric functions, simplifying calculations with negative angles or using function properties.

Secant, being the reciprocal of cosine, can be found by first calculating the cosine of the angles and then taking the reciprocal.

Sum and difference formulas can be applied in reverse to simplify expressions by recognizing patterns that match the formulas' structures.

Understanding and applying sum and difference formulas for trigonometric functions is essential for advanced mathematics and calculus.

Transcripts

Browse More Related Video

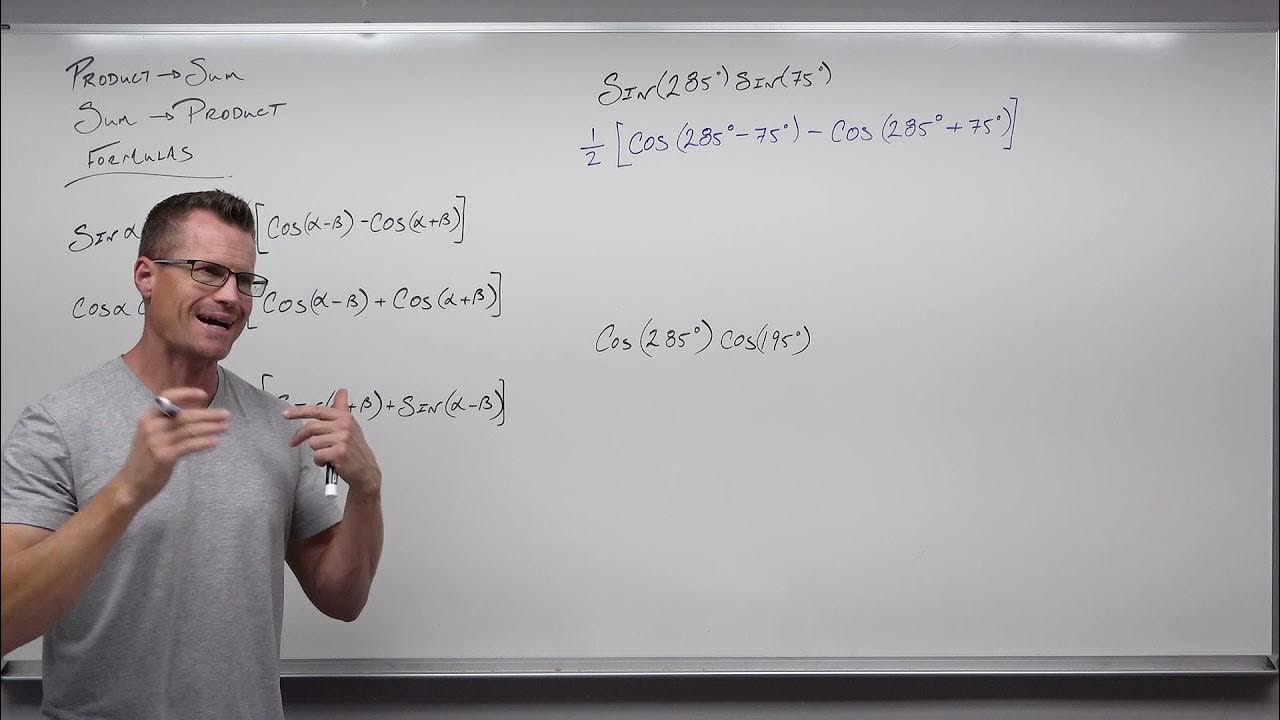
How to Use Product to Sum and Sum to Product Formulas in Trig (Precalculus - Trigonometry 29)

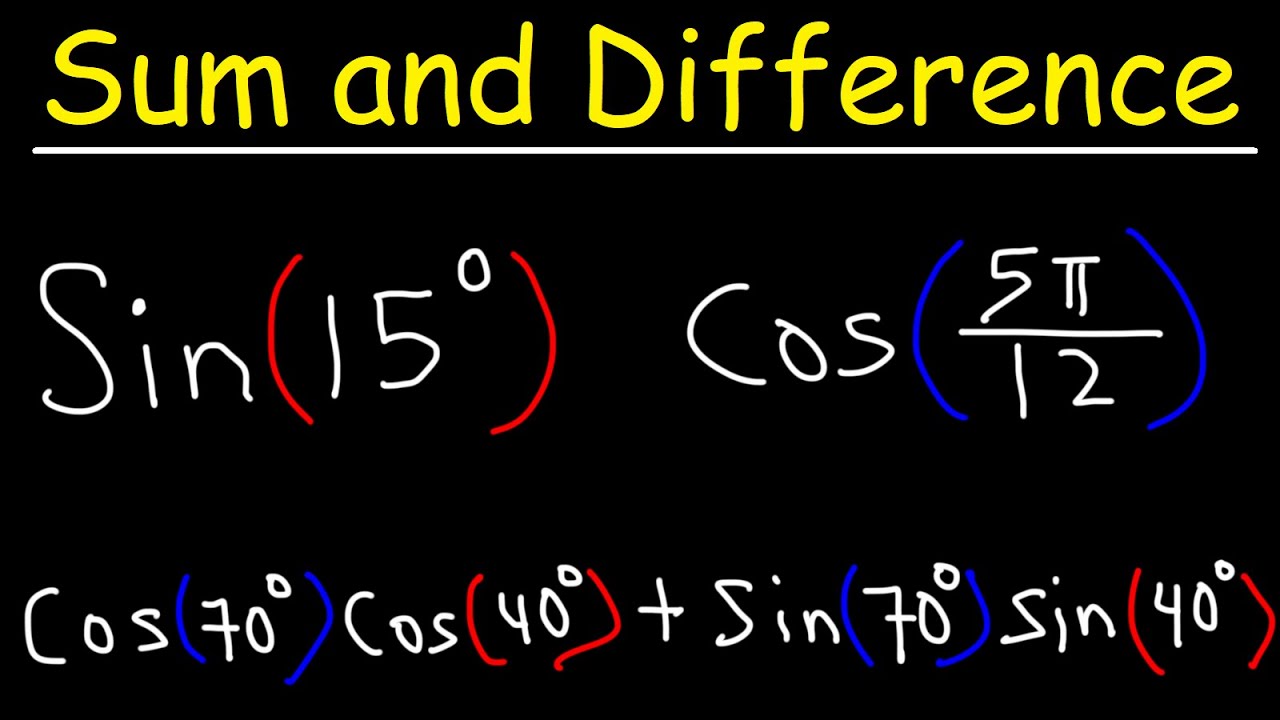
Sum and Difference Identities of Sine and Cosine

Ultimate trig identity problem study guide!

Reciprocal Identities - Evaluating Secant and Cosecant Functions

Pythagorean Identities for Trigonometric Functions (Precalculus - Trigonometry 10)

Basic Properties of Trigonometric Functions (Precalculus - Trigonometry 8)

Related Tags

Trigonometry Sum Difference Unit Circle Sine Cosine Tangent Mathematics Calculus Angle Solutions Trig Identities Educational Content