Solving Systems of Equations By Elimination & Substitution With 2 Variables
TLDRThis educational video script covers two methods for solving systems of linear equations: elimination and substitution. The elimination method involves adding or subtracting equations to cancel out one variable, simplifying the system to find the value of the other. The substitution method requires expressing one variable in terms of the other and then substituting it into the second equation. The script provides step-by-step examples for each method, demonstrating how to manipulate equations to isolate and solve for x and y, ultimately finding the solution as an ordered pair.
Takeaways
- 📚 The script explains how to solve systems of two equations using two methods: elimination and substitution.
- ➕ In the elimination method, the goal is to add or subtract equations to cancel out one variable, making it easier to solve for the other.
- 🔍 The first example uses elimination to solve for x and y in the equations 2x + 3y = 8 and 5x - 3y = -1, resulting in the solution (x, y) = (1, 2).
- 🔄 The second example demonstrates modifying equations by multiplying to facilitate the cancellation of a variable, leading to the solution (x, y) = (2, 3).
- 📝 The substitution method is introduced as an alternative approach, where one variable is expressed in terms of the other and then substituted into the second equation.
- 🔑 The third example uses substitution to solve for x and y in the equations y = 5 - 2x and 4x + 3y = 13, yielding the solution (x, y) = (1, 3).
- 🔍 The fourth example shows how to solve for x and y using substitution when given y = 3x + 2 and y = 7x - 6, resulting in the solution (x, y) = (2, 8).
- 🤔 The script mentions a preference for the elimination method when both x and y have coefficients that could be easily manipulated, but acknowledges that substitution may be necessary in some cases.
- 📉 The fifth example illustrates the substitution method with the equations 4x + 2y = 14 and 3x - 5y = -22, solving for x and y to find the solution (x, y) = (1, 5).
- 📝 The transcript emphasizes the step-by-step process, including distributing, combining like terms, and isolating variables to find the solution for each method.
- 👍 The script concludes by reinforcing the ability to solve systems of equations using both elimination and substitution methods, providing clear examples for each.
Q & A
What is the elimination method for solving a system of equations?
-The elimination method involves adding or subtracting the equations to eliminate one variable, making it possible to solve for the other variable.
How do you use the elimination method to solve the system of equations 2x + 3y = 8 and 5x - 3y = -1?
-By adding the two equations, the y terms cancel out, leaving 7x = 7, which simplifies to x = 1. Substituting x = 1 into one of the original equations allows you to solve for y.
What is the result of adding the equations 2x + 3y = 8 and 5x - 3y = -1 using the elimination method?
-The result is 7x = 7, as the y terms (3y and -3y) cancel each other out, and 8 + (-1) simplifies to 7.
How do you find the value of x in the system of equations 2x + 5y = 19 and x - 2y = -4?
-By multiplying the second equation by -2 and adding it to the first equation, the x terms cancel out, allowing you to solve for y, and then substitute back to find x.
What is the solution to the system of equations 2x + 5y = 19 and x - 2y = -4 after using the elimination method?
-The solution is x = 2 and y = 3, which can be represented as the ordered pair (2, 3).
What is substitution in the context of solving a system of equations?
-Substitution is a method where you express one variable in terms of the other from one equation and then substitute this expression into the second equation to solve for the remaining variable.
How do you use the substitution method to solve the system of equations y = 5 - 2x and 4x + 3y = 13?
-By substituting y = 5 - 2x into the second equation, you get an equation in terms of x only, which you can solve to find the value of x, and then substitute back to find y.
What is the solution to the system of equations y = 5 - 2x and 4x + 3y = 13 using the substitution method?
-The solution is x = 1 and y = 3, which can be represented as the ordered pair (1, 3).
How do you solve the system of equations y = 3x + 2 and y = 7x - 6 using substitution?
-By setting 3x + 2 equal to 7x - 6 and solving for x, then substituting the value of x back into either equation to find y.
What is the solution to the system of equations 4x + 2y = 14 and 3x - 5y = -22 using substitution?
-The solution is x = 1 and y = 5, which can be represented as the ordered pair (1, 5).
Why might the elimination method be preferred over substitution in some cases?
-The elimination method might be preferred when the coefficients of the variables in the equations are smaller or more easily manipulated to cancel out one of the variables.
Outlines
📚 Solving Systems of Equations Using Elimination Method
This paragraph introduces the elimination method for solving systems of linear equations with two variables. It demonstrates how to add equations to eliminate one variable by making the coefficients of that variable equal and opposite. The example provided solves for x and y in the equations 2x + 3y = 8 and 5x - 3y = -1, resulting in the solution (x, y) = (1, 2). The method is further illustrated with a second example where the equations are 2x + 5y = 19 and x - 2y = -4, leading to the solution (x, y) = (2, 3). The paragraph effectively explains the steps of the elimination process, including multiplying equations to facilitate the cancellation of a variable and substituting the found value back into one of the original equations to solve for the second variable.
🔍 Solving Systems of Equations Using Substitution Method
The second paragraph explains the substitution method for solving systems of equations. It begins with an example where one equation is solved for y, resulting in y = 5 - 2x, and then this expression for y is substituted into the second equation, 4x + 3y = 13, to find the value of x. After solving, it is found that x = 1, and subsequently, y = 3, giving the solution (x, y) = (1, 3). The paragraph continues with another example where two expressions for y are given as y = 3x + 2 and y = 7x - 6. By setting these equal to each other, the paragraph shows how to solve for x, resulting in x = 2, and then y = 8, leading to the solution (x, y) = (2, 8). The final example in the paragraph involves the equations 4x + 2y = 14 and 3x - 5y = -22. The paragraph details the process of isolating y and substituting it into the other equation to solve for x, and then finding y, resulting in the solution (x, y) = (1, 5). The summary emphasizes the step-by-step approach of the substitution method and its application to different types of systems of equations.
Mindmap
Keywords
💡Equations
💡Elimination Method
💡Addition Method
💡Substitution
💡Ordered Pair
💡Like Terms
💡Distributive Property
💡Isolate
💡Divide
💡Simplify
Highlights
Introduction to solving a system of two equations using the elimination method.
Explanation of how to eliminate variables by adding equations to simplify the system.
Solution for the first example: finding x and y values using the elimination method.
Division of both sides by seven to isolate x in the equation.
Substitution of x value into the first equation to find y.
Final ordered pair solution for the first example: (1,2).
Introduction to the second example with equations 2x + 5y = 19 and x - 2y = -4.
Strategy for choosing which variable to eliminate based on coefficients.
Multiplication of the second equation by negative two to cancel x.
Combining modified equations to eliminate x and solve for y.
Division by 9 to find the value of y after combining equations.
Substitution of y value into the first equation to solve for x.
Solution for the second example: ordered pair (2,3).
Introduction to solving systems of equations using the substitution method.
Substitution of y with 5 - 2x in the second equation to solve for x.
Combining like terms and solving for x after substitution.
Finding the value of y by substituting x into the first equation.
Solution for the substitution example: ordered pair (1,3).
Approach to solving a system with y = 3x + 2 and y = 7x - 6 using substitution.
Solving for x by simplifying the equation 3x + 2 = 7x - 6.
Finding the value of x and subsequently y for the given system.
Solution as an ordered pair (2,8) for the substitution example with y equations.
Introduction to solving the system 4x + 2y = 14 and 3x - 5y = -22 using substitution.
Isolating y in the first equation to facilitate substitution into the second equation.
Substituting y with -2x + 7 into the second equation and solving for x.
Final solution for the system as an ordered pair (1,5).
Transcripts
Browse More Related Video

7.1.1 Solving a System of Equations Using Substitution

Solving Systems of Two Equations and Two Unknowns: Graphing, Substitution, and Elimination

Simultaneous Equations - Tons of Examples!
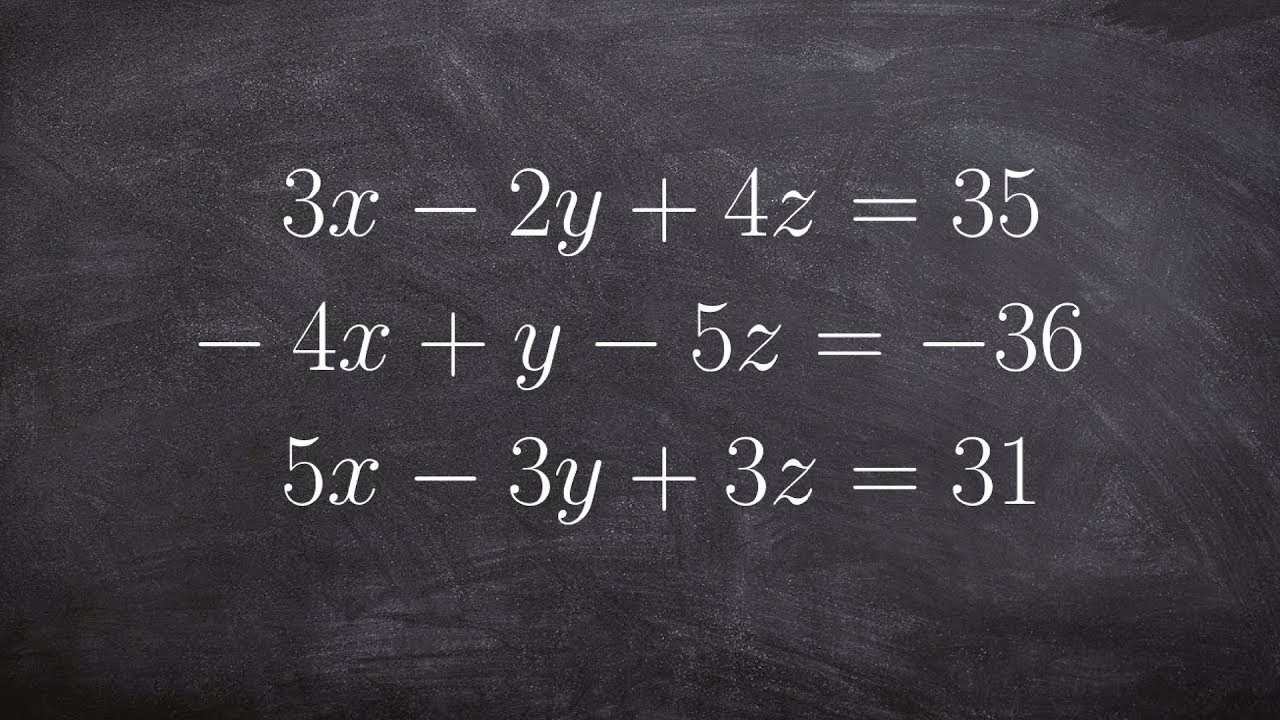
Solve a system with three variables
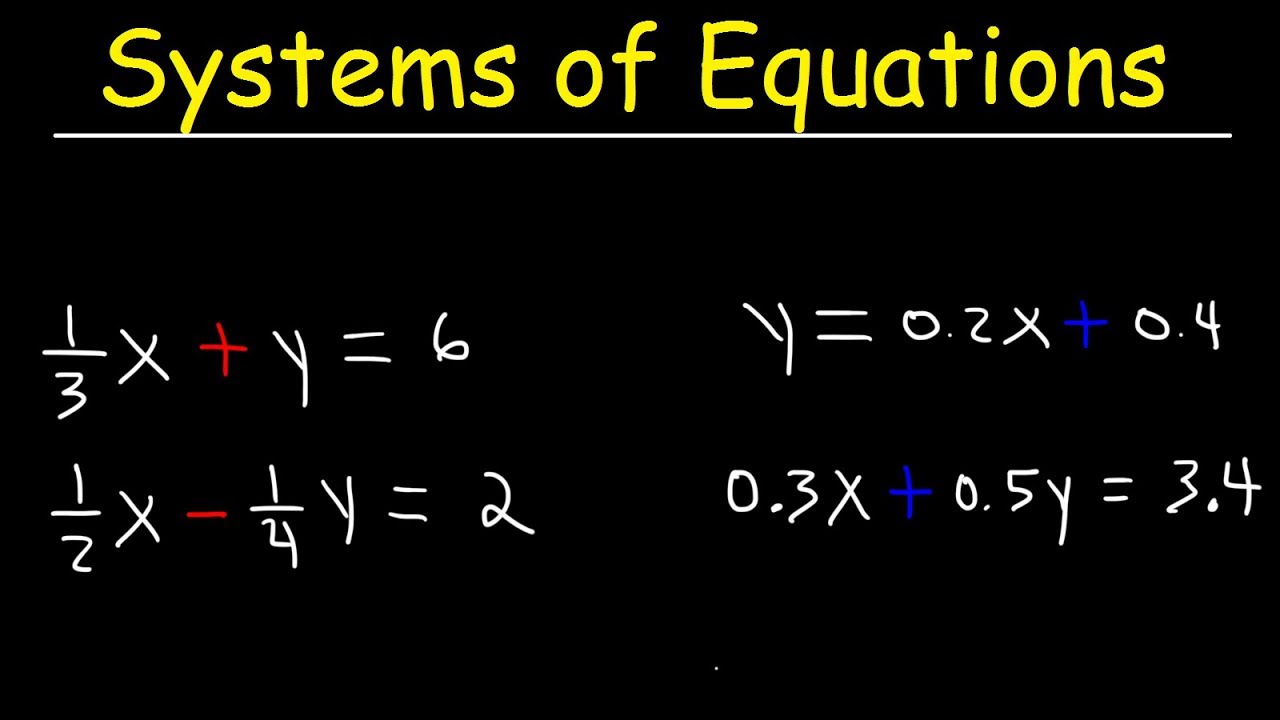
Solving Systems of Equations With Fractions and Decimals
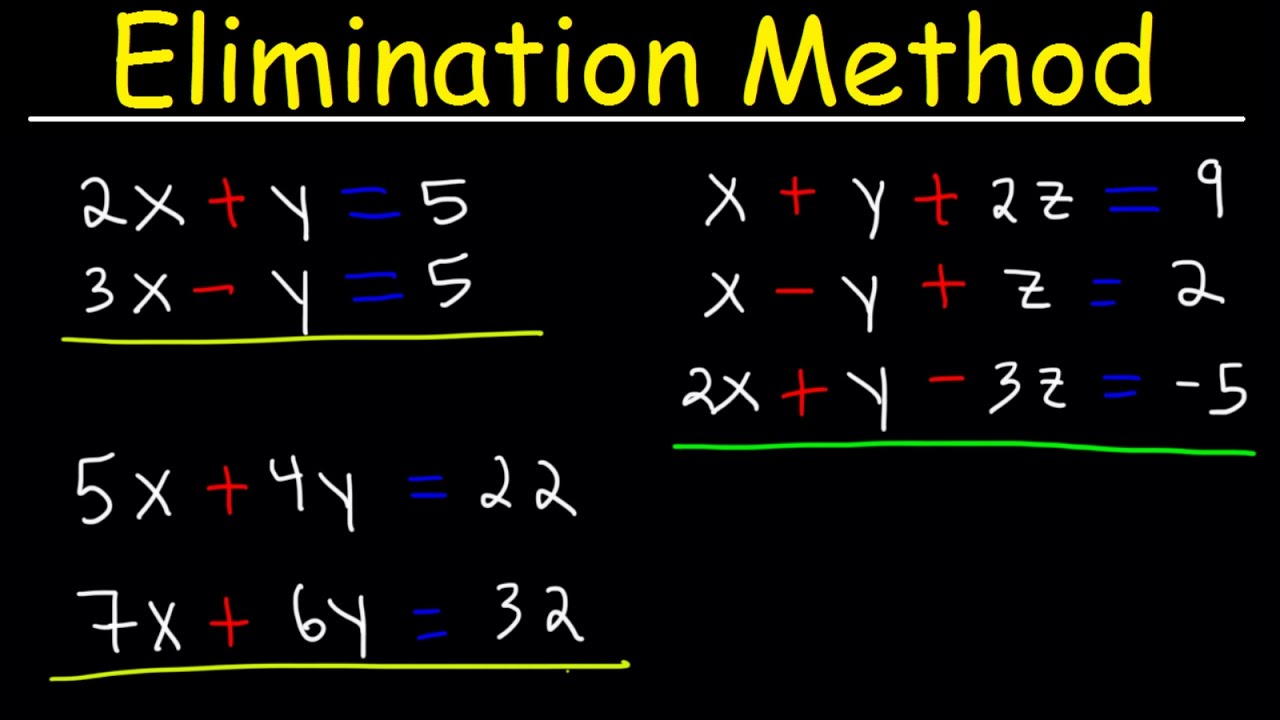
Elimination Method For Solving Systems of Linear Equations Using Addition and Multiplication, Algebr
5.0 / 5 (0 votes)
Thanks for rating: