Solving Polynomial Equations By Factoring and Using Synthetic Division

The Organic Chemistry Tutor
14 Feb 201814:19
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial offers a comprehensive guide on solving polynomial equations through various methods. It begins with factoring by grouping, demonstrating the solution to a cubic equation using the common ratio of coefficients. The video then covers solving a quartic equation by substitution, transforming it into a quadratic form and factoring it. The rational zero theorem is introduced for finding potential rational zeros, followed by synthetic division to determine the remaining zeros. The script also tackles a quartic equation with no straightforward factoring, showcasing the step-by-step process of applying the theorem and division to find all solutions. Finally, the tutorial concludes with a summary of the three methods: factoring by grouping, substitution, and the rational zero theorem with synthetic division, providing viewers with a solid foundation in polynomial equation solving.

Takeaways
  • 📚 The tutorial focuses on solving polynomial equations using various methods.
  • 🔍 Factoring by grouping is a quick method when the ratios of coefficients are the same.
  • 🧩 In the equation x^3 + 3x^2 - 4x - 12 = 0, the ratio of the coefficients of the first two terms matches the last two, allowing for factoring by grouping.
  • 📉 The greatest common factor (GCF) is used to simplify terms before factoring.
  • 📈 The difference of squares can be used to factor expressions like x^2 - 4.
  • 🔢 Rational zeros can be found using the rational root theorem and synthetic division to find remaining zeros.
  • 🔑 The substitution method can reduce a polynomial to a quadratic form for easier factoring.
  • 📝 The script demonstrates solving the equation x^4 - 5x^2 - 36 = 0 by substitution and finding both real and imaginary solutions.
  • 🔍 The rational zero theorem is used to list possible rational zeros and test them in the polynomial.
  • 📉 Synthetic division is a tool to simplify the process of finding zeros after identifying one zero.
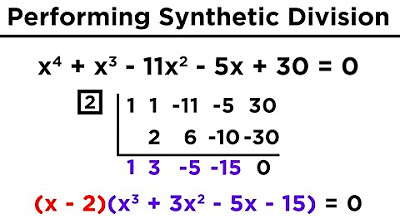
  • 📊 The tutorial covers solving higher degree polynomials like x^4 - 7x^3 + 7x^2 + 35x - 60 = 0 using a combination of the rational zero theorem and synthetic division.
Q & A

  • What is the first step in solving the given polynomial equation x^3 + 3x^2 - 4x - 12 = 0?

    -The first step is to notice that the ratio of the first two coefficients (1:3) is the same as the ratio of the last two coefficients (-4:-12). This indicates that factoring by grouping might be possible.

  • How does the greatest common factor (GCF) help in factoring the polynomial x^3 + 3x^2 - 4x - 12?

    -The GCF, which is x^2 for the first two terms and -4 for the last two terms, is used to group and simplify the polynomial. This allows us to factor out x + 3, leading to x^2 - 4, which is a difference of squares and can be further factored.

  • What are the solutions to the polynomial equation x^3 + 3x^2 - 4x - 12 = 0 after factoring?

    -The solutions are x = -3, x = -2, and x = 2. These are found by setting each factor equal to zero and solving for x.

  • How is the polynomial equation x^4 - 5x^2 - 36 equal to zero reducible to a quadratic form?

    -By substituting a = x^2, the equation becomes a^2 - 5a - 36 = 0, which is a quadratic form that can be factored using standard quadratic factoring techniques.

  • What are the solutions to the polynomial equation x^4 - 5x^2 - 36 = 0?

    -The solutions are x = ±3, x = ±2i. There are two real solutions (x = ±3) and two imaginary solutions (x = ±2i).

  • Why can't the polynomial x^3 - 3x^2 - 6x + 8 be factored by grouping?

    -The polynomial cannot be factored by grouping because the ratios of the coefficients of the first two terms (1:-3) do not match the ratios of the last two terms (-6:8), which simplifies to -4/3.

  • What is the Rational Zero Theorem and how is it used to find possible rational zeros of a polynomial?

    -The Rational Zero Theorem states that any possible rational zero of a polynomial, with integer coefficients, can be expressed as the quotient of a factor of the constant term divided by a factor of the leading coefficient. It is used to list all possible rational zeros before applying synthetic division to find the actual zeros.

  • How is synthetic division used to find the remaining zeros of a polynomial after one zero is found?

    -Synthetic division is used to divide the polynomial by the factor corresponding to the found zero, which results in a polynomial of one degree lower. This process can then be repeated to find the remaining zeros.

  • What are the solutions to the polynomial equation x^4 - 7x^3 + 7x^2 + 35x - 60 = 0?

    -The solutions are x = 1, x = 3, x = 4, and x = -√5, x = √5. There are three real solutions and two complex solutions.

  • How does factoring by grouping work in the context of solving the polynomial x^4 - 7x^3 + 7x^2 + 35x - 60?

    -After finding one zero (x = 3) and reducing the polynomial using synthetic division, the resulting cubic polynomial can be factored by grouping. This involves factoring out common factors from groups of terms, leading to further factorization and the identification of additional zeros.

  • What are the key takeaways from this tutorial for solving polynomial equations?

    -The key takeaways are the methods of factoring by grouping, factoring by substitution, and using the Rational Zero Theorem along with synthetic division to solve polynomial equations. These techniques are crucial for finding the zeros of polynomials of various degrees.

Outlines
00:00
🔍 Solving Polynomial Equations by Factoring

This paragraph focuses on solving polynomial equations, specifically x^3 + 3x^2 - 4x - 12 = 0, by factoring by grouping. It explains how to identify common factors, take out the greatest common factor (GCF), and use the difference of perfect squares to completely factor the polynomial. The paragraph provides a step-by-step solution, resulting in the answers x = -3, x = -2, and x = 2.

05:01
🔄 Using Substitution to Solve Polynomial Equations

This section demonstrates solving the polynomial equation x^4 - 5x^2 - 36 = 0 by reducing it to a quadratic form using substitution. It introduces the substitution method where a = x^2 and then factors the resulting trinomial. The equation is factored into real and imaginary roots, resulting in the solutions x = ±3 and x = ±2i.

10:02
📊 Rational Zero Theorem and Synthetic Division

This part explains solving the polynomial equation x^3 - 3x^2 - 6x + 8 = 0 using the Rational Zero Theorem and synthetic division. It lists all possible rational zeros and tests them to find actual zeros. The method successfully identifies x = 1, x = 4, and x = -2 as the solutions. The process of synthetic division is detailed, along with factoring the resulting polynomial.

Mindmap
Keywords
💡Polynomial Equations
Polynomial equations are algebraic expressions that involve a variable raised to various powers, with coefficients that are constants. They are fundamental in mathematics and are used to model a wide range of phenomena. In the video, the main theme revolves around solving these equations, which is a critical skill in algebra. The script provides several examples of polynomial equations, such as 'x cubed plus 3x squared minus 4x minus 12 equals zero', and demonstrates different methods to solve them.
💡Factoring by Grouping
Factoring by grouping is a method used to simplify polynomial equations by grouping terms and factoring out common factors. It is a strategic approach that can simplify the process of finding roots of polynomials. In the script, this method is illustrated when solving 'x cubed plus 3x squared minus 4x minus 12 equals zero', where the polynomial is grouped and the greatest common factor is extracted to simplify the equation.
💡Greatest Common Factor (GCF)
The greatest common factor, or GCF, is the largest number that divides two or more numbers without leaving a remainder. In the context of the video, the GCF is used to simplify terms within a polynomial equation before further factoring. For instance, in the equation 'x cubed plus 3x squared minus 4x minus 12 equals zero', the GCF of the first two terms is x squared, and for the last two terms, it is -4.
💡Difference of Perfect Squares
A difference of perfect squares is a specific algebraic identity that states that the difference between the squares of two numbers can be factored into the product of the sum and difference of the two numbers. In the video, this concept is applied when factoring 'x squared minus four' into '(x + 2)(x - 2)', which simplifies the process of finding the roots of the polynomial.
💡Rational Zero Theorem
The rational zero theorem, also known as the rational root theorem, provides a way to find possible rational solutions to a polynomial equation. It states that any rational solution, expressed as a fraction p/q, must have p as a factor of the constant term and q as a factor of the leading coefficient. In the script, the theorem is used to list all possible rational zeros for the polynomial 'x cubed minus three x squared minus six x plus eight equals zero'.
💡Synthetic Division
Synthetic division is a simplified form of polynomial division used primarily to divide polynomials by binomials of the form (x - c). It is a quick method to find the quotient and remainder without performing long division. In the video, synthetic division is used after identifying a rational zero to find the remaining factors of the polynomial, as demonstrated with the polynomial 'x cubed minus three x squared minus six x plus eight equals zero'.
💡Quadratic Form
A quadratic form is a second-degree polynomial equation, which can often be manipulated to resemble the standard form of a quadratic equation, ax^2 + bx + c = 0. In the video, the script mentions reducing a higher-degree polynomial to a quadratic form to make it easier to factor, as seen in the equation 'x to the fourth minus 5x squared minus 36 equals zero'.
💡Imaginary Numbers
Imaginary numbers are a set of numbers that, when squared, give a negative result. They are represented by the symbol 'i', where i^2 = -1. In the context of solving polynomial equations, imaginary numbers can be solutions when the equation does not have real roots. The script discusses this in the example 'x to the fourth minus 5x squared minus 36 equals zero', where the solutions include 'x equals plus or minus 2i'.
💡Substitution
In mathematics, substitution is a method where one expression is replaced by another to simplify the problem or to make it easier to solve. In the script, substitution is used to solve the polynomial equation 'x to the fourth minus 5x squared minus 36 equals zero' by setting 'a' equal to 'x squared', thus transforming the equation into a more manageable form.
💡Roots of a Polynomial
The roots of a polynomial are the values of the variable for which the polynomial equals zero. Finding the roots is a primary goal when solving polynomial equations. The video script demonstrates various methods to find the roots of different polynomials, such as factoring and using the rational zero theorem, with examples including 'x cubed plus 3x squared minus 4x minus 12 equals zero' and 'x to the fourth power minus seven x cubed plus seven x squared plus thirty five x minus sixty equals zero'.
Highlights

Tutorial focuses on solving polynomial equations.

Introduction to solving x^3 + 3x^2 - 4x - 12 = 0 by recognizing coefficient ratios.

Factor by grouping technique is the fastest way to solve the equation.

Greatest common factor (GCF) is used to simplify terms.

Complete factorization leads to three solutions: x = -3, -2, 2.

Solving x^4 - 5x^2 - 36 = 0 using substitution method.

Reduction of a quartic equation to a quadratic form.

Factoring trinomial with a leading coefficient of one.

Introduction of imaginary numbers for factoring.

Four solutions for the quartic equation: x = ±3, ±2i.

Approach to solving x^3 - 3x^2 - 6x + 8 = 0 without factor by grouping.

Use of the rational zero theorem to list possible rational zeros.

Synthetic division to find other zeros after identifying one.

Three real solutions found: x = 4, -2, 1.

Solving x^4 - 7x^3 + 7x^2 + 35x - 60 using rational zero theorem.

Testing factors of the constant term for potential zeros.

Identification of x = 3 as a zero through synthetic division.

Further factorization and finding all four solutions: x = 4, -√5, √5.

Summary of solving polynomial equations by factoring and the rational zero theorem.

Transcripts

Browse More Related Video

Factoring Cubic Polynomials- Algebra 2 & Precalculus

Factoring Higher Degree Polynomial Functions & Equations - Algebra 2

How To Find The Real & Imaginary Solutions of Polynomial Equations

Finding All Zeros of a Polynomial Function Using The Rational Zero Theorem

Solving Higher-Degree Polynomials by Synthetic Division and the Rational Roots Test

How to Use the Rational Zeros Theorem (Precalculus - College Algebra 34)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Polynomial EquationsFactoring TechniquesRational Zero TheoremSynthetic DivisionAlgebra TutorialMath EducationProblem SolvingEducational ContentMathematicsLearning Resource