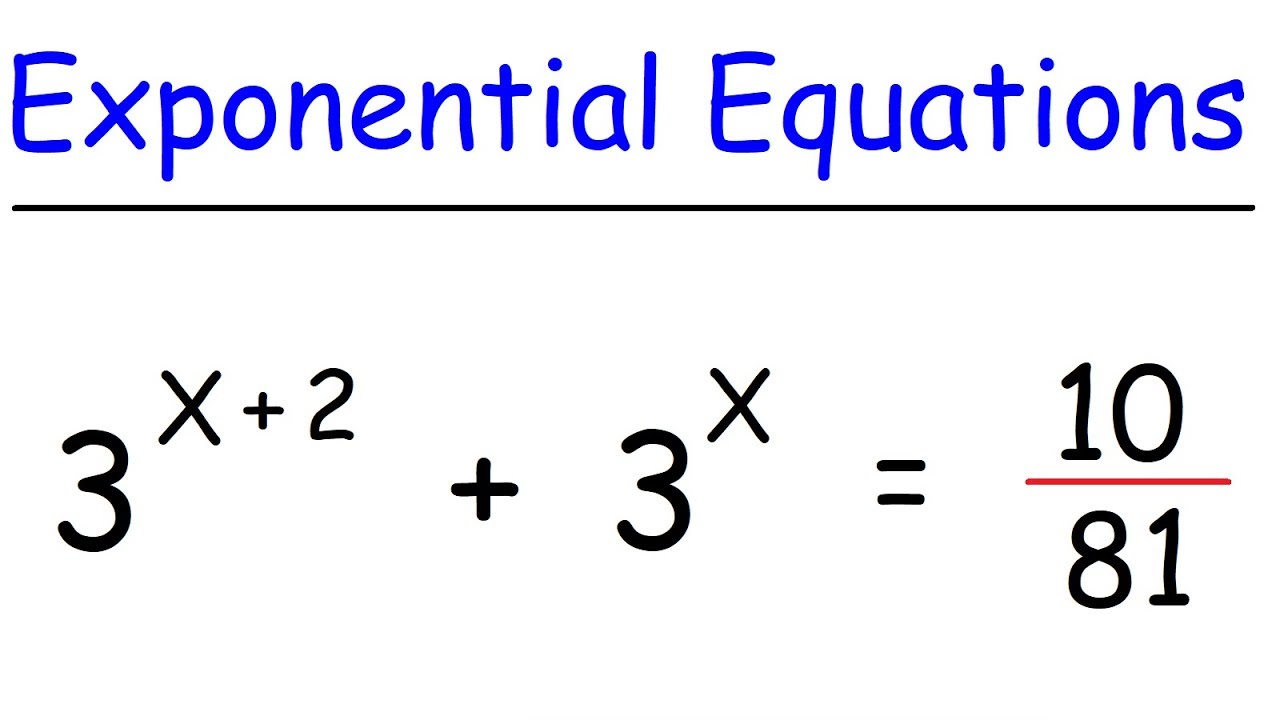Solving Exponential Equations Using Logarithms & The Quadratic Formula

The Organic Chemistry Tutor
23 Feb 202011:05
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script offers a step-by-step guide to solving an exponential equation, 2^x + 4^x = 8^x. It begins by simplifying the equation to a common base of 2, transforming it into 2^x + 2^(2x) = 2^(3x). The script then demonstrates how to isolate the terms and use substitution to form a quadratic equation. Applying the quadratic formula, two potential solutions for y are found, but only the positive one is valid due to the nature of exponential functions. The script concludes by using logarithms to solve for x, providing the exact and decimal values for the solution. The process is verified by substituting the solution back into the original equation, confirming its correctness.

Takeaways
  • πŸ” The video discusses solving an exponential equation of the form 2^x + 4^x = 8^x.
  • πŸ“ The bases 4 and 8 are multiples of 2, and the equation is simplified by expressing all terms with base 2.
  • πŸ“ The equation is rewritten using exponent rules: 4^x becomes (2^2)^x = 2^(2x), and 8^x becomes (2^3)^x = 2^(3x).
  • βž— The equation is rearranged to isolate terms on one side, resulting in 0 = 2^(3x) - 2^(2x) - 2^x.
  • 🧩 Each term is divided by 2^x to simplify the equation further, leading to 0 = 2^(2x) - 2^x - 1.
  • πŸ”„ The expression is recognized as a quadratic in terms of 2^x, and substitution is used to set y = 2^x.
  • πŸ”’ The quadratic equation y^2 - y - 1 = 0 is formed and solved using the quadratic formula.
  • πŸ“ˆ The quadratic formula yields two potential solutions for y, which are then evaluated for their decimal values.
  • ❌ The negative solution for y is discarded because 2^x cannot be negative.
  • πŸ”‘ The positive value of y is used to find x by taking logarithms, specifically log base 2.
  • πŸ“Š The final solution for x is obtained by dividing the logarithm of the positive y value by the logarithm of 2.
  • πŸ” The solution is verified by substituting the value of x back into the original equation to ensure it holds true.
Q & A

  • What is the exponential equation discussed in the video?

    -The exponential equation discussed in the video is 2^x + 4^x = 8^x.

  • Why are the bases of the equation multiples of two?

    -The bases 2, 4, and 8 are multiples of two, which allows for a common base to be used when simplifying the equation.

  • What is the common base used to simplify the equation?

    -The common base used to simplify the equation is 2, because 4 can be represented as 2^2 and 8 as 2^3.

  • How do you rewrite 4^x and 8^x using the common base 2?

    -4^x is rewritten as (2^2)^x = 2^(2x), and 8^x is rewritten as (2^3)^x = 2^(3x).

  • What is the next step after rewriting the equation with a common base?

    -The next step is to move all terms to one side of the equation to set it equal to zero and then divide every term by 2^x to simplify further.

  • What substitution is used to transform the equation into a quadratic form?

    -The substitution used is to let y = 2^x, transforming the equation into 0 = y^2 - y - 1.

  • Why is the quadratic formula necessary to solve the quadratic equation?

    -The quadratic formula is necessary because the quadratic equation y^2 - y - 1 cannot be factored easily.

  • What are the values of a, b, and c in the quadratic formula for the equation y^2 - y - 1?

    -In the quadratic formula, a = 1, b = -1, and c = -1 for the equation y^2 - y - 1.

  • What are the two possible values for y after applying the quadratic formula?

    -The two possible values for y are 1 + sqrt(5)/2 (approximately 1.618034), which is the golden ratio, and 1 - sqrt(5)/2 (approximately -0.618034).

  • Why can't 2^x be equal to a negative number?

    -2^x cannot be equal to a negative number because any positive number raised to a power will always result in a positive number.

  • How is the value of x found after determining the value of y?

    -The value of x is found by taking the logarithm of both sides of the equation with base 2, isolating x by dividing by log(2).

  • What is the final step to ensure the correctness of the solution?

    -The final step is to plug the found value of x back into the original exponential equation to check if both sides of the equation are equal.

  • What is the approximate decimal value of x after solving the logarithmic equation?

    -The approximate decimal value of x is 0.694242.

Outlines
00:00
πŸ§‘β€πŸ« Solving Exponential Equations with Common Bases

In this educational segment, the video explains how to solve an exponential equation where the bases are multiples of each other. The equation given is 2^x + 4^x = 8^x. The first step is to recognize that 4 and 8 can be expressed as powers of 2, simplifying the equation to a common base. By rewriting the equation with base 2, we get 2^x + 2^(2x) = 2^(3x). The next step involves isolating terms and simplifying the equation to 0 = 2^(3x) - 2^(2x) - 2^x. Dividing through by 2^x yields x^3 - x^2 - 1 = 0. The video then introduces substitution, setting y = 2^x, transforming the equation into a quadratic form y^2 - y - 1 = 0. The quadratic formula is applied to find the possible values of y, resulting in two solutions, one of which is discarded due to the nature of exponential functions not being negative. The remaining solution is then used to solve for x using logarithms, leading to the exact value of x being the logarithm of the golden ratio divided by the logarithm of 2.

05:02
πŸ” Applying the Quadratic Formula and Logarithms

This part of the video script delves into the application of the quadratic formula to solve for y in the equation y^2 - y - 1 = 0. The formula is y = (-b ± √(b^2 - 4ac)) / (2a), where a, b, and c are coefficients from the quadratic equation in the form ay^2 + by + c. Here, a=1, b=-1, and c=-1, leading to y = 1 ± √5 / 2. The two potential solutions for y are calculated, yielding approximately 1.618034 (the golden ratio) and -0.618034. Since an exponential function cannot have a negative result, the negative solution is discarded. The positive solution is then used to express 2^x in terms of the golden ratio. To isolate x, logarithms are employed, specifically the base 2 logarithm of both sides of the equation, resulting in x = log((1 + √5) / 2) / log(2). The decimal approximation of x is calculated to be approximately 0.694242. The video concludes with a verification step, plugging the value of x back into the original equation to confirm its correctness.

10:05
πŸ“ Verifying the Solution to the Exponential Equation

The final part of the video script is dedicated to verifying the solution obtained for the exponential equation. By substituting x = 0.694242 back into the original equation, the video demonstrates that 2^x, 4^x, and 8^x yield values that sum up correctly to 8^x, confirming the accuracy of the solution. The verification process involves calculating the exponential values for the base of 2 raised to the power of the solution x, and showing that when these values are summed, they match the right side of the original equation, thus validating the solution.

Mindmap
Keywords
πŸ’‘Exponential Equation
An exponential equation is a mathematical equation involving a variable in the exponent. In the video, the equation is 2^x + 4^x = 8^x, which is the central problem being solved. It is a key concept because the entire video is dedicated to finding the value of x that satisfies this equation.
πŸ’‘Common Base
A common base in the context of exponential equations refers to expressing all terms with the same base to simplify the equation. In the video, the bases of 4 and 8 are replaced with 2^2 and 2^3 respectively, to create a common base of 2, which is crucial for solving the equation.
πŸ’‘Exponentiation
Exponentiation is the operation of raising a number to the power of another number. In the video, it is used to express the bases 4 and 8 as powers of 2, and to transform the equation into a form that can be solved. For example, 4^x is rewritten as (2^2)^x, which simplifies to 2^(2x).
πŸ’‘Subtracting Exponents
Subtracting exponents is a process used when dividing terms with the same base. In the video, this concept is applied to simplify the equation by subtracting the exponents when dividing 2^(3x) by 2^x, resulting in 2^(2x).
πŸ’‘Quadratic Equation
A quadratic equation is a polynomial equation of the second degree, typically in the form of ax^2 + bx + c = 0. After rearranging the original exponential equation, the video presents a quadratic equation in terms of y, which is 0 = y^2 - y - 1, and this is solved using the quadratic formula.
πŸ’‘Quadratic Formula
The quadratic formula is used to solve quadratic equations and is given by y = (-b ± √(b^2 - 4ac)) / (2a). In the video, it is applied to find the values of y, which are then used to determine the possible values of x in the original exponential equation.
πŸ’‘Substitution
Substitution is a mathematical technique where a complex expression is replaced by a simpler variable to simplify the problem. In the video, y is substituted for 2^x to transform the exponential equation into a quadratic equation, which is easier to solve.
πŸ’‘Logarithms
Logarithms are the inverse operation to exponentiation and are used to solve for the exponent in an exponential equation. In the video, logarithms are applied to isolate x by taking the log of both sides of the equation, which allows the exponent x to be moved to the front.
πŸ’‘Golden Ratio
The golden ratio, often denoted by the Greek letter Ο† (phi), is a mathematical constant approximately equal to 1.618. In the video, one of the solutions for y is found to be related to the golden ratio, which is a significant discovery in the context of the equation being solved.
πŸ’‘Logarithmic Properties
Logarithmic properties are rules that govern the behavior of logarithms, such as the ability to move the exponent from its position in an exponent to a coefficient position. In the video, these properties are used to simplify the logarithmic equation and solve for x.
πŸ’‘Checking the Solution
Checking the solution involves substituting the found value(s) of the variable back into the original equation to verify that it is correct. In the video, the calculated value of x is plugged back into the exponential equation to ensure that it is indeed a valid solution.
Highlights

Introduction to solving an exponential equation with a base of 2.

Equation presented: 2^x + 4^x = 8^x.

Observation that all bases are multiples of two.

Transformation of the equation to a common base of 2.

Explanation of exponent rules for simplifying the equation.

Rewriting the equation with a common base and simplified exponents.

Isolating terms and setting up the equation for further simplification.

Division of each term by 2^x to simplify the equation.

Introduction of substitution method with y = 2^x.

Transformation of the equation into a quadratic form.

Application of the quadratic formula to solve for y.

Identification of coefficients a, b, and c for the quadratic equation.

Calculation of the two possible values for y using the quadratic formula.

Elimination of the negative solution for y due to the nature of exponential functions.

Use of logarithms to isolate x in the exponential equation.

Final solution for x using logarithmic properties.

Verification of the solution by substituting it back into the original equation.

Conclusion on the method to solve exponential equations of this form.

Transcripts

Browse More Related Video

Exponential Equations - Algebra and Precalculus

Solving Quadratic Equations With Exponents

Logarithmic Equations

Solving Exponential Equations In Quadratic Form - Using Logarithms, With e

Solving Systems of Equations By Graphing

Exponential Logarithmic Equations

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Exponential EquationsMath TutorialCommon BaseAlgebraic TechniquesEducational ContentProblem SolvingLogarithmsQuadratic FormulaSubstitution MethodMathematics Learning