Average Rate of Change of a Function (Precalculus - College Algebra 11)

Professor Leonard
6 Nov 201910:32
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the concept of the average rate of change of a function, a fundamental topic in calculus. It explains that the average rate of change is essentially the slope between two points on a curve, often referred to as the secant line. The presenter clarifies that while the instantaneous rate of change, or the slope of the tangent line at a specific point on the curve, can be found using calculus, the average rate of change provides an approximation by considering the slope of a line connecting two points on the curve. The script illustrates this by showing how to calculate the average rate of change for a given function, using the formula (f(b) - f(a)) / (b - a), where f represents the function, and a and b are two x-values. The video aims to demystify the concept and connect it to the broader understanding of calculus, emphasizing the importance of understanding the average rate of change as a stepping stone to more advanced calculus concepts.

Takeaways
  • 📈 The average rate of change of a function is essentially the slope between two points on a curve, often referred to as the secant line.
  • 🔍 Calculus allows us to find the slope of a curve at a specific point, which is an extension of the concept of average rate of change.
  • 🤔 The main question addressed is whether one can find the slope of a curve at a point, which calculus helps to answer.
  • 📌 As you get the points on the curve closer together, the slope of the secant line approaches the slope of the tangent line at that point.
  • 👉 The formula for average rate of change is similar to the slope formula, represented as (f(b) - f(a)) / (b - a), where f represents the function's output.
  • 🧮 To find the average rate of change, you calculate the change in the function's output values (y2 - y1) over the change in input values (x2 - x1).
  • 📍 You can find the average rate of change for any function given two x-values by plugging them into the function to find the corresponding y-values.
  • 🤓 The process involves finding two points on the curve, then applying the slope formula to these points to calculate the average rate of change.
  • 💡 The concept of average rate of change is foundational and leads to the idea of instantaneous rate of change, which is a core concept in calculus.
  • 📊 The equation of the secant line can be written using the point-slope form, Y - y1 = M(x - x1), where M is the average rate of change and (x1, y1) is a point on the line.
  • 📖 Understanding average rate of change is important as it sets the stage for more advanced calculus concepts, such as derivatives and the slope of the tangent line.
Q & A

  • What is the average rate of change of a function?

    -The average rate of change of a function is the slope between two points on a curve, often referred to as the secant line between those points.

  • Why is it important to learn about the average rate of change?

    -It is important because it serves as a foundation for understanding calculus concepts, particularly the slope of a curve at a specific point, which is the tangent line.

  • What is the formula for calculating the average rate of change?

    -The formula for calculating the average rate of change is (f(b) - f(a)) / (b - a), where f(a) and f(b) are the outputs of the function at inputs a and b, respectively.

  • How does the concept of the average rate of change lead to the idea of the instantaneous rate of change in calculus?

    -As the points a and b get closer together, the slope of the secant line approaches the slope of the tangent line at point a. In calculus, this is formalized as the derivative, which gives the instantaneous rate of change at a point.

  • What is the relationship between the average rate of change and the slope of a line?

    -The average rate of change is essentially the slope of a secant line connecting two points on a curve. It represents the average change in the output values over the change in the input values between those two points.

  • How do you find the average rate of change for a given function between two specific x-values?

    -You first find the corresponding y-values (outputs) for the given x-values by plugging them into the function. Then you apply the average rate of change formula using these values.

  • What is the significance of the secant line in understanding the average rate of change?

    -The secant line represents the average rate of change between two points on a curve. It is a linear approximation that helps in understanding how the function's rate of change varies over an interval.

  • Can you find the slope of a curve at a single point using the average rate of change?

    -No, the average rate of change gives the slope between two points. However, in calculus, the concept of the derivative allows you to find the instantaneous rate of change, which is the slope of the curve at a single point.

  • What is the equation of a secant line that intersects a curve at two points?

    -The equation of a secant line is given by Y - y1 = M(X - x1), where M is the slope of the line (average rate of change) and (x1, y1) is one of the points on the curve.

  • How does the process of finding the average rate of change help in the study of calculus?

    -Finding the average rate of change is a stepping stone to understanding more advanced calculus concepts. It introduces the idea of approximating the rate of change over an interval, which leads to the concept of the derivative for instantaneous rate of change.

  • What is the practical application of understanding the average rate of change?

    -Understanding the average rate of change is useful in various real-world scenarios where one needs to determine how a quantity changes over time or space, such as calculating average speed, growth rates, or the rate of return on investments.

Outlines
00:00
🔍 Understanding Average Rate of Change

This section introduces the concept of the average rate of change in mathematics, particularly as it relates to calculus. The presenter emphasizes the importance of understanding this concept beyond just memorizing formulas. They explain that the average rate of change is similar to calculating the slope between two points on a curve, which is referred to as a secant line. However, unlike simple slopes, this involves complex functions where direct slope calculation at a point is not possible. The presenter sets up the concept of approaching the instantaneous rate of change, using calculus, by making points on the secant line infinitesimally close, leading into the derivative concept.

05:03
📉 Average Rate of Change vs. Slope

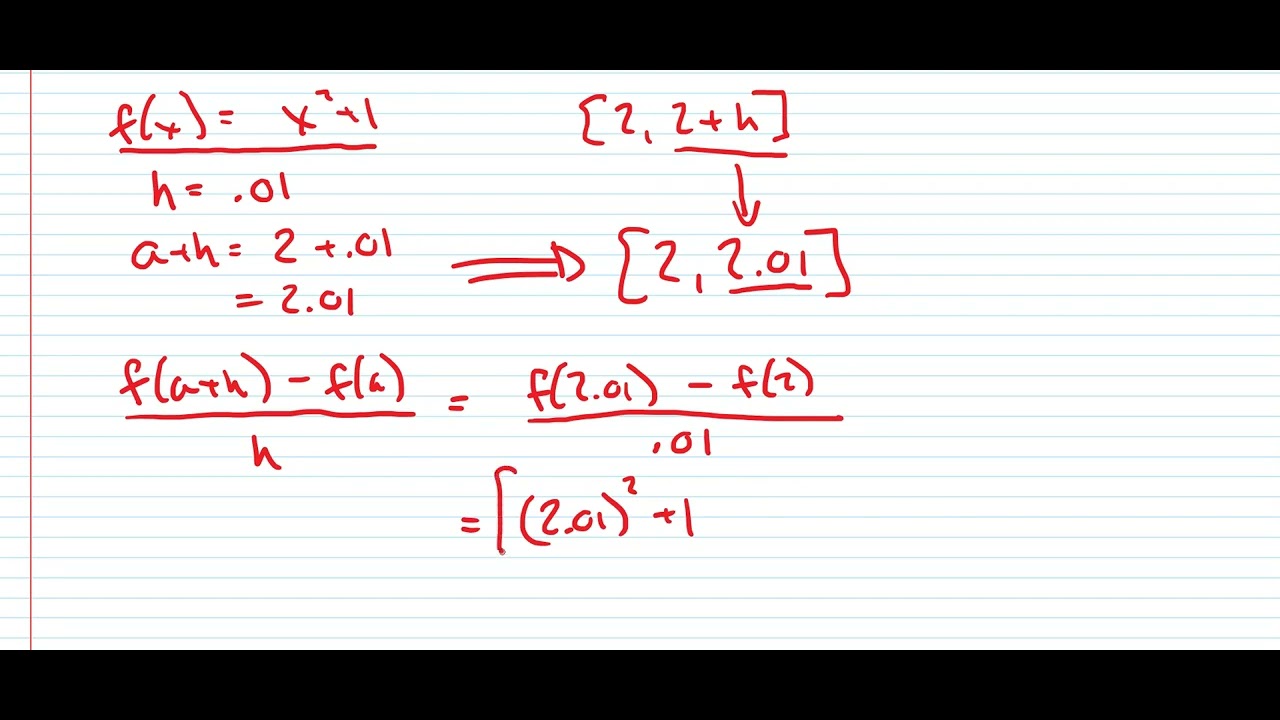
This section delves deeper into the average rate of change, illustrating it with a specific example involving a parabolic function. The presenter explains how to calculate the average rate of change by selecting two points on the function and using the slope formula. They illustrate this using a hypothetical function with points where x equals 3 and 5, calculating the outputs for these inputs and then using these values to determine the slope of the secant line. This process is described as a fundamental application of the slope formula in a way that is specific to calculus, preparing the learner for more complex concepts like derivatives.

10:04
📚 Why Learn Average Rate of Change?

In the final section, the presenter explains why learning about the average rate of change is crucial for understanding calculus. They emphasize that this foundational concept is not just a fancy version of the slope formula but a stepping stone towards understanding how rates of change work in calculus. The section aims to motivate learners to appreciate these basics as they prepare for more advanced topics in calculus, highlighting the practical progression from average to instantaneous rates of change.

Mindmap
Keywords
💡Average Rate of Change
The average rate of change is a concept in calculus that describes the rate at which a function changes over an interval. It is defined as the difference in the function's output values (y-values) divided by the difference in the input values (x-values) between two points on the function's graph. In the video, this concept is central to understanding how the slope of a curve can be determined between two points, which is crucial for later discussions on calculus and the concept of the derivative.
💡Secant Line
A secant line is a straight line that intersects a curve at two or more points. In the context of the video, the secant line is used to approximate the slope of a curve between two points. It is a fundamental tool for understanding the average rate of change because the slope of the secant line represents the average rate at which the function changes over the interval between the two points. The video uses the secant line to demonstrate how one can find the average rate of change of a function.
💡Slope
Slope is a measure of the steepness of a line, and it is calculated as the change in the y-values divided by the change in the x-values between two points on the line. In the video, the concept of slope is used to explain the average rate of change of a function. The slope of the secant line, which connects two points on a curve, is used to find the average rate of change between those points. The video emphasizes that the average rate of change is essentially the slope of a secant line.
💡Function
A function is a mathematical relationship that assigns to each element from a set of inputs (called the domain) exactly one output from a set of permissible outputs (called the codomain). In the video, the function is represented by a curve on a graph, and the task is to find the average rate of change of this function over a certain interval. The function is used to generate points on the curve, which are then used to calculate the average rate of change.
💡Calculus
Calculus is a branch of mathematics that deals with the study of change and motion. It is mentioned in the video as the broader mathematical context in which the concept of the average rate of change is used. The video explains that while the average rate of change is an introductory concept, calculus allows for the refinement of this idea to find the instantaneous rate of change at a specific point on a curve, which is represented by the slope of the tangent line at that point.
💡Tangent Line
A tangent line is a line that touches a curve at a single point and has the same slope as the curve at that point. In the video, the concept of the tangent line is introduced as a more precise way to find the slope of a curve at a specific point, which is a concept developed further in calculus. The video explains that as the points used to draw the secant line get closer together, the slope of the secant line approaches the slope of the tangent line at the point of interest.
💡Instantaneous Rate of Change
The instantaneous rate of change refers to the rate of change of a function at a specific point, as opposed to an average rate of change over an interval. It is a concept that is introduced in the video as a goal of calculus. The video explains that by taking the limit as the interval between two points on a curve approaches zero, one can find the instantaneous rate of change, which is the slope of the tangent line to the curve at that point.
💡Derivative
In calculus, the derivative of a function at a point is the slope of the tangent line to the graph of the function at that point. The video mentions the derivative as a concept that is used to find the instantaneous rate of change of a function. It is the mathematical tool that allows one to refine the average rate of change to the precise rate of change at a specific point on a curve.
💡Input/Output
In the context of functions, input refers to the independent variable (usually denoted by x), and output refers to the dependent variable (usually denoted by y or as f(x), which represents the function evaluated at x). The video uses the terms input and output to describe the coordinates of points on the graph of a function. The average rate of change is calculated by considering the change in outputs over the change in inputs between two points on the graph.
💡Graph
A graph is a visual representation of a function where the x-values are plotted on a horizontal axis and the y-values are plotted on a vertical axis. In the video, the graph is used to visualize the curve of a function and to identify points between which the average rate of change is to be calculated. The graph is essential for understanding how the secant line and the tangent line relate to the curve of the function.
💡Limit
In calculus, a limit is a value that a function or sequence approaches as the input (or index) approaches some value or infinity. The concept of a limit is alluded to in the video when discussing how the average rate of change can be refined to find the instantaneous rate of change by making the interval between two points on the curve arbitrarily small, effectively taking a limit.
Highlights

The video discusses the concept of the average rate of change of a function, which is important in understanding calculus.

The average rate of change is the slope between two points on a curve, also known as a secant line.

Finding the slope of a curve at a single point is challenging, but the average rate of change between two points is more accessible.

The concept of the average rate of change is foundational for later understanding of the instantaneous rate of change in calculus.

The slope of the secant line can approximate the slope of the tangent line as the points get closer together.

The formula for the average rate of change is derived from the slope formula, which is (y2 - y1) / (x2 - x1).

The average rate of change can be found for any function by using two input values and the corresponding outputs.

To find the average rate of change, you calculate the outputs for given inputs and then apply the slope formula.

The average rate of change is essentially the slope of the secant line connecting two points on the curve.

The video provides a clear example of finding the average rate of change for a parabola function.

The process of finding the average rate of change involves plugging in x-values into the function to get the corresponding y-values.

The average rate of change is a way to understand the function's change in slope on average between two points.

The video explains how to find the equation of the secant line using the average rate of change as the slope.

The average rate of change is a fundamental concept that leads to more advanced calculus topics such as the derivative.

The video emphasizes the importance of understanding the average rate of change as a stepping stone to learning calculus.

The average rate of change is calculated by subtracting outputs and inputs in a specific order to get the slope formula.

The video concludes by reinforcing the idea that the average rate of change is the slope of a secant line, which is a basic concept in calculus.

Transcripts

Browse More Related Video

Math 11 - Sections 1.3-1.4

Business Calculus - Math 1329 - Section 2.1 - The Derivative

1.3 - Average Rates of Change

Average Rate of Change of a Function Over an Interval

Introduction to the Difference Quotient

Introduction to Average Rate of Change

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusRate of ChangeSlopeSecant LineTangent LineMathematicsLearningEducationDerivativeFunction AnalysisInstantaneous Rate