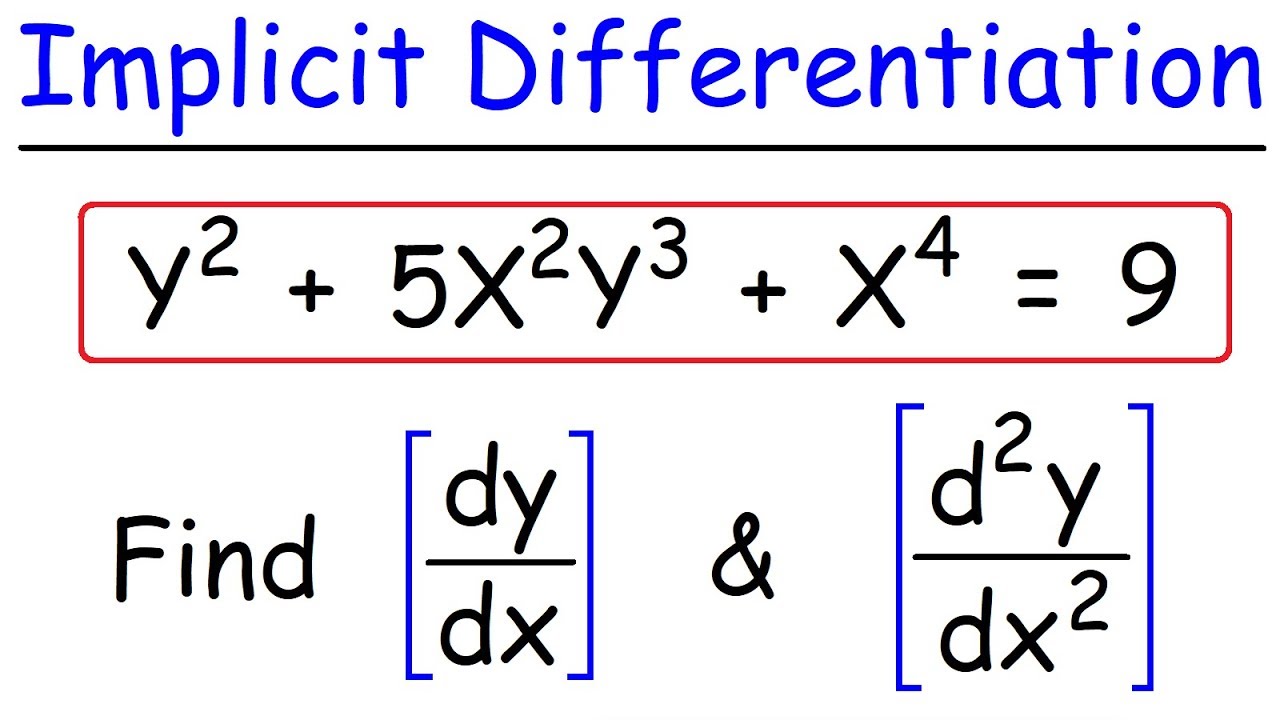
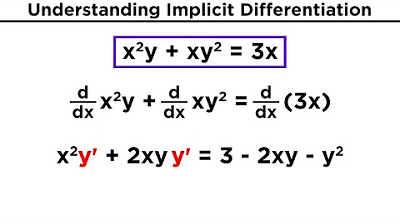
Implicit Differentiation Explained - Product Rule, Quotient & Chain Rule - Calculus
TLDRThis video tutorial provides a comprehensive guide on implicit differentiation, a technique used in calculus to find the derivative of a function without explicitly solving for the variable. It covers the process of differentiating both sides of an equation with respect to a variable, using the product rule for terms involving the variable, and isolating the derivative to solve for it. The video includes several examples, demonstrating how to handle constants, combine like terms, and apply the quotient rule for second derivatives. By the end, viewers should have a solid understanding of implicit differentiation and its applications in solving complex mathematical problems.
Takeaways
- 📚 Implicit differentiation is used to find the derivative of a function when the equation is not explicitly solved for y.
- 🔢 For the equation x^3 + y^3 = 8, the derivative dy/dx is calculated as -x^2/y^2 by differentiating both sides with respect to x and isolating dy/dx.
- 🌟 When differentiating a product, the product rule is applied, differentiating one part and keeping the other constant.
- 📈 In the equation x^2 + 2xy + y^2 = 5, the term 2xy requires the product rule, resulting in dy/dx = -1 after simplification.
- 🤝 For the equation 5xy - y^3 = 8, the terms are separated and differentiated individually, leading to dy/dx = -5y / (5x - 3y^2).
- 🔄 When dealing with trigonometric functions like tangent, the derivative (secant squared) is used, and the chain rule is applied to differentiate the inner function.
- 📊 For the equation √(x^2 + y^2) = 36, squaring both sides simplifies the process, and the derivative dy/dx is found as -x/y.
- 📌 When finding the second derivative, the quotient rule is used, which is expressed as (v * u' - u * v') / v^2, where v and u are the respective parts of the fraction.
- 🔢 For the second derivative of the function dy/dx = -x/y, the quotient rule results in d^2y/dx^2 = -x^2/y^2 after plugging in the first derivative.
- 📍 The process of implicit differentiation involves differentiating both sides of an equation with respect to x and isolating dy/dx to find the derivative.
- 🎯 Practice is essential for mastering implicit differentiation, as demonstrated by the various examples provided in the script.
Q & A
What is implicit differentiation?
-Implicit differentiation is a method used to find the derivative of a function when the variable of interest is not isolated on one side of an equation. It involves differentiating both sides of the equation with respect to the variable you are differentiating with respect to, while keeping in mind the implicit relationship between the variables.
How do you differentiate a constant with respect to a variable in implicit differentiation?
-The derivative of a constant with respect to any variable is zero. This is because a constant does not change with respect to the variable you are differentiating with respect to.
What is the product rule used for in implicit differentiation?
-The product rule is used in implicit differentiation when you have a term in the equation that is the product of two factors, one of which is the variable you are differentiating with respect to. The product rule states that you differentiate each factor separately and then add the results. In implicit differentiation, you also include the term dy/dx when differentiating the variable part of the product.
How do you isolate dy/dx in an equation?
-To isolate dy/dx in an equation, you need to move all terms that do not have the derivative dy/dx to one side of the equation and all terms that include dy/dx to the other side. After doing this, you simplify the equation to solve for dy/dx.
What is the quotient rule used for in finding the second derivative?
-The quotient rule is used for finding the derivative of a fraction. When you have a fraction where the numerator and the denominator are both functions of the variable you are differentiating with respect to, the quotient rule allows you to find the derivative of the entire fraction by using the formula: (v * u' - u * v') / v^2, where u is the numerator, v is the denominator, u' is the derivative of the numerator, and v' is the derivative of the denominator.
What is the derivative of the tangent function?
-The derivative of the tangent function is the secant function squared. This means that if you have a function that involves the tangent of x, you differentiate it keeping the inside of the tangent function (which is x) the same, and then apply the chain rule to find the derivative of the outer function, which in this case is the secant squared.
How do you simplify the equation √(x^2 + y^2) = 36 before differentiation?
-Before differentiating the equation √(x^2 + y^2) = 36, you can square both sides of the equation to eliminate the square root. This simplifies the equation to x^2 + y^2 = 36^2, which is x^2 + y^2 = 1369. This makes it easier to differentiate with respect to x since the square root is removed and the equation is now a simple sum of squares.
What is the second derivative of the function dy/dx = -x/y?
-To find the second derivative of the function dy/dx = -x/y, you first apply the quotient rule. The second derivative, denoted as d^2y/dx^2, is calculated as (v * u' - u * v') / v^2, where u is -x (the numerator), v is y (the denominator), u' is -1 (the derivative of -x), and v' is 1 (the derivative of y). Plugging these values in, the second derivative is y(-1) - (-x)(1) / y^2, which simplifies to -y + x/y^2. After simplifying further, you get the second derivative as -x^2 / (y^3).
What is the method to solve the equation x^3 + y^3 = 8 for dy/dx?
-To solve the equation x^3 + y^3 = 8 for dy/dx, you first differentiate both sides of the equation with respect to x. The derivative of x^3 is 3x^2, and the derivative of y^3 is 3y^2 multiplied by dy/dx. After differentiating, you get 3x^2 + 3y^2(dy/dx) = 0. Since the derivative of the constant 8 is zero, it disappears from the equation. Next, you isolate dy/dx by moving the 3x^2 term to the other side of the equation, which gives you dy/dx = -x^2/y^2. This is the derivative of y with respect to x for the given function.
How do you differentiate the equation x^2 + 2xy + y^2 = 5?
-To differentiate the equation x^2 + 2xy + y^2 = 5, you apply the product rule to the term 2xy. The derivative of x^2 is 2x. For 2xy, you differentiate 2x (which becomes 2) and keep y the same, then differentiate y (which is 1) and multiply by dy/dx. The derivative of y^2 is 2y, and you multiply this by dy/dx as well. After differentiating, you get 2x + 2y(dy/dx) + 2y(dy/dx) = 0. Now, you isolate dy/dx by moving all terms without dy/dx to the other side of the equation, which gives you 2x(dy/dx) + 2y(dy/dx) = -2x - 2y. Dividing everything by 2, and then factoring out dy/dx, you get dy/dx(x + y) = -x - y. Finally, dividing both sides by (x + y), you find that dy/dx = -1.
What is the process for differentiating the equation 5xy - y^3 = 8?
-To differentiate the equation 5xy - y^3 = 8, you first identify the two parts of the equation: 5x and y. For 5x, the derivative is simply 5, as it is a constant multiple of x. For y, you differentiate it normally, which gives you 1, and then apply the chain rule by multiplying by dy/dx. This gives you 5(dy/dx) for the first part and -3y^2(dy/dx) for the second part. The derivative of the constant 8 is zero, so it does not appear in the differentiated equation. Now, you have 5(dy/dx) - 3y^2(dy/dx) = -8. To solve for dy/dx, you factor out dy/dx, which gives you dy/dx(5x - 3y^2) = -8. Finally, dividing both sides by (5x - 3y^2), you get dy/dx = -8 / (5x - 3y^2).
What is the derivative of the equation secant(x*y) = 7?
-To find the derivative of the equation secant(x*y) = 7, you first need to differentiate both sides with respect to x. The derivative of secant is the square of the secant function, which means the derivative of secant(x*y) is (secant(x*y))^2. Applying the product rule, you differentiate x and keep y constant, and then differentiate y and keep x constant (multiplied by dy/dx). This gives you (x * secant(x*y)) * (1) + (y * secant(x*y)) * (dy/dx). Since the secant squared is a constant (7^2), its derivative is zero. Now, you isolate dy/dx by moving the x term to the other side, which gives you (y * secant(x*y) * dy/dx) = -x * secant(x*y). Dividing both sides by (x * secant(x*y)), you find that dy/dx = -y/x.
Outlines
📚 Introduction to Implicit Differentiation
This paragraph introduces the concept of implicit differentiation, a technique used to find the derivative of a function when it is not explicitly expressed in terms of y. The video begins by presenting a specific function, x^3 + y^3 = 8, and demonstrates the process of differentiating both sides with respect to x. The explanation highlights the importance of treating y as a function of x by including dy/dx in the differentiation process. The goal is to isolate dy/dx, and through algebraic manipulation, the paragraph explains how to solve for it in different scenarios. The paragraph also provides additional examples to illustrate the method, emphasizing the use of the product rule and chain rule when necessary.
🔢 Solving Implicit Differentiation Problems
This paragraph delves into the process of solving more complex implicit differentiation problems. It starts by presenting a new function, x^2 + 2xy + y^2 = 5, and walks through the steps of differentiating each term while applying the product rule where needed. The explanation shows how to isolate the derivative term, dy/dx, by moving other terms to the right side of the equation. The paragraph then presents another problem, 5xy - y^3 = 8, and explains the use of the product rule again. It emphasizes the importance of factoring out common terms and isolating dy/dx to find the solution. The paragraph also touches on alternative methods to solve the same problem, showcasing the flexibility in approaching implicit differentiation.
📈 Advanced Implicit Differentiation Techniques
This paragraph discusses advanced techniques in implicit differentiation, including the use of the quotient rule for finding second derivatives. It presents a new function, tangent(xy) = 7, and explains how to differentiate the inside of the tangent function using the chain rule. The explanation shows how to distribute the derivative of the constant part and isolate dy/dx. The paragraph then presents a method for simplifying the problem by adjusting the equation before differentiation, as seen in the example with √(x^2 + y^2) = 36. The explanation highlights the benefits of simplifying the radical before differentiating. Finally, the paragraph demonstrates how to find the second derivative using the quotient rule, providing a detailed example with the derived equation dy/dx = -x/y.
Mindmap
Keywords
💡Implicit Differentiation
💡Derivative
💡Product Rule
💡Chain Rule
💡Quotient Rule
💡Trigonometric Functions
💡Constant
💡Solving Equations
💡Algebraic Manipulation
💡Rate of Change
💡Simplifying Equations
Highlights
Introduction to implicit differentiation and its application in solving problems involving functions.
Differentiating both sides of an equation with respect to x to find dy/dx.
Example of differentiating x^3 + y^3 = 8 to find dy/dx, resulting in -x^2/y^2.
Using the product rule for implicit differentiation when dealing with terms involving both x and y.
Solution of the equation x^2 + 2xy + y^2 = 5 for dy/dx, which results in -1.
Applying the product rule to the problem 5xy - y^3 = 8, and isolating dy/dx.
Deriving the equation for tangent(x, y) = 7 and finding dy/dx through implicit differentiation.
Alternative method for solving the tangent problem by simplifying the equation before differentiation.
Solving the equation √(x^2 + y^2) = 36 for dy/dx by first squaring both sides and then differentiating.
Deriving the second derivative using the quotient rule from the first derivative dy/dx = -x/y.
Explanation of the quotient rule for finding the second derivative, with u and v representing the numerator and denominator of a fraction.
Final expression for the second derivative, demonstrating the application of the quotient rule.
Emphasis on the importance of understanding implicit differentiation for solving complex mathematical problems.
The video provides a comprehensive guide on implicit differentiation, covering various methods and examples.
The process of isolating dy/dx is emphasized in each example to illustrate the method clearly.
The video demonstrates the use of the chain rule in implicit differentiation when dealing with functions like tangent(x, y).
The video highlights the importance of simplifying equations before differentiation to make the process more manageable.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: