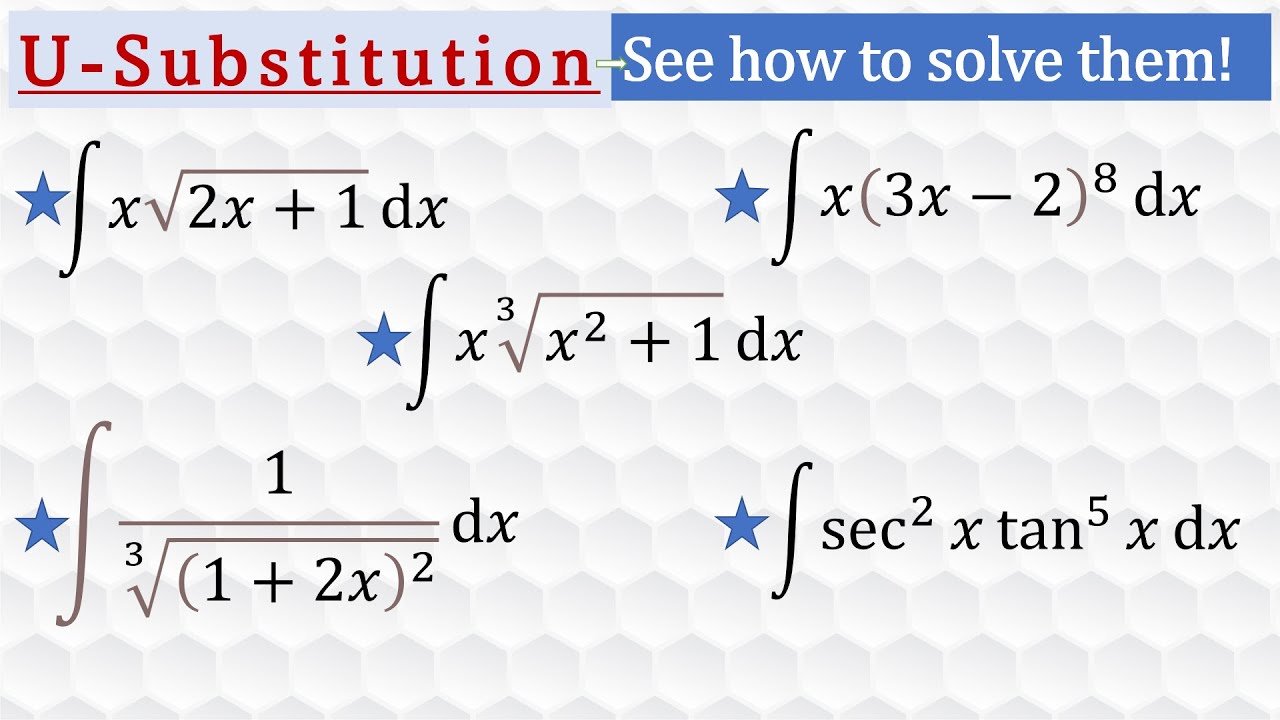
Understand u-substitution, the idea!
TLDRThe video script discusses the u-substitution technique for integration, drawing parallels with the chain rule for differentiation. It illustrates the process through the example of integrating the derivative of the tangent function, highlighting the connection between the two techniques. The script emphasizes the importance of understanding the steps involved in u-substitution and the ability to switch between the x and u variables. It also touches on the concept of integrating complex functions by recognizing patterns from the derivative table and applying the u-substitution method effectively.
Takeaways
- 📚 The script introduces the second technique of integration known as u-substitution, which is analogous to the chain rule used in differentiation.
- 🔄 The connection between u-substitution and the chain rule is highlighted, emphasizing that u-substitution is essentially the reverse process of the chain rule.
- 🌟 The example of differentiating the function tan(x^4) is used to demonstrate the application of the chain rule, resulting in the derivative being sec(x^4) * (4x^3).
- 📈 The process of u-substitution is explained step-by-step, starting with the selection of the inside function (x^4) as u, and then differentiating both sides to solve for du/dx.
- 🛠️ The script shows how to convert the original integral in the 'x-world' to the 'u-world' by isolating dx and expressing it in terms of du.
- 🧩 The integral in the 'u-world' is then evaluated by recognizing the function secant squared u, which corresponds to the derivative function of tan(u).
- 🔄 After completing the integration in the 'u-world', the result is converted back to the 'x-world' by substituting u back with x^4.
- ✍️ The importance of including a plus C at the end of the integral is mentioned, signifying the constant of integration.
- 📊 Another example is provided to integrate 1/(5x-2)dx, where u-substitution is used again with u = 5x-2, leading to the integral becoming ln|u| + C in the 'u-world'.
- 🔢 The script emphasizes the need to attach an absolute value when integrating functions involving u, to ensure the correct result.
- 🔄 The final step involves differentiating the result of the integral to confirm the process, showing that the derivative of the integral result matches the original function.
Q & A
What is the second technique of integration discussed in the transcript?
-The second technique of integration discussed is u-substitution.
How is the connection between u-substitution and the chain rule demonstrated in the transcript?
-The connection is shown by taking the derivative of the tangent of x to the fourth power, where the chain rule is applied to the inside function. The same process is then used in u-substitution, but instead of multiplying by the derivative, you divide by it.
What is the first step in applying u-substitution?
-The first step is to set u equal to the inside function that requires the chain rule.
How is the differential (dx) transformed in the u-substitution process?
-The differential dx is isolated and then transformed based on the derivative of u with respect to x. In the example given, dx becomes du/(4x^3).
What function is used to replace the original function in u-substitution?
-The function used to replace the original function is the derivative of u with respect to x, which is written as du/(4x^3) in the example.
What is the purpose of converting the integral from the x-world to the u-world?
-The purpose is to simplify the integral by transforming it into a form that can be recognized from the derivative table, making it easier to integrate.
How is the final result of the u-substitution integral converted back to the x-world?
-The final result is converted back to the x-world by replacing u with its original x-dependent expression, which is x^4 in the given example.
What is the significance of the plus C at the end of the integral?
-The plus C represents the constant of integration, which is necessary because the indefinite integral is not unique.
How does the transcript relate the process of u-substitution to the chain rule?
-The transcript explains that u-substitution is essentially the reverse process of the chain rule. While the chain rule involves multiplying by the derivative of the inside function, u-substitution involves dividing by it.
What is the integral of 1/(5x - 2)dx as per the transcript?
-The integral is 1/5 * ln|5x - 2| + C.
How is the absolute value handled in the integral of 1/(5x - 2)dx?
-An absolute value is placed around the u (which is 5x - 2) when integrating, but it is removed when differentiating the result to confirm the original function.
Outlines
📚 Introduction to Integration by U-Substitution
This paragraph introduces the concept of U-substitution, a technique used in integration. It explains the historical connection between U-substitution and the chain rule from differentiation. The speaker begins by differentiating the function tangent of X to the fourth power, highlighting how the chain rule is applied. The paragraph then transitions into explaining how to integrate the derived function by setting up the U-substitution with detailed steps, emphasizing the importance of understanding the process to solve more complex integrals effectively.
🔍 Elaborating U-Substitution with a Specific Example
The speaker elaborates on the U-substitution technique by working through a specific example. The example involves integrating a function that initially does not have a direct antiderivative in the standard tables. The speaker demonstrates how to transform the original integral in the X-world to the U-world, simplify the expression, and then integrate. The paragraph also explains the importance of converting back to the original variable (X) after integration and the significance of adding a plus C at the end of the process.
🔧 Applying U-Substitution to a Tricky Integral
This paragraph further illustrates the application of U-substitution to a more complex integral involving a rational function. The speaker chooses an appropriate U variable, differentiates it, and sets up the integral in terms of U. The explanation includes isolating the differential (DX), transforming the integral into a recognizable form from the derivative table, and integrating to obtain the result in terms of U. Finally, the speaker shows how to convert the result back into terms of X and emphasizes the importance of the absolute value in certain cases.
Mindmap
Keywords
💡Integration
💡u-substitution
💡Derivative
💡Chain Rule
💡Secant Function
💡Tangent Function
💡Differential
💡Natural Logarithm
💡Constant Multiple
💡Absolute Value
💡Antiderivative
Highlights
Introduction to u-substitution as the second technique of integration.
Connection between u-substitution and the chain rule in differentiation.
Derivative of tangent of X to the fourth power using the chain rule.
Integration of a function involving secant squared and X to the fourth power using u-substitution.
Setting up u as the inside function, X to the fourth power.
Differentiating both sides to find the differential du dx.
Isolating dx to get the relationship between x and u.
Transforming the integral from the X world to the u world using u-substitution.
Integration of secant squared u in the u world.
Returning to the X world after integration by substituting u back with X to the fourth power.
The importance of adding a plus C at the end of the integration process.
Next example: Integration of 1 over (5x - 2)DX using u-substitution.
Choosing u as 5x - 2, the denominator of the integrand.
Differentiating u to find du dx and isolating dx.
Transforming the integral to the u world by replacing X with u.
Integration of 1 over u in terms of natural logarithm.
Substituting u back with 5x - 2 to return to the X world for the final answer.
Verification of the result by differentiating the integrated function and comparing it to the original function.
The relationship between u-substitution and the chain rule, with u-substitution being the reverse process.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: