Projectile Motion and Conservation of Energy - College Physics
TLDRThe script presents a detailed analysis of a projectile motion problem, where a ball is launched at a 60-degree angle with an initial speed of 80 m/s and strikes a building 200 meters high. Two methods are used to determine the ball's speed upon impact: kinematics and conservation of energy. The kinematic approach involves solving a quadratic equation to find the time of flight and then calculating the final velocity components. The conservation of energy method offers a simpler and quicker solution by equating initial kinetic energy to the sum of final kinetic and potential energy at the point of impact. Both methods yield a final speed of approximately 49.8 m/s, showcasing alternative ways to tackle physics problems.
Takeaways
- 🚀 The problem involves a projectile motion scenario with a ball launched at a 60-degree angle and 80 m/s speed.
- 📐 Two methods are used to solve the problem: kinematics and conservation of energy.
- 🕒 The time of flight is calculated using the kinematic equation for vertical displacement with the initial and final heights.
- 🔢 Two possible times are found (10.1 seconds and 4.04 seconds), but only the larger value (10.1 seconds) is relevant for the impact with the building.
- 🏢 The horizontal velocity (VX) remains constant throughout the motion, calculated as the initial velocity cosine of the launch angle.
- 📉 The vertical velocity (VY) changes due to gravity and is calculated using the initial vertical velocity minus the product of gravity and time.
- 📌 The final velocity (VF) at the point of impact is found using the Pythagorean theorem, combining the constant horizontal and the changed vertical components.
- 🌐 Conservation of energy is applied by equating the initial kinetic energy to the sum of final kinetic and potential energy at the building's height.
- 🔄 The mass of the ball cancels out in the energy conservation calculation, simplifying the process.
- ⏰ The conservation of energy method provides a quicker way to find the final velocity compared to the kinematic approach.
- 📚 Additional resources for projectile motion and physics problems are available in the description section for further study.
Q & A
What is the initial velocity of the ball in the projectile motion problem?
-The initial velocity of the ball is 80 meters per second.
What angle is the ball launched at from the ground?
-The ball is launched at an angle of 60 degrees from the ground.
What is the height of the building that the ball strikes?
-The building is 200 meters high above the ground level.
How many seconds does it take for the ball to reach the top of the building?
-It takes approximately 10.1 seconds for the ball to reach the top of the building.
What is the horizontal velocity component of the ball at the moment it strikes the building?
-The horizontal velocity component of the ball at the moment it strikes the building is 40 meters per second.
What is the vertical velocity component of the ball just before it hits the building?
-The vertical velocity component of the ball just before it hits the building is approximately -29.698 meters per second (negative sign indicates downward direction).
What is the final speed of the ball just before it hits the building?
-The final speed of the ball just before it hits the building is approximately 49.08 meters per second.
How does the conservation of energy method simplify the solution to the projectile motion problem?
-The conservation of energy method simplifies the solution by directly relating the initial and final energies of the ball, without the need to calculate the intermediate positions or velocities, leading to a quicker calculation of the final velocity.
What are the two main types of energy considered in the conservation of energy approach for this problem?
-The two main types of energy considered are kinetic energy and gravitational potential energy.
Why is the acceleration due to gravity considered positive in the calculation of gravitational potential energy?
-The acceleration due to gravity is considered positive in the calculation of gravitational potential energy because we are interested in the potential energy of the ball relative to the ground level, which is a positive value.
How do the results obtained from the kinematic equations and the conservation of energy method compare?
-Both methods yield the same result for the final speed of the ball before it hits the building, approximately 49.08 meters per second, demonstrating that both approaches are valid and can be used to solve projectile motion problems.
What is the significance of the two different time values obtained from the quadratic equation?
-The two different time values represent the two instances during the projectile motion when the ball is at a height of 200 meters. The smaller value (4.04 seconds) corresponds to the initial height, and the larger value (10.1 seconds) corresponds to the time when the ball reaches the top of the building.
Outlines
🚀 Solving Projectile Motion with Kinematics
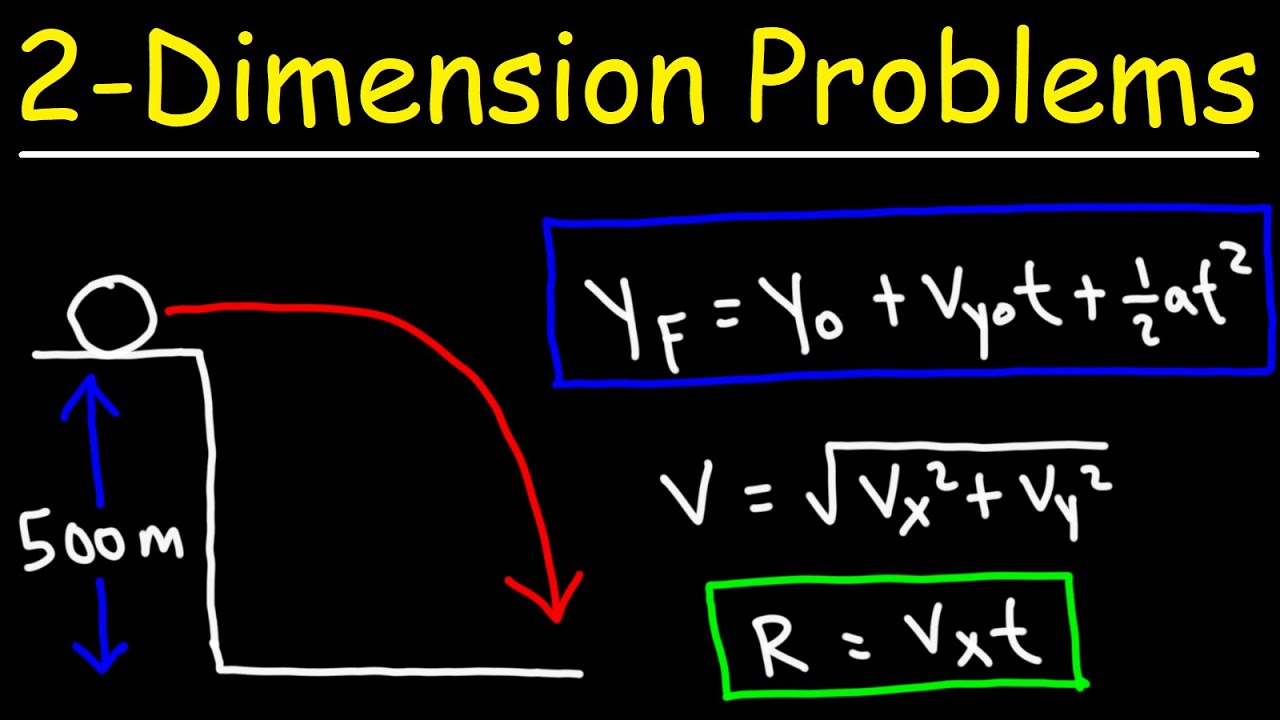
This paragraph introduces a projectile motion problem where a ball is launched at a 60-degree angle with an initial speed of 80 meters per second. The goal is to find the speed of the ball when it strikes a 200-meter tall building. Two methods are proposed for solving the problem: kinematics and conservation of energy. The paragraph begins by setting up the kinematic equation to find the time it takes for the ball to travel from its initial position to the point where it hits the building. The initial conditions are used to derive a quadratic equation in standard form, which is then solved to find two possible time values. The first method focuses on the kinematic analysis, which involves breaking down the motion into horizontal and vertical components and using the equations of motion to determine the final speed.
🕒 Determining Time and Velocity Components
In this paragraph, the two time values obtained from the quadratic equation are analyzed to determine which one is relevant for the problem. It is concluded that the smaller time value corresponds to the first point of interest, and the larger one is discarded. The paragraph then proceeds to calculate the horizontal and vertical velocity components at the point of impact with the building using the derived time value of 10.1 seconds. The horizontal velocity component remains constant, while the vertical component is determined by taking into account the acceleration due to gravity. The final velocity of the projectile is then found using the Pythagorean theorem, which combines the horizontal and vertical components to give a final speed of 49.08 meters per second.
🌐 Conservation of Energy Method
The third paragraph presents an alternative method for solving the projectile motion problem using the principle of conservation of energy. This approach simplifies the calculation by considering the types of energy at the initial and final points of the trajectory. The initial kinetic energy and the final kinetic energy, along with the potential energy at the point of impact, are used to set up an equation. By canceling out the mass of the ball and simplifying the equation, the final velocity is calculated to be 49.8 meters per second, which matches the result obtained using the kinematic method. This paragraph emphasizes the efficiency of using conservation of energy for solving problems in physics and encourages exploring additional resources for further understanding of projectile motion and related topics.
Mindmap
Keywords
💡Projectile Motion
💡Kinematics
💡Conservation of Energy
💡Acceleration Due to Gravity
💡Velocity Components
💡Gravitational Potential Energy
💡Quadratic Equation
💡Pythagorean Theorem
💡Horizontal and Vertical Velocity
💡Time of Flight
Highlights
Projectile motion problem involving a ball launched at a 60-degree angle with an initial speed of 80 meters per second.
Two techniques used to solve the problem: kinematics and conservation of energy.
The ball is launched from the ground and lands on a building 200 meters high.
Kinematic equation used for calculation: y_final = y_initial + v_y_initial*t + 0.5*a*t^2.
The time of flight is calculated using a quadratic equation in standard form.
Two possible time values obtained (10.1 seconds and 4.04 seconds), with the latter being discarded as it corresponds to the initial height.
Horizontal velocity component (VX) remains constant at 40 meters per second.
Vertical velocity component (VY) changes due to gravity and is calculated using the equation v_y_final = v_y_initial + a*t.
The final speed of the ball just before striking the building is found using the Pythagorean theorem.
The final speed calculated is 49.08 meters per second.
Conservation of energy method is applied as a simpler and faster alternative to kinematics.
Initial and final energies are compared, considering kinetic and potential energies.
The mass of the ball cancels out in the energy conservation equation, simplifying the calculation.
The final velocity obtained using energy conservation also equals 49.8 meters per second, confirming the result from the kinematic method.
The problem demonstrates the versatility of physics methods in solving projectile motion issues.
Additional resources and example problems for further study are provided in the description section.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: