Horizontal Line Test and One to One Functions
TLDRThe video script discusses the concept of inverse functions in mathematics, emphasizing the importance of one-to-one functions. It explains that a function must pass the horizontal line test to be considered one-to-one, thereby having an inverse. Using examples of linear and parabolic functions, the script illustrates how intersections with horizontal lines determine if a function is one-to-one. It concludes by reinforcing that functions passing the horizontal line test, like 'g of x', have inverse functions, while those failing the test do not.
Takeaways
- 📌 A linear function, f(x), is used as an example to discuss inverse functions.
- 🔍 To determine if f(x) has an inverse, one must prove it is a one-to-one function.
- 🎢 The horizontal line test is a method to check if a function is one-to-one; the function must intersect any horizontal line at most once.
- 🚫 If a function, like a parabolic f(x), intersects a horizontal line more than once, it is not one-to-one and lacks an inverse function.
- ✅ A function has an inverse if it passes the horizontal line test, intersecting each horizontal line only at a single point.
- 📊 The script provides visual examples to illustrate the concepts of one-to-one functions and their inverses.
- 🔄 The inverse of a function is not a function if it fails the vertical line test, which means it's not uniquely defined for each input.
- 🚦 Functions g(x) and another unnamed function are analyzed for one-to-one properties and inverse existence.
- ✖️ The unnamed function that touches a horizontal line at three points is not one-to-one and does not have an inverse.
- 📋 The video concludes with a summary of how to determine if a function has an inverse and the importance of the horizontal line test.
Q & A
What is the main concept discussed in the video?
-The main concept discussed in the video is how to determine if a function has an inverse function, specifically by checking if it is a one-to-one function and passes the horizontal line test.
What is a linear function?
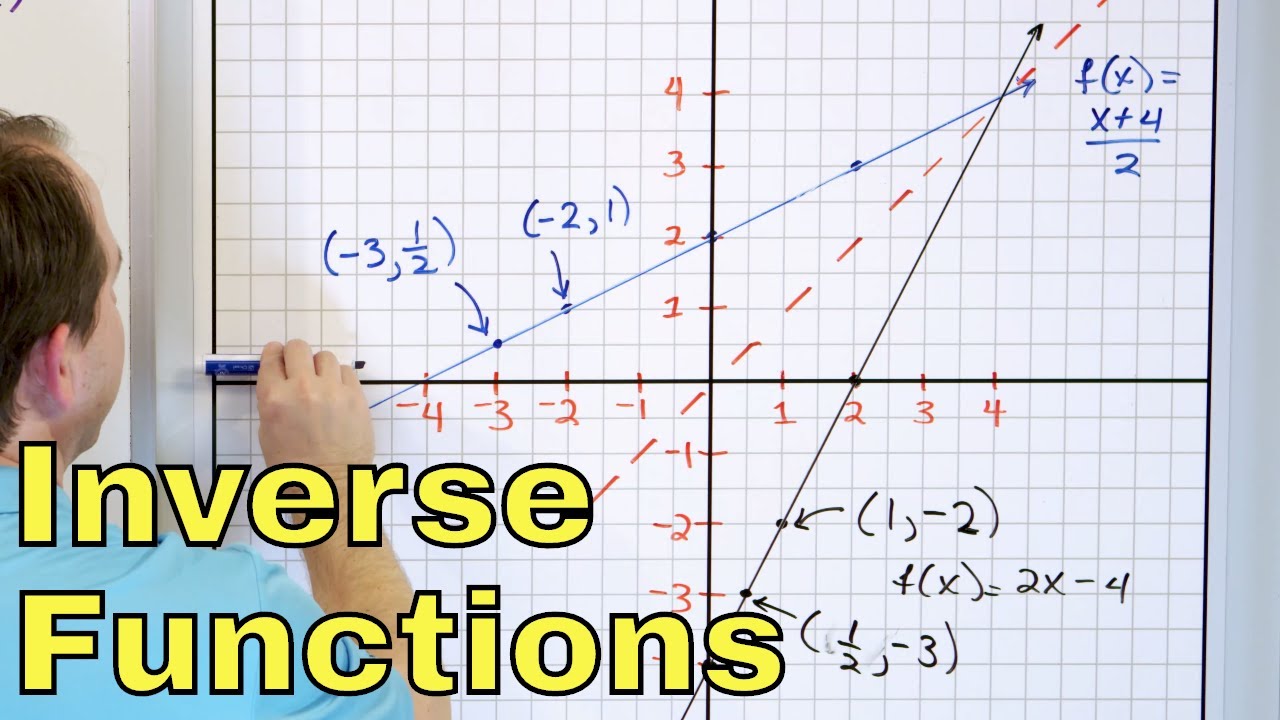
-A linear function is a mathematical function that has the form f(x) = mx + b, where m and b are constants, and m is the slope of the line. In the video, f(x) is used as an example of a linear function.
What is the horizontal line test?
-The horizontal line test is a method used to determine if a function is one-to-one. If any horizontal line intersects a graph of a function more than once, the function is not one-to-one and does not have an inverse. If a horizontal line intersects the graph at no more than one point, the function is one-to-one and has an inverse.
What is a one-to-one function?
-A one-to-one function, also known as an injective function, is a function where each input (x-value) corresponds to exactly one output (y-value). This means that no two inputs map to the same output, which is a requirement for a function to have an inverse.
Why is it important for a function to be one-to-one to have an inverse?
-It is important for a function to be one-to-one to have an inverse because the inverse function must be able to uniquely determine the original input for each output. If a function is not one-to-one, there would be ambiguity in the inverse since multiple inputs could produce the same output, violating the definition of a function.
What happens if a function does not pass the horizontal line test?
-If a function does not pass the horizontal line test, it means the function is not one-to-one because there exists at least one horizontal line that intersects the graph at more than one point. As a result, the function does not have an inverse.
How does the parabolic function relate to the concept of one-to-one functions and inverses?
-The parabolic function was used in the video as an example of a function that does not pass the horizontal line test. Since it intersects horizontal lines at more than one point, it is not a one-to-one function and therefore does not have an inverse function.
What is the vertical line test, and how does it relate to inverse functions?
-The vertical line test is a method to determine if a graph represents a function. A graph passes the vertical line test if every vertical line intersects the graph at most once. In the context of inverse functions, if the original function does not pass the horizontal line test, its inverse relation (if graphed) would not pass the vertical line test, meaning it is not a function.
How can you find the inverse of a function that has an inverse?
-To find the inverse of a function that has an inverse, you would swap the x and y variables (inputs and outputs) and then solve for the new y variable. This process gives you the inverse function, which undoes the operation of the original function.
What is the significance of the example provided with three functions, f(x), g(x), and another unnamed function?
-The significance of the example with three functions is to illustrate the different scenarios of functions in relation to the horizontal line test and the existence of inverse functions. f(x) fails the test and does not have an inverse, g(x) passes the test and has an inverse, and the unnamed function intersects a horizontal line at three points, indicating it does not have an inverse.
How can you determine if a function has an inverse without graphing?
-You can determine if a function has an inverse without graphing by analyzing its algebraic expression. If the function can be expressed in a way that ensures a unique output for each input (for example, by having a unique solution for y when solving for x), then it is a one-to-one function and has an inverse.
Outlines
📚 Introduction to One-to-One Functions and Inverse Functions
This paragraph introduces the concept of one-to-one functions and their inverses. It uses a linear function, f(x), as an example and explains that to determine if a function has an inverse, one must show that it is a one-to-one function by passing the horizontal line test. The test involves drawing a horizontal line and checking if the function intersects this line at only one point, indicating a one-to-one correspondence. The paragraph further discusses the parabolic function, g(x), which fails the horizontal line test as it intersects the line at two points, meaning it does not have an inverse function. The explanation is supplemented with a rough sketch to illustrate the concept.
Mindmap
Keywords
💡linear function
💡inverse function
💡one-to-one function
💡horizontal line test
💡parabolic function
💡vertical line test
💡function
💡graph
💡intersection
💡uniqueness
💡example
Highlights
The concept of a linear function, f(x), is introduced as an example.
To determine if f(x) has an inverse, it must be shown that it is a one-to-one function.
The horizontal line test is a method to check if a function is one-to-one by ensuring it intersects any horizontal line at most once.
In the given example, f(x) passes the horizontal line test and therefore has an inverse function.
A parabolic function, g(x), is analyzed for having an inverse function.
The parabolic function fails the horizontal line test as it intersects the line at two points, indicating it is not one-to-one and lacks an inverse function.
The concept of the inverse relation is briefly discussed, noting that it won't be a function if the original function isn't one-to-one.
Another function, f(x), is examined, and it is determined not to pass the horizontal line test, meaning it isn't one-to-one and doesn't have an inverse function.
Function g(x) is shown to pass the horizontal line test and thus has an inverse function, being a one-to-one function.
A third function is analyzed, touching the horizontal line at three points, failing the test and indicating it is not one-to-one, without an inverse function.
The video concludes by emphasizing the method to determine if a function has an inverse and is one-to-one: passing the horizontal line test.
The importance of a function being one-to-one to have an inverse function is reiterated, with a clear explanation provided.
The video provides a clear and concise explanation of the horizontal line test and its significance in determining the existence of an inverse function.
The transcript outlines the process of determining if multiple functions have inverse functions, offering a comparative analysis.
The transcript serves as an educational resource for understanding the properties of functions and their inverses.
The practical application of the horizontal line test is demonstrated through various function examples.
The transcript effectively communicates the concept of one-to-one functions and their relation to inverse functions.
The examples provided in the transcript help illustrate the theoretical concepts in a tangible and understandable way.
The transcript's methodical approach to explaining the horizontal line test and one-to-one functions is beneficial for learners.
The transcript's content is relevant for anyone studying or reviewing the basics of functions and their inverses in mathematics.
The video's content is a valuable resource for educators looking to explain the concept of one-to-one functions and inverses to their students.
Transcripts
Browse More Related Video
One to One Functions (Precalculus - College Algebra 50)
Ch. 2.8 One-to-One Functions and their Inverses
Introduction to Inverse Trigonometric Functions (Precalculus - Trigonometry 17)
Introduction to Inverse Functions
12 - What are Inverse Functions? (Part 1) - Find the Inverse of a Function & Graph
Inverse Functions
5.0 / 5 (0 votes)
Thanks for rating: