High School Physics - Vertical Circular Motion
TLDRThe video script delves into the physics of vertical circular motion, using examples like roller coasters to explain the concepts. It covers how objects in vertical circles experience varying speeds and the resultant forces, such as centripetal force and gravity, that keep them in motion. The discussion includes the impact of speed on the normal force felt by riders and how these forces prevent them from falling out of their seats. Additionally, the script explores problems involving circular motion with a rubber stopper and calculates parameters like speed and centripetal force, offering a comprehensive understanding of the dynamics at play.
Takeaways
- 📊 Vertical circular motion involves objects moving in circles but not at a constant speed, affecting the uniformity of the motion.
- 🎢 Roller coasters in loops provide a real-world example of vertical circular motion, where the forces acting on the cart are crucial to understanding the motion.
- 🔝 At the bottom of a roller coaster loop, the net force acting towards the center of the circle is the difference between the normal force and the weight of the cart.
- 🚀 The normal force felt at the bottom of a loop is greater than the weight due to the additional centripetal force, making riders feel heavier.
- 🔽 At the top of a loop, the normal force is less than the weight, leading to a feeling of weightlessness or reduced force.
- 💡 The faster an object moves in a circular path (with a smaller radius), the greater the additional force (G Force) felt by the object.
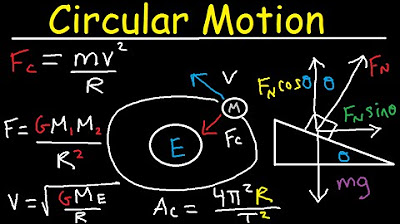
- 🔗 Newton's Second Law is used to analyze the forces in vertical circular motion, with the equation FN = m(V^2/R) + mg for the bottom of a loop and FN = m(V^2/R) - mg for the top.
- 🔄 Inertia is what keeps an object moving in a circle, with the track applying a normal force to direct the object towards the center of the circle.
- 🧩 If the normal force becomes zero, the object will no longer be in contact with the track and will start to fall due to gravity.
- 📐 The radius of a circular motion can change with the speed; if the speed increases, the radius must also increase to maintain the same centripetal force.
- 📈 The centripetal force acting on an object in circular motion can be calculated using the formula FC = m(V^2/R), which is helpful in understanding the dynamics of the motion.
Q & A
What is the main topic of the transcript?
-The main topic of the transcript is vertical circular motion and its analysis, specifically in the context of roller coasters and other circular motion scenarios.
Why don't people fall out of roller coasters when they are upside down?
-People don't fall out of roller coasters due to the normal force exerted by the track on the cart, which provides the necessary centripetal force to keep the riders moving in a circular path. This force counteracts gravity and keeps the riders pressed into their seats.
What is the free body diagram for a roller coaster at the bottom of the loop?
-The free body diagram for a roller coaster at the bottom of the loop includes the gravitational force (mg) pulling down and the normal force from the track pushing up.
How can we calculate the normal force experienced by a rider at the bottom of a roller coaster loop?
-The normal force can be calculated using the equation FN = m*v^2/R + mg, where m is the mass of the rider, v is the velocity of the roller coaster, R is the radius of the loop, and mg represents the gravitational force.
What is the relationship between the normal force and the G-Force felt by a rider?
-The normal force is related to the G-Force felt by a rider as it is the force pushing them into their seat. The G-Force increases with the square of the velocity (v^2) and decreases with an increase in the radius (R) of the circular path.
What happens at the top of a roller coaster loop in terms of the forces acting on the cart?
-At the top of a roller coaster loop, gravity (mg) pulls the cart down, and the normal force points toward the center of the circle, providing the centripetal force needed to keep the cart moving in a circular path.
How can we determine the normal force at the top of a roller coaster loop?
-The normal force at the top of the loop can be determined using the equation FN = m*v^2/R - mg, where the term mv^2/R represents the centripetal force required for circular motion and mg is the gravitational force.
What is the effect of increasing the speed of a whirling object on its circular path radius?
-If the speed of a whirling object increases, the radius of its circular path must also increase to maintain the same centripetal force, as the centripetal force is given by the equation Fnet = m*v^2/R and R is inversely proportional to V.
How does the direction of the centripetal force change in a vertical circular motion?
-In a vertical circular motion, the direction of the centripetal force always points toward the center of the circle, regardless of the position in the loop.
What is the centripetal force acting on a 0.028 kg stopper whirled in a horizontal circle with a radius of 1 meter?
-The centripetal force acting on the stopper can be calculated using the formula Fc = m*v^2/R. With a mass of 0.028 kg, a velocity of 2π*1 m/s (which is 2 m/s), and a radius of 1 meter, the centripetal force is approximately 1.11 Newtons.
How does inertia play a role in keeping an object moving in a circular path?
-Inertia is the property of an object to resist changes in its state of motion. In circular motion, inertia wants to pull the object in a straight line, but the track (or in the case of a roller coaster, the rails) applies a normal force that provides the centripetal force, which keeps the object moving in a circular path.
Outlines
🎢 Understanding Vertical Circular Motion
This paragraph introduces the concept of vertical circular motion, emphasizing that objects in such motion do not move at a constant speed, thus deviating from uniform circular motion. The discussion revolves around the physics behind roller coasters, particularly the forces acting on a cart as it goes through a loop. It explains how the normal force and gravitational force contribute to the centripetal force required for circular motion, and how these forces affect the perceived weight of passengers. The paragraph also touches on the relationship between speed, radius, and the experienced G-forces, illustrating how increasing speed can prevent riders from falling out of their seats due to the increased normal force.
🔁离心力与圆周运动的实例分析
本段落通过两个实验性问题来进一步阐释圆周运动中的物理概念。第一个问题探讨了当学生以更快的速度旋转橡胶塞时,圆周运动的半径如何变化。通过分析,得出了半径与速度的关系,即速度增加时,为了保持向心力不变,半径也必须增加。第二个问题则是一个实际的圆周运动案例,涉及一个0.028kg的橡胶塞以一定的速度在水平面内做圆周运动,并要求计算出橡胶塞所受的向心力。通过速度和质量的计算,得出向心力的具体数值。这两个问题帮助理解在圆周运动中,物体如何受到向心力的作用,并且展示了如何通过物理公式计算相关力的大小。
Mindmap
Keywords
💡Vertical Circular Motion
💡Centripetal Force
💡Newton's Second Law
💡Normal Force
💡G-Force
💡Inertia
💡Free Body Diagram
💡Loop
💡Velocity
💡Acceleration
💡Radius
Highlights
Introduction to vertical circular motion and its non-uniform speed characteristics.
Use of tools developed for uniform circular motion to analyze vertical circular motion with small variations in speed.
Explanation of why riders don't fall out of a roller coaster using vertical circular motion principles.
Discussion on the free body diagram of a roller coaster at the bottom of the loop, including forces acting on the cart.
Application of Newton's Second Law to analyze vertical circular motion and calculate the net force.
Feeling of increased weight at the bottom of a roller coaster due to the normal force.
Equation rearrangement to find the normal force and its relation to G-force experienced by riders.
Explanation of the sensation of G-force in relation to speed and radius of the circle.
Analysis of the top of the roller coaster loop and the forces acting on the cart.
Importance of velocity and normal force in keeping the cart in contact with the rails.
Role of inertia in maintaining circular motion and the track's application of normal force.
Experiment with a rubber stopper in a horizontal circular path to illustrate the analysis of circular motion.
Effect of increasing the speed on the radius of the circular path in the experiment.
Labeling of vectors in a vertical circular motion diagram for a rubber stopper.
Determination of the centripetal force direction in a vertical circular motion scenario.
Calculation of the speed of a whirling stopper in a horizontal circle using given parameters.
Determination of the centripetal force acting on the stopper in a horizontal circular motion experiment.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: