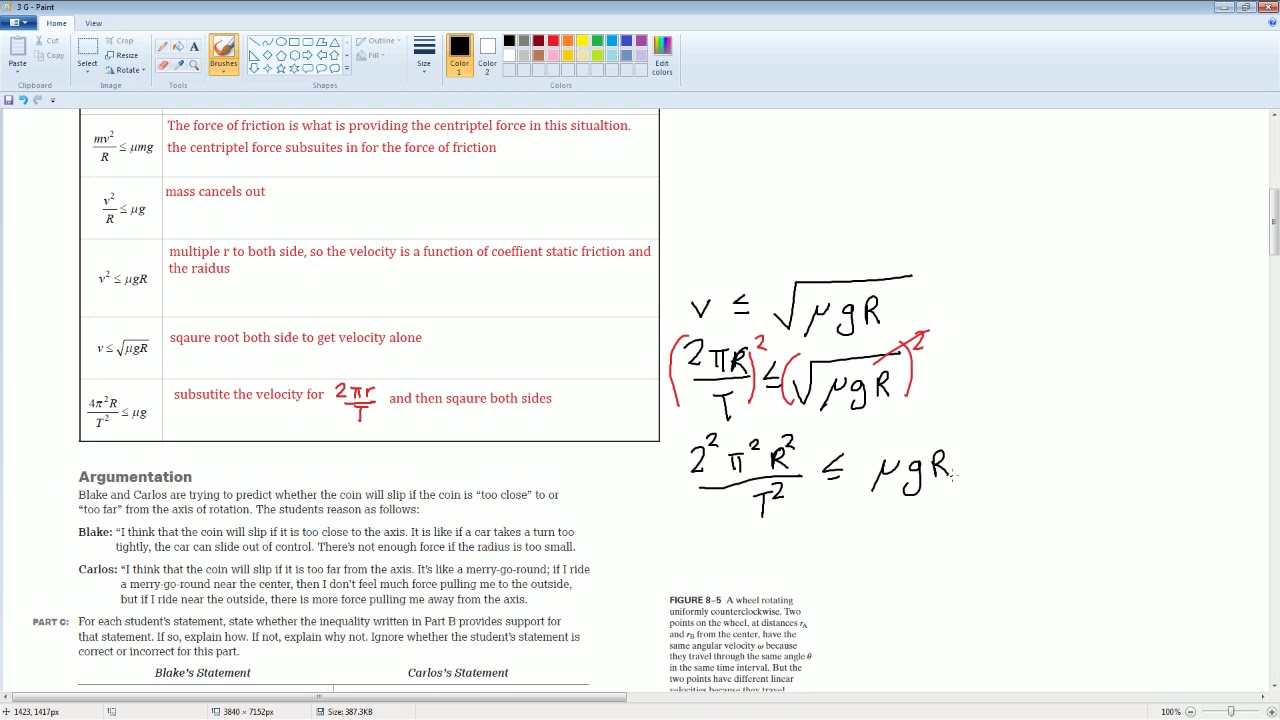
AP Physics Workbook 2.I Stopping Distance
TLDRThe video script discusses a physics problem involving a car that brakes suddenly to avoid debris, sliding on a flat road until it stops. It explains the forces at play, such as gravity and friction, and how they affect the car's motion. The script then delves into the impact of initial velocity and the coefficient of friction on the distance the car slides. It uses kinematics equations to derive a formula for the sliding distance, demonstrating that an increase in initial velocity or a decrease in the coefficient of friction results in a longer slide. The explanation is complemented by a discussion on kinetic and static friction, emphasizing the role of friction in determining the car's motion.
Takeaways
- 🚗 A car with mass M is moving with initial velocity v and brakes suddenly to avoid debris on a flat road.
- 🔢 The distance the car slides (d) is determined by the friction between the tires and the road, characterized by the coefficient mu (μ).
- 📈 As the initial velocity (v) increases, the sliding distance (d) also increases due to the car's greater momentum.
- 🔄 The frictional force acts in the opposite direction of the car's motion, causing it to slow down and eventually stop.
- 📉 Increasing the coefficient of friction (μ) results in a decrease in the sliding distance (d) because the car experiences greater resistance.
- 🎯 The car's deceleration is equal to the coefficient of friction times the acceleration due to gravity (μg).
- 📐 The vertical forces (gravity and normal force) balance each other out, confirming that there is no vertical acceleration.
- 🚦 Kinematics equations are used to solve for the distance (d) without a given time value, using the relationship between initial velocity, acceleration, and distance.
- 🌟 The equation ΔX = v₀² / (2μg) relates the initial velocity, the coefficient of friction, and the gravitational force to the sliding distance.
- 🔧 The static coefficient of friction is higher than the kinetic coefficient, requiring a greater force to initiate movement.
- 📚 Understanding the dynamics of the car's motion and the role of friction is essential for predicting and analyzing real-world scenarios involving braking and sliding.
Q & A
What is the scenario described in the AP Physics workbook?
-The scenario involves a car of mass M moving with an initial speed v, which has to brake suddenly to avoid colliding with debris on a straight flat road. The car's wheels lock, causing it to slide until it stops before running over the debris, and the distance it slides is denoted as d.
What forces are acting on the car during the sliding phase?
-During the sliding phase, the car experiences gravitational force downward and a normal force upward, which are equal in magnitude. The only horizontal force acting on the car is the force of friction, which acts backward (opposite to the initial direction of motion).
How does the car's initial velocity affect the sliding distance?
-The sliding distance (d) increases with an increase in the initial velocity. This is because a higher initial velocity means the car has more kinetic energy, which will result in a longer slide before coming to a stop.
What happens to the sliding distance if the coefficient of friction is increased?
-If the coefficient of friction is increased, the sliding distance decreases. A higher coefficient of friction means a greater frictional force acting on the car, which opposes the forward motion more effectively, causing the car to stop sooner.
What are the two types of friction mentioned in the script?
-The two types of friction mentioned are kinetic friction, which occurs when two surfaces are moving relative to each other, and static friction, which occurs when the surfaces are not moving relative to each other but there is a tendency for motion.
How does the static coefficient of friction compare to the kinetic coefficient?
-The static coefficient of friction is typically larger than the kinetic coefficient. This means that it requires a greater force to initiate movement from rest than to keep an object moving once it is already in motion.
What is the relationship between the force of friction and the normal force?
-The force of friction is equal to the coefficient of friction (mu) times the normal force (FN). Since FN is equal to the gravitational force (FG) on a horizontal surface, the force of friction can be expressed as mu times the product of mass (m) and gravitational acceleration (g).
What kinematic equation is used to solve for the sliding distance?
-The kinematic equation used to solve for the sliding distance is Delta X = v^2 / (2a), where v is the initial velocity, and a is the acceleration (which is equal to mu * g in this case).
How does the car's deceleration relate to the coefficient of friction and gravitational acceleration?
-The car's deceleration is equal to the coefficient of friction times gravitational acceleration (mu * g). This indicates that the deceleration is directly proportional to the frictional force acting on the car.
What can we infer about the relationship between velocity and distance from the kinematic equation?
-From the kinematic equation, we can infer that the distance is directly proportional to the square of the initial velocity. This means that if the initial velocity doubles, the sliding distance will increase by a factor of four.
How does the increase in the static coefficient affect the car's stopping distance in terms of physics principles?
-An increase in the static coefficient results in a greater frictional force, which in turn increases the car's deceleration. As a result, the car will stop more quickly, leading to a shorter stopping distance. This is due to the enhanced frictional force being more effective in dissipating the car's kinetic energy.
Outlines
🚗 Car Braking and Friction Analysis
This paragraph introduces a scenario where a car with mass M is moving with an initial velocity v. The driver applies brakes to avoid hitting debris on a flat road, causing the car to slide until it stops. The distance the car slides is denoted as d. The coefficient of friction, μ, between the tires and the road is considered. The paragraph discusses the free body diagram of the car, showing forces acting on it, including gravity and friction. It explains how an increase in initial velocity leads to an increase in the sliding distance. The impact of increasing the coefficient of friction on the sliding distance is also analyzed, noting that higher friction leads to a shorter sliding distance due to greater opposing force to the car's motion. The paragraph also explains the concepts of kinetic and static friction, highlighting that static friction requires a larger force to initiate movement, which decreases once the object is in motion.
📐 Kinematics and Frictional Force Equations
In this paragraph, the focus is on deriving equations related to the car's deceleration and the frictional force acting on it. It starts by establishing that the force normal (FN) is equal to the force of gravity (FG) on a horizontal surface. The paragraph then uses kinematics to solve for the distance (ΔX), which is replaced by D for simplicity. The deceleration of the car is shown to be equal to the product of the coefficient of friction (μ) and gravity (g). The derived equation relates the initial velocity, distance, and frictional force, showing that an increase in initial velocity results in a longer sliding distance. The effect of increasing the coefficient of static friction on the distance is also discussed, with the conclusion that an increase in static friction coefficient results in a shorter sliding distance. The paragraph provides a comprehensive understanding of the physics principles involved in the car's motion and the role of friction.
Mindmap
Keywords
💡Dynamics
💡Friction
💡Coefficient of Friction
💡Kinematics
💡Acceleration
💡Free Body Diagram
💡Kinetic Friction
💡Static Friction
💡Braking Distance
💡Deceleration
💡Normal Force
Highlights
The scenario involves a car of mass M moving with an initial speed v, which brakes to avoid debris on a flat road.
The car wheels lock, causing it to slide on the road until it stops, with the distance slid being d.
The coefficient of friction between the car tires and the roadway is a constant mu.
The first task is to draw a free body diagram, identifying forces such as gravity, normal force, and friction.
The distance the car slides (d) is related to the initial velocity and the coefficient of friction.
An increase in initial velocity results in an increase in the sliding distance (d).
An increase in the coefficient of friction (mu) results in a decrease in the sliding distance (d).
There are two types of friction: kinetic friction (moving) and static friction (stationary).
The static coefficient is actually a larger value than the kinetic coefficient.
Friction is a force that opposes the motion of objects and acts on a microscopic level.
In the vertical direction, the force normal (FN) equals the force of gravity (FG), indicating no acceleration in this direction.
The deceleration of the car is equal to the coefficient of friction times gravity (mu * g).
The equation to solve for the distance (D) involves kinematics and the force of friction.
The final equation derived shows that D = v^2 / (2 * mu * g), highlighting the relationship between distance, initial velocity, and friction.
The car will travel a longer distance with a higher initial velocity and a lower coefficient of friction.
The analysis demonstrates the practical application of physics principles in understanding vehicle motion and stopping distances.
This problem-solving approach can be applied to real-world scenarios, such as improving road safety through understanding friction and vehicle dynamics.
The transcript provides a comprehensive understanding of the dynamics of a car sliding on a road, incorporating both theoretical and practical aspects.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: