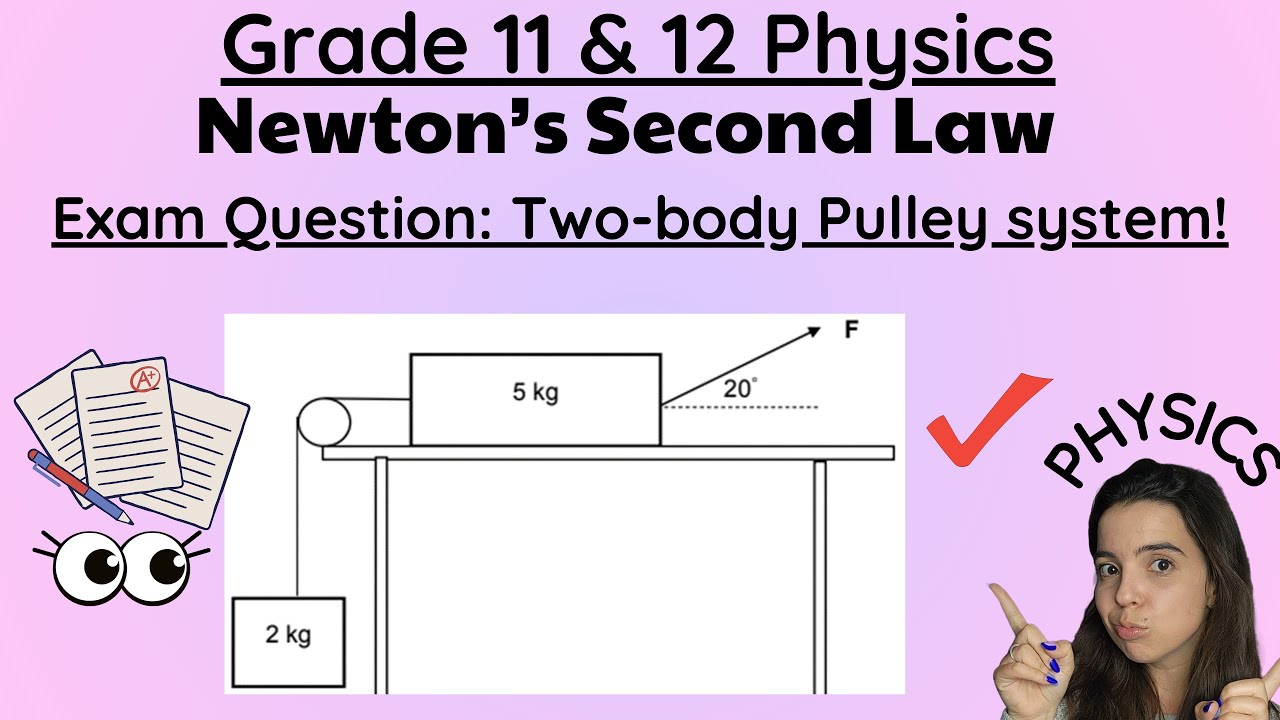
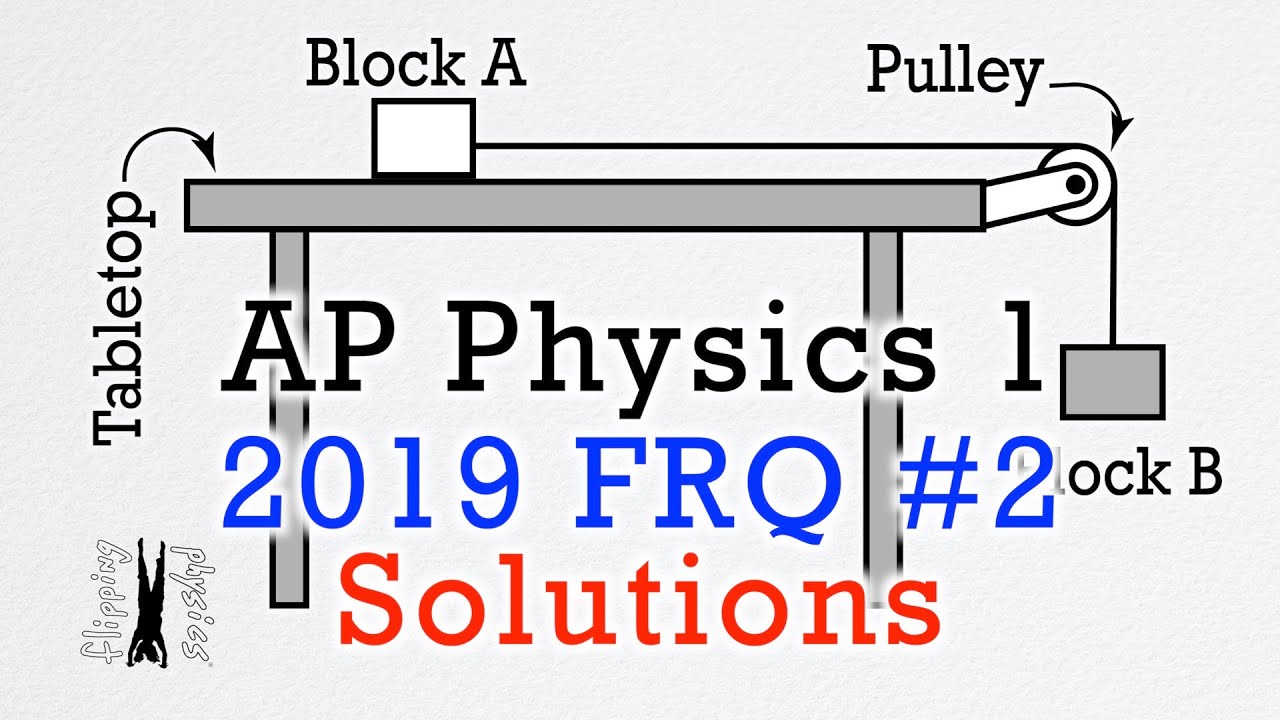
2015 AP Physics 1 free response 1b

Khan Academy
5 Apr 201609:35
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the derivation of the magnitude of acceleration for block 2 in a two-block system connected by a string. The explanation involves understanding the direction of acceleration due to the weight of the blocks and the tension in the string. By applying Newton's second law and considering the net forces on both blocks, the acceleration is determined to be the same for both blocks, leading to a formula for acceleration in terms of the masses of the blocks (m1 and m2) and the acceleration due to gravity (g). The script also presents an analogous method of thinking about the problem by imagining the blocks in space, emphasizing that logical approaches in science yield consistent results.

Takeaways
  • 📚 The problem involves deriving the magnitude of acceleration for block 2 using Newton's 2nd Law.
  • 🔢 Block 2 accelerates downward due to its weight being larger than the tension force from block 1.
  • 📈 The acceleration of block 2 has the same magnitude but in the upward direction as block 1, since they are connected by a string.
  • 🎯 To find the acceleration, set up equations considering the net forces acting on both blocks in the vertical direction.
  • 🔄 Use the positive direction downward for block 2's weight and upward for block 1's tension to establish the equations.
  • 🧩 Create two equations, one for each block, to account for the forces and accelerations involved.
  • ⚖️ By adding the equations, the tension terms cancel out, leaving an expression that relates the weights of the blocks to the acceleration.
  • 📊 Solve for the acceleration 'a' by factoring it out and dividing by the sum of the block masses (m1 + m2).
  • 🌌 An alternative approach is to conceptualize the problem in a zero-gravity environment, treating the forces as equivalent to the weights of the blocks.
  • 🔠 The final expression for the magnitude of acceleration is given by 'a = (m2g - m1g) / (m1 + m2)', which is applicable to both block 1 and block 2.
  • 💡 Both methods of solving the problem yield the same result, demonstrating the consistency of physical laws.
Q & A

  • What is the main objective of part b in the video?

    -The main objective of part b is to derive the magnitude of the acceleration of block 2 using Newton's 2nd Law, considering the weights of blocks 1 and 2 and the tension in the string connecting them.

  • In which direction does block 2 accelerate?

    -Block 2 accelerates downwards. This is due to its weight being larger than the tension force in the string, and the weight of block 1 being less than the upward tension force.

  • How is the acceleration of block 1 related to the acceleration of block 2?

    -The acceleration of block 1 is equal in magnitude but opposite in direction to the acceleration of block 2 because they are connected by a string. This means that while block 2 accelerates downwards, block 1 accelerates upwards.

  • What is the initial equation set up for block 2 to find its acceleration?

    -The initial equation for block 2 is m2g (weight of block 2) minus T (tension) equals m2 times the acceleration (a). Here, T is the tension in the string, m2 is the mass of block 2, g is the acceleration due to gravity, and a is the acceleration we want to find.

  • How do we eliminate the tension (T) from the equation?

    -We eliminate the tension (T) by setting up a similar equation for block 1, where the tension is larger than the weight of block 1 (T minus m1g), and then adding both equations to eliminate T by canceling it out.

  • What are the final equations for block 1 and block 2 after canceling out the tension?

    -After canceling out the tension, the final equation is m2g minus m1g equals a times (m2 plus m1), which simplifies to a = (m2g - m1g) / (m2 + m1).

  • What is the significance of solving for acceleration in terms of m1, m2, and g?

    -Solving for acceleration in terms of m1, m2, and g allows us to understand the relationship between the masses of the blocks and the gravitational force, providing a general solution that can be applied to similar problems.

  • How does the analogy of blocks in space help in understanding the problem?

    -The analogy of blocks in space simplifies the problem by imagining the two blocks and the string as one combined mass being pulled in opposite directions by forces equivalent to their weights. This helps in visualizing the net force and understanding how it leads to acceleration.

  • What is the alternative method to find the acceleration of block 2 mentioned in the video?

    -The alternative method is to view the system as a single mass equivalent to m1 plus m2, and then find the acceleration by dividing the net force (m2g - m1g) by the total mass (m1 + m2).

  • How does the video emphasize the consistency of results in science?

    -The video emphasizes that whether using the method of free-body diagrams and Newton's 2nd Law or the analogy of blocks in space, the logical approach to the problem will yield the same result, demonstrating the consistency and reliability of scientific methods.

  • What is the final expression for the magnitude of the acceleration of block 2?

    -The final expression for the magnitude of the acceleration of block 2 is a = (m2g - m1g) / (m2 + m1), where a represents the acceleration, m1 and m2 are the masses of the two blocks, g is the acceleration due to gravity, and the sum m2 + m1 represents the total mass of the system.

Outlines
00:00
📚 Introduction to Block 2's Acceleration

The paragraph begins by introducing the task of deriving the magnitude of acceleration for block 2, using the variables m1, m2, and g. It encourages viewers to pause the video and attempt the problem independently. A brief recap of part 1 is provided, followed by an explanation of the free-body diagrams that will be used to determine the acceleration. The key concept is that the acceleration of both blocks, connected by a string, will be the same in magnitude but opposite in direction. The paragraph concludes with an application of Newton's second law to block 2, setting up the equation for net forces and acceleration in the vertical direction.

05:00
🔍 Solving for Acceleration with Block 1's Equation

This paragraph continues the process of solving for block 2's acceleration by setting up a similar equation for block 1. It explains how the tension in the string is equal for both blocks and how this can be used to eliminate the variable T (tension) by combining the equations for both blocks. The paragraph then simplifies the combined equation to isolate the variable a (acceleration), ultimately solving for a in terms of m1, m2, and g. It also offers an alternative perspective by imagining the blocks in space, emphasizing that the logical approach to the problem will yield the same result regardless of the method used.

Mindmap
Keywords
💡Acceleration
Acceleration is a physical quantity that describes the rate of change of velocity of an object. In the context of the video, it refers to the rate at which the blocks are speeding up or slowing down in the vertical direction due to the forces acting on them. The video focuses on deriving the magnitude of acceleration for block 2, which is a key concept in understanding the dynamics of the system.
💡Free-body diagrams
Free-body diagrams are graphical representations that show all the forces acting on an object in isolation. They are a fundamental tool in physics for analyzing mechanical systems. In the video, the free-body diagrams are used to visualize and calculate the forces on blocks 1 and 2, which helps in determining their accelerations.
💡Newton's 2nd Law
Newton's second law of motion states that the net force acting on an object is equal to the mass of the object multiplied by its acceleration (F = ma). This law is a cornerstone of classical mechanics and is used in the video to relate the forces acting on the blocks to their resulting accelerations.
💡Tension
Tension is a force that is transmitted through a string, rope, cable, or any similar medium that is pulled tight by the forces acting on the objects at its ends. In the video, tension is one of the key forces that need to be accounted for when analyzing the motion of the two blocks connected by a string, as it influences the acceleration of both blocks.
💡Weight
Weight is the force exerted on an object due to gravity. It is calculated as the product of an object's mass and the acceleration due to gravity (W = mg). In the video, the weight of the blocks is a critical force that contributes to their acceleration when connected in a pulley system.
💡Net force
Net force is the vector sum of all the individual forces acting on an object. It is the force that will actually cause the object to accelerate according to Newton's second law. In the video, the net force on block 2 is the difference between its weight and the tension in the string, which determines its acceleration.
💡Mass
Mass is a measure of the amount of matter in an object, and it is an intrinsic property that determines the object's inertia and the weight it experiences under gravity. In the video, the masses of blocks 1 and 2 are essential parameters used in the equations to calculate the acceleration of the blocks.
💡Gravitational field
A gravitational field is the region around a massive object where its gravitational force affects other objects. In the context of the video, the gravitational field is what causes the weight of the blocks and influences their motion.
💡Pulleys
Pulleys are mechanical devices used to change the direction and/or magnitude of a force applied to them, often used in systems involving ropes or strings. In the video, the pulleys are part of the setup that allows the blocks to be connected by a string and move in response to the forces acting on them.
💡Vector
A vector is a quantity that has both magnitude and direction. In physics, vectors are used to describe forces, velocities, and accelerations. In the video, the concept of vectors is crucial for understanding the net force acting on the blocks and the direction of their acceleration.
💡Equilibrium
Equilibrium refers to a state where the forces acting on a system are balanced, resulting in no net force and no acceleration. While the video does not explicitly mention equilibrium, the concept is related to the analysis of forces and motion.
Highlights

Derive the magnitude of acceleration of block 2 using Newton's 2nd Law.

Acceleration will be downwards due to the weight of block 2 being larger than the weight of block 1.

The magnitude of acceleration for both blocks connected by the string will be the same.

The net force on block 2 is the weight minus the tension, directed downwards.

The equation for block 2's net force is m2g (weight) minus T (tension) equals m2 times acceleration.

To solve for acceleration, set up a similar equation for block 1, considering the tension and weight.

By adding the equations for block 1 and block 2, the tension terms cancel out, leaving an equation with the masses and acceleration.

The acceleration a can be factored out, leading to a formula for a in terms of m1, m2, and g.

The final formula for acceleration is a = (m2g - m1g) / (m1 + m2).

An alternative approach is to consider the blocks as one combined mass in a hypothetical scenario of drifting in space.

In the space analogy, the net force is the difference between the forces acting on m1 and m2.

The acceleration is found by dividing the net force by the total mass (m1 + m2).

Both methods yield the same result, demonstrating the consistency of physical laws.

The problem-solving process emphasizes the importance of understanding the direction of forces and acceleration.

The concept of free-body diagrams is crucial for visualizing and solving the problem.

The weight of the blocks and the tension in the string are key components in the equations.

The process of eliminating variables, such as tension T, is essential for solving complex problems.

The problem can be approached with different methods, showcasing the versatility of physics.

Transcripts

Browse More Related Video

2015 AP Physics 1 free response 1a

AP Physics Workbook 2.E Newton's Second and Third Laws

2015 AP Physics 1 free response 1c

Newton's Second Law Exam Question: Two-body systems Pulley practice

2019 AP Physics 1 Solutions Free Response #2

2019 #2 Free Response Question - AP Physics 1 - Exam Solution

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics ConceptsNewton's LawsAcceleration CalculationFree-Body DiagramsMechanical ForcesTension AnalysisMotional AnalysisEducational ContentProblem SolvingScience Education