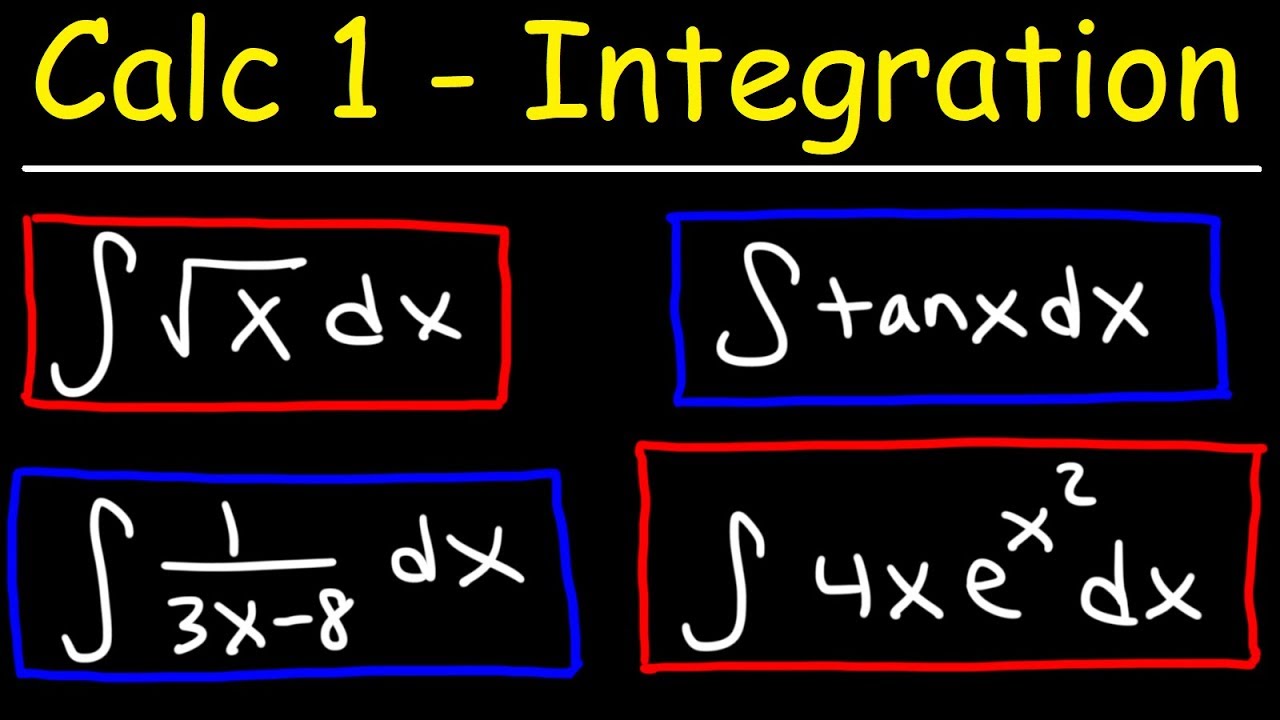
Calculus 1: Indefinite Integrals and the Net Change Theorem (Section 5.4) | Math with Professor V

Math with Professor V

26 Apr 202030:23

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video delves into the fundamentals of indefinite integrals and the Net Change Theorem, building upon the previously discussed Fundamental Theorem of Calculus. It clarifies how indefinite integrals represent antiderivatives, emphasizing the process of integration without limits and the importance of including the constant of integration (C). Through a series of examples, the video demonstrates how to manipulate and integrate various functions, including polynomial, trigonometric, and piecewise functions. It concludes with practical applications of the Net Change Theorem in physics and other fields, showcasing how integrals can represent the net change in a quantity over an interval, thus bridging theoretical calculus with real-world scenarios.

Takeaways

📚 The fundamental theorem of calculus establishes a connection between antiderivatives and definite integrals, leading to the concept of indefinite integrals for computing antiderivatives.
📈 Indefinite integrals, represented without limits of integration, are used to find the antiderivative of a function, emphasizing the necessity of including the constant of integration (+C).
🔢 Basic rules for finding antiderivatives include pulling out constants from integrals, applying the power rule by increasing the exponent by 1 and dividing by the new exponent, and remembering trigonometric function integrals.
📝 Working through examples is key to understanding the process of finding indefinite integrals, showcasing the application of rules for constants, power functions, and trigonometric functions.
🎲 The Net Change Theorem relates the integral of a rate of change (derivative) over an interval to the net change in the function, allowing the calculation of quantities like displacement and charge over time.
🚀 Real-world applications of indefinite integrals and the Net Change Theorem include calculating the total change in quantities such as charge in a wire and population growth over specific intervals.
💡 Understanding piecewise functions and their absolute values is crucial for solving integrals involving absolute values, which often require splitting the integral at points where the function's behavior changes.
🤖 The distinction between displacement and total distance traveled is important in motion problems, with displacement calculated directly from the velocity function and total distance requiring the absolute value of velocity.
🏃‍♂️ Particle motion examples illustrate the application of indefinite integrals to calculate displacement and total distance, highlighting the importance of evaluating the velocity function over the specified interval.
💾 Techniques and shortcuts, such as using piecewise functions to manage absolute values in integrals, can streamline the calculation process, but should be used with understanding of the underlying concepts.

Q & A

What is the main concept discussed in the transcript?
-The main concept discussed in the transcript is indefinite integrals, their calculation, and the application of the net change theorem in various scenarios.
How does the fundamental theorem of calculus connect anti-derivatives and definite integrals?
-The fundamental theorem of calculus establishes a connection between anti-derivatives and definite integrals by stating that if a function has an antiderivative, then the definite integral of the function from one point to another is equal to the antiderivative evaluated at the upper limit of integration minus the antiderivative evaluated at the lower limit.
What is the difference between a definite integral and an indefinite integral?
-A definite integral represents the area under the curve of a function over a specified interval and includes limits of integration, whereas an indefinite integral represents the antiderivative of a function without any specified limits of integration and includes a constant 'C' to account for the infinite number of possible antiderivatives.
What is the general process for finding the indefinite integral of a function?
-The general process for finding the indefinite integral of a function involves applying the rules of anti-differentiation to the function, such as increasing the exponent by 1 and dividing by the new exponent for power rules, and adding the constant 'C' at the end to account for the family of functions that can be antiderivatives of the given function.
How does the net change theorem relate to the concept of charge and current in a wire?
-The net change theorem relates to the concept of charge and current in a wire by stating that the integral of the current (rate of change of charge) over a time interval is equal to the net change in the charge over that same time interval.
What is the difference between displacement and distance traveled?
-Displacement is the change in position from the initial point to the final point, while distance traveled is the total length of the path taken by an object, accounting for any changes in direction.
How do you calculate the total distance traveled by an object?
-To calculate the total distance traveled by an object, you need to integrate the absolute value of the velocity function over the given time interval, ensuring that the negative and positive displacements do not cancel each other out.
What is the significance of the constant 'C' in the indefinite integral?
-The constant 'C' in the indefinite integral represents the family of antiderivatives that differ by a constant, reflecting the fact that there are infinitely many functions that can be antiderivatives of a given function.
How does the process of anti-differentiation help in solving real-world problems?
-Anti-differentiation, through the process of finding indefinite integrals, helps in solving real-world problems by allowing us to determine quantities such as net changes in physical quantities (like charge, population, or elevation), displacement and distance traveled, and other accumulated values over a period of time or along a path.
What is the role of trigonometric identities in simplifying integrals involving trigonometric functions?
-Trigonometric identities play a crucial role in simplifying integrals involving trigonometric functions by allowing us to rewrite complex expressions in terms of simpler functions, which can then be more easily integrated.
How does the process of splitting the integration interval help in dealing with absolute value functions?
-Splitting the integration interval helps in dealing with absolute value functions by allowing us to consider the function in a piecewise manner, where we can remove the absolute value and deal with the function in a simpler form over each subinterval where the function is either positive or negative.

Outlines

00:00

📘 Introduction to Indefinite Integrals and the Net Change Theorem

This section delves into the concept of indefinite integrals, explaining their notation and relationship with antiderivatives, following the fundamental theorem of calculus. The discussion covers how indefinite integrals, represented without limits of integration, are used to compute antiderivatives of functions. It introduces basic rules for integration, including handling constants, trigonometric functions, and the general power rule, emphasizing the importance of adding a constant ('C') to the result. Various examples illustrate how to perform integration with different functions, reinforcing the method of increasing the exponent by one and dividing by the new exponent, and showcasing the application of these rules to solve integration problems.

05:05

🔍 Exploring Definite Integrals and Area Computation

Transitioning to definite integrals, this segment explains how they differ from indefinite integrals by having limits of integration, which means their computation results in a constant rather than a function with an arbitrary constant 'C'. Through examples involving polynomial and trigonometric functions, the narrative demonstrates how to expand, distribute, and integrate expressions within defined limits to compute areas under curves. It also touches on the significance of rewriting integrands in an 'integral-friendly format' and the methodical approach to evaluating definite integrals, leading to a discussion on how integration techniques apply to computing areas under curves between specified limits.

10:07

📐 Advanced Integration Techniques and Strategies

This part focuses on advanced integration techniques and strategies for handling more complex functions, including those involving absolute values, trigonometric identities, and piecewise functions. It stresses the importance of cleaning up the integrand for easier integration and showcases how trigonometric identities can simplify integration tasks. Through step-by-step examples, the narrative covers various scenarios, such as integrating functions with absolute values by defining them piecewise, and applying trigonometric identities to simplify integrands before integration. The explanations aim to equip learners with the skills to tackle a wide range of integration problems by adopting strategic approaches and understanding the underlying mathematical principles.

15:08

🧮 Application of Integration to Physical Concepts and Theorems

The narrative transitions to the practical application of integration in physics and other fields, introducing the Net Change Theorem. This theorem relates the integral of a rate of change (derivative) to the net change in the function, offering a foundation for understanding how integration can quantify changes over intervals in various contexts, such as current in a wire, honeybee population growth, and trail elevation changes. Examples illustrate the theorem's utility in calculating the net change in quantities like charge, population, and elevation, highlighting integration's role in translating mathematical models into meaningful physical interpretations.

20:12

📉 Particle Motion and the Distinction Between Displacement and Distance Traveled

Focusing on particle motion, this section elucidates the distinction between displacement and total distance traveled, demonstrating how integration applies to each concept. Using a velocity function example, it explains that displacement can be computed directly by integrating the velocity function over a time interval, whereas calculating the total distance traveled requires integrating the absolute value of the velocity function to account for direction changes. The discussion includes a detailed example illustrating the process of computing both displacement and distance traveled, emphasizing the importance of considering the velocity function's sign changes to accurately determine distance.

Mindmap

Keywords

💡Indefinite Integrals

Indefinite integrals, also known as antiderivatives, represent the general form of integrating a function without specific limits. They are fundamental to calculus as they provide the reverse operation of differentiation. The notation for an indefinite integral is the integral sign without limits of integration, emphasizing the 'indefinite' aspect, which means the result includes an arbitrary constant, denoted as +C. This concept connects deeply with the fundamental theorem of calculus, bridging differentiation and integration, illustrated by examples of integrating functions like x^2 or sine functions without specifying bounds.

💡Net Change Theorem

The Net Change Theorem is a principle that connects the integral of a function over an interval to the total change in the function's antiderivative over that interval. It essentially states that the definite integral of a rate of change gives the net change in the original function. This theorem has practical applications in physics, economics, and other fields, as seen in examples involving calculating the change in charge in a wire or the change in population size over time. The theorem underscores the integral's role in quantifying changes between two points.

💡Fundamental Theorem of Calculus

The Fundamental Theorem of Calculus establishes a connection between differentiation and integration, two core operations in calculus. It asserts that if a function is continuous over an interval and is the derivative of another function within that interval, the definite integral of the function over the interval equals the difference in the values of the antiderivative at the bounds of the interval. This theorem is foundational because it not only provides a method to evaluate definite integrals but also conceptualizes integration and differentiation as inverse processes.

💡Antiderivative

An antiderivative of a function is another function whose derivative is the original function. The process of finding antiderivatives is known as antidifferentiation and is synonymous with integrating a function indefinitely. This concept is pivotal in calculus, allowing for the calculation of areas under curves, solving differential equations, and more. The antiderivative is not unique; hence, the integration constant +C is added to account for all possible antiderivatives. The video explores this concept through examples, emphasizing its relevance to indefinite integrals and the net change theorem.

💡Definite Integrals

Definite integrals calculate the net area under a curve between two specific points, providing a precise measurement of the total accumulation of a quantity. They are represented by the integral sign with upper and lower limits of integration. This concept contrasts with indefinite integrals by yielding a numerical value rather than a function, as it accounts for the exact limits within which the integration is performed. Examples in the video highlight calculating areas under curves and their application to real-world scenarios, like computing distances.

💡Integration Techniques

Integration techniques refer to the various methods used to perform integrations, such as substitution, integration by parts, and partial fraction decomposition. These techniques are essential for solving integrals that are not immediately apparent or straightforward. The video discusses how to manipulate expressions to make them 'integration-friendly', illustrating this with examples that involve rearranging terms or applying identities to simplify the integral before applying a specific technique.

💡Limits of Integration

Limits of integration define the bounds within which the integration of a function is to be calculated, crucial for determining definite integrals. They specify the interval over which the function's accumulation or area under the curve is considered, directly affecting the result of the integration. In the context of the video, the transition from indefinite to definite integrals is marked by the introduction of these limits, as seen in examples where evaluating the integral within specified bounds yields a numerical value representing physical quantities like area or change.

💡Trigonometric Integrals

Trigonometric integrals involve the integration of trigonometric functions and are a staple in calculus due to their prevalence in modeling periodic phenomena. The video delves into examples of integrating functions like secant squared or sine, utilizing trigonometric identities to simplify expressions for integration. These examples underscore the importance of understanding trigonometric derivatives and their inverses for effective antidifferentiation and integration in various applications.

💡Piecewise Functions

Piecewise functions are defined by different expressions for different parts of their domain. In the context of integration, especially with absolute value functions, they require a piecewise approach to integrate over intervals where the function's behavior changes. The video illustrates this with an example involving the absolute value of a linear expression, emphasizing the need to break the integration into parts corresponding to where the function is positive or negative and then integrate each part separately.

💡Velocity and Displacement

Velocity and displacement relate to motion, where velocity is the rate of change of position, and displacement measures the change in position over a time interval. Integrating a velocity function over time gives the displacement, as shown in examples where the net change theorem is applied to calculate the motion of particles. This application illustrates how integration can be used to find the total change in position, offering insights into the object's movement, including direction and magnitude, over specified time intervals.

Highlights

Introduction to indefinite integrals and the connection to the fundamental theorem of calculus.

Explanation of indefinite integrals and their notation without limits of integration.

General power rule for anti-differentiation and importance of adding '+C' to the indefinite integral.

Examples of calculating indefinite integrals with various functions, highlighting step-by-step processes.

Introduction to the net change theorem and its application in calculating net changes through integration.

Explanation of how to handle absolute value functions in integration, including rewriting them as piecewise functions.

Application of the net change theorem in physical contexts, such as current in a wire and charge over time.

Real-world example involving honeybee population growth and how integration applies to biological contexts.

Application of the net change theorem to calculate changes in elevation along a trail.

Discussion on displacement and distance traveled in particle motion, emphasizing the role of integration.

Example calculation of displacement using velocity function integration.

Explanation of calculating total distance traveled by taking the absolute value of the velocity function before integration.

Technique for handling integrals involving absolute values by splitting the integral at points where the function changes sign.

Final example illustrating a simplified method for calculating total distance traveled without testing intervals.

Summary of indefinite integrals, the net change theorem, and their applications in various contexts.

Transcripts

Browse More Related Video

Calculus Chapter 3 Lecture 26 The FTIC

Evaluating Indefinite Integrals

AP Calculus AB: Lesson 6.5 Accumulation of Change

AP Calculus AB: Lesson 6.3 Fundamental Theorem of Calculus Part 1

The Fundamental Theorem of Calculus

Calculus 1 - Integration & Antiderivatives

Related Tags

Calculus Integrals Net Change Antiderivatives Trigonometry Physics Applications Mathematical Concepts Educational Content Math Tutorial Mathematics Learning