Lesson 5 - Finding The Resultant Of Two Forces, Part 1 (Engineering Mechanics Statics)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRThis lesson in engineering mechanics focuses on the practice of adding vectors, particularly conceptualizing them as forces acting on a point. The instructor introduces two forces, F1 and F2, with F1 exerting 500 Newtons horizontally and F2 exerting 800 Newtons at a 56-degree angle from the horizontal. The goal is to find the resultant vector's magnitude and direction from the x-axis by adding these vectors together. The instructor discusses various methods for vector addition, including the parallelogram and triangle rules, and emphasizes the importance of predicting the result for a sanity check before calculation. This approach ensures a comprehensive understanding of vectors' magnitude and direction, crucial for solving problems in engineering mechanics.

Takeaways
  • 📐 The lesson focuses on the addition of vectors, specifically considering them as forces.
  • 🤔 Vectors can represent various physical quantities, but in this context, they are treated as forces acting on a point.
  • 📈 Two forces, F1 and F2, are introduced with F1 being 500 Newtons and F2 being 800 Newtons at a 56° angle from the horizontal.
  • 🎯 The goal is to find the resultant vector, which includes both magnitude and direction (angle Theta R from the x-axis).
  • 📊 To solve vector addition problems, multiple methods are available, including graphical approaches like the parallelogram rule and triangle rule, as well as trigonometric calculations.
  • 🤝 The instructor emphasizes that there is not just one way to solve a problem and encourages finding alternative solutions.
  • 🔍 Before starting calculations, it's beneficial to make an educated guess about the outcome to perform a sanity check on the final result.
  • 🏷️ A vector is fully defined only by its magnitude and direction; knowing one without the other does not constitute a complete solution.
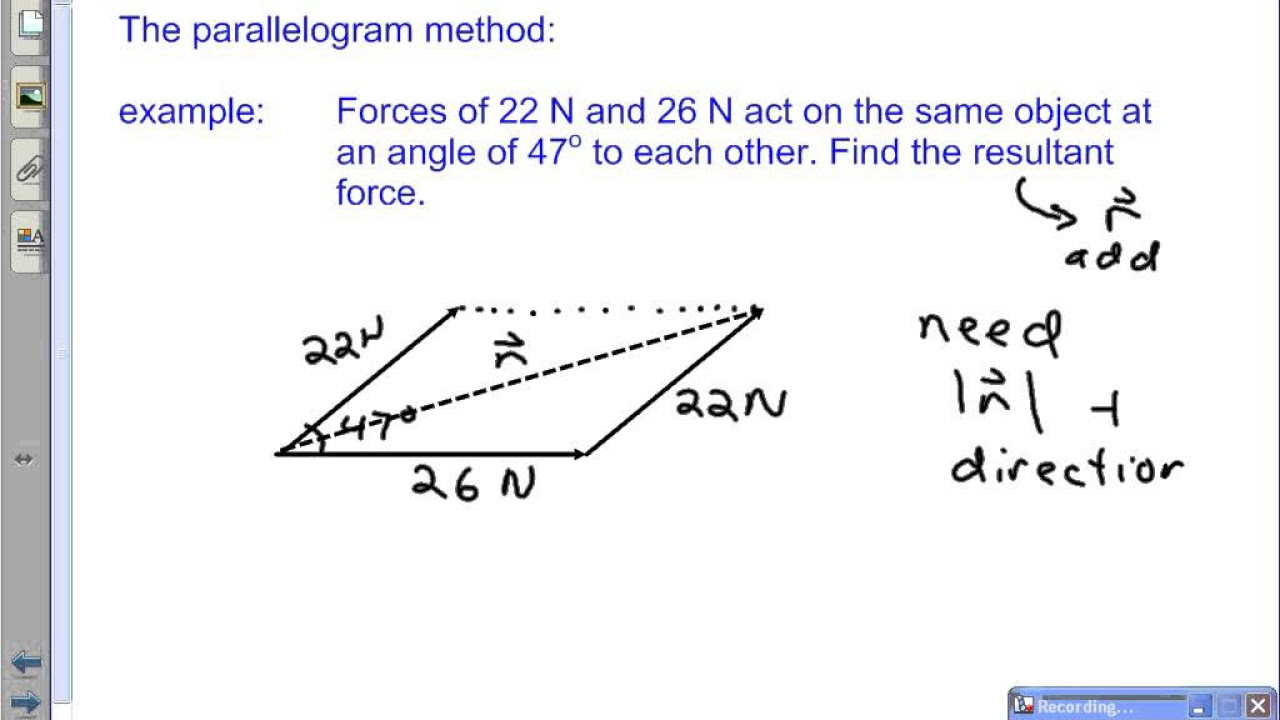
  • 🖌️ The process begins by drawing a parallelogram with the given vectors as two of its sides to visualize the resultant.
  • 🛠️ The instructor suggests that the resultant should be expected to have a direction倾斜 towards F2, given the disparity in magnitudes between F1 and F2.
  • 📋 The lesson underscores the importance of precision and accuracy in vector calculations, acknowledging potential minor discrepancies due to decimal truncation.
Q & A

  • What is the primary focus of the lesson in the provided transcript?

    -The primary focus of the lesson is on adding vectors, specifically in the context of engineering mechanics, where vectors are mostly considered as forces.

  • How are vectors represented in this engineering mechanics lesson?

    -Vectors are represented as forces acting on a point, with one force (F1) pointing to the right with 500 Newtons and another force (F2) pointing upwards at a 56-degree angle from the horizontal with 800 Newtons.

  • What are the goals when adding vectors according to the transcript?

    -The goal is to find the resultant vector by adding the vectors together, which includes determining the magnitude and the direction angle (Theta R) from the x-axis of the resultant vector.

  • Why is it important to find both magnitude and direction when solving for a vector sum?

    -It's important because a vector is defined by both its magnitude and direction. Having only one without the other means the problem is not fully solved, as vectors represent quantities that have both size and direction.

  • What does the lecturer suggest doing before calculating vector addition?

    -The lecturer suggests estimating what the resultant vector should look like based on the direction and magnitude of the forces involved. This helps in doing a sanity check of the calculated answer to ensure it makes sense.

  • How does the transcript propose one should begin solving vector addition problems graphically?

    -The transcript suggests starting by drawing a parallelogram with the given vectors, using the vectors as two sides of the parallelogram, to visually assist in the process of adding vectors.

  • What educational approach does the lecturer emphasize for solving vector problems?

    -The lecturer emphasizes that there are multiple ways to solve vector problems, including the parallelogram rule, triangle rule, and trigonometry methods, and encourages finding and using the method that works best for the student.

  • What is the lecturer's stance on finding a different method to solve the vector problem than the one presented?

    -The lecturer encourages finding different methods to solve the problem and applauds students who can find an alternative approach, as long as it leads to a similar magnitude and direction within a small error margin.

  • What does the lecturer predict about the direction of the resultant vector before calculation?

    -The lecturer predicts that the resultant vector will likely be in an upward direction, tilted towards Force F2, since F2 has a larger magnitude than F1 and is angled upwards.

  • What does drawing a parallelogram with the forces as sides help visualize in vector addition?

    -Drawing a parallelogram helps visualize the potential direction and magnitude of the resultant vector by extending the given vectors into a parallelogram shape, aiding in understanding how the forces combine.

Outlines
00:00
🔍 Introduction to Vector Addition in Engineering Mechanics

This segment introduces the concept of vector addition within the context of engineering mechanics, emphasizing its application to forces. The instructor begins by presenting a problem that involves adding two vectors: Vector F1, which is directed horizontally to the right with a magnitude of 500 Newtons, and Vector F2, which acts at a 56-degree angle from the horizontal with a magnitude of 800 Newtons. The objective is to find the resultant vector, characterized by both its magnitude and direction relative to the x-axis. The importance of determining both the magnitude and the angle to fully resolve a vector is highlighted. The instructor also discusses multiple methodologies for vector addition, including graphical methods like the parallelogram and triangle rules, as well as trigonometric approaches like the law of sines and cosines. Emphasis is placed on the diversity of valid approaches to solving vector problems and the importance of making an intuitive estimate of the resultant vector's direction before performing detailed calculations.

Mindmap
Keywords
💡Vector
In the context of the video, a vector represents a quantity that has both magnitude and direction, often used to model forces in physics. The instructor emphasizes that vectors can represent various concepts, but in this lesson, they are primarily considered as forces acting on a point. For example, vectors F1 and F2 in the video are forces with specific magnitudes (in Newtons) and directions (either horizontal or at an angle).
💡Magnitude
Magnitude refers to the size or length of a vector and is a measure of the force's strength in the video. It is a scalar quantity, meaning it has only magnitude and no direction. The instructor mentions that finding the magnitude of the resultant vector, which combines the effects of F1 and F2, is a crucial part of solving the problem. For instance, F1 has a magnitude of 500 Newtons.
💡Resultant
The resultant is the single vector that has the same effect as two or more vectors acting together. In the lesson, the instructor aims to find the resultant of forces F1 and F2, meaning the combined effect of these forces in terms of both magnitude and direction. The resultant vector is significant because it simplifies the vector analysis by replacing multiple forces with one equivalent force.
💡Angle
In vector analysis, the angle of a vector often refers to its direction relative to a reference axis, typically the horizontal or x-axis. The video discusses finding the angle Theta R for the resultant vector, which indicates its direction. This angle helps in fully defining the vector's orientation in space and is essential for understanding and predicting the behavior of forces in mechanics.
💡Parallelogram Rule
The parallelogram rule is a method for adding two vectors graphically by arranging them to form adjacent sides of a parallelogram. The diagonal of the parallelogram then represents the resultant vector. In the video, the instructor mentions this rule as one of the methods for vector addition, highlighting its usefulness in visually determining the magnitude and direction of the combined forces.
💡Trigonometry
Trigonometry is a branch of mathematics dealing with the relationships between the angles and sides of triangles. The instructor mentions trigonometric principles like the law of sines and cosines in the context of finding vector magnitudes and angles. These principles are fundamental for solving vector problems in engineering mechanics, especially when dealing with forces at angles.
💡Direction
Direction in vector analysis indicates where the vector points in space. The video emphasizes that a vector's direction, along with its magnitude, fully defines it. For example, force F2 is not only described by its 800 Newtons of magnitude but also by its direction at a 56° angle from the horizontal, which together specify its complete vectorial nature.
💡Triangle Rule
The triangle rule, also mentioned in the video, is a method for adding vectors by connecting them head-to-tail in a chain, with the resultant vector drawn from the tail of the first vector to the head of the last. This rule is particularly helpful in the graphical representation and addition of vectors, illustrating the combined effect of multiple forces acting on a point.
💡Sanity Check
A sanity check, as discussed in the video, is a basic test to check if the results of a calculation are reasonable or make logical sense. The instructor suggests estimating the direction and magnitude of the resultant force before performing detailed calculations to predict the expected outcome and verify the final result's accuracy.
💡Graphical Method
Graphical methods in vector addition involve using diagrams to visually represent and solve vector problems. The video discusses using such methods, like the parallelogram or triangle rule, to add forces represented as vectors. These methods provide intuitive and visual insights into the nature and result of combining different vectors.
Highlights

The lesson focuses on the practice of adding vectors, specifically in the context of engineering mechanics.

Vectors are being considered as forces in this part of the class, emphasizing their physical representation.

Vector F1 is described, with a magnitude of 500 Newtons acting to the right.

Vector F2 is introduced, an 800 Newton force at a 56° angle from the horizontal.

The goal is to find the resultant vector, which includes both magnitude and direction (angle Theta R).

The importance of understanding both magnitude and direction when solving vector problems is emphasized.

Multiple methods for solving vector problems are acknowledged, including the parallelogram rule and triangle rule.

Trigonometry, such as the law of sines, law of cosines, and basic definitions of sine, cosine, and tangent, is mentioned as a tool for vector addition.

The instructor shares their approach to the problem, encouraging students to find their own methods as well.

A sanity check is recommended before solving, to estimate the result and verify its sensibility.

The concept of the resultant force's direction is discussed, with an expectation that it will tilt towards the larger force component.

A parallelogram is drawn to visually represent the vector addition, with the forces as two sides.

The lesson stresses the flexibility in solving vector problems and the acceptability of small errors due to decimal truncation.

The magnitude and direction of the resultant vector must be consistent with the calculated values to confirm a correct solution.

The process of adding vectors is not only about the mathematical calculation but also involves visual and intuitive estimation.

The lesson concludes with a practical example of how forces act on a point, reinforcing the concept of vector addition in a real-world context.

Transcripts

Browse More Related Video

Lesson 6 - Finding The Resultant Of Two Forces, Part 2 (Engineering Mechanics Statics)

How To Use The Parallelogram Method To Find The Resultant Vector

Calculating the Resultant force Using Parallelogram Law, ለኢትዮጵያውያን

1-2 C Vector Addition - 2 (Parallelogram Method)

Vectors Full Topic -Physics

Resultant vector | Vector addition: Grade 10 Physics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Vector AdditionEngineering MechanicsForce VectorsResultant MagnitudeDirection AngleTrigonometryParallelogram RuleGraphical SolutionSanity CheckPhysics Concepts