Calculating the Resultant force Using Parallelogram Law, ለኢትዮጵያውያን
TLDRIn this educational video, the presenter demonstrates how to calculate the magnitude and direction of a resultant force using the parallelogram law. The scenario involves a screw subjected to two forces, F1 and F2, with F1 being 100 Newtons at an angle of 15 degrees to the horizontal axis, and F2 being 150 Newtons at an angle of 10 degrees to the vertical axis. The presenter explains the process step by step, starting with constructing a parallelogram to represent the forces. By applying the laws of cosines and sines, the magnitude of the resultant force is determined to be 212.6 Newtons. The direction is calculated using trigonometric relationships, with the angle between the horizontal axis and the resultant force (phi) being 54.8 degrees. The video concludes with the presentation of the resultant vector in polar form, providing a clear and concise explanation of a fundamental concept in physics.
Takeaways
- 📐 The video demonstrates how to determine the magnitude and direction of a resultant vector using the parallelogram law.
- 🔍 The problem involves calculating the resultant force on a screw subjected to two forces, F1 and F2.
- 📏 F1 is given as 100 Newtons, and F2 is 150 Newtons, with specific angles to the horizontal and vertical axes.
- 🎚️ The angle between F1 and the horizontal x-axis is 15 degrees, and the angle between F2 and the vertical y-axis is 10 degrees.
- 🔢 The angle between F1 and F2, denoted as alpha, is calculated to be 65 degrees.
- 📐 The internal angles of the parallelogram formed by F1 and F2 are such that their sum equals 360 degrees, which helps to find the angle beta.
- 🧮 Using the law of cosines, the magnitude of the resultant force (FR) is calculated to be 212.6 Newtons.
- 📐 To find the direction of the resultant force, the angle theta between F1 and FR is determined using trigonometry.
- 📐 The angle phi, which is the direction of the resultant vector from the horizontal axis, is calculated by adding the angle between F1 and the horizontal axis to theta.
- 📈 The final direction of the resultant vector is given as 54.8 degrees from the horizontal axis.
- 📝 The resultant vector is represented in polar form, showing both its magnitude (212.6 Newtons) and direction (54.8 degrees).
Q & A
What is the main topic of the video?
-The video explains how to determine the magnitude and direction of the resultant vector using the parallelogram law.
What are the two forces acting on the screw in the given problem?
-The two forces acting on the screw are F1, which is 100 Newtons, and F2, which is 150 Newtons.
What are the angles given for F1 and F2 with respect to the horizontal and vertical axes?
-The angle between F1 and the horizontal x-axis is 15 degrees, and the angle between F2 and the vertical y-axis is 10 degrees.
How is the angle between F1 and F2 calculated?
-The angle between F1 and F2, denoted as alpha, is calculated as 90 degrees minus 15 degrees plus 10 degrees, which equals 65 degrees.
What is the angle beta in the parallelogram formed by F1 and F2?
-The angle beta is calculated as 360 degrees minus 2 times 65 degrees, which simplifies to 115 degrees.
What is the formula used to determine the magnitude of the resultant force?
-The formula used to determine the magnitude of the resultant force (FR) is the square root of (F1 squared plus F2 squared minus 2 times F1 times F2 times cos(beta)).
What is the calculated magnitude of the resultant force?
-The calculated magnitude of the resultant force is 212.6 Newtons.
How is the direction of the resultant force determined?
-The direction of the resultant force is determined by calculating the angle theta between F1 and the resultant force, and then adding the angle between the horizontal axis and F1 to find the total direction angle phi.
What is the calculated direction of the resultant force?
-The calculated direction of the resultant force is 54.8 degrees from the horizontal axis.
What is the polar form representation of the resultant vector?
-The polar form representation of the resultant vector is a combination of its magnitude (212.6 Newtons) and its direction angle (54.8 degrees from the horizontal).
What is the law of cosines used for in this context?
-In this context, the law of cosines is used to calculate the magnitude of the resultant vector from the triangle formed by the two forces and the resultant vector.
How is the law of sines applied in determining the angle theta?
-The law of sines is applied by setting up a ratio between the sides of the triangle formed by the forces, where F2 is divided by the sine of theta equals the resultant force divided by the sine of the angle beta, to solve for theta.
Outlines
🔍 Determining Resultant Vector Using Parallelogram Law
The video begins by introducing the topic of determining the magnitude and direction of a resultant vector using the parallelogram law. The scenario involves a screw subjected to two forces, F1 and F2, with specific magnitudes and angles relative to the axes. The process involves visualizing these forces as vectors and constructing a parallelogram with them. The angles between the forces and the resultant force are calculated, and the internal angles of the parallelogram are used to find the necessary angles for the calculation. The law of cosines is then applied to find the magnitude of the resultant force, which is determined to be 212.6 Newtons. The video provides a step-by-step approach to this physical problem, emphasizing the application of vector addition principles.
📐 Calculating the Direction of the Resultant Force
Following the calculation of the magnitude of the resultant force, the video moves on to determine its direction. This is done by considering the triangle formed by the forces and the resultant vector. The angle theta, which is the angle between F1 and the resultant force, is calculated using the law of sines. With known values for F2, the resultant force, and the angle beta, the sine of theta is determined, which leads to finding theta to be 39.8 degrees. The final step is to calculate the angle phi, which is the angle from the horizontal axis to the resultant vector. By adding the angle between F1 and the horizontal axis (15 degrees) to theta, phi is found to be 54.8 degrees. The video concludes with the representation of the resultant vector in polar form, indicating both its magnitude (212.6 Newtons) and direction (54.8 degrees from the horizontal).
Mindmap
Keywords
💡Resultant Vector
💡Parallelogram Law
💡Forces F1 and F2
💡Angle Theta (θ)
💡Angle Alpha (α)
💡Angle Beta (β)
💡Law of Cosines
💡Law of Sines
💡Magnitude
💡Direction
💡Polar Form
Highlights
The video demonstrates how to determine the magnitude and direction of a resultant vector using the parallelogram law.
A screw is subjected to two forces, F1 and F2, and the task is to find the resultant force's magnitude and direction.
F1 is 100 Newtons, F2 is 150 Newtons, with F1 at a 15-degree angle to the horizontal axis and F2 at a 10-degree angle to the vertical axis.
The parallelogram rule is used to represent the two forces with a single resultant force and direction angle theta.
The angle between F1 and the resultant force FR is denoted as theta, and the angle between F1 and F2 is calculated to be 65 degrees.
The internal angles of the parallelogram are used to calculate another angle, beta, which is found to be 115 degrees.
The Law of Cosines is applied to find the magnitude of the resultant vector FR, which is calculated to be 212.6 Newtons.
The direction of the resultant force is determined by calculating the angle theta, which is found using the Law of Sines.
Theta is calculated to be 39.8 degrees, which is the angle between F1 and the resultant force FR.
The angle phi, which represents the direction of the resultant vector from the horizontal axis, is calculated to be 54.8 degrees.
The resultant vector is represented in polar form with its magnitude and direction, providing a complete solution to the problem.
The video provides a step-by-step guide on constructing a parallelogram to visualize and solve for the resultant force.
Trigonometric functions are used extensively to solve for the angles and magnitudes involved in the problem.
The Law of Cosines is crucial for determining the magnitude of the resultant force from the given forces and their angles.
The Law of Sines is essential for finding the direction of the resultant force by relating the known sides and angles of the triangle.
The process involves understanding the geometric representation of forces and their vector addition through the parallelogram law.
The video concludes with a clear explanation of how to represent the final answer in polar form, including both magnitude and direction.
The practical application of vector addition using the parallelogram law is demonstrated through a real-world example of a screw subjected to forces.
This method can be applied to various engineering and physics problems involving force analysis and vector summation.
Transcripts
Browse More Related Video

Lesson 6 - Finding The Resultant Of Two Forces, Part 2 (Engineering Mechanics Statics)

How To Use The Parallelogram Method To Find The Resultant Vector
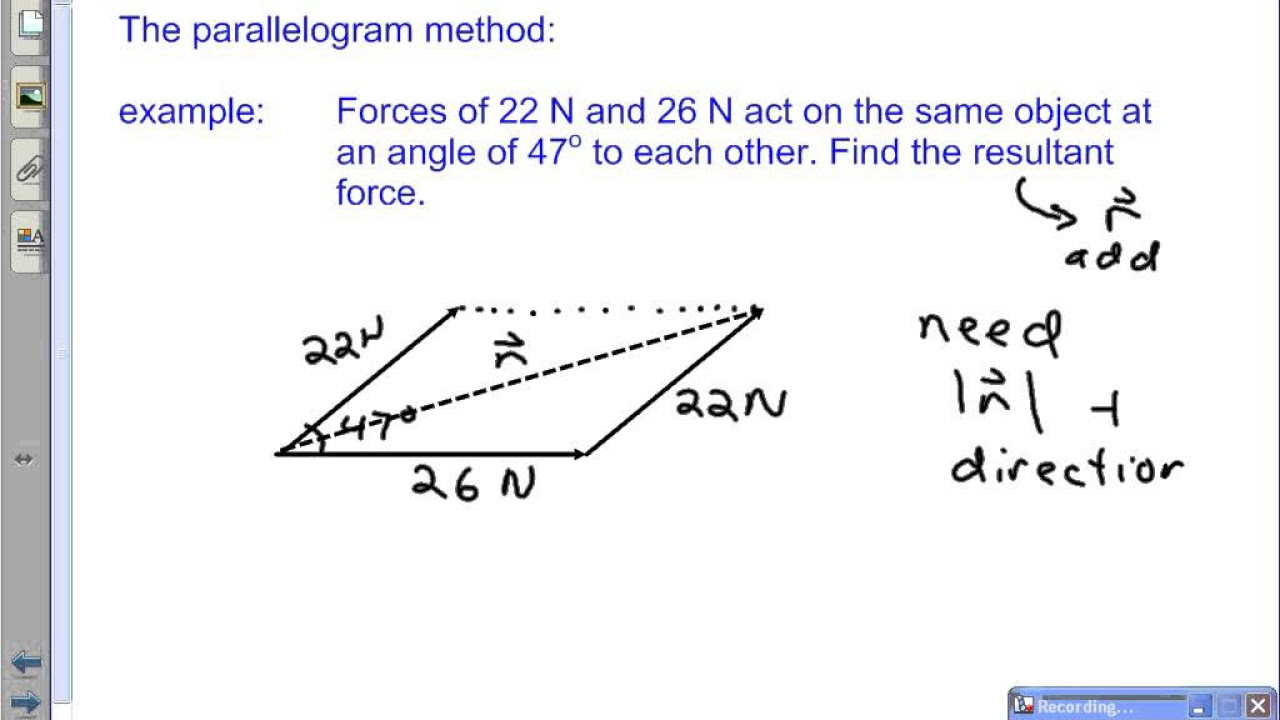
1-2 C Vector Addition - 2 (Parallelogram Method)

4 Resultant force of two vectors at different angles
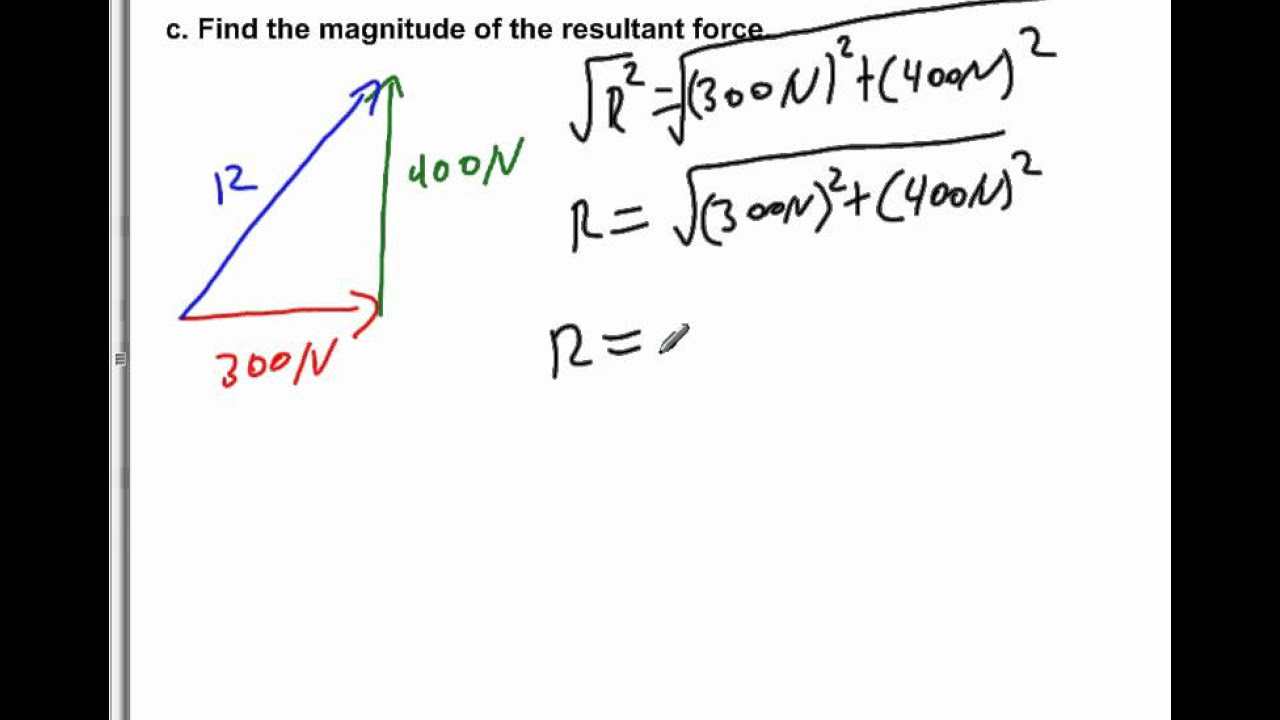
AP Physics B Kinematics Presentation General Problems #07
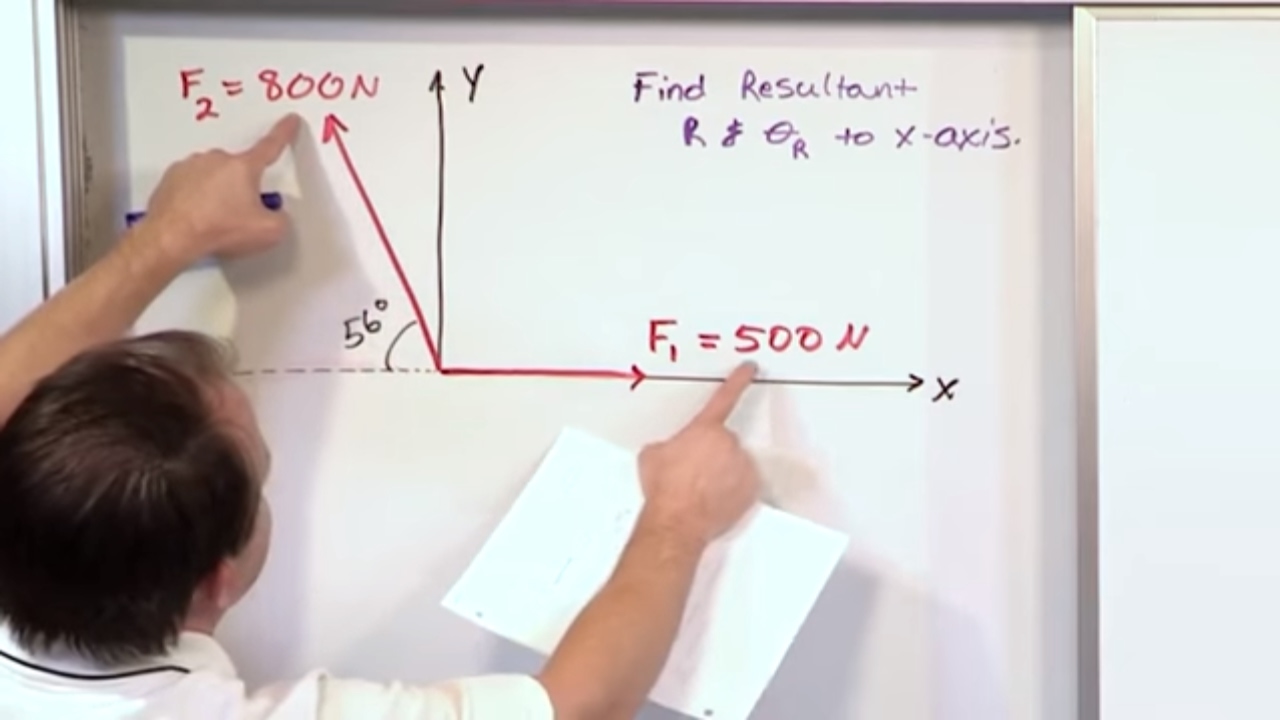
Lesson 5 - Finding The Resultant Of Two Forces, Part 1 (Engineering Mechanics Statics)
5.0 / 5 (0 votes)
Thanks for rating: