Derivative of log_x (for any positive base a­1) | AP Calculus AB | Khan Academy

Khan Academy
22 Jul 201604:47
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explains the concept of finding the derivative of a logarithm with an arbitrary base, using the natural logarithm as a foundation. It introduces the change of base formula, demonstrating its utility in converting logarithms between different bases, and shows how to apply this to the derivative of log base A of X, which is expressed as 1/(natural log of A) * 1/X. The explanation is clear and provides examples to help users understand the process, making it an engaging and informative resource for those interested in calculus and logarithms.

Takeaways
  • 📚 The derivative of the natural log of X (ln(X)) with respect to X is 1/X.
  • 🔄 The concept of changing the base of a logarithm is introduced, using the formula log base A of B = log base C of B / log base C of A.
  • 📱 Calculators typically have a 'log' button for base 10 logarithms and a 'natural log' button for base e logarithms.
  • 🌐 To find logarithms of other bases, one can utilize the change of base formula and properties of natural logs.
  • 🤔 The derivative of a logarithm with a variable base can be found by rewriting the log as a natural log and applying the chain rule.
  • 📈 The derivative of log base A of X (log_A(X)) is given by (1 / (ln(A) * X))
  • 🌟 The natural log function's derivative (1/X) is fundamental in deriving the derivative of logarithms with arbitrary bases.
  • 📌 Example given: If F(X) = log base 7 of X, then F'(X) = 1 / (ln(7) * X).
  • ⏪ For a function with a constant coefficient, like G(X) = -3 * log base pi of X, the constant multiplies the derivative of the logarithm.
  • 🔢 The derivative of G(X) = -3 * log base pi of X is -3 / (ln(pi) * X).
  • 💡 This approach to derivatives of logarithmic functions provides a powerful tool for analyzing various mathematical and real-world problems.
Q & A

  • What is the derivative of the natural log of X with respect to X?

    -The derivative of the natural log of X (ln(X)) with respect to X is 1/X.

  • How can you express the logarithm of an arbitrary base in terms of natural logarithms?

    -You can express the logarithm of an arbitrary base (log base A of X) as the natural logarithm of X divided by the natural logarithm of A (ln(X) / ln(A)).

  • What is the change of base formula for logarithms?

    -The change of base formula states that log base A of B can be rewritten as log base C of B divided by log base C of A.

  • How does the change of base formula apply to a calculator that uses base 10 logarithms by default?

    -To find a logarithm in a different base using a calculator that uses base 10 (log) by default, you can input the log of the number you want to find the logarithm of, divided by the log of the base (e.g., log(8) / log(3) for log base 3 of 8).

  • What is the derivative of log base A of X with respect to X?

    -The derivative of log base A of X with respect to X is (1 / (ln(A))) * (1 / X), where ln(A) is a constant and ln(X) is the natural logarithm of X.

  • How can you find the derivative of a logarithmic function with a different base if the base is not the natural logarithm base (e)?

    -You can find the derivative by using the chain rule and the change of base formula, rewriting the logarithmic function in terms of natural logarithms and then differentiating with respect to X.

  • What is the relationship between the natural logarithm and the common logarithm?

    -The natural logarithm (ln) is the logarithm with base e, while the common logarithm (log) has base 10. The natural logarithm of X is equal to the common logarithm of X multiplied by ln(10).

  • What is the derivative of a constant multiplied by a logarithmic function?

    -The derivative of a constant (c) multiplied by a logarithmic function (log base A of X) is the constant divided by the logarithmic base (ln(A)) times the derivative of the argument of the logarithm (1/X).

  • How does the derivative of a logarithmic function change if there is a negative sign in front of the logarithm?

    -If there is a negative sign in front of the logarithm, the derivative will also have a negative sign. The derivative will be -(1 / (ln(A))) * (1 / X).

  • What is the significance of the derivative of a logarithmic function in calculus?

    -The derivative of a logarithmic function is significant in calculus as it allows us to analyze the rate of change of the function, which is crucial for optimization problems, understanding the behavior of functions, and solving related differential equations.

  • Can you use the derivative of a logarithmic function to find the critical points of a function?

    -Yes, the derivative of a logarithmic function can be used to find the critical points of a function by setting the derivative equal to zero and solving for X, which will indicate potential maximum or minimum points of the original function.

Outlines
00:00
📚 Understanding Derivatives of Logarithmic Functions

This paragraph introduces the concept of finding the derivative with respect to X of a logarithm with an arbitrary base. It begins by referencing previous knowledge of the derivative of the natural log of X, which is 1/X. The voiceover explains the goal of the video is to extend this understanding to logarithms with different bases, denoted as log base A of X. The key concept introduced is the change of base formula, which allows the conversion of logarithms from one base to another. This is demonstrated using the formula log base C of B equals log base C of B divided by log base C of A. The paragraph emphasizes the practical application of this formula, especially when using a calculator that typically has buttons for log base 10 (log) and log base E (natural log). The video aims to show how to find derivatives of logarithmic functions with various bases by leveraging the known derivative of the natural log.

Mindmap
Keywords
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the context of the video, it refers to the derivative with respect to X of various logarithmic functions, which is the focus of the explanation provided. For example, the derivative of the natural log of X is shown to be 1/X, which is a foundational result used to derive the derivatives of logarithms with arbitrary bases.
💡natural log
The natural log, often denoted as ln(X), is a logarithm to the base 'e', where 'e' is a mathematical constant approximately equal to 2.71828. It is a crucial concept in the video as the natural log is used to derive the derivatives of logarithms with other bases. The natural log is also the basis for the change of base formula discussed in the video.
💡logarithm
A logarithm is the inverse operation to exponentiation, and it is used to find the power to which a base must be raised to produce a given value. In the video, the focus is on logarithms with arbitrary bases, denoted as 'log base A of X'. The logarithm is a key concept as it is the main function whose derivative is being explored.
💡change of base
The change of base formula is a method for converting a logarithm from one base to another. It states that 'log base A of B' is equal to 'log base C of B' divided by 'log base C of A'. This formula is essential in the video as it allows the conversion of logarithms to the natural log, which has a known derivative, facilitating the calculation of derivatives for other bases.
💡algebra
Algebra is a branch of mathematics that uses symbols and rules to represent and solve equations. In the context of the video, algebra is mentioned as a field where the change of base formula is likely familiar to viewers, indicating its utility in manipulating and solving equations involving logarithms.
💡pre calculus
Pre calculus is a course of study that prepares students for calculus by introducing them to concepts such as functions, limits, and continuity, which are foundational for more advanced calculus topics. In the video, pre calculus is mentioned as a context in which students may have encountered the change of base formula for logarithms, indicating its importance in the progression of mathematical understanding.
💡calculator
A calculator is an electronic device used to perform mathematical calculations, including those involving logarithms. In the video, the use of a calculator is discussed to illustrate how logarithms with different bases can be computed, such as log base 10 (common log) and natural log (log base 'e'), which are typically available on most calculators.
💡constant
In mathematics, a constant is a value that does not change. In the context of the video, constants are used to simplify the expression for the derivative of a logarithmic function. For instance, the natural log of a base 'A' is a constant because it represents a fixed number, which is then used to calculate the derivative of the logarithm function.
💡rate of change
The rate of change, in the context of derivatives, refers to the speed at which a function's output changes as its input varies. It is a central idea in the video as it explains how the derivative of a logarithmic function can be interpreted as the rate at which the value of the logarithm changes with respect to the variable X.
💡function
In mathematics, a function is a relation that assigns a single output value to each input value. Functions are the primary objects of study in calculus, and in this video, the functions of interest are logarithmic functions with arbitrary bases. The properties and derivatives of these functions are the focus of the video's content.
💡exponential growth
Exponential growth refers to a process where a quantity increases at a rate proportional to its current value. While not explicitly mentioned in the video, the concept of exponential growth is related to logarithms because logarithmic and exponential functions are inverse operations. Understanding logarithms helps in analyzing situations that involve exponential growth or decay.
Highlights

The derivative with respect to X of the natural log of X is equal to 1 over X.

The goal is to find the derivative of a logarithm with an arbitrary base.

A change of base formula is introduced, which is key to understanding logarithms with different bases.

The change of base formula is log base C of B equals log base C of B divided by log base C of A.

Calculators have a log button for base 10 logarithms and a natural log button for base e logarithms.

To find logarithms with other bases, the change of base formula can be utilized.

The derivative of log base A of X can be rewritten using the natural log of X over the natural log of A.

The derivative of log base A of X simplifies to 1 over the natural log of A times the derivative of the natural log of X.

The derivative of the natural log of X is known to be 1 over X.

The derivative of log base A of X is thus 1 over the natural log of A times 1 over X.

The formula can be further simplified to 1 over the natural log of A times X.

The process demonstrated can be applied to find derivatives of logarithms with various bases.

An example is given where F of X is log base 7 of X, and its derivative is found using the derived formula.

For a function like G of X which is negative 3 times log base pi of X, the derivative is calculated by applying the formula.

The video provides a comprehensive understanding of logarithmic derivatives and how to calculate them.

The use of the natural log makes the process of finding derivatives of logarithms with arbitrary bases more accessible.

The video is a valuable resource for those looking to understand the mathematical principles behind logarithms and their derivatives.

Transcripts

Browse More Related Video

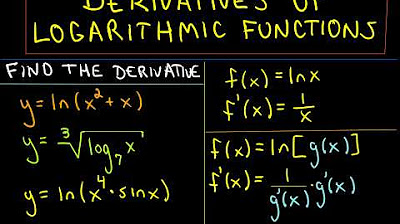
Derivatives of Logarithmic Functions - More Examples

How to Change the Base of a Logarithm (Precalculus - College Algebra 61)

Math1325 Lecture 11 1 Derivatives of Logarithm

Deriving Natural Logarithms!

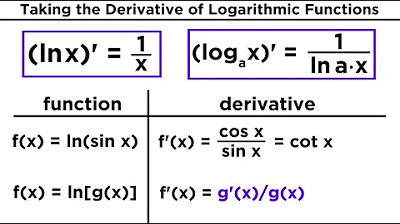
Derivatives of Logarithmic and Exponential Functions

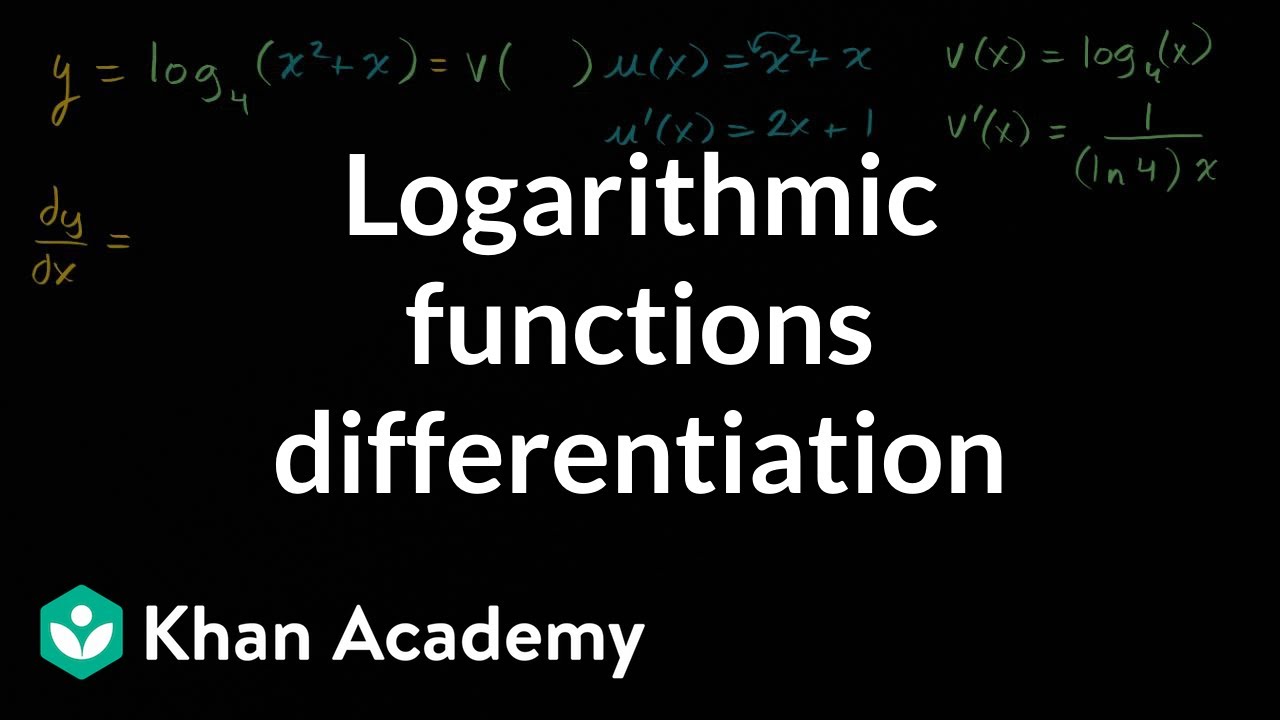
Logarithmic functions differentiation | Advanced derivatives | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Logarithmic DerivativesChange of BaseNatural LogsCalculus ConceptsMathematical TutorialAlgebraic TechniquesPre-CalculusLogarithm PropertiesEducational ContentKhan Academy