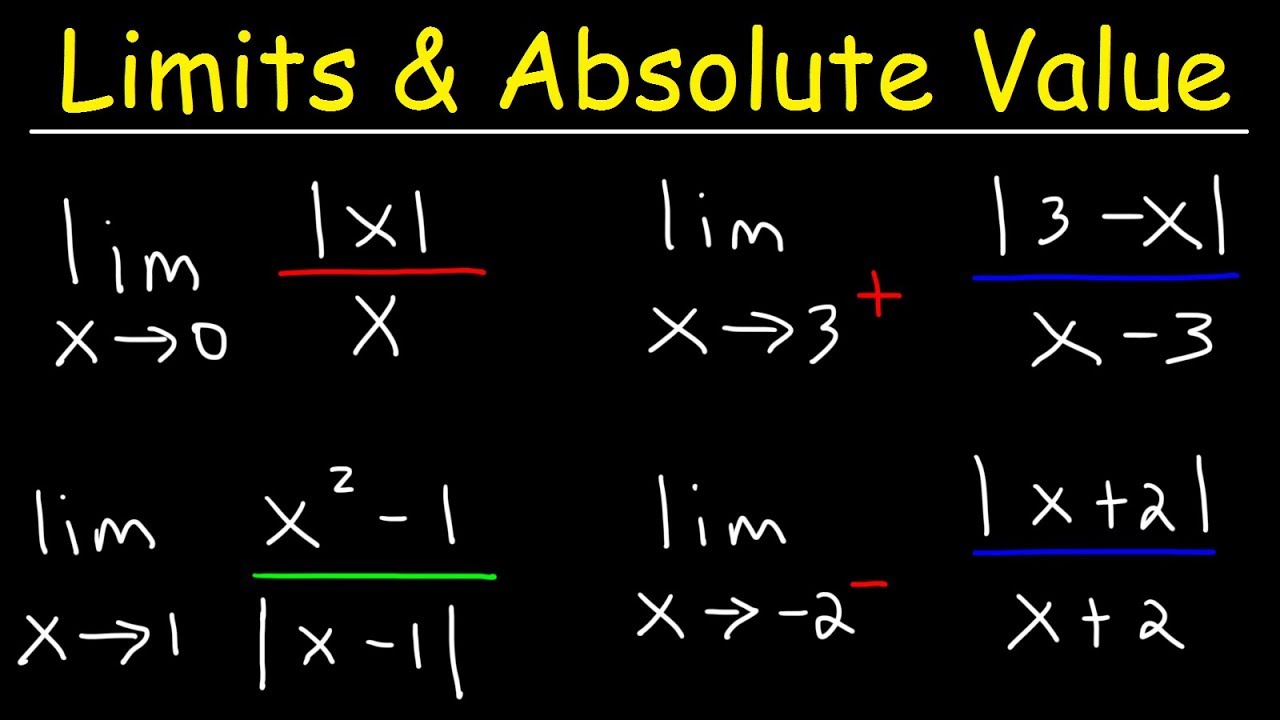Limits of piecewise functions | Limits and continuity | AP Calculus AB | Khan Academy
TLDRThe video script discusses the concept of limits for piecewise functions, using algebraic definitions to explore one-sided and two-sided limits. It illustrates the process with examples, showing how to calculate limits as x approaches specific values for different piecewise functions. The importance of approaching from both the left and right to determine two-sided limits is emphasized, and the continuity of functions at certain points is also discussed, providing a clear understanding of the mathematical principles involved.
Takeaways
- 📚 The concept of limits for piecewise functions is discussed, emphasizing the importance of one-sided and two-sided limits.
- 🔢 For the function f(x) as x approaches 4 from the right, the limit is 2, based on the definition of the function for x > 4.
- 🔄 When approaching 4 from the left, the function f(x) yields a limit of 2, calculated from the expression 4 + 2 / (4 - 1).
- 🎯 The limit of f(x) as x approaches 4 from both sides is 2, indicating that the function is continuous at x = 4.
- 📈 At x = 2, despite a potential discontinuity at x = 1 (denominator becomes zero), the function is continuous, and the limit is 4.
- 🌟 Another piecewise function example is introduced, with a focus on the limit as x approaches -1 from both sides.
- 👉 For the second function, the one-sided limit from the right as x approaches -1 is 1/2, calculated from 2^(-1).
- 🌌 Conversely, from the left as x approaches -1, the limit is 0, derived from the sine function at x = -1 + 1, which is sin(0).
- 🔄 The two-sided limit as x approaches -1 does not exist for the second function, as the one-sided limits from the left and right are not equal.
- 🎯 Lastly, the limit of the second function as x approaches 0 from the right is 1, found by substituting x = 0 into the function, resulting in 2^0.
Q & A
What is the one-sided limit of f(x) as x approaches 4 from the right?
-The one-sided limit of f(x) as x approaches 4 from the right is 2, since when x is greater than 4, f(x) is equal to the square root of x, and the square root of 4 is 2.
What is the one-sided limit of f(x) as x approaches 4 from the left?
-The one-sided limit of f(x) as x approaches 4 from the left is 2, which is calculated by substituting x with 4 in the expression 4 + 2 / (4 - 1), resulting in 6 / 3, which simplifies to 2.
What is the two-sided limit of f(x) as x approaches 4?
-The two-sided limit of f(x) as x approaches 4 exists and is equal to 2, since the one-sided limits from both the left and the right are equal.
What is the limit of f(x) as x approaches 2?
-The limit of f(x) as x approaches 2 is 4, since at x equals 2, the function is continuous and we can substitute the value directly to get (2 + 2) / (2 - 1), which simplifies to 4 / 1, equal to 4.
What is the one-sided limit of g(x) as x approaches -1 from the right?
-The one-sided limit of g(x) as x approaches -1 from the right is 1/2, because when approaching from the right (x greater than or equal to -1), g(x) approaches 2 to the power of -1, which is 1/2.
What is the one-sided limit of g(x) as x approaches -1 from the left?
-The one-sided limit of g(x) as x approaches -1 from the left is 0, since in this case, g(x) is equal to the sine of (-1 + 1), which is the sine of 0, resulting in 0.
Does the two-sided limit of g(x) as x approaches -1 exist?
-No, the two-sided limit of g(x) as x approaches -1 does not exist because the one-sided limits from the left and the right are not equal (0 from the left and 1/2 from the right).
What is the limit of g(x) as x approaches 0 from the right?
-The limit of g(x) as x approaches 0 from the right is 1, since in this interval the function is continuous and substituting x with 0 gives us 2 to the power of 0, which is 1.
How can we determine if a two-sided limit exists?
-A two-sided limit exists if and only if the one-sided limits from the left and the right both exist and are equal. If they are not equal or if one of them does not exist, then the two-sided limit does not exist.
What is the significance of a piecewise function being continuous at a certain point?
-If a piecewise function is continuous at a certain point, it means that the function can be evaluated at that point without any discontinuities or breaks. This allows us to directly substitute the value of x into the function to find the limit at that point.
How does the behavior of a function near a certain point affect its limit?
-The behavior of a function near a certain point greatly affects its limit. If the function approaches the same value from both the left and the right, then the limit exists. However, if the function approaches different values or has discontinuities, the limit may not exist or could be undefined.
Outlines
📚 Analysis of Piecewise Function Limits
This paragraph introduces the concept of limits for piecewise functions, focusing on the algebraic definition of functions. The instructor prompts viewers to consider various limits, including one-sided and two-sided limits. A specific example is given where the function f(x) is defined for x greater than 4 as the square root of x. The limit as x approaches 4 from the right is found to be 2, as is the limit from the left, confirming that the two-sided limit exists and is equal to 2. The discussion continues with the limit of f(x) as x approaches 2, noting the continuity at x=2 despite a denominator becoming zero at x=1, and the limit is found to be 4. Another piecewise function example is introduced for further analysis.
Mindmap
Keywords
💡Piecewise Functions
💡Limits
💡One-Sided Limits
💡Two-Sided Limits
💡Continuous
💡Denominator
💡Square Root
💡Sine Function
💡Substitute
💡Exponential Functions
💡Breakpoints
Highlights
Introduction to limits of piecewise functions
One-sided limit as x approaches 4 from the right, using the algebraic definition of f(x)
The limit as x approaches 4 from the right is equal to 2, based on the square root function
One-sided limit as x approaches 4 from the left, using the algebraic definition of f(x)
The limit as x approaches 4 from the left is 6/3 or 2, using the linear function definition
Two-sided limit as x approaches 4, which is equal to 2, demonstrating the concept of one-valued limits
Limit as x approaches 2 for f(x), considering the continuity at x equals 2
The limit at x equals 2 is 4, using the algebraic definition of f(x) and substitution
Introduction to another piecewise function and its limit analysis
One-sided limit as x approaches -1 from the right, using the algebraic definition of g(x)
The limit as x approaches -1 from the right is 1/2, based on the exponent function
One-sided limit as x approaches -1 from the left, using the sine function in the piecewise definition
The limit as x approaches -1 from the left is 0, using the sine of zero
Two-sided limit as x approaches -1 does not exist, due to differing one-sided limits
Limit as x approaches 0 from the right, considering the continuity and substitution in the function definition
The limit as x approaches 0 from the right is 1, using the exponent function with base 2
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: