Work Done By Gravity and Gravitational Potential Energy - Physics
TLDRThe video script discusses the concept of gravitational potential energy (GPE) and how it is calculated. It begins with a straightforward example of a 5 kg book at a height of 10 m above the ground, using the formula GPE = mgh to find the energy. The script then explores changes in GPE as the book's height changes to 25 m. It delves into the work done by gravity, explaining that work is negative when the force and displacement are in opposite directions. The script also addresses the limitations of the formula when dealing with objects at great heights, such as satellites, and introduces the formula involving the universal gravitational constant and the masses of the Earth and the object, as well as the distance between them. The key takeaway is understanding when to apply each formula based on the proximity of the object to the Earth's surface.
Takeaways
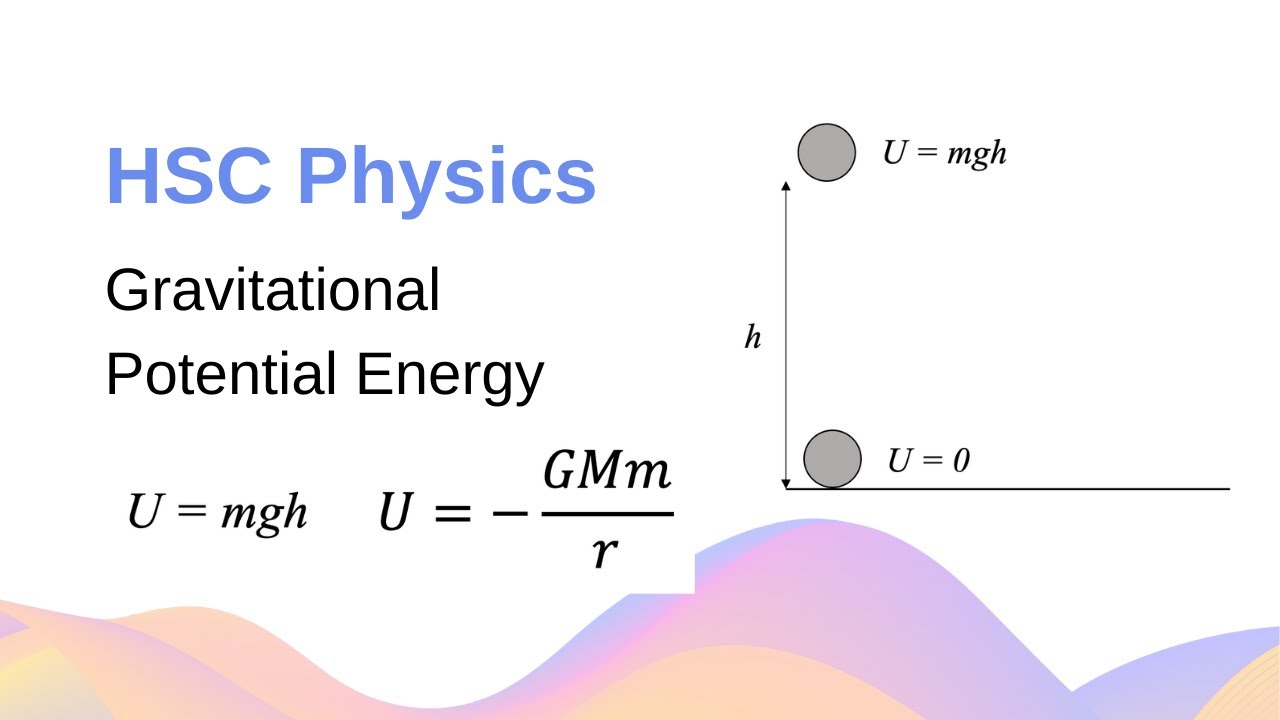
- 📚 The gravitational potential energy (GPE) of an object is calculated using the formula mgh or MgY, where m is mass, g is gravitational acceleration, and h (or Y) is height above the ground.
- 📈 For an object (like a 5 kg book) 10 m above the ground, the GPE is 490 Joules (using Earth's standard g of 9.8 m/s²).
- 🔄 When the height changes, as in moving from 10 m to 25 m, the new GPE is calculated with the updated height value, resulting in 1225 Joules for the book.
- 🚀 The work done by gravity when moving an object against the gravitational force (upward) is negative work, which is equal to the negative change in GPE.
- 🔄 The work done by gravity in moving the book from 10 m to 25 m is -735 Joules, calculated as the difference between the final and initial GPE.
- 🌍 For objects close to Earth, where the height (h) is small compared to Earth's radius (R), the simplified GPE formula U = mgh or mgy can be used.
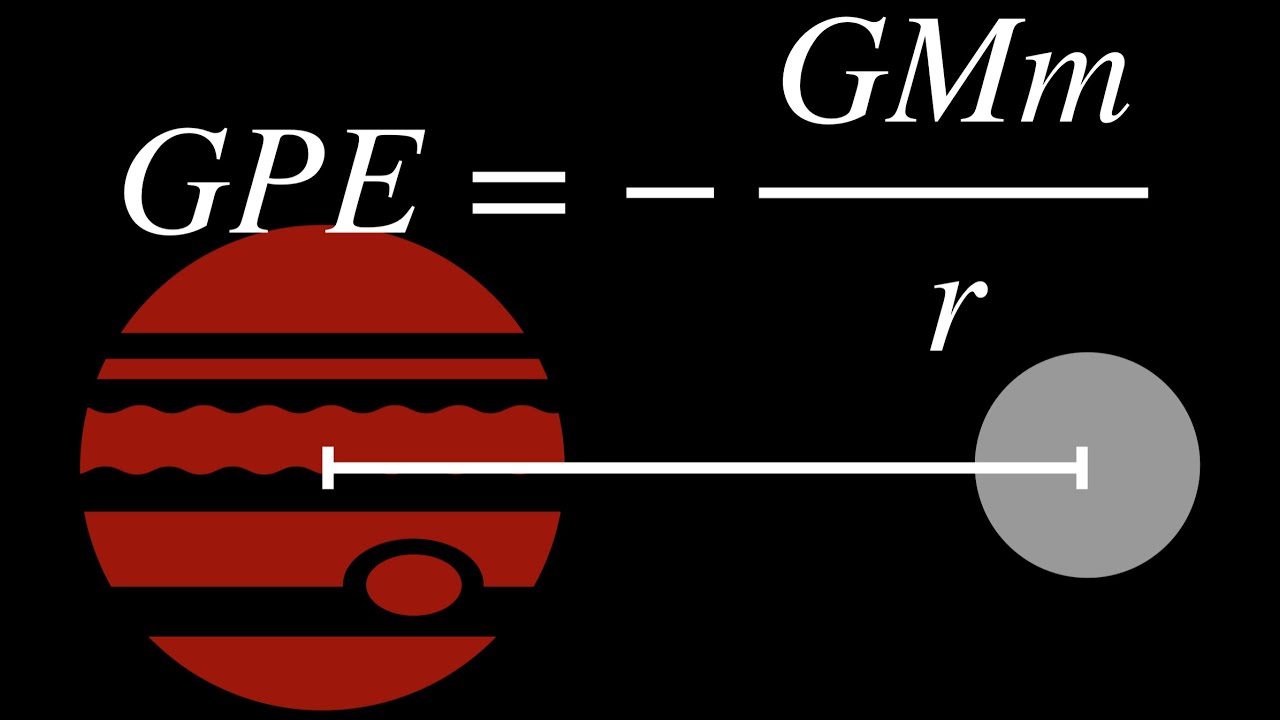
- 🌌 For objects far from Earth, such as satellites, the gravitational acceleration (g) changes and must be calculated using the formula g = GM/r², where G is the gravitational constant, M is Earth's mass, and r is the distance from the center of Earth.
- 📈 The GPE for a satellite 9000 km above Earth's surface is calculated using the formula U = Gmem/R, resulting in a value of 2.07 * 10^11 Joules.
- 🔑 It's crucial to know when to use which formula for GPE; the simplified one for objects close to Earth and the more complex one for those far away.
- 🌐 Gravitational acceleration (g) varies with distance from Earth, being approximately 9.8 m/s² at the surface and decreasing as one moves further away.
- 🔄 The concept of negative work is important when considering the work done against gravity, as it indicates the energy input required to move an object to a higher position.
Q & A
What is the gravitational potential energy of a 5 kg book placed 10 m above the ground?
-The gravitational potential energy of the book is 490 Joules, calculated using the formula mgh, where m is the mass (5 kg), g is the gravitational acceleration (9.8 m/s^2), and h is the height (10 m).
How does the height of an object affect the calculation of its gravitational potential energy?
-The height of an object directly affects the gravitational potential energy calculation through the formula mgh. As the height (h) increases, the potential energy also increases, assuming the mass (m) and gravitational acceleration (g) remain constant.
What is the work done by gravity when a 5 kg book is moved from 10 m to 25 m above the ground?
-The work done by gravity is -735 Joules. Since the book is moved against the force of gravity, the work done is negative. It is calculated by the change in potential energy, which is the final potential energy (1225 Joules at 25 m) minus the initial potential energy (490 Joules at 10 m).
What is the relationship between work done by a force and the direction of the force relative to displacement?
-If the force and displacement vectors are in the same direction, positive work is done on the object. If they are in opposite directions, negative work is done. In the case of moving the book against gravity, the work is negative because the force of gravity is downward while the displacement is upward.
Why can't the simple formula U = mgy be used for calculating the gravitational potential energy of a satellite far from Earth's surface?
-The simple formula U = mgy is not suitable for objects far from Earth's surface because gravitational acceleration (g) changes significantly with distance from Earth. At greater distances, g is much smaller than the value at or near the Earth's surface, so the correct formula involving the universal gravitational constant (G), Earth's mass, and the distance from the center of Earth must be used.
What is the correct formula for calculating the gravitational potential energy of an object when it is at a height comparable to the radius of the Earth?
-For objects at heights comparable to the Earth's radius, the formula U = Ghm/R is used, where G is the universal gravitational constant, h is the height of the object above the Earth's surface, m is the mass of the object, and R is the distance from the center of the Earth to the object (sum of the Earth's radius and the object's height).
How does the gravitational acceleration change with distance from the Earth's surface?
-Gravitational acceleration decreases as you move farther from the Earth's surface. Near the surface, it is approximately 9.8 m/s^2, but it becomes significantly smaller at great distances, such as 1.68 m/s^2 for an object 9,000 km above the Earth's surface.
What is the gravitational potential energy of an 8,000 kg satellite located 9,000 km above the Earth's surface?
-The gravitational potential energy of the satellite is 2.07 * 10^11 Joules, calculated using the formula U = Ghm/R, where G is the universal gravitational constant, m is the mass of the satellite (8,000 kg), and R is the total distance from the center of the Earth to the satellite (9,000 km + Earth's radius of 6,378 km).
What are the two scenarios in which the simple formula for gravitational potential energy U = mgy can be used?
-The simple formula U = mgy can be used when the height of the object is significantly less than the Earth's radius, typically for heights of 1,000 m or less, and even up to 2-3,000 m, as these are considered relatively small numbers in comparison to the Earth's radius.
How does the work done by gravity relate to the change in gravitational potential energy?
-The work done by gravity is equal to the negative change in gravitational potential energy. When an object is moved against the force of gravity (upward), the work done is negative, and this negative work results in an increase in the object's gravitational potential energy.
What is the significance of the negative sign in the work done by gravity?
-The negative sign in the work done by gravity indicates that the work is done against the direction of the gravitational force. It means that energy is being expended to move the object in the opposite direction of the gravitational pull, which results in an increase in the object's potential energy.
Outlines
📚 Gravitational Potential Energy Calculation
This paragraph explains the concept of gravitational potential energy (GPE) and demonstrates how to calculate it using the formula mgy, where m is mass, g is the acceleration due to gravity (9.8 m/s²), and y is the height above the ground. A practical example is given, calculating the GPE of a 5 kg book at 10 m height as 490 Joules. The paragraph further discusses the work done by gravity when the book is moved from 10 m to 25 m height, resulting in a negative work value of 735 Joules, indicating that work is done against the gravitational force.
🌍 Earth's Gravitational Potential Energy Formula
This section introduces an alternative formula for calculating gravitational potential energy (U = mgy) for objects at a height (y) significantly smaller than the Earth's radius. It explains that this simplified formula is applicable when y is much smaller than the Earth's radius (6.38 * 10^6 m). However, for objects far from the Earth's surface, such as satellites, this formula is inadequate due to the significant decrease in gravitational acceleration with distance. The gravitational acceleration at a satellite's location (9,000 km from Earth) is calculated to be 1.68 m/s², highlighting the difference from the surface value of 9.8 m/s².
🚀 Gravitational Potential Energy for Distant Objects
The paragraph discusses the correct formula for calculating the gravitational potential energy for objects that are very far from the Earth's surface. It introduces the formula U = (G * M * m) / r, where G is the gravitational constant, M is the mass of the Earth, m is the mass of the object, and r is the distance from the center of the Earth to the object. Using this formula, the GPE for an 8,000 kg satellite 9,000 km above the Earth's surface is calculated to be 2.07 * 10^11 Joules. The paragraph emphasizes the importance of using the correct formula based on the object's distance from the Earth.
Mindmap
Keywords
💡Gravitational Potential Energy
💡Work Done
💡Displacement
💡Gravitational Acceleration
💡Radius of Earth
💡Universal Gravitational Constant
💡Satellite
💡Force
💡Energy
💡Height
💡Formula
Highlights
Gravitational potential energy calculation for a 5 kg book 10 m above the ground using the formula mgy.
The gravitational potential energy of the book at 10 m height is 490 Joules.
For Part B, the book is at a new height of 25 m above the ground, and the gravitational potential energy is recalculated.
The gravitational potential energy at 25 m is 1225 Joules.
In Part C, the work done by gravity as the book moves from 10 m to 25 m height is determined by the change in potential energy.
The work done by gravity is negative since the book is moved against the force of gravity.
The formula for work done by gravity is mg(Y2 - Y1), where Y1 and Y2 are the initial and final heights.
The work done in moving the book from 10 m to 25 m is 735 Joules.
A different formula for gravitational potential energy is introduced for objects close to the Earth's surface, U = mgY.
For objects far from the Earth's surface, the gravitational acceleration (g) changes and a different formula is required, U = G * (m1 * m2) / r.
The gravitational acceleration (g) decreases significantly as distance from the Earth increases.
A satellite 9000 km from the Earth's surface has a different gravitational acceleration, approximately 1.68 m/s^2.
The gravitational potential energy for a satellite far from the Earth is calculated using the formula U = G * (m1 * m2) / r, resulting in 2.07 * 10^11 Joules.
Two formulas are presented for calculating gravitational potential energy depending on the distance from the Earth: one for close objects and another for distant objects.
The concept of positive and negative work is explained in the context of force and displacement vectors' directions.
The practical application of these concepts is demonstrated through the example of a book being lifted and a satellite in orbit.
The importance of understanding when to use each formula for gravitational potential energy is emphasized.
Transcripts
Browse More Related Video

Gravitational Potential Energy Calculations (GPE) - Mass x Gravity x Height OR GPE=mgh

13.3a Gravitational Potential Theory | A2 G-Fields | Cambridge A Level Physics
Gravitational Potential Energy & Work Done + Calculation Example // HSC Physics
Deriving The Formula For Gravitational Potential Energy

Potential energy and gravity

Calculating gravitational potential energy | Modeling energy | High school physics | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: