Learn Topology in 5 minutes (joke video)

eigenchris
1 Apr 202005:02
EducationalLearning
32 Likes 10 Comments

TLDRThis video introduces the viewer to the fascinating world of topology, a branch of mathematics concerned with the properties of topological spaces preserved under homeomorphisms. The presenter explains key concepts such as open and closed sets, continuity, and compactness, highlighting their importance in understanding more advanced topics like functors and natural transformations. The Heine-Borel theorem and the Poincare Conjecture are also briefly mentioned, emphasizing the practical applications of topology in various mathematical contexts.

Takeaways
  • 📚 Topology is the study of topological spaces and their properties under homeomorphisms.
  • 🎓 The presenter offers a companion textbook for further study in topology.
  • 🖼️ Topology is often illustrated with interesting shapes like knots and Mobius strips, but these are not used in the course.
  • 🔄 Topological spaces are transformed using continuous maps, which are foundational for understanding functors and natural transformations.
  • 📌 Definition 1.1: A topology T on a set X is a family of sets that includes the empty set and X, and satisfies the conditions of unions and intersections.
  • 📊 Theorem 1.2: A subset Q of real numbers is open in the Euclidean topology if it is a union of open intervals.
  • 🚫 Definition 1.4: A subset P of X is closed if its complement (X - P) is open in the topology T.
  • 🔍 Theorem 1.5: The closure of a set P is the union of P and all its limit points, where a limit point is a point whose every neighborhood contains a point from P.
  • 📐 Theorem 1.6: The closure of a set is closed, which is proven by considering open sets and points not in the closure.
  • 🌐 Definition 1.7: A topological space is compact if every open cover has a finite subcover.
  • 📈 Theorem 1.8 (Heine Borel theorem): Every closed bounded subset of RN is compact.
  • 🌍 Theorem 1.9 (Poincaré conjecture): Every simply connected closed three-manifold is homeomorphic to the 3-sphere, with the proof left as an exercise.
Q & A

  • What is topology?

    -Topology is the study of topological spaces and their properties preserved under homeomorphisms.

  • What are some examples of topological figures shown in the video?

    -Examples include a knot, a Möbius strip, and a coffee mug turning into a donut.

  • Why is the study of topology important?

    -Topology has practical applications, such as understanding continuous maps, functors, natural transformations, and adjoint functors.

  • What is a topology T on a set X according to definition 1.1?

    -A topology T on X is a family of sets that includes the empty set and X, where the possibly infinite union of any sets in T is in T, and the finite intersection of any sets in T is in T.

  • How is a subset Q of the real numbers defined as open in the Euclidean topology according to theorem 1.2?

    -A subset Q is open in the Euclidean topology if and only if it is a union of open intervals.

  • What does it mean for a subset P of X to be closed according to definition 1.4?

    -A subset P of X is closed if its complement (X minus P), also written as P^C, is open in the topology T.

  • What is the definition of the closure of a set P according to theorem 1.5?

    -The closure of a set P, denoted by P-bar, is the union of P and all its limit points, where a limit point of a set J is a point Y such that every neighborhood of Y contains a point in J different from Y itself.

  • Why is the closure of a set considered closed according to theorem 1.6?

    -The closure of a set is closed because it can be expressed as the union of the set and its limit points, and the union of open sets is an open set.

  • What does compactness mean in topology as defined in definition 1.7?

    -A topological space X is compact if every open cover of X has a finite subcover.

  • What is the Heine Borel theorem as stated in theorem 1.8?

    -The Heine Borel theorem states that every closed bounded subset of RN (n-dimensional Euclidean space) is compact.

  • What is the Poincare Conjecture mentioned in theorem 1.9?

    -The Poincare Conjecture states that every simply connected closed three-manifold is homeomorphic to the 3-sphere.

Outlines
00:00
📚 Introduction to Topology

This paragraph introduces the topic of topology, a branch of mathematics that studies topological spaces and their properties under homeomorphisms. The speaker offers a brief, five-minute course and mentions a companion textbook for further learning. It also highlights the practical applications of topology in understanding continuous maps, functors, natural transformations, and adjoint functors. The speaker emphasizes the importance of studying topology and sets the stage for the course by discussing the motivation behind it.

📐 Basic Concepts and Definitions

The paragraph delves into the foundational concepts of topology, including the definition of a topology T on a set X, which must satisfy certain conditions like the inclusion of the empty set and the union of any sets in T. It introduces the notion of open and closed sets, and the concept of closure as the union of a set and its limit points. The speaker also presents theorems and definitions related to open covers, compactness, and the Heine-Borel theorem, which are fundamental to understanding the Euclidean topology and the properties of real numbers in it.

🌐 The Poincare Conjecture and Reflections on Topology

In this final paragraph, the speaker discusses the Poincare Conjecture, which is a significant problem in topology stating that every simply connected closed three-manifold is homeomorphic to the 3-sphere. The proof of this conjecture is left as an exercise for the viewer. The speaker then humorously addresses the value of studying topology, questioning its social relevance and suggesting that it is a question students may ponder throughout their academic journey, especially in graduate school.

Mindmap
Keywords
💡Topology
Topology is a branch of mathematics that studies the properties of spaces that are preserved under continuous deformations, such as stretching, twisting, and bending, but not tearing or gluing. In the context of the video, topology focuses on how topological spaces and their properties are maintained under homeomorphisms, which are continuous mappings with continuous inverses.
💡Homeomorphisms
Homeomorphisms are continuous functions between topological spaces that have continuous inverses. They are essential in topology because they preserve the topological properties of spaces. Two spaces that can be continuously deformed into each other are considered homeomorphic, and thus they share the same topological properties.
💡Continuous Maps
Continuous maps, or continuous functions, are mathematical functions where small changes in the input result in small changes in the output. In the context of topological spaces, these maps help define the structure and properties of the space, and are crucial for understanding concepts like homeomorphisms and topological spaces.
💡Euclidean Topology
Euclidean topology is the topology derived from the Euclidean space, where the basic open sets are intervals in the number line, plane, or space. It is the simplest and most familiar kind of topology, used to define concepts like open and closed sets, as well as continuity in the context of real numbers.
💡Open Sets
In topology, open sets are sets that do not contain their boundary points. They are fundamental to defining topological spaces and are used to construct the topology on a set. Open sets are important in understanding the structure of the space and are part of the definition of a topological space.
💡Closed Sets
Closed sets in topology are those whose complement in the topological space is an open set. They are the opposite of open sets and include their boundary points. Closed sets are crucial in defining the structure of topological spaces and are part of the basic vocabulary of topology.
💡Closure
The closure of a set in topology is the smallest closed set that contains the original set and all its limit points. A limit point of a set is a point where every neighborhood of that point contains at least one point from the set other than the point itself. Closure is an important concept in understanding the behavior of sets and their boundaries in topological spaces.
💡Compactness
Compactness is a topological property of a space where every open cover of the space has a finite subcover. This means that the space can be covered by a finite number of smaller open sets from any collection of open sets that cover it. Compactness is a central concept in topology and has significant implications for the behavior of continuous functions on the space.
💡Heine-Borel Theorem
The Heine-Borel theorem is a fundamental result in real analysis and topology. It states that in Euclidean space, a subset is compact if and only if it is closed and bounded. This theorem connects the concepts of compactness, closure, and boundedness, and is a key result in understanding the structure of Euclidean spaces.
💡Poincare Conjecture
The Poincare Conjecture is a famous problem in the field of topology that was proposed by Henri Poincare. It states that every simply connected, closed three-dimensional manifold is homeomorphic to a three-dimensional sphere (the 3-sphere). This conjecture has implications for the understanding of the global structure of three-dimensional spaces.
💡Category Theory
Category theory is a branch of mathematics that deals with abstract structures and their relationships. It introduces concepts like functors, natural transformations, and adjoint functors, which are used to describe mappings and structures between different mathematical categories. In the context of the video, category theory is related to the study of topological spaces and continuous maps.
Highlights

The video introduces a five-minute course on topology, a branch of mathematics.

Topology is the study of topological spaces and their properties under homeomorphisms.

The course mentions a companion textbook for further learning.

Topology is related to practical applications through the study of continuous maps, functors, natural transformations, and adjoint functors.

A topology T on a set X is defined by three properties: the empty set and X are in T, the possibly infinite union of sets in T is in T, and the finite intersection of sets in T is in T.

A subset Q of the real numbers is open in the Euclidean topology if it is a union of open intervals.

A subset P of a set X with topology T is closed if its complement is open in T.

The closure of a set P is the union of P and all its limit points.

The closure of a set is closed, as proven in the video.

Compactness is a very important concept in topology, defined as every open cover of a topological space having a finite subcover.

The Heine-Borel theorem states that every closed bounded subset of RN is compact.

The Poincare conjecture is mentioned, stating that every simply connected closed three-manifold is homeomorphic to the 3-sphere.

The proof of the Poincare conjecture is left as an exercise for the viewer.

The video ends with a reflection on the value and application of studying topology, posing a question about its social value and contribution to society.

The course is described as an online course, and viewers are congratulated for 'finishing' it.

The video challenges students in topology classes to consider why they are paying for an education in a field that may seem to have no direct social value.

Transcripts

Browse More Related Video

What is Topology?

Topological spaces - construction and purpose - Lec 04 - Frederic Schuller

Classification of sets - Lec 03 - Frederic Schuller

The second most beautiful equation and its surprising applications

Who cares about topology? (Inscribed rectangle problem)

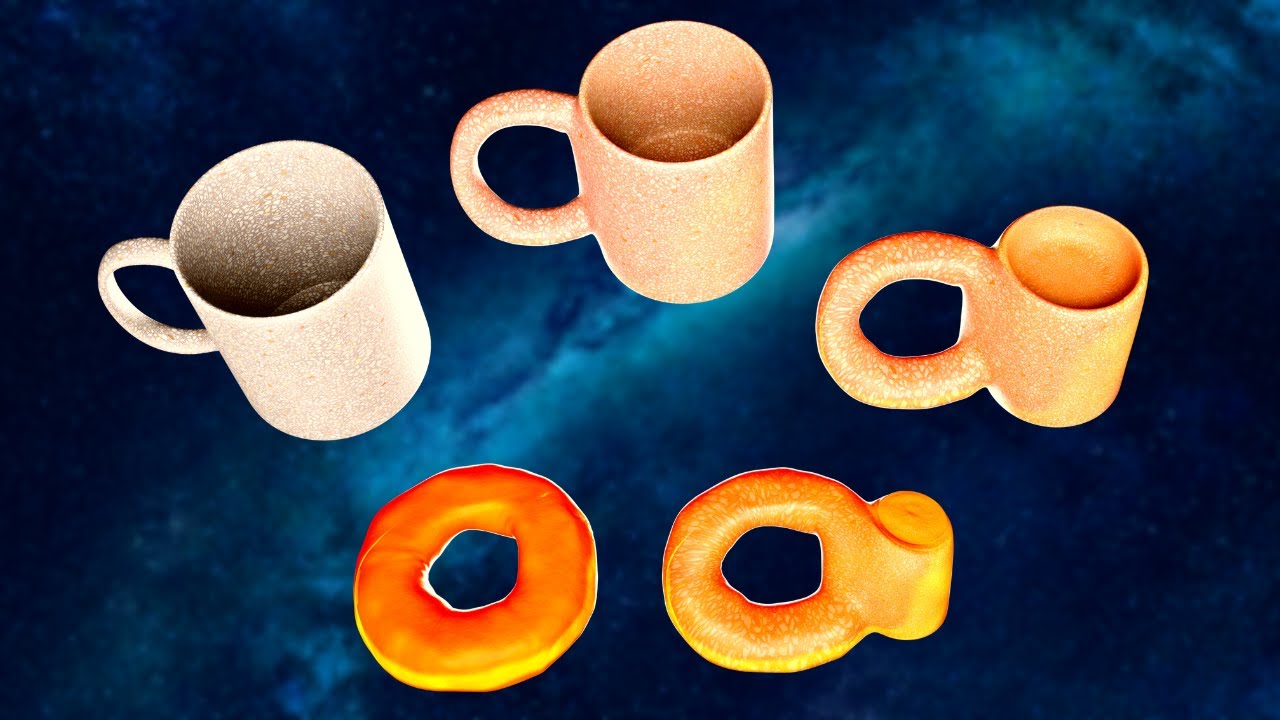
Intro to Topology - Turning a Mug Into a Doughnut

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Topology101MathematicsHomeomorphismsEuclideanTopologyOpenClosedSetsCompactnessHeineBorel3DManifoldsPoincareConjectureAcademicCourse