Domain Restrictions In Differential Equations and Integrating Factors (Differential Equations 17)

Professor Leonard
29 Oct 201811:06
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the advanced techniques for solving first-order differential equations, emphasizing the importance of understanding the domain restrictions when applying these methods. It introduces the concept of an 'integrating factor' used in linear first-order differential equations and explains the need to avoid dividing by zero and dealing with absolute values. The script prepares viewers for upcoming videos that will delve deeper into these concepts, highlighting the significance of technique and domain considerations in differential equation solving.

Takeaways
  • 📚 The focus of the discussion is on solving first-order differential equations, with an emphasis on linear first-order equations and basic integration techniques.
  • 🔍 The concept of 'separable equations' is introduced as a more specific topic within differential equations that will be explored in future videos.
  • 🔧 The term 'integrating factor' is highlighted as a crucial element in solving linear first-order differential equations, which was previously unmentioned in the series.
  • 📌 The importance of understanding the domain of the variables involved in differential equations is stressed, as it can affect the applicability of certain techniques.
  • ⚠️ Domain restrictions are often necessary to avoid issues such as division by zero or dealing with absolute values, which complicate the solution process.
  • 🌟 The integrating factor is described as the 'missing piece' that completes the product rule, allowing for easier integration.
  • 📈 Examples of linear first-order differential equations are used to illustrate how dividing by a variable can lead to domain restrictions, such as requiring the variable to be greater than zero.
  • 📚 The technique of solving linear first-order differential equations involves multiplying both sides by an integrating factor to simplify the equation.
  • 🤔 The video script encourages viewers to reflect on the technique and domain considerations after learning the basics, to gain a deeper understanding of the process.
  • 🔄 The process of solving differential equations often involves transforming the equation to fit a certain technique, which may introduce domain limitations.
  • 🎯 The goal is to master the technique of solving linear first-order differential equations and to understand the reasoning behind domain restrictions.
Q & A

  • What is the main focus of the video script?

    -The main focus of the video script is to discuss the techniques for solving first-order differential equations, with an emphasis on linear first-order differential equations and the concept of domain issues that may arise when applying these techniques.

  • What is the term used for the missing piece in linear first-order differential equations that completes the product rule?

    -The missing piece in linear first-order differential equations that completes the product rule is called the integrating factor.

  • Why is it important to consider domain issues when solving differential equations?

    -Domain issues are important to consider because they can affect the validity of the solution. Certain techniques may not work if the domain is not properly restricted, and understanding these restrictions can prevent errors and ensure the accuracy of the solutions.

  • What is a common domain restriction for linear first-order differential equations?

    -A common domain restriction for linear first-order differential equations is that the variable with respect to which you are dividing (often X) cannot be zero, as division by zero is undefined.

  • How does avoiding absolute values help in simplifying differential equations?

    -Avoiding absolute values simplifies the differential equations because it allows for easier integration and manipulation of the equations. Having absolute values can complicate the process, especially when trying to apply techniques like the integrating factor.

  • What is the role of the integrating factor in solving linear first-order differential equations?

    -The integrating factor is used to simplify the process of integration by completing the product rule. It is multiplied on both sides of the equation to transform it into a form that can be easily integrated.

  • Why might we restrict the domain to X being greater than zero in some cases?

    -We might restrict the domain to X being greater than zero to avoid dealing with absolute values and to ensure that the integrating factor and the left-hand side of the equation do not include absolute values, which simplifies the process of finding a solution.

  • What is the significance of the term 'integrating factor' in the context of differential equations?

    -The term 'integrating factor' is significant because it refers to a crucial component used in solving certain types of differential equations. It is a factor that, when multiplied with the equation, allows for the application of integration techniques to find a solution.

  • How does the script suggest improving understanding of differential equations?

    -The script suggests that understanding the specific techniques, such as the use of an integrating factor, and being aware of potential domain issues, will improve one's ability to solve differential equations. It emphasizes the importance of not only knowing the techniques but also understanding the underlying principles and potential pitfalls.

  • What type of differential equation often involves the use of an integrating factor?

    -Linear first-order differential equations often involve the use of an integrating factor to simplify the equation and facilitate the integration process.

  • What is the purpose of discussing domain restrictions in the context of solving differential equations?

    -Discussing domain restrictions helps to clarify the conditions under which a particular solution technique can be applied. It ensures that the solutions obtained are valid and applicable within the specified domain, preventing potential errors and misunderstandings.

Outlines
00:00
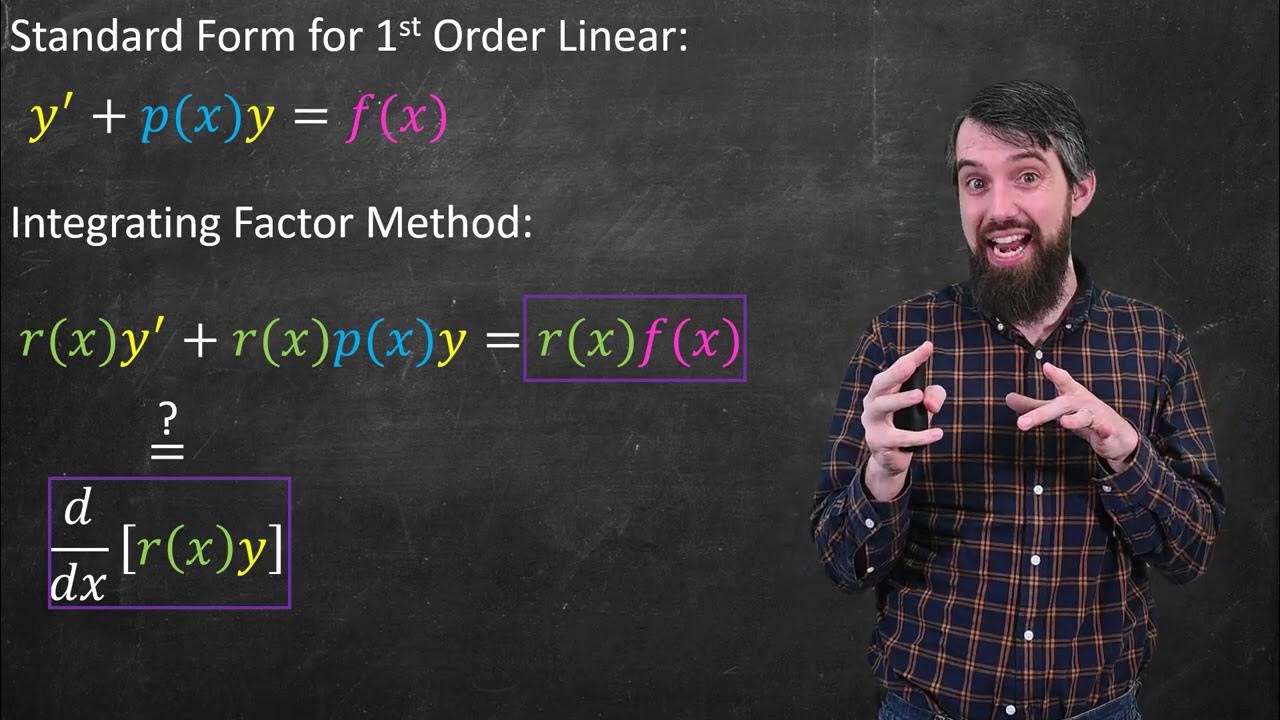
📚 Introduction to Differential Equations and Integrating Factors

The paragraph introduces the concept of first-order differential equations, emphasizing the importance of understanding the techniques for solving them, particularly the separable and linear first-order types. It highlights the need to address domain issues that arise when manipulating these equations to fit specific solving techniques. The paragraph also introduces the term 'integrating factor' as a crucial element in solving linear first-order differential equations, explaining its role in completing the product rule for integration. The speaker acknowledges that previous videos focused on technique rather than terminology but indicates that future videos will delve into both technique and the underlying principles, including potential domain restrictions.

05:00
🔢 Domain Restrictions and Handling Absolute Values in Linear Equations

This paragraph delves into the nuances of solving linear first-order differential equations, particularly the domain restrictions that may be necessary. It explains that dividing by a variable in the process can lead to issues if the variable is zero, hence the need to impose conditions such as ensuring the variable is greater than zero. This not only prevents division by zero but also avoids dealing with absolute values, which can complicate the simplification of equations. The paragraph clarifies that these restrictions are not just about preventing errors but also about facilitating a more straightforward integration process by ensuring that both sides of the equation can be simplified without dealing with absolute values.

10:02
🎓 Recap and Future Focus on Integrating Factors and Domain Conditions

The final paragraph serves as a recap of the key points discussed in the script, emphasizing the importance of understanding both the technique and the domain conditions when working with linear first-order differential equations. It reiterates the role of the integrating factor and the rationale behind avoiding absolute values by restricting the domain to cases where the variable is greater than zero. The speaker assures the audience that future videos will continue to build on the mastery of technique while also addressing the finer points of domain restrictions and their implications for solving differential equations.

Mindmap
Keywords
💡First Order Differential Equations
First Order Differential Equations are equations that involve an unknown function and its first derivative. In the context of the video, the focus is on solving these equations using various techniques, particularly after mastering basic integration methods.
💡Separable Equations
Separable equations are a type of first order differential equation that can be solved by separating the variables into two functions, one involving only 'x' and the other only 'y', which can then be integrated separately. The video script implies that the viewer has previously learned how to handle separable equations and is now moving on to more complex topics.
💡Integrating Factor
The integrating factor is a multiplicative factor used in the solution of certain types of differential equations, particularly linear first order differential equations. It is introduced to make the left-hand side of the differential equation exactly the derivative of a product of the integrating factor and the dependent variable.
💡Domain Issues
Domain issues refer to the restrictions placed on the domain of the independent variable in a function in order for certain mathematical operations or techniques to be valid. In the context of differential equations, these issues often arise when specific techniques require certain conditions to be met, such as the variable not being zero.
💡Product Rule
The product rule is a fundamental calculus rule that states the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function. In the context of the video, the product rule is used when solving differential equations with an integrating factor.
💡Linear Differential Equations
Linear differential equations are equations where the dependent variable and its derivatives appear only to the first power and in a linear combination. The video script focuses on solving these types of equations, especially when they are first order, and introduces the concept of an integrating factor to aid in their solution.
💡Division by Zero
Division by zero is an undefined mathematical operation because zero cannot be a divisor. In the context of the video, this concept is important when solving differential equations, as it is necessary to avoid division by the variable that could potentially be zero.
💡Absolute Value
The absolute value of a number is the non-negative value of that number without regard to its sign. In the context of the video, the absolute value is used in the context of simplifying expressions that come from integrating factors and differential equations, and it is often desirable to avoid dealing with absolute values as they can complicate simplification.
💡Homogeneous Equations
Homogeneous equations are a type of differential equation where the dependent variable and all its derivatives are proportional to the same power of the independent variable. These equations often arise in physics and engineering and can be solved using special techniques.
💡Natural Logarithm
The natural logarithm, denoted as ln, is the logarithm to the base e (where e is an irrational number approximately equal to 2.71828). It is a fundamental concept in calculus and is used in the video to describe the result of integrating certain types of expressions involving division by the variable.
Highlights

Mastering techniques for solving first order differential equations with basic integration.

Transitioning from basic techniques to more specific methods, including handling domain issues.

The importance of understanding when to apply the integrating factor in linear first order differential equations.

The role of the integrating factor in completing the product rule for linear equations.

Addressing domain restrictions, particularly when dividing by zero is not allowed.

The necessity of avoiding absolute values when simplifying differential equations.

How domain issues arise when manipulating differential equations to fit specific solving techniques.

The concept of restricting the domain to X > 0 to simplify equations and avoid absolute values.

The practical application of integrating factors and domain restrictions in solving linear first order differential equations.

The potential complications with homogeneous equations and the use of absolute values or natural logarithms.

The strategic decision to avoid dividing by zero by setting X > 0 in linear differential equations.

The educational approach of first mastering the technique and then understanding the underlying reasons for domain restrictions.

The预告 of upcoming videos that will focus on technique and then revisit the importance of domain considerations.

The emphasis on understanding the technique and the 'why' behind the steps in solving differential equations.

The anticipation of future videos covering the Bernoulli equation and the use of integrating factors.

Transcripts

Browse More Related Video

Substitutions for Homogeneous First Order Differential Equations (Differential Equations 20)

Linear Differential Equations & the Method of Integrating Factors

The Theory of 2nd Order ODEs // Existence & Uniqueness, Superposition, & Linear Independence

Separable Differential Equations (Differential Equations 12)

Special Integration in a Linear Differential Equation Problem (Differential Equations 18)

Reducible Second Order Differential Equations, Missing Y (Differential Equations 26)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Differential EquationsIntegrating FactorsDomain RestrictionsLinear EquationsMathematical TechniquesEducational ContentProblem SolvingAbsolute ValueX Greater Than ZeroProduct Rule