Linear Differential Equations & the Method of Integrating Factors
TLDRThis video script delves into the world of linear differential equations, with a focus on first-order equations and the integrating factor method—a powerful technique for solving these types of equations. The script highlights the general ease of working with linear equations compared to their non-linear counterparts and introduces the concept of a linear ordinary differential equation (ODE). It then presents a standard form for first-order linear ODEs and illustrates the process of using an integrating factor to transform the equation into a more solvable form. The method involves multiplying the equation by a function, r(x), which is derived from the coefficient function of y. The script walks through the steps to find this integrating factor, which turns out to be e^(∫p(x)dx), and then uses it to integrate and solve the equation. The video also touches on the existence and uniqueness of solutions for first-order linear ODEs, provided the functions involved are continuous. The summary concludes with a reminder of the importance of including the 1/r(x) factor when solving these equations, a common oversight among students.
Takeaways
- 📚 The video focuses on first-order linear differential equations and the method of integrating factors, which is a technique for solving such equations.
- 🔍 Linear differential equations are generally easier to understand and work with compared to nonlinear equations, due to their simpler structure.
- 🌟 A linear differential equation is characterized by derivatives of the function y being to the power of 1 and multiplied by coefficient functions.
- 📉 If the right-hand side of the equation (b of x) is zero, the equation is called a homogeneous linear ordinary differential equation.
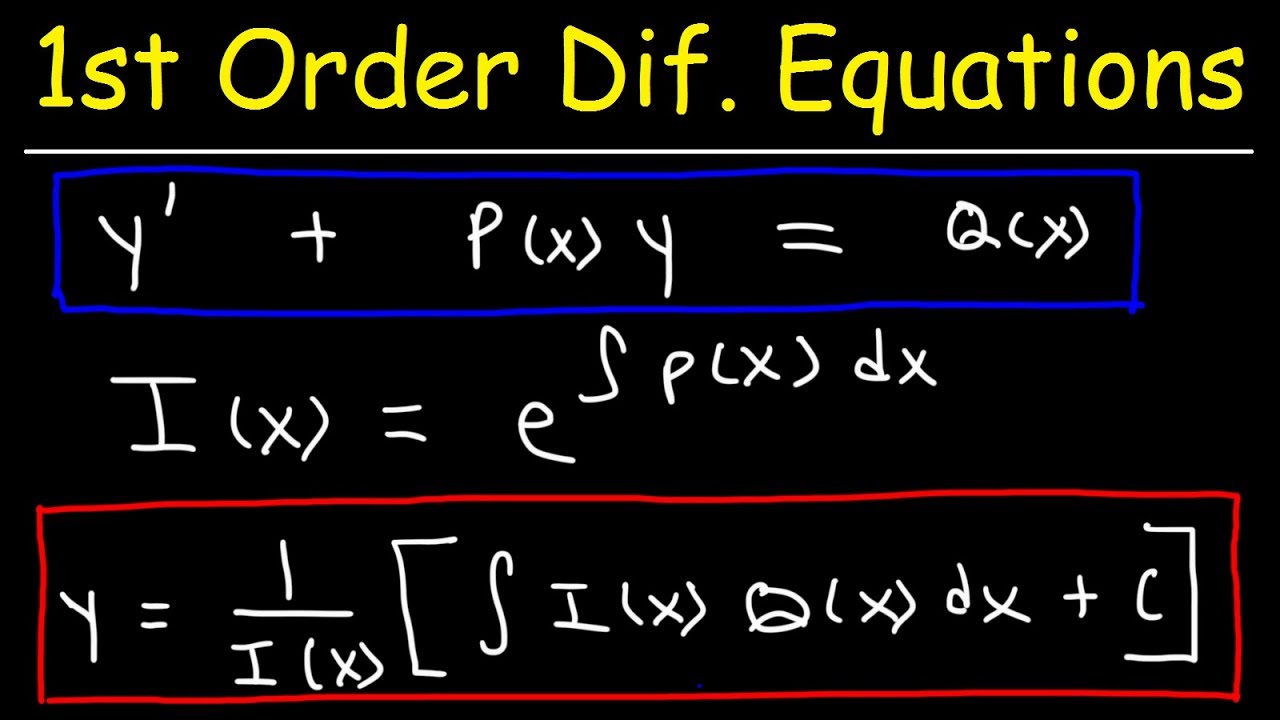
- 📌 The standard form of a first-order linear ordinary differential equation is given by y' + p(x)y = f(x), where p(x) and f(x) are functions of x.
- 🤔 If p(x) were zero, the equation would simplify to y' = f(x), which could be easily solved by integration.
- ✨ The method of integrating factors involves multiplying the differential equation by a function r(x), the integrating factor, to simplify the equation.
- ⚙️ By choosing r(x) = e^(∫p(x)dx), the left-hand side of the equation can be written as the derivative of the product r(x)y, allowing for easy integration.
- 🧮 The integrating factor r(x) is found by solving the differential equation r'(x) = p(x)r(x), which leads to the formula mentioned above.
- 📝 The general solution to the differential equation is y = (∫r(x)f(x)dx) / r(x), where r(x) is the integrating factor.
- ✅ The existence and uniqueness theorem for first-order linear differential equations states that if p(x) and f(x) are continuous on an interval, there exists a unique solution on that interval.
Q & A
What is the main topic of the video?
-The main topic of the video is the study of linear differential equations, with a focus on first order linear differential equations and the method of integrating factors.
Why are linear equations generally easier to work with in mathematics?
-Linear equations are easier to work with because they tend to be more straightforward to understand, manage, and manipulate. They also allow for the development of theorems more readily compared to non-linear equations.
What is a linear differential equation?
-A linear differential equation is an equation where the function y and its derivatives up to the nth order are to the power of 1 and multiplied by coefficient functions of x, without any cross-multiplication or higher powers of the derivatives.
What is a homogeneous linear ordinary differential equation?
-A homogeneous linear ordinary differential equation is a linear ordinary differential equation where the function b of x, which is the term on the right-hand side without any y's, is equal to zero.
What is the standard form of a first order linear ordinary differential equation?
-The standard form of a first order linear ordinary differential equation is expressed as dy/dx + p(x)y = f(x), where p(x) is the coefficient function of y and f(x) is a function of x.
What is the method of integrating factors?
-The method of integrating factors is a technique used to solve first order linear differential equations by multiplying the equation by a function, called the integrating factor, which allows the left-hand side of the equation to be written as the derivative of a product of the integrating factor and the function y.
How is the integrating factor found?
-The integrating factor is found by solving a first order differential equation derived from setting the product of the integrating factor and the coefficient of y in the differential equation equal to the derivative of the integrating factor. The integrating factor is then e to the integral of the coefficient function p(x) dx.
What is the general solution form for a first order linear differential equation using the integrating factor?
-The general solution form is y = (1/R(x)) * ∫(R(x) * f(x) dx), where R(x) is the integrating factor e^(∫p(x) dx).
What is the significance of the existence and uniqueness theorem for first order linear differential equations?
-The existence and uniqueness theorem states that if the functions f(x) and p(x) are continuous on an interval, there exists a unique solution to the first order linear differential equation on that interval.
Why is it important to remember the 1/R(x) factor when solving the differential equation?
-The 1/R(x) factor is crucial because it ensures the solution is correctly scaled. Students often forget this factor, leading to an incorrect solution. It results from dividing both sides of the integrated equation by the integrating factor R(x).
What is the next step after finding the integrating factor in the method of solving a first order linear differential equation?
-After finding the integrating factor, the next step is to multiply the entire differential equation by this factor, then integrate both sides with respect to x to find the function y.
Why does the video mention that constants of integration are not a concern when finding the integrating factor?
-Constants of integration do not affect the method of finding the integrating factor because the integrating factor is used to transform the differential equation into a form that can be directly integrated. The constant will be accounted for when the final solution is integrated.
Outlines
📚 Introduction to Linear Differential Equations
This paragraph introduces the topic of linear differential equations, specifically focusing on first order equations. It discusses the method of integrating factors, which is a technique for solving such equations. The video is part of a larger playlist on differential equations, and a free open source textbook accompanies the content. The paragraph emphasizes the general ease of understanding linear equations compared to non-linear ones, and provides a definition of a linear ordinary differential equation (ODE). It also distinguishes between homogeneous and non-homogeneous linear ODEs, drawing parallels with linear algebra concepts.
🧮 The Integrating Factor Method
The second paragraph delves into the method of integrating factors, which is used to solve first order linear ODEs. It begins by considering what would happen if the coefficient function p(x) were zero, which would simplify the equation to a straightforward integration. The paragraph then explores how to 'fake' this condition by introducing an integrating factor, r(x), which is a function yet to be determined. The goal is to manipulate the equation so that it can be expressed as the derivative of a product (r(x)y), which would allow for easy integration. The paragraph concludes with the derivation of the integrating factor, which is found by setting up an equation that equates r(x)p(x) with the derivative of r(x), and solving for r(x).
🔍 Existence and Uniqueness of Solutions
The final paragraph discusses the theoretical underpinnings of the integrating factor method, specifically the existence and uniqueness of solutions to first order linear ODEs. It states that if the functions f(x) and p(x) are continuous on an interval, then an integral solution exists and is unique on that interval. The paragraph explains that the methodology of multiplying the original equation by the integrating factor and integrating both sides leads to a solution in a specific format. It also touches upon the importance of remembering the factor of 1/r(x) in the final solution, which is a common oversight among students.
Mindmap
Keywords
💡Linear Differential Equations
💡Integrating Factors
💡First Order Linear Differential Equation
💡Coefficient Function
💡Ordinary Differential Equation (ODE)
💡Homogeneous Linear ODE
💡Standard Form
💡Product Rule
💡Existence and Uniqueness
💡Separation of Variables
💡Exponential Function
Highlights
The video focuses on first order linear differential equations and the method of integrating factors.
Linear differential equations are generally easier to understand and manipulate than non-linear equations.
A linear differential equation is characterized by its derivatives being to the power of 1 and multiplied by coefficient functions.
An ordinary differential equation (ODE) is called linear if it follows a specific property involving the coefficient functions of its derivatives.
A homogeneous linear ODE is one where the right-hand side function, multiplied by just the function 1, is zero.
The integrating factor method is introduced as a technique to solve first order linear differential equations.
If p(x) in the differential equation were zero, the equation could be easily solved by simple integration.
The integrating factor, r(x), is found by solving a separable first order differential equation involving p(x).
The integrating factor r(x) is determined to be e^(∫p(x)dx), which simplifies the equation for integration.
The solution to the differential equation is obtained by integrating both sides of the equation after multiplying by the integrating factor.
Students often forget to include the 1/r(x) factor in the solution, which is a common mistake to be aware of.
An existence and uniqueness theorem is presented for first order linear differential equations, stating that if p(x) and f(x) are continuous on an interval, a unique solution exists.
The method of integrating factors can be applied as long as the integrals of p(x) and r(x)f(x) can be computed.
The video provides a general approach to solving first order linear differential equations, with a concrete example to be discussed in the next video.
The importance of verifying the solution by taking the derivative and plugging it back into the original equation is emphasized.
The integrating factor method is a powerful tool for finding solutions to a wide range of first order linear differential equations.
Transcripts
Browse More Related Video

The Theory of 2nd Order ODEs // Existence & Uniqueness, Superposition, & Linear Independence

SC_V1_2 Integrating Factor to Solve Linear ODEs

First order, Ordinary Differential Equations.
First Order Linear Differential Equations

Lec 1 | MIT 18.03 Differential Equations, Spring 2006

Domain Restrictions In Differential Equations and Integrating Factors (Differential Equations 17)
5.0 / 5 (0 votes)
Thanks for rating: