Introduction to Initial Value Problems (Differential Equations 4)
TLDRThe video script offers a comprehensive guide on solving first-order differential equations with initial value problems. It emphasizes the importance of verifying the general solution before solving for the constant 'C' using the initial condition. The process is demonstrated through several examples, each highlighting the steps of verifying the solution, solving for 'C', and rewriting the equation as a particular solution. The script also touches on the application of trigonometric identities and product rules in differentiation, and concludes with a unique problem identifying specific values of a variable that yield a solution to a given differential equation.
Takeaways
- 📚 Differential equations with initial values involve finding particular solutions that satisfy specific conditions.
- 🔍 To solve these problems, there are three main steps: verifying the solution, solving for the constant (C) using initial conditions, and rewriting the equation.
- 🌟 The verification process involves taking the derivative of the proposed solution and ensuring it matches the original differential equation.
- 📈 Initial conditions provide the value of the independent variable (usually X) and the corresponding value of the function (Y).
- 🔧 When verifying solutions, it's crucial to ensure that the solution actually works because if it doesn't, finding a particular solution is irrelevant.
- 📊 The general solution of a differential equation contains an arbitrary constant, representing a family of solutions.
- 🎯 To find a particular solution, replace the arbitrary constant (C) with the specific value derived from the initial condition.
- 🧠 Understanding and applying mathematical identities, such as product and chain rules, is essential for solving and verifying solutions.
- 🔄 The process of solving for C and rewriting the solution is repeated for each initial value problem.
- 🤔 Sometimes, problems may ask for all the values of a certain variable that make a given function a solution to the differential equation, which follows the same process.
- 🚀 Practice is key; the more initial value problems you solve, the more comfortable you'll become with the process and the concepts involved.
Q & A
What is the main topic of the transcript?
-The main topic of the transcript is solving first-order differential equations with initial value problems.
What are the general steps to find particular solutions to a differential equation?
-The three general steps are: 1) Find or verify the solution, 2) Use the initial condition to solve for the constant (C), and 3) Replace the C in the verified solution with the value found to obtain the particular solution.
How do you verify a solution to a differential equation?
-To verify a solution, you take the derivative of the proposed solution and plug it back into the original differential equation to check if it equals the right-hand side of the equation.
What is the role of the constant (C) in the general solution of a differential equation?
-The constant (C) represents the arbitrary nature of the general solution, which is a family of solutions that can be obtained by varying the value of C.
What is an initial condition in the context of differential equations?
-An initial condition is a specified value of the function at a particular point (usually given as (x0, y0)), which is used to determine the particular solution from the general solution.
How does the process of solving for C change when the differential equation involves a function of x with x in it?
-When the differential equation involves a function of x with x in it, you need to apply the product rule when finding the derivative and then verify the solution by plugging the derivative and the original function into the equation.
What is the significance of verifying a solution before solving for C?
-Verifying a solution ensures that the proposed solution is indeed a valid solution to the differential equation. It is crucial because if the solution is not valid, solving for C would be irrelevant and a waste of time.
How can you find all the values of a variable that make a given function a solution to a differential equation?
-You find the derivatives of the given function, plug them into the differential equation, and solve for the variable using algebraic manipulation and identities until you isolate the variable and find its permissible values.
What is the role of trigonometric identities in solving differential equations involving trigonometric functions?
-Trigonometric identities can simplify the expressions when solving differential equations involving trigonometric functions, making it easier to verify the solution and solve for the constant (C).
What is the final step after finding the particular solution to a differential equation?
-The final step is to rewrite the general solution with the particular value of C found from the initial condition, thus obtaining the specific solution that satisfies the given initial value problem.
Outlines
📚 Introduction to Solving Differential Equations
This paragraph introduces the process of solving differential equations with initial values. It explains that differential equations contain derivatives and typically have a general solution with a constant (C). To find a particular solution, an initial value is needed. The speaker outlines a three-step method: verifying the solution, solving for C using the initial condition, and replacing C in the general solution. The paragraph emphasizes the importance of verification to ensure the solution is correct before proceeding.
🔍 Verification and Solution for C
The speaker delves into the verification process of a solution to a first-order differential equation. It describes how to take the first derivative, plug it back into the original equation to confirm it's a valid solution, and then use the initial condition to solve for the constant C. The paragraph provides a worked example, showing the steps to find the particular solution from a general solution for a given differential equation.
📈 Understanding Families of Curves and General Solutions
This paragraph discusses the concept of families of curves and general solutions in the context of differential equations. It explains that a general solution represents a family of curves, each corresponding to a different value of the constant C. The goal is to find the specific curve that passes through a given point, which is done by solving for C using the initial condition. The paragraph reinforces the idea of verifying the solution before solving for C and highlights the process with an example.
🧠 Derivatives and Verification of Solutions
The paragraph focuses on the importance of taking derivatives and verifying solutions for first-order differential equations. It explains the product rule involved in finding derivatives and the process of verifying a solution by plugging the derivative back into the original equation. The speaker provides an example to illustrate the verification process and emphasizes the necessity of this step to ensure the correctness of the solution before solving for C.
🌟 Application of Trigonometric Identities in Verification
This section discusses the application of trigonometric identities during the verification process of a solution to a differential equation. It shows how to use the derivatives of trigonometric functions and identities like secant squared equals tangent squared plus one to verify that a proposed solution is indeed correct. The paragraph includes a detailed example of verifying a solution involving tangent and secant functions.
🔢 Finding Specific Values for a Given Solution
The final paragraph addresses a slightly different problem: finding all the values of a variable that make a given function a solution to a differential equation. The speaker explains that this process involves taking derivatives, plugging them into the equation, and solving for the variable. An example is provided to demonstrate how to find the values of R that satisfy the differential equation, showcasing the steps and the use of algebraic manipulation to arrive at the solution.
Mindmap
Keywords
💡Differential Equations
💡Initial Value Problem
💡Derivatives
💡General Solution
💡Particular Solution
💡Verification
💡Chain Rule
💡Logarithm
💡Exponential Functions
💡Trigonometric Identities
Highlights
The process of solving differential equations with initial values is introduced, emphasizing the importance of verifying the solution before proceeding.
Differential equations contain derivatives and typically have a general solution with a constant 'C' representing a family of solutions.
To find a particular solution, one must use an initial condition to solve for 'C', thus narrowing down the family of solutions to a specific one.
The three-step process for finding particular solutions is outlined: verification of the solution, solving for 'C' using initial conditions, and replacing 'C' in the general solution.
Verification of a solution involves taking the derivative and plugging it back into the original differential equation to ensure it holds true.
The concept of a general solution with an arbitrary constant 'C' is explained, representing different curves that satisfy the differential equation.
The process of solving for 'C' using initial conditions is demonstrated, showing how to replace variables with given values to find the specific solution.
The terminology of differential equations, general solutions, and particular solutions is clarified to enhance understanding.
An example is provided to illustrate the steps of verifying a solution, solving for 'C', and obtaining a particular solution for a first-order differential equation.
The importance of practicing verification and solving for 'C' is emphasized, as it is a fundamental skill for dealing with initial value problems.
The concept of a family of curves is introduced, showing how different values of 'C' result in different curves that satisfy the differential equation.
The process of finding particular solutions is reiterated, emphasizing the need to verify the solution and solve for 'C' using initial conditions.
Additional examples are provided for practice, encouraging learners to apply the taught methods to enhance their understanding.
The use of trigonometric identities is highlighted in the process of verifying solutions and solving for 'C' in differential equations involving trigonometric functions.
The method of solving for specific values of a variable that make a given function a solution to a differential equation is discussed, using the example of finding 'R' values.
The application of product rule and chain rule in finding derivatives of complex functions is demonstrated, crucial for verifying solutions and solving for constants.
The transcript concludes with a preview of future topics, indicating a continuation of the learning journey in understanding rates of change and applications of differential equations.
Transcripts
Browse More Related Video

Initial Value Problems!

Solving Basic Differential Equations with Integration (Differential Equations 6)
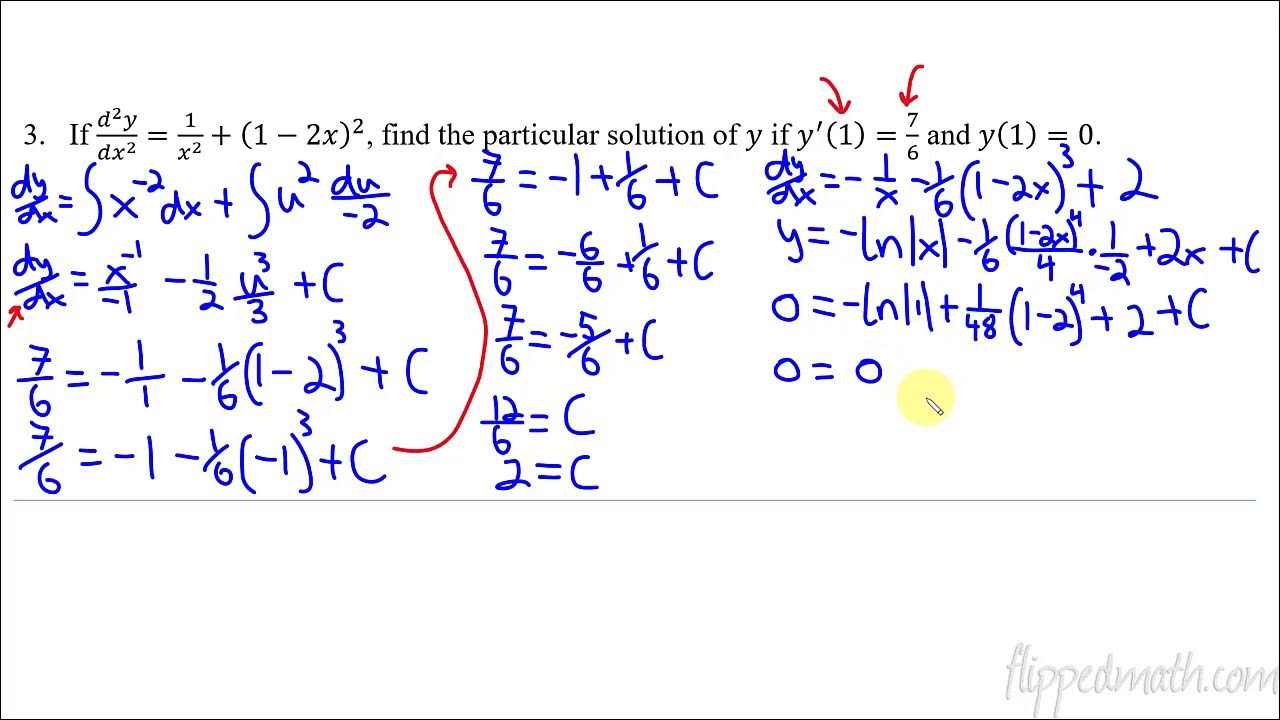
Calculus AB/BC – 7.2 Verifying Solutions for Differential Equations
Homogeneous Differential Equations

Initial Value Problem

Separable differential equations introduction | First order differential equations | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: