2021 Live Review 2 | AP Calculus BC | Preparing for Polar Equations Questions
TLDRIn this instructional video, Tony Record and Brian Pasquader from Avon High School, Indiana, tackle the challenges of polar equations and graphs in BC Calculus. They demystify the concept of polar area, discuss the importance of understanding trigonometric relationships in polar coordinates, and provide strategies for tackling various polar-related problems. The video covers topics such as finding the tangent line to a polar curve, minimizing distance, and calculating polar area, emphasizing the use of technology and graphing calculators to enhance problem-solving skills.
Takeaways
- 📚 The main focus of the transcript is on tackling calculus problems involving polar equations and graphs, particularly for students preparing for the AP Calculus exam.
- 👨🏫 The speaker, Tony Record from Avon High School, and his colleague Brian Pasquader, aim to demystify polar graphs and build students' confidence in solving related problems.
- 📊 The transcript discusses the importance of understanding polar graphs and how they can be converted into Cartesian coordinates, which is crucial for solving AP exam questions.
- 🤔 Students often struggle with polar area problems, but the key formula for calculating the area of a polar region is emphasized as a must-know for the exam.
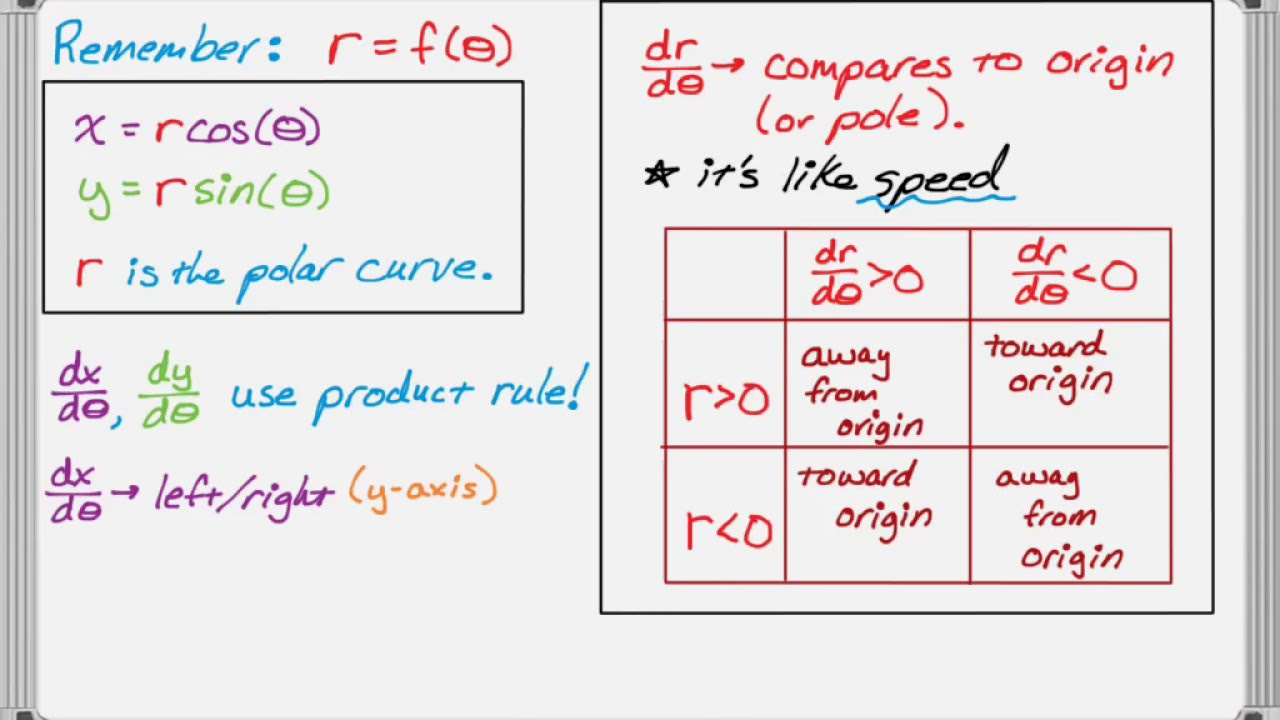
- 📐 The concept of converting polar equations to Cartesian coordinates using the relationships y = r * sin(theta) and x = r * cos(theta) is highlighted.
- 🔢 The use of graphing calculators is encouraged for solving polar problems, especially for finding derivatives, integrals, and intersections, which can expedite the problem-solving process.
- 📝 The transcript provides a step-by-step guide on setting up and solving problems, including finding the area of a polar region, the derivative of a polar function, and the maximum distance between two curves.
- 🌟 The importance of not being afraid of polar graphs and applying knowledge from the entire year of calculus to these problems is stressed.
- 📈 The concept of the average value of a function, in the context of finding the average distance between a point and the origin over a given interval, is introduced.
- 🚫 The transcript warns against common mistakes, such as forgetting to square the r value in polar area calculations or not including the 1/2 factor.
- 🎯 The goal of the session is to help students recognize that polar problems are not as intimidating as they may seem and to equip them with the tools to tackle such problems effectively on the AP exam.
Q & A
What is the main topic discussed in the video?
-The main topic discussed in the video is the calculus of polar equations and how to approach problems involving polar graphs, especially in the context of the AP exam.
Who are the presenters in the video?
-The presenters in the video are Tony Record from Avon High School in Avon, Indiana, and his fellow Hoosier, Brian Pasquader.
What is the formula for the area of a polar region mentioned in the video?
-The formula for the area of a polar region mentioned in the video is (1/2) * ∫[r^2 dθ] from α to β, where f is continuous and not negative on the interval α to β.
How does the video address the common fear students have towards polar graphs?
-The video addresses the common fear students have towards polar graphs by demystifying the concepts and providing detailed explanations on how to tackle different types of polar graph problems, emphasizing that they are not as scary as students might think.
What is the significance of the 'flashlight' analogy used in the video?
-The 'flashlight' analogy is used to illustrate the concept of breaking down the area of a polar region into manageable parts, helping students visualize and calculate the area of complex polar curves more easily.
How does the video handle the transition from polar to Cartesian coordinates?
-The video handles the transition from polar to Cartesian coordinates by using the relationships y = r * sin(θ) and x = r * cos(θ), which allows students to convert polar problems into Cartesian form when necessary.
What is the role of technology in solving polar graph problems as discussed in the video?
-The role of technology in solving polar graph problems, as discussed in the video, is to assist students in performing calculations, such as finding derivatives and integrals, and visualizing the graphs on a calculator, thus streamlining the problem-solving process.
What is the main strategy suggested in the video for tackling polar area problems?
-The main strategy suggested in the video for tackling polar area problems is to carefully apply the formula for polar area, ensuring that the function r is squared and the limits of integration are correctly identified from the problem statement.
How does the video advise students to handle the occurrence of x and y in polar problems?
-The video advises students to handle the occurrence of x and y in polar problems by immediately recognizing the need to switch from polar to Cartesian coordinates and using the relationships x = r * cos(θ) and y = r * sin(θ) to solve the problem.
What is the key takeaway from the video regarding the approach to polar graph problems?
-The key takeaway from the video regarding the approach to polar graph problems is that students should not be afraid of them, as the same principles and calculus knowledge applied to other contexts can be used to solve polar graph problems, and that technology can be a valuable tool in these calculations.
Outlines
📚 Introduction to Polar Equations
The speaker introduces the topic of polar equations, acknowledging the common struggle students face when transitioning from trigonometry to calculus of polar equations. The speaker, Tony Record from Avon High School, assures viewers that they will build confidence in tackling polar problems, and introduces his colleague, Brian Pasquader, to delve deeper into the topic. Brian emphasizes the importance of understanding polar problems, especially on the AP exam, and reviews the weakest area of polar problems where students often score low.
📈 Understanding Polar Graphs and Area Calculation
Brian explains the importance of knowing how to calculate the area of polar graphs, a common question on the AP exam. He provides the formula for polar area and discusses the common mistakes students make, such as forgetting to square the r value or omitting the one-half factor. He also talks about the use of graphing calculators for solving polar problems and emphasizes the importance of not doing calculations by hand when a calculator is allowed.
🤔 Dealing with Unexpected Variables in Polar Problems
The speakers address the common confusion that arises when Cartesian coordinates (x and y) appear in polar problems. They explain the relationship between polar and Cartesian coordinates, using the equations y = r * sin(theta) and x = r * cos(theta). They also discuss how to handle problems that involve finding the derivative or the slope of a tangent line in polar coordinates.
📊 Interpreting Derivatives in Polar Coordinates
Brian and Tony walk through the process of finding the derivative of a polar function and interpreting its meaning. They calculate the derivative dr/dtheta when theta is 5 pi/6 and explain that it represents the rate of change of distance from the origin. They emphasize the importance of understanding what r and theta represent in polar coordinates and how to communicate these concepts clearly in exam responses.
🔍 Finding Theta for a Given y Coordinate
The speakers discuss how to find the value of theta when the graph of r has a y-coordinate of two within a specific interval. They explain the need to convert polar equations to Cartesian coordinates using the formulas y = r * sin(theta) and x = r * cos(theta), and how to solve for theta when given a y value in the context of a polar graph.
📝 Setting Up for Average Distance Calculation
The speakers introduce a problem involving the calculation of the average distance between a point and the origin over a given interval in polar coordinates. They explain that this can be approached as finding the average value of a function, in this case, the distance represented by r. They emphasize the importance of understanding the boundaries provided in the problem and how to set up the integral expression correctly without evaluating it.
🌀 Analyzing Polar Curve Intersections and Areas
The speakers present a problem involving the intersection of two polar curves and the calculation of the area between them. They use the 'magical flashlight' analogy to visualize the area of interest and explain how to set up the integral for the area calculation. They emphasize the importance of understanding the boundaries of integration and how to apply the formula for polar area effectively.
🏆 Maximizing Distance Between Two Polar Curves
The speakers tackle a problem that requires finding the maximum distance between two polar curves. They explain the process of finding critical values by taking the derivative of the distance function and using the candidate's test to determine the maximum value. They demonstrate how to set up and solve the problem using a graphing calculator, highlighting the importance of accurate calculations and the application of calculus principles to polar problems.
🍰 Multiple Choice Questions on Polar Coordinates
The speakers discuss the appearance of polar coordinates in multiple choice questions on the AP exam. They provide a sample problem involving the area of a shaded region of a polar curve and guide viewers through the process of solving it. They emphasize the importance of understanding the context of the problem and applying the correct formula for the area of polar regions.
🚀 Wrapping Up Polar Coordinates Discussion
The speakers conclude their discussion on polar coordinates, encouraging students to focus on the big ideas and not be intimidated by the complexity of polar graphs. They remind students of the key formulas and concepts, such as the relationship between x, y, and r in polar coordinates, and the importance of understanding the meaning behind the calculations. They also encourage students to apply the same principles they've learned throughout the year to polar problems, assuring them that they can achieve high scores on these traditionally challenging sections of the AP exam.
Mindmap
Keywords
💡Polar Equations
💡Trigonometry
💡Graphing Calculator
💡Polar Area
💡Free Response Question (FRQ)
💡Taylor Series
💡Derivative
💡Polar to Cartesian Conversion
💡Average Value
💡Technology Issues
Highlights
The video aims to help students overcome their fear of polar graphs and calculus problems, particularly those related to trigonometry.
The presenter, Tony Record from Avon High School, emphasizes the importance of confidence in tackling polar and trigonometry problems.
The video discusses the common struggles students face with polar area problems and the dread associated with them.
The formula for polar area is demystified, aiming to make students realize its intuitive nature rather than being intimidated by its technical appearance.
The video introduces the concept of using technology, specifically graphing calculators, to solve polar problems efficiently.
The importance of understanding the relationship between polar and Cartesian coordinates is highlighted, especially when unexpected variables like x and y appear in polar problems.
The video provides a detailed walkthrough of solving a polar area problem using the correct formula and calculator techniques.
The concept of finding the derivative of a polar function and its interpretation in terms of distance from the origin is explained.
A method for finding the maximum distance between two polar curves is demonstrated, emphasizing the transferability of calculus concepts to new contexts.
The video addresses how to handle multiple-choice questions involving polar graphs and provides strategies for tackling them.
The presenter shares a technique called the 'magical flashlight' to visualize and calculate the area of polar regions.
The video encourages students to apply the knowledge they've gained throughout the year to solve a variety of polar problems.
The importance of accurate decimal places in intermediate steps is stressed to ensure precision in final answers for the AP exam.
The video concludes with an encouragement for students to practice and familiarize themselves with polar problems to achieve success in the AP exam.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: