Integral Test and p-Series

Chad Gilliland

5 Feb 201415:26

EducationalLearning

32 Likes 10 Comments

TLDRThis video script delves into the concepts of the Integral Test and the P-Series Test for determining the convergence or divergence of a series. It explains the conditions for the Integral Test, including positivity, continuity, and decreasing terms, and demonstrates its application with examples. The script clarifies misconceptions about the relationship between the integral and the series. It also introduces the P-Series Test, highlighting the importance of the power 'P' in the series and providing a quick test for its convergence based on the value of 'P'. The video aims to guide students through these mathematical tests with clear examples and explanations.

Takeaways

📚 The lecture covers the interval test and the p-series test, which are methods to determine the convergence or divergence of a series.
🔍 The interval test involves comparing the integral of a function from 1 to infinity to the series. If the integral converges, so does the series, and vice versa.
📉 The nth term test is used to check the limit as n approaches infinity. If the limit is zero, the series might converge, but further tests are needed for confirmation.
🧩 The first example in the script involves a function that is positive, continuous, and decreasing. The integral test is used to determine its convergence.
📈 The integral of x over x squared plus 1 is used in the first example. The antiderivative is found using u-substitution, leading to a natural logarithm function.
🔍 The natural logarithm of infinity minus the natural logarithm of two is calculated, showing that the integral diverges and thus the series also diverges.
📚 The second example involves the integral of 1 over x squared plus 1. The antiderivative is the arctan of x, and the limit as B approaches infinity is calculated.
📉 The limit of the arctan function as x approaches infinity is π/2, and the integral converges, indicating that the series also converges.
🔢 The p-series test is introduced for series of the form 1/n^p, where p is a positive constant. The series converges if p > 1 and diverges if 0 < p ≤ 1.
📈 The harmonic series, which is a special case of the p-series with p = 1, is shown to diverge using the p-series test.
📚 The lecture also discusses the concept of error estimation in series approximation, demonstrating how to calculate the maximum error using the integral test.

Q & A

What is the purpose of the interval test in the context of the video?
-The interval test is used to determine whether a series converges or diverges by comparing the behavior of the series to the integral of its terms from 1 to infinity.
What conditions must a function meet to be used with the interval test?
-The function must be positive, continuous, and decreasing for the interval test to be applicable.
How does the video explain the relationship between the convergence of an integral and the convergence of a series?
-The video states that if the integral from 1 to infinity of a function converges, then the series formed by the function's terms also converges, and vice versa if the integral diverges.
What is the nth term test mentioned in the video, and what does it indicate?
-The nth term test involves taking the limit of the function's terms as n approaches infinity. If this limit is zero, the test is inconclusive for determining convergence or divergence.
Can the convergence of an integral imply that a series converges to a specific number?
-No, the convergence of an integral to a number does not imply that the series converges to that number. It only indicates that both the integral and the series converge or diverge in the same manner.
What is a Riemann sum, and how is it related to the series discussed in the video?
-A Riemann sum is a method of approximating the definite integral of a function by summing the areas of rectangles. In the context of the video, the series is related to the Riemann sum for the given function over intervals, but it's noted that the Riemann sum is not exactly equal to the integral's value.
How does the video demonstrate the divergence of the series with the integral of 1/(x^2 + 1)?
-The video shows that the integral of 1/(x^2 + 1) diverges by evaluating the antiderivative and taking the limit as the upper limit of integration approaches infinity, which results in the natural logarithm of infinity, indicating divergence.
What is the antiderivative of 1/(x^2 + 1) used in the video to determine the convergence of the series?
-The antiderivative of 1/(x^2 + 1) is the arctan(x), which is used to evaluate the integral from 1 to infinity to determine if the series converges.
What is the significance of the limit of the arctan function as x approaches infinity?
-The limit of the arctan function as x approaches infinity is π/2. This value is used to determine the convergence of the integral and, by extension, the series.
How does the video explain the relationship between the integral test remainder and the approximation of a series?
-The video explains that the integral test remainder provides a way to estimate the maximum error of an approximation when a series is truncated after a certain number of terms. It involves integrating the function from the point of truncation to infinity.
What is a p-series, and how does the video describe its convergence properties?
-A p-series is a series of the form 1/n^p where p is a positive constant. The video describes that a p-series diverges if 0 < p ≤ 1 and converges if p > 1.
How does the video demonstrate the use of the p-series test on a series with a term of 1/(n^(3/2))?
-The video shows that since 3/2 is greater than 1, the series with terms of 1/(n^(3/2)) is a convergent p-series according to the p-series test.

Outlines

00:00

📚 Introduction to Interval and p-Series Tests

The script begins with an introduction to the interval test and the p-series test, which are methods used to determine the convergence or divergence of a series. The interval test involves comparing the series to an integral and checking if the integral converges. If it does, so does the series, and vice versa. The script then explains that the nth term test is not conclusive for the series in question since the limit as n approaches infinity equals zero. The integral test is then applied to a function, and it is shown that if the integral diverges, the series also diverges. The script emphasizes the importance of understanding the relationship between the integral and the series without confusing convergence to a number with the actual convergence of the series.

05:00

🔍 Applying the Integral Test to a Series

In this paragraph, the script delves into the application of the integral test to a specific series. The function to be integrated is 1/(x^2 + 1), and the script explains the process of finding the antiderivative and evaluating the improper integral. The integral is shown to diverge by evaluating the limit as B approaches infinity, which leads to the conclusion that the series also diverges. The script corrects a common misconception about the relationship between the integral and the series's convergence to a number, emphasizing that divergence of the integral implies divergence of the series, but not that the series converges to the value of the integral.

10:04

📉 Estimating Error in Series Approximation

This section of the script discusses how to estimate the error when approximating a series by using a finite number of terms. It introduces the concept of the remainder of a series, which can be found using the integral from the point where the series is truncated to infinity. The script provides an example of approximating a series by summing the first few terms and then calculating the integral from that term to infinity to estimate the maximum error. The example uses a series of 1/n^4 terms, and the script shows how to calculate the error by integrating from the sixth term to infinity, resulting in an error estimate that can be used to bound the actual value of the series.

15:05

📈 The p-Series Test and Its Application

The final paragraph introduces the p-series test, a quick method to determine the convergence of series of the form 1/n^p. The script explains the rules for the p-series test: if p ≤ 1, the series diverges, as exemplified by the harmonic series (p=1). If p > 1, the series converges. The script applies the p-series test to a specific series with p = 3/2, showing that since p is greater than 1, the series converges. The paragraph concludes with a preview of further discussions on different types of series in upcoming sessions.

Mindmap

Keywords

💡Interval Test

The Interval Test is a method used to determine whether an infinite series converges or diverges by comparing it to an improper integral. In the video, the test is applied to a series with a positive, continuous, and decreasing function, where the integral from 1 to infinity of the function is evaluated. If the integral converges, so does the series, and vice versa. The test is central to the video's theme of series convergence.

💡p-Series Test

The p-Series Test is a specific type of test used for series of the form 1/n^p, where p is a positive constant. The test quickly determines the convergence of the series based on the value of p. If p > 1, the series converges; if p ≤ 1, it diverges. The video explains this test as a quick way to analyze series and provides examples to illustrate its application.

💡Convergence

Convergence in the context of the video refers to the property of a series or an integral where the sum or area under the curve approaches a finite limit. The video discusses the conditions under which a series or integral converges, such as when the nth term approaches zero or when the integral of a function from 1 to infinity yields a finite value.

💡Divergence

Divergence is the opposite of convergence, indicating that a series or integral does not approach a finite limit but instead grows without bound. The video script discusses divergence in relation to the nth term test and the improper integral test, showing that if the integral diverges, the series does as well.

💡nth Term Test

The nth Term Test is a preliminary test used to check for divergence of a series by examining the limit of the nth term as n approaches infinity. If this limit is not zero, the test is inconclusive for convergence. In the script, the test is applied to series to determine if further tests are needed to establish convergence or divergence.

💡Integral

An integral in the context of the video represents the area under the curve of a function from one point to infinity. The video uses integrals to compare with series, showing that if the integral converges, the series does as well, and if the integral diverges, so does the series. The integral is a key tool in the interval test.

💡Improper Integral

An improper integral is an integral that has an infinite limit of integration or a discontinuity in the function's domain. In the video, the improper integral is used in the context of the interval test, where the integral from 1 to infinity of a function is evaluated to determine the convergence of a related series.

💡Riemann Sum

A Riemann Sum is a method of approximating the definite integral of a function by summing the areas of rectangles. In the video, the script mentions that plugging in endpoints in a series is similar to calculating a Riemann Sum, but clarifies that the Riemann Sum is not exactly equal to the value of the integral.

💡Antiderivative

An antiderivative is a function that represents the reverse process of differentiation. In the video, finding the antiderivative of a function is a crucial step in evaluating the integral from 1 to infinity, which in turn helps determine the convergence of a series.

💡Harmonic Series

The Harmonic Series is a specific type of series, 1/1 + 1/2 + 1/3 + ..., where each term is the reciprocal of a natural number. The video mentions the harmonic series as an example of a series that diverges, illustrating the application of the p-Series Test.

💡Error Estimate

An error estimate provides a measure of the accuracy of an approximation, such as a series approximation. In the video, the script explains how to calculate the error estimate by integrating the function from the point where the series is truncated to infinity, providing a boundary for the actual value of the series.

Highlights

Introduction to interval test and p-series test for determining series convergence or divergence.

Condition for the series to be tested: positive, continuous, and decreasing function.

Application of integral test for series convergence: if the integral converges, so does the series.

Clarification on the relationship between integral and series convergence without implying the same limit.

Explanation of the nth term test and its limitations with bottom-heavy series.

Demonstration of improper integral handling in the interval test with infinity as a limit.

Integration technique using u-substitution for the function x/(x^2 + 1).

Determination of series divergence through the divergence of the integral.

Second example with a different function and its integral test for convergence.

Recollection of the antiderivative of 1/(x^2 + 1) as arctan(x).

Use of the arctan function's horizontal asymptote to determine integral convergence.

Application of the integral test remainder to estimate series approximation error.

Calculation of error estimation for the series 1/n^4 using integral from a certain term to infinity.

Introduction to the p-series test for series with the form 1/n^p.

Explanation of the divergence of the harmonic series (1/n) when p=1.

Rule for p-series convergence: series converges if p > 1.

Application of the p-series test to determine the convergence of a series with p=3/2.

Transcripts

Browse More Related Video

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

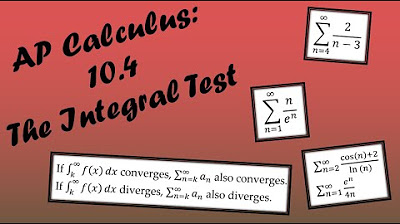
AP Calculus BC Lesson 10.4

Calculus 2 Lecture 9.3: Using the Integral Test for Convergence/Divergence of Series, P-Series

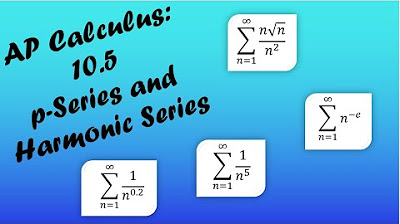
AP Calculus BC Lesson 10.5

Tests for Convergence

Infinite Series Multiple Choice Practice for Calc BC (Part 6)

Integral Test and p-Series

Takeaways

Q & A

What is the purpose of the interval test in the context of the video?

What conditions must a function meet to be used with the interval test?

How does the video explain the relationship between the convergence of an integral and the convergence of a series?

What is the nth term test mentioned in the video, and what does it indicate?

Can the convergence of an integral imply that a series converges to a specific number?

What is a Riemann sum, and how is it related to the series discussed in the video?

How does the video demonstrate the divergence of the series with the integral of 1/(x^2 + 1)?

What is the antiderivative of 1/(x^2 + 1) used in the video to determine the convergence of the series?

What is the significance of the limit of the arctan function as x approaches infinity?

How does the video explain the relationship between the integral test remainder and the approximation of a series?

What is a p-series, and how does the video describe its convergence properties?

How does the video demonstrate the use of the p-series test on a series with a term of 1/(n^(3/2))?