AP Calculus AB 2003 Multiple Choice (no calculator) - Questions 1-28

vinteachesmath

23 Apr 202240:17

EducationalLearning

32 Likes 10 Comments

TLDRIn this detailed video script, Vin walks through the 2003 AP Calculus BC Multiple Choice section without a calculator, explaining each problem's solution methodically. He covers a variety of calculus topics, including the chain rule, u-substitution, limits, derivatives, and integrals. Vin emphasizes the importance of understanding the fundamental theorem of calculus, the quotient rule, and the concept of horizontal asymptotes. The script also discusses the properties of functions, such as continuity, differentiability, and concavity, and provides strategies for solving complex problems efficiently. This comprehensive guide is aimed at helping students prepare for the AP exam by practicing and understanding key calculus concepts.

Takeaways

📚 The script is a walkthrough of the 2003 AP Calculus BC multiple-choice section without a calculator, focusing on problem-solving techniques.
🔑 The first question demonstrates the use of the chain rule for differentiation, emphasizing the importance of applying the power rule correctly.
📈 For definite integrals, the script explains the use of substitution and the fundamental theorem of calculus, highlighting the need to adjust limits of integration correctly.
🌟 The video illustrates how to find horizontal asymptotes using limit definitions, which is crucial for understanding the end behavior of functions.
📝 The quotient rule is covered in detail, showing how to differentiate complex rational expressions by breaking them down into simpler components.
🧩 The script discusses the application of the fundamental theorem of calculus to find antiderivatives, particularly for trigonometric functions like sine.
🔍 When analyzing the behavior of functions, the importance of understanding limits at infinity is highlighted, especially for rational functions.
📉 The video explains how to interpret graphs of derivatives to determine the increasing and decreasing nature of a function.
🔄 The process of u-substitution for integrals is thoroughly explained, including the steps to solve for 'dx' and transform the integral in terms of the new variable.
📌 The chain rule is emphasized as a recurring theme in calculus, especially when differentiating composite functions involving natural logarithms.
⏫ The concept of direct and inverse proportionality is clarified, with examples showing how to express these relationships mathematically.

Q & A

What is the derivative of 2x^3 + 1 using the chain rule?
-The derivative is 6x^2. First, treat (x^3 + 1) as a whole and differentiate it to get 3x^2, then multiply by the derivative of 2x, which is 2.
How does the formula for the integral of e^(ax)dx help in solving definite integrals?
-The formula 1/a * e^(ax) simplifies the process by allowing you to directly compute the integral without the need for u-substitution, as long as you evaluate from the given limits and apply the fundamental theorem of calculus.
What does it mean for a function to have a horizontal asymptote at y=2?
-A horizontal asymptote at y=2 indicates that as x approaches infinity or negative infinity, the function approaches but does not cross the line y=2, meaning the end behavior of the function is to get closer to y=2.
What is the quotient rule for differentiation?
-The quotient rule states that the derivative of u/v is given by (v*u' - u*v')/v^2, where u and v are functions of x, and u' and v' are their respective derivatives.
How does the fundamental theorem of calculus relate to the anti-derivative of sine x?
-The fundamental theorem of calculus connects differentiation and integration. The anti-derivative of sine x is -cos x, which can be verified by differentiating -cos x to get back to sine x.
What is the significance of the leading terms in determining the limit of a rational function as x approaches infinity?
-The leading terms, which are the terms with the highest power of x in both the numerator and the denominator, are the most important in determining the limit of a rational function as x approaches infinity because they dictate the overall behavior of the function at extreme values.
What is the meaning of f'(x) being positive between two points on the graph of a function?
-If f'(x) is positive between two points, it indicates that the function f(x) is increasing in that interval because the derivative represents the slope of the tangent line to the function at any given point.
What is the process of u-substitution for definite integrals?
-U-substitution for definite integrals involves changing the variable of integration to a new variable u, which simplifies the integral. You find du in terms of dx, rewrite the integral in terms of u, and adjust the limits of integration according to the new variable.
What does it imply if a function is directly proportional to another?
-If a function y is directly proportional to another function x, it implies that y can be expressed as y = kx, where k is a constant. This relationship indicates that as x changes, y changes at a rate directly determined by the constant k.
How can you determine if a function is continuous but not differentiable at a certain point?
-A function is continuous at a point if the limit as x approaches that point exists and is equal to the function's value at that point. It is not differentiable at a point if there is a sharp turn or corner in the graph, which means the derivative does not exist or is not defined at that point.
What is the product rule for differentiation and how is it applied?
-The product rule states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function. It is applied by identifying two functions that are being multiplied together in the expression to be differentiated and then applying the rule to find their combined derivative.
What does it mean for a function to be decreasing and how do you find intervals where this occurs?
-A function is decreasing on an interval if its derivative is less than zero on that interval. To find where a function is decreasing, you first find the critical points by setting the derivative equal to zero and solving for x. Then, you test values in the intervals created by these critical points to determine where the derivative is negative.
How do you find the slope of a tangent line to a curve at a given point using the coordinates of the point and another point?
-The slope of the tangent line to a curve at a given point (x1, y1) that also passes through another point (x2, y2) can be found using the formula for the slope of a line: (y2 - y1) / (x2 - x1).
What is the condition for a function to have a point of inflection?
-A function has a point of inflection at a certain point if its second derivative changes signs at that point, indicating a change in concavity.
How is the net change of a function determined from its derivative?
-The net change of a function can be determined by integrating its derivative from an initial point to a final point. According to the fundamental theorem of calculus, this process gives the difference in the function's values at the two points, representing the net change.
What is the formula used to find the derivative of an area function with variable limits?
-The derivative of an area function with variable limits, such as ∫[a(x) to b(x)] f(t) dt, is found using the formula: f(b(x)) * b'(x) - f(a(x)) * a'(x), which is a direct application of the fundamental theorem of calculus with a chain rule.
What are the steps to write the equation of a tangent line given a point and a function?
-To write the equation of a tangent line, you need a point through which the line passes and the slope of the line. The slope is found by differentiating the function and evaluating it at the x-coordinate of the given point. The y-coordinate of the given point provides the value of the function at that point. The equation of the tangent line is then written in point-slope form and simplified as needed.
What does it mean for a particle to be at rest in the context of motion problems?
-In the context of motion problems, a particle is at rest when its velocity, which is the derivative of its position function with respect to time, is equal to zero.
How is implicit differentiation applied with product rules in calculus?
-Implicit differentiation is used when the equation involves a product of variables that cannot be easily separated. The product rule is applied by taking the derivative of each term in the product with respect to x, treating y as a function of x and adding dy/dx accordingly.
What is the formula for the derivative of an inverse function?
-The derivative of an inverse function g(x), where g is the inverse of f, is given by the formula g'(x) = 1 / f'(g(x)). This formula is derived using the definition of an inverse functions and the chain rule.
What does it imply if a function's first and second derivatives are both positive for all real numbers?
-If a function's first and second derivatives are both positive for all real numbers, it implies that the function is strictly increasing and concave up, meaning that the slope of the function is not only positive but also increasing as x increases.

Outlines

00:00

📚 Calculus Multiple Choice Questions Overview

The script begins with Vin introducing a walkthrough of the 2003 Calculus BC multiple-choice section without a calculator. The focus is on solving a variety of calculus problems, starting with the application of the chain rule and power rule for derivatives, moving on to integrals using substitution and the fundamental theorem of calculus, and discussing horizontal asymptotes, quotient rules, and the anti-derivatives of trigonometric functions. Each concept is applied to specific questions, with solutions matched to multiple-choice answers.

05:00

🔍 Analyzing Limits and Derivatives for Function Behavior

This paragraph delves into the analysis of limits at infinity for rational functions, the interpretation of graphs of derivatives to determine the behavior of functions, and the application of u-substitution for integrals. It also covers the chain rule for derivatives of composite functions and the determination of horizontal asymptotes. The discussion includes strategies for solving calculus problems, such as simplifying expressions and using algebraic manipulation to match answer choices.

10:00

📉 Understanding Continuity, Differentiability, and Graphs

The script continues with an exploration of the concepts of continuity and differentiability, using piecewise functions and their properties. It discusses how to determine where functions are increasing or decreasing by analyzing derivatives and how to identify points of inflection by examining second derivatives. The importance of recognizing the shape of graphs and their concavity is highlighted, along with the application of these concepts to multiple-choice questions.

15:02

📝 Techniques for Solving Derivatives and Integrals

Here, the focus shifts to techniques for solving derivatives and integrals, including the product rule, chain rule, and the fundamental theorem of calculus. The paragraph covers how to find the equation of a tangent line at a given point on a curve, how to determine where a function is concave down, and how to interpret tables of values for derivatives. The summary of each technique is followed by an application to a specific problem with a corresponding answer choice.

20:04

🧩 Solving Complex Calculus Problems with Multiple Concepts

The script presents a series of complex calculus problems that require the simultaneous application of multiple concepts, such as the product rule, chain rule, and understanding the behavior of piecewise functions. It discusses how to find derivatives of implicit functions, solve for the equation of a curve given a differential equation, and determine the continuity and differentiability of functions at specific points. Each problem is solved step by step, with an emphasis on the logical flow and mathematical reasoning involved.

25:05

📈 Interpreting Graphs and Calculating Areas for Net Change

This paragraph discusses the interpretation of graphs to find the net change of a function, using the integral of a derivative. It explains how to calculate the value of a function at a specific point by considering the area under the curve and the application of the fundamental theorem of calculus. The explanation includes the use of right triangles to represent areas and the importance of understanding the geometric interpretation of integrals.

30:08

🤔 Advanced Calculus Concepts and Problem-Solving Strategies

The script concludes with advanced calculus concepts, such as the derivative of an area function with variable limits, the equation of a tangent line, and the motion of a particle. It emphasizes problem-solving strategies, including the use of the fundamental theorem of calculus with chain rules, point-slope form for tangent lines, and implicit differentiation. Each concept is applied to a problem, with a clear explanation of the steps involved in finding the solution.

35:09

🏁 Wrapping Up the No Calculator Section

In the final paragraph, the script wraps up the no calculator section of the calculus problems. It briefly mentions the importance of understanding the behavior of functions given their derivatives and the significance of knowing calculus formulas for the AP test. The summary ends with a note of thanks for watching the tutorial and wishing good luck on the AP test.

Mindmap

Keywords

💡Chain Rule

The Chain Rule is a fundamental principle in calculus for finding the derivative of a composite function. It states that the derivative of a function composed of two functions is the derivative of the outer function times the derivative of the inner function. In the video, the Chain Rule is applied to find the derivative of a function where an exponent is applied to a sum, exemplified by the derivative of (2x^3 + 1)^1.

💡Power Rule

The Power Rule is a basic differentiation rule stating that if you have a function of the form f(x) = x^n, where n is a constant, then the derivative f'(x) = n*x^(n-1). In the script, the Power Rule is used to differentiate the inner function of a composite function and to find the derivative of terms like x^3 in various contexts.

💡U-Substitution

U-Substitution is a technique used in integral calculus to simplify the evaluation of integrals. It involves substituting a part of the integrand with a new variable, often to create a new integral that is easier to solve. In the video, U-Substitution is used to evaluate integrals involving exponential functions multiplied by a linear term.

💡Fundamental Theorem of Calculus

The Fundamental Theorem of Calculus is a key theorem that links the concept of integration with differentiation. It states that the definite integral of a function can be calculated using the antiderivative of the function, evaluated at the limits of integration. The video mentions this theorem in the context of evaluating definite integrals without the need for U-Substitution.

💡Horizontal Asymptote

A horizontal asymptote is a horizontal line that a function approaches but never crosses as the function's input (x) approaches infinity or negative infinity. In the script, the concept is discussed in the context of a function's end behavior, where the function gets closer and closer to the line y=2 without crossing it.

💡Quotient Rule

The Quotient Rule is a differentiation rule for finding the derivative of a quotient of two functions. It states that the derivative of the quotient u/v is (v*u' - u*v') / v^2. In the video, the Quotient Rule is applied to find the derivative of a function that is a fraction of two polynomials.

💡Anti-Derivative

An anti-derivative, also known as an integral, is a function that represents the area under the curve of another function. In the context of the video, anti-derivatives are used to find the net change of a function over an interval, as well as to solve integrals.

💡Limit Definition

The limit definition is a concept in calculus that describes the behavior of a function as the input approaches a certain value. In the script, the limit definition is used to discuss the behavior of a function as x approaches infinity and to define horizontal asymptotes.

💡Continuity and Differentiability

Continuity and differentiability are properties of functions. A function is continuous at a point if the limit of the function as x approaches that point is equal to the function's value at that point. A function is differentiable at a point if it has a derivative at that point. In the video, these concepts are discussed in the context of piecewise functions and their properties at certain points.

💡Product Rule

The Product Rule is a differentiation rule used when finding the derivative of a product of two functions. It states that the derivative of uv is u'v + uv'. In the script, the Product Rule is used in conjunction with the Chain Rule to differentiate complex functions involving products.

💡Implicit Differentiation

Implicit Differentiation is a technique used when a function is not explicitly given in the form y = f(x), but rather the relationship between x and y is given implicitly. It involves differentiating both sides of an equation with respect to x and solving for the derivative of the function of interest. In the video, implicit differentiation is used to find the derivative of an equation where y is squared and multiplied by a term involving x.

Highlights

Derivative of a function using the chain rule and power rule for the first question.

Using the formula for the integral of e^(kx) for a definite integral without substitution.

Understanding the limit definition of a horizontal asymptote and its application to a function.

Applying the quotient rule to find the derivative of a rational function.

Evaluating the integral of sine using the fundamental theorem of calculus.

Analyzing the behavior of a rational function as x approaches infinity to determine its horizontal asymptote.

Interpreting a graph of f' to make a true statement about the function f(x).

Performing u-substitution for an integral involving cosine and a cubic function.

Using the chain rule to find the derivative of a composite function involving a natural logarithm.

Determining the graph of a function based on the signs of f(x), f'(x), and f''(x).

Applying u-substitution to a definite integral with a new variable u.

Understanding direct and inverse proportionality to solve a problem involving volume and its rate of change.

Identifying the point where a function is continuous but not differentiable based on its graph.

Using the product and chain rules to find the derivative of a function involving x^2 and sine(2x).

Determining the intervals where a function is decreasing by analyzing its first derivative.

Solving for the slope of a tangent line to a curve at a given point and its implications.

Finding where a function is concave down by analyzing the second derivative.

Interpreting a table of values for g'(x) to determine where g(x) is decreasing.

Solving a differential equation using separation of variables and an initial condition.

Analyzing the continuity and differentiability of a piecewise function at a specific point.

Identifying points of inflection by analyzing the sign changes of the second derivative.

Calculating the net change of a function using the integral of its derivative.

Applying the fundamental theorem of calculus with a chain rule to find a derivative.

Writing the equation of a tangent line using a given point and the slope from the derivative.

Determining when a particle is at rest by finding the roots of its velocity function.

Using implicit differentiation and product rules to solve for dy/dx in a given equation.

Finding the derivative of an inverse function using a specific formula.

Estimating a possible value for g(6) given that g'(x) and g''(x) are positive for all real numbers x.

Transcripts

Browse More Related Video

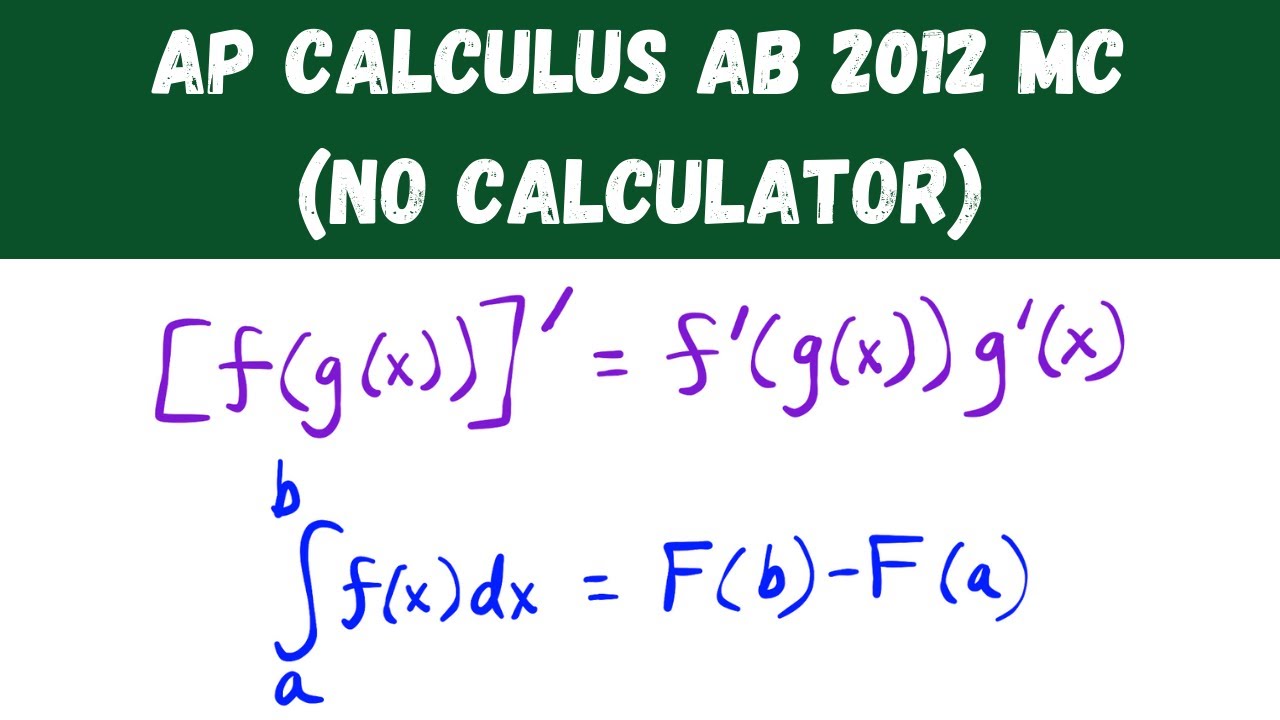
AP Calculus AB 2012 Multiple Choice (no calculator) - Questions 1-28

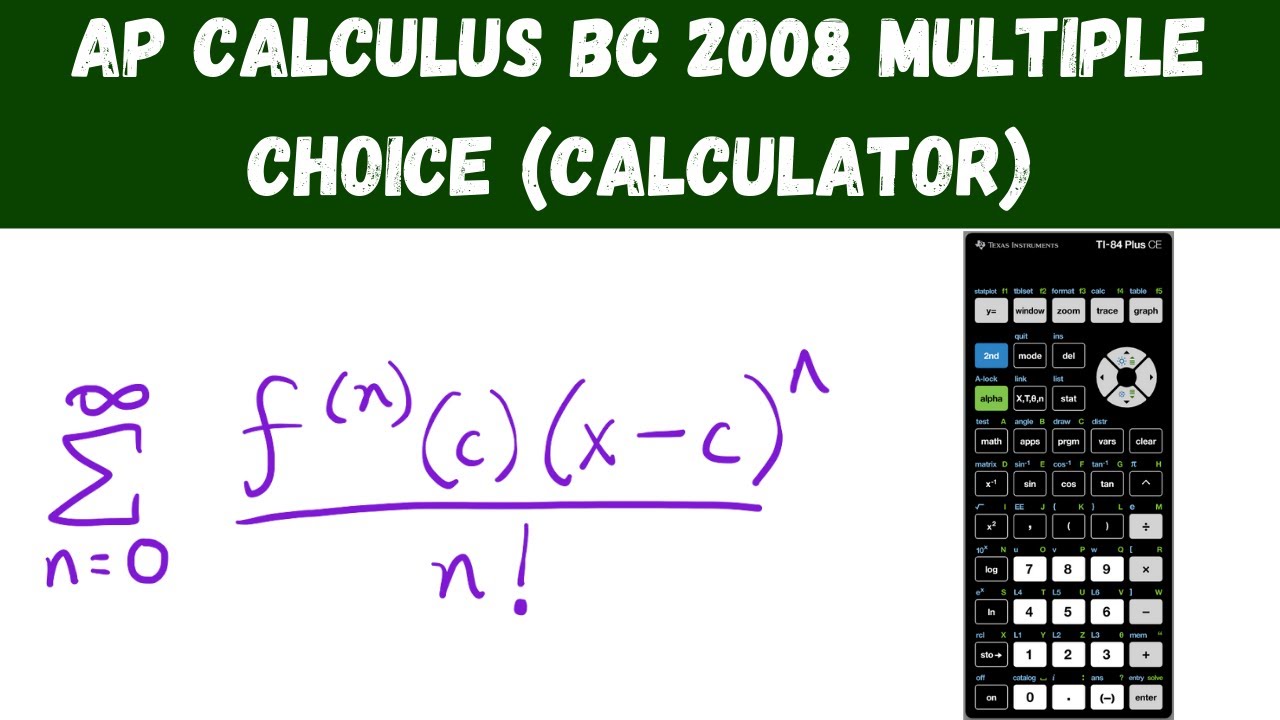
AP Calculus BC 2008 Multiple Choice (calculator) - Questions 76 - 92

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92

AP Calculus BC 2008 Multiple Choice (no calculator) - questions 1 - 28

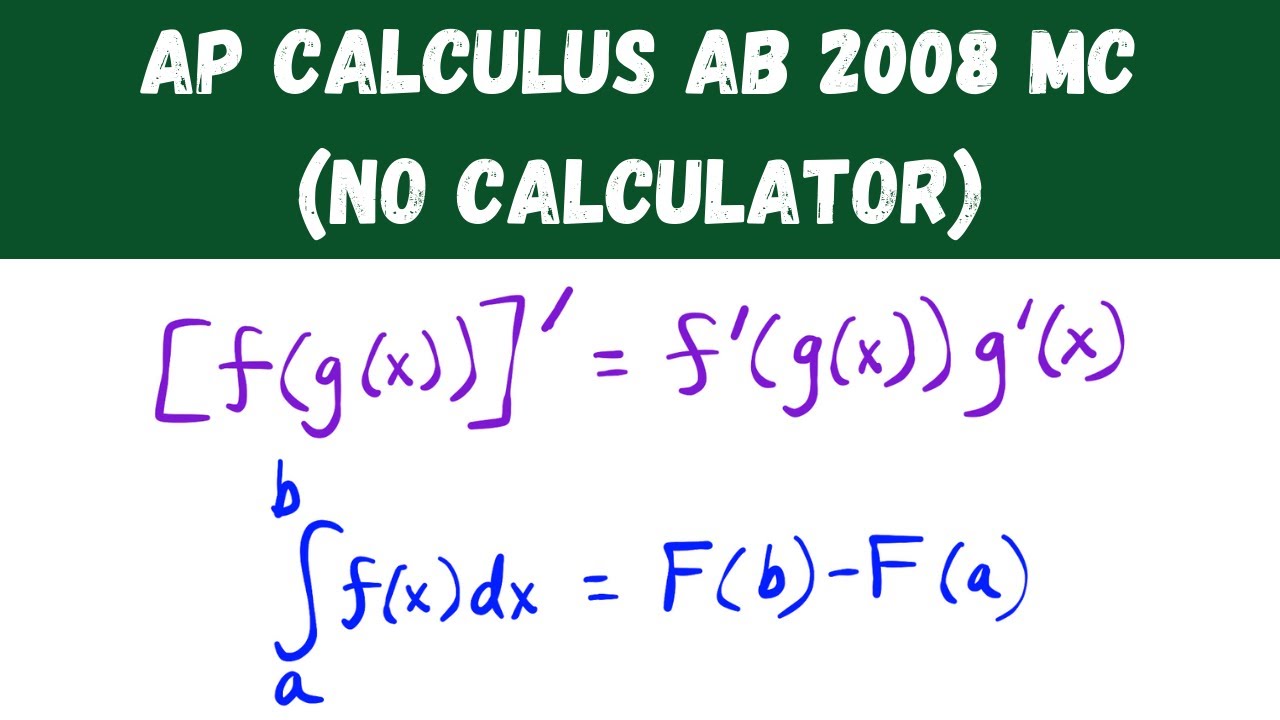
AP Calculus AB 2008 Multiple Choice (No Calculator)

AP Calculus AB Review

Related Tags

Calculus AP Test No Calculator Derivatives Integrals Chain Rule U-Substitution Horizontal Asymptote Quotient Rule Fundamental Theorem