Tensor Calculus Lecture 13a: Integration - The Arithmetic Integral
TLDRThis lecture delves into the concept of invariant integration, a fundamental aspect of calculus. It discusses translating invariant notions into coordinate representations, focusing on defining invariant integrals over domains and surfaces without relying on coordinate systems. The lecturer then explains how to represent these integrals analytically once a coordinate system is introduced, emphasizing the importance of choosing appropriate limits to ensure the domain is accurately captured. The summary also hints at the divergence theorem and its applications in Riemann spaces, promising a deeper exploration in subsequent sessions.
Takeaways
- 📚 The script discusses the concept of integration in the context of calculus, emphasizing the translation of invariant notions into coordinate representations.
- 🔍 It introduces the idea of invariant integrals, which are integrals that remain consistent regardless of the coordinate system used.
- 📐 The script explains that to define an invariant integral, one must consider a closed domain and an invariant field, which could be a scalar or vector field.
- 🧩 The process of defining an invariant integral involves breaking up the domain into small segments or patches and summing the product of the field's value and the volume or area of each segment.
- 🌐 The script mentions that the definition of the invariant integral is conceptual and does not require coordinates, but an intuitive understanding of volume and area is necessary.
- 📈 The script transitions to discussing how to represent these invariant integrals algebraically or analytically once a coordinate system is introduced.
- 📝 It explains that once a coordinate system is chosen, the invariant field becomes a function of the coordinates, and the volume or area elements become part of the analytical representation of the integral.
- 📖 The script references a textbook for technical proofs and details, indicating that the focus is on the conceptual understanding rather than the proofs themselves.
- 🌟 The fundamental theorem of integration, Gauss's theorem, is mentioned as a key concept, with the script stating it as a slight generalization of the fundamental theorem of calculus.
- 📊 The script promises to show applications of the divergence theorem, which is a significant application in Riemann spaces and is related to the fundamental theorem of calculus.
- 📚 The final takeaway is the importance of understanding how to translate the geometric definition of an integral into an analytical expression that yields consistent results across different coordinate systems.
Q & A
What is the primary goal of all authentic calculus?
-The primary goal of all authentic calculus is to translate invariant notions into coordinate representation.
What is an invariant integral?
-An invariant integral is a concept in calculus where the integral of a field over a domain is defined without reference to a specific coordinate system, ensuring the result is consistent regardless of the coordinate system used.
What is the fundamental theorem of integration in the context of the script?
-The fundamental theorem of integration mentioned in the script refers to Gauss's theorem, which is a generalization of the fundamental theorem of calculus and is used in the context of divergence theorem applications.
What is the divergence theorem?
-The divergence theorem, also known as Gauss's divergence theorem, is a theorem in vector calculus that relates the flux of a vector field through a closed surface to the divergence of the field in the volume enclosed by the surface.
How is the invariant integral defined conceptually?
-The invariant integral is defined conceptually by breaking up the domain into small patches, multiplying the value of the field at any point within the patch by the volume of the patch, summing these products, and taking the limit as the patches become infinitesimally small.
What is the significance of the volume element in the context of the script?
-The volume element is significant as it represents the infinitesimal volume in a coordinate system that is used in the analytical representation of the invariant integral, ensuring the integral's result is invariant under coordinate transformations.
How does the choice of coordinate system affect the analytical representation of an integral?
-The choice of coordinate system affects the analytical representation of an integral by determining the specific form of the function representing the field and the limits of integration, but the final numerical result of the integral should remain invariant under different coordinate systems.
What is the role of the area element in the analytical representation of a surface integral?
-The area element in the analytical representation of a surface integral represents the infinitesimal area on the surface, which is multiplied by the value of the field at that point and integrated over the surface to give the total flux.
Why is it important to carefully consider the limits of integration?
-It is important to carefully consider the limits of integration to ensure that the domain of integration is properly captured, which is crucial for obtaining the correct result of the integral.
What are some applications of the divergence theorem mentioned in the script?
-The script mentions three very striking vivid applications of the divergence theorem, although it does not specify what these applications are. The theorem has wide-ranging applications in physics, including fluid dynamics and electromagnetism.
Outlines
📚 Introduction to Integration and Invariant Integrals
This paragraph introduces the concept of integration within the context of calculus, emphasizing the importance of translating invariant notions into coordinate representations. The speaker outlines the plan to discuss invariant integrals and their arithmetic representation, including the fundamental theorem of integration, which is a generalization of the familiar fundamental theorem of calculus. The paragraph also mentions Gauss's divergence theorem, which is central to integration in Riemann spaces, and promises to present three vivid applications of this theorem. The discussion begins with an invariant geometric constant and its representation without the use of coordinates, using a closed domain Omega and an invariant field F as examples. The concept of the invariant integral is explained through the intuitive method of breaking up the domain into small segments and summing their contributions to find the total content within the domain.
📘 Analytical Representation of Invariant Integrals
The second paragraph delves into the translation of the invariant integral into an analytical form that can be evaluated in any coordinate system. The speaker explains that once a coordinate system is introduced, the invariant field F becomes a function of the coordinates, and the volume or area elements become dependent on the choice of coordinates. The process involves taking repeated integrals with respect to the coordinate variables, ensuring that the limits of integration properly capture the domain of interest. The paragraph illustrates how to apply this process in three-dimensional space using spherical and Cartesian coordinates, highlighting the importance of considering the correct limits and signs to ensure the result's invariance. The speaker concludes by emphasizing that this analytical representation of the geometric definition allows for consistent numerical results across different coordinate systems, as detailed in the textbook.
Mindmap
Keywords
💡Integration
💡Invariant Integrals
💡Arithmetic Operation
💡Fundamental Theorem of Calculus
💡Gauss's Theorem
💡Convergence Theorem
💡Riemann Spaces
💡Vector Field
💡Coordinate System
💡Volume Element
💡Surface Integral
💡Analytical Representation
Highlights
Introduction to integration as a vast topic with a focused approach in authentic calculus.
The goal of translating invariant notions into coordinate representation in calculus.
Discussion on invariant integrals and their representation through arithmetic operations.
The fundamental theorem of integration and its relation to Gauss's theorem.
A slight generalization of the fundamental theorem of calculus as presented.
Three vivid applications of the divergence theorem in the context of integration.
Clarification on the divergence theorem being the primary theorem in Riemann spaces.
The conceptual definition of an invariant integral without coordinates.
Breaking down the domain into small segments to define the invariant integral.
The intuitive understanding of the invariant integral in various dimensions.
Transition to expressing the integral algebraically after introducing a coordinate system.
The importance of the volume element in the analytical representation of an integral.
How the limits of integration must capture the domain properly for an accurate result.
The invariant representation of the geometric definition in analytical terms.
The significance of the area element in the analytical representation of surface integrals.
The process of translating the integral into an analytical expression for evaluation in any coordinate system.
The explanation of why the analytical expression remains invariant regardless of coordinate choice.
The upcoming discussion on the divergence theorem and its importance in calculus.
Transcripts
Browse More Related Video
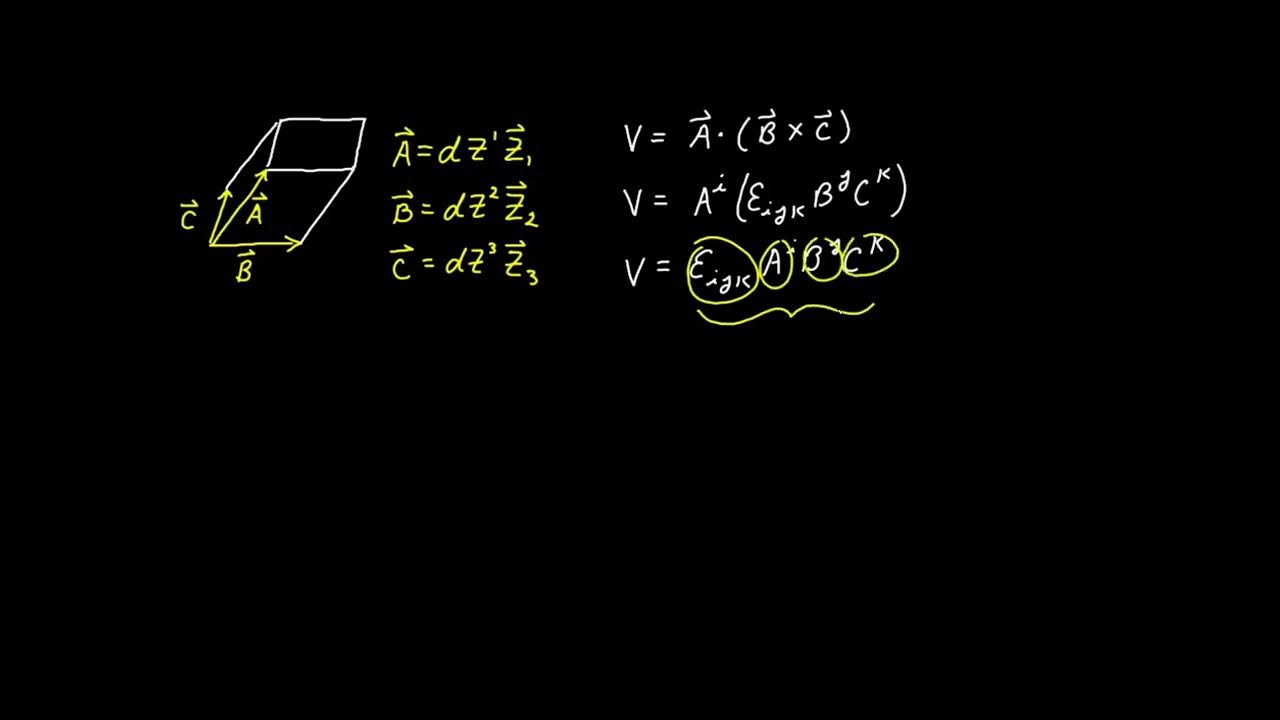
Video 54 - Integration

Multivariable Calculus Lecture 1 - Oxford Mathematics 1st Year Student Lecture
Tensor Calculus 3b: Change of Coordinates

Tensor Calculus 4b: Position Vector, Covariant Basis, Covariant Metric Tensor, Contravariant Basis

Tensor Calculus Lecture 8b: The Surface Derivative of the Normal

Video 17 - Contravariant Basis
5.0 / 5 (0 votes)
Thanks for rating: