Tensor Calculus 12: The Metric Tensor in Curved Spaces for Measuring Arc Length
TLDRThis educational video explores the concept of calculating curve lengths in curved spaces using the metric tensor. It delves into the distinction between extrinsic and intrinsic geometry, emphasizing the importance of the metric tensor in measuring distances accurately in curved spaces like a sphere. The video illustrates the process of mapping a 2D plane onto a 3D surface and demonstrates how to compute the arc length of a curve on a sphere both extrinsically and intrinsically. It concludes by highlighting the significance of the metric tensor field in understanding the varying rules for measuring distance across different points in a curved space.
Takeaways
- 📚 The video discusses calculating the lengths of curves in curved spaces using the metric tensor and covers the differences between extrinsic and intrinsic geometry.
- 🔗 It is recommended to watch previous videos on the metric tensor for foundational concepts before understanding this video's content.
- 🌐 The script introduces the concept of the metric tensor, also known as the first fundamental form, essential for understanding curved spaces.
- 📘 The video references course notes and a PDF on surfaces that discuss the metric tensor for those interested in further study.
- 🌀 The main example used in the video is the sphere, explaining how to mathematically describe a 2D surface by transforming a flat plane into a 3D space.
- 📈 The transformation of a flat plane to a spherical surface is illustrated using the UV coordinates and their corresponding X, Y, Z coordinates.
- 📍 The video explains how to map paths from the UV plane onto the spherical surface and how to calculate the length of these paths using the tangent vector.
- 🧭 The concept of extrinsic geometry is introduced, which involves analyzing the surface in relation to the surrounding 3D space, using Cartesian coordinates.
- 🌈 Intrinsic geometry is presented as an alternative, focusing solely on the surface itself without reference to the external 3D space, using UV coordinates.
- 📊 The video demonstrates how to compute the metric tensor components for a sphere to understand how distance measurement changes across the surface.
- ⚖️ The importance of the metric tensor in curved spaces is emphasized, as it provides the rules for measuring distance, which can vary from point to point.
- 🌍 The script concludes by explaining that every curved space has its own metric tensor field, which is crucial for understanding the geometry of the space.
Q & A
What is the main topic of the video?
-The video discusses how to calculate the lengths of curves in curved spaces using the metric tensor and covers the differences between extrinsic and intrinsic geometry.
What are the two types of geometry the video mentions?
-The two types of geometry mentioned are extrinsic geometry, which involves analyzing curved spaces in relation to the surrounding 3D space, and intrinsic geometry, which focuses on the curved space itself without reference to the surrounding space.
What is the metric tensor also known as?
-The metric tensor is also known as the first fundamental form in the context of the geometry of curved spaces.
What is the purpose of the metric tensor in the context of curved spaces?
-The metric tensor is used to compute the lengths of curves on a surface and provides the rules for measuring distance in curved spaces.
Why is it recommended to watch the previous videos on the metric tensor before this video?
-It is recommended to watch the previous videos because this video builds on the concepts introduced in those videos, and understanding those concepts is essential for grasping the material in this video.
What is an example of a curved 2D space discussed in the video?
-Examples of curved 2D spaces discussed in the video include the cylinder, the hyperbolic paraboloid, and the sphere, with a focus on the sphere.
How is a 2D surface mathematically described according to the video?
-A 2D surface is mathematically described by starting with a flat 2D plane with UV coordinates and applying a function that stretches and transforms the plane into a 2D surface in 3D space.
What is the significance of the transformation equations for the sphere example given in the video?
-The transformation equations for the sphere example map the UV plane onto a spherical surface, with the U coordinate lines becoming lines of longitude and the V coordinate lines becoming lines of latitude.
How does the video explain the process of drawing curves on a spherical surface?
-The video explains that to draw curves on a spherical surface, one takes the equation of a path in the UV plane and applies the same function used to map the plane onto the sphere, resulting in a path on the 2D spherical surface.
What is the key to getting the lengths of curves in curved spaces as per the video?
-The key to getting the lengths of curves in curved spaces is to use the magnitude of the tangent vector dR/dλ, where R is the position vector of a point on the curve in 3D space.
What is the difference between extrinsic and intrinsic approaches to studying surfaces as explained in the video?
-The extrinsic approach involves analyzing curved 2D spaces like a sphere within the surrounding 3D space, while the intrinsic approach focuses solely on the curved space itself, without considering the surrounding space.
How does the video illustrate the concept of intrinsic geometry?
-The video illustrates intrinsic geometry by examining the surface without looking at the surrounding space, using the UV plane as a reference and considering the tangent vectors in terms of U and V variables.
What is the role of the metric tensor in calculating the arc length of a curve on a surface?
-The metric tensor is used to compute the squared magnitude of the curve's tangent vector, which is then used to calculate the arc length of the curve on the surface.
Why does the video mention using Wolfram Alpha to compute the arc length?
-The video mentions using Wolfram Alpha because the integral required to compute the arc length of a curve is difficult to solve by hand, and Wolfram Alpha can provide the solution efficiently.
What does the video imply about the relationship between the metric tensor and the measurement of distance in curved spaces?
-The video implies that the metric tensor provides the rules for measuring distance in curved spaces, and that these rules can vary from point to point on the surface due to the curvature.
How does the video explain the concept of a metric tensor field?
-The video explains a metric tensor field as a field where a different metric tensor is placed everywhere in space, changing from point to point, which gives the rules for measuring distance on a curved surface.
What is the final takeaway from the video regarding the study of surfaces?
-The final takeaway is that when studying surfaces intrinsically, they all have their own metric tensor field that provides the rules for measuring distance at various places in the curved space, and this is essential for accurately understanding the geometry of the surface.
Outlines
📚 Introduction to Curved Spaces and Geometry
This paragraph introduces the topic of calculating the lengths of curves in curved spaces using the metric tensor. It differentiates between extrinsic and intrinsic geometry, and suggests watching previous videos for foundational knowledge. The speaker also recommends course notes for further study, particularly the section on surfaces and the metric tensor, also known as the first fundamental form. The paragraph sets the stage for a deeper dive into the geometry of spheres, starting with the mathematical description of a 2D surface in 3D space, using UV coordinates and transformation functions.
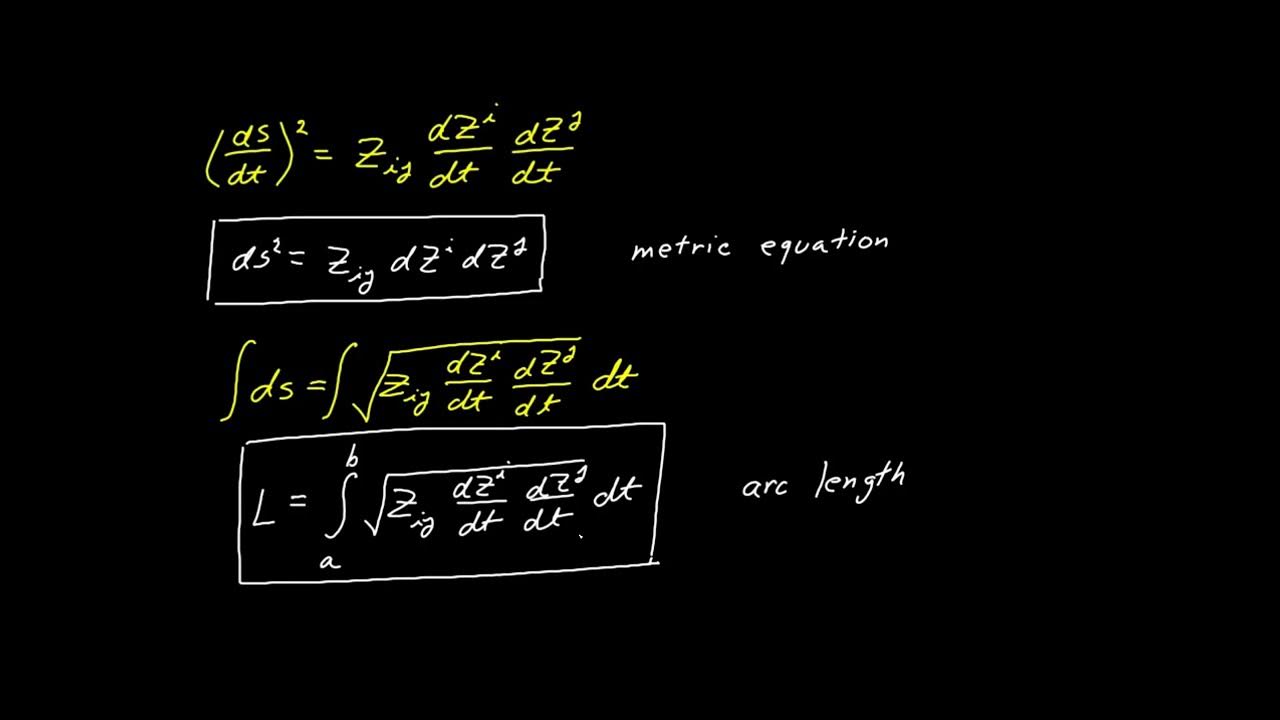
🌐 Understanding Curved Lengths and the Metric Tensor
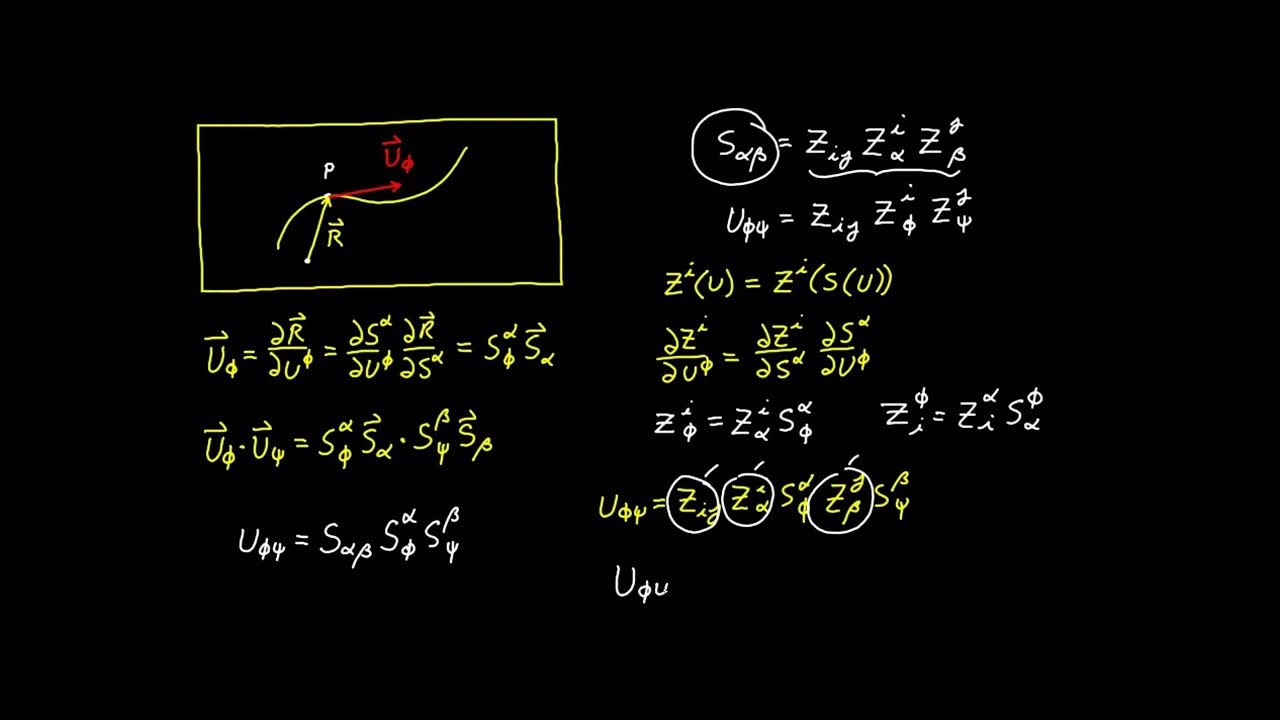
The speaker explains how to calculate the length of a curve on a spherical surface using the metric tensor in an extrinsic geometry context. The process involves transforming a path from the UV plane to 3D space and then using the tangent vector's magnitude to determine the arc length. The paragraph delves into the mathematical details of this process, including the use of the chain rule and the simplification of the dot product due to the orthonormal basis of the Cartesian coordinate system. The intrinsic geometry approach is also introduced, contrasting it with the extrinsic method by focusing on the UV plane instead of the surrounding XYZ space.
📐 Calculating the Sphere's Metric Tensor
This paragraph focuses on the intrinsic geometry of a sphere, detailing the steps to compute the metric tensor components by differentiating the transformation equations with respect to the UV coordinates. The tangent vectors along the U and V curves are expanded in terms of the Cartesian variables, and the metric tensor is calculated using the dot products of these basis vectors. The resulting metric tensor is shown to have a diagonal form with sine squared of U as a scaling factor for the V component, illustrating how the measurement of distance varies across the sphere's surface.
🌍 Analyzing Curve Lengths on a Sphere's Surface
The speaker explores the implications of the computed metric tensor for measuring distances on the surface of a sphere. Using the intrinsic approach, the paragraph demonstrates how to calculate the length of a diagonal path on the sphere, highlighting the difference in length measurements compared to those in the UV plane. The importance of the sine squared of U term in the metric tensor is emphasized, showing how it affects the perceived length of paths at different latitudes on the sphere.
🌌 The Concept of Metric Tensor Fields in Curved Spaces
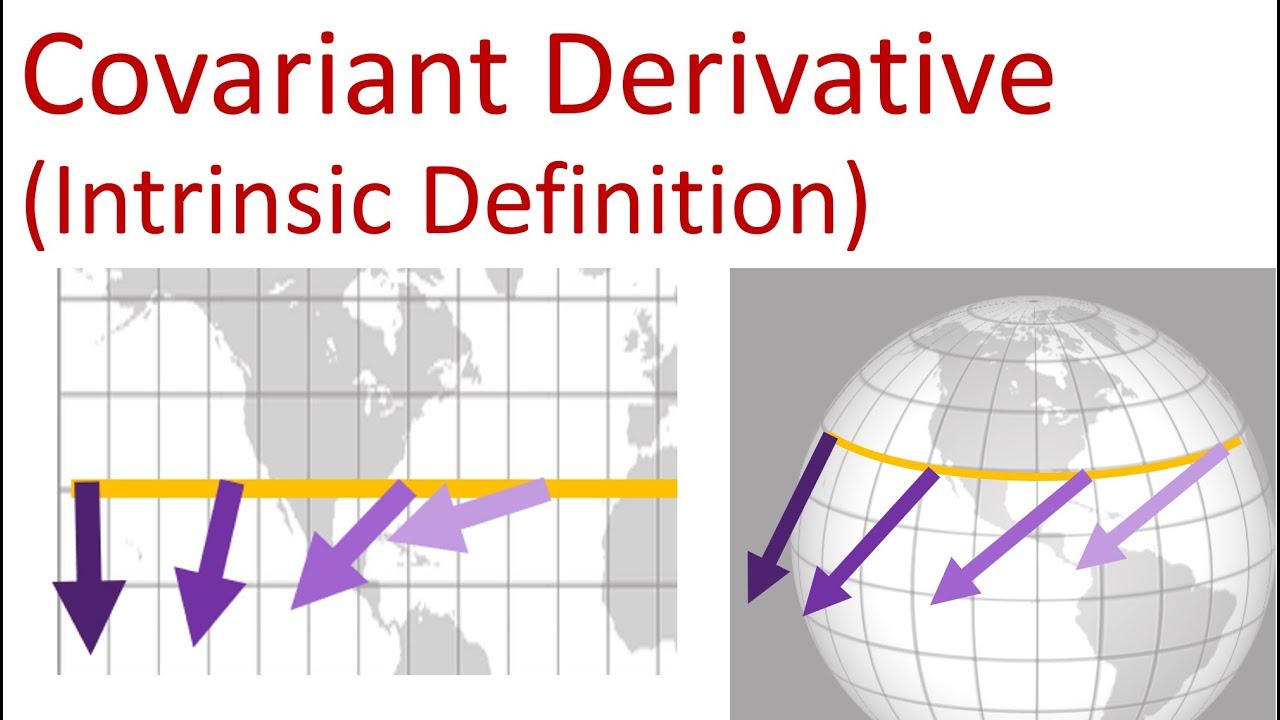
The final paragraph concludes the discussion by emphasizing the importance of the metric tensor field in curved spaces. It explains that every point in a curved space has its own metric tensor, which defines the local rules for measuring distance. The paragraph also touches on the difference between extrinsic and intrinsic views of curved spaces, using the example of the Earth's surface and its distortions in flat maps. The intrinsic geometry is further explained by removing the position vector from the equations and considering the derivative operators as vectors themselves, encapsulating the rules for distance measurement within the curved space.
Mindmap
Keywords
💡Metric Tensor
💡Extrinsic Geometry
💡Intrinsic Geometry
💡Curved Spaces
💡Surfaces
💡UV Coordinates
💡Tangent Vector
💡Arc Length
💡Chain Rule
💡Trigonometric Identities
💡Orthonormal Basis
Highlights
The video discusses calculating lengths of curves in curved spaces using the metric tensor.
It covers the differences between extrinsic and intrinsic geometry.
Recommendation to watch previous videos on the metric tensor for foundational concepts.
Introduction of the metric tensor, also known as the first fundamental form.
Explanation of using the metric tensor to get lengths of vectors and compute curved lengths in flat space.
Examples of curved 2D spaces such as the cylinder, hyperbolic paraboloid, and sphere.
Focus on the sphere for the demonstration of calculating curve lengths.
Describing a 2D surface mathematically by transforming the flat 2D plane with UV coordinates.
The process of mapping the UV plane onto a sphere using transformation equations.
Visual representation of how the UV plane's coordinates map to lines of longitude and latitude on a sphere.
Using a path in the UV plane and transforming it into a path on the spherical surface.
The key to getting lengths of curves is using the magnitude of the tangent vector.
Demonstration of the formula for the squared magnitude of the tangent vector in XYZ space.
Explanation of the simplification due to the orthonormal nature of the XYZ basis vectors.
Calculation of the arc length using the integral of the tangent vector's magnitude.
Difference between extrinsic geometry, analyzing the surface in the surrounding 3D space, and intrinsic geometry, focusing solely on the surface itself.
Introduction to intrinsic geometry, analyzing the surface without reference to the surrounding space.
Computation of the metric tensor components for the sphere using the UV coordinates.
Illustration of how the metric tensor field changes from point to point in curved space.
The importance of the metric tensor in measuring distances in curved spaces accurately.
Practical example of how maps of the Earth distort sizes of countries due to the curvature of the Earth's surface.
Summary of the two approaches to studying curved spaces: extrinsic and intrinsic geometry.
Final takeaway emphasizing the role of the metric tensor field in curved spaces for distance measurement.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: