HARD last problem from the F=ma exam
TLDRThis script explores a physics problem involving a car turning left on a circular track with a cylindrical beaker of water inside. It examines the forces and accelerations acting on a droplet of water, including centripetal acceleration and gravity, resulting in a diagonal total acceleration. Using trigonometry and Bernoulli's equation, the script derives the speed at which water escapes from a hole in the beaker based on the water's height and the car's motion. The problem is a stimulating challenge for those interested in physics and problem-solving, with a direct application of fundamental principles to a real-world scenario.
Takeaways
- 🚗 The problem involves a car turning left on a circular track with a constant speed, carrying a cylindrical beaker with a small hole.
- 🔄 The water in the beaker experiences centripetal acceleration towards the center of the circular path and gravitational acceleration downwards.
- 📐 The total acceleration of the water droplet can be decomposed into horizontal (centripetal) and vertical (gravity) components.
- 📏 The angle between the resultant acceleration and the vertical is labeled as theta, and trigonometric functions are used to relate the components.
- 🧩 The effective height of the water, h', is reduced due to the car's turn, and it is given by h multiplied by the cosine of theta.
- 💧 The pressure at a height h in the water is affected by the resultant acceleration and is not simply the product of density, gravity, and height.
- 🌀 Bernoulli's equation is applied to relate the potential and kinetic energy of the fluid, considering the car's turning motion.
- ⚖️ The potential energy due to the height of the water is equated to the kinetic energy of the escaping water, allowing for the calculation of the water's exit speed.
- 🔢 The speed of the water escaping through the hole, denoted as 'u', is derived to be the square root of 2 times the gravitational acceleration times the effective height.
- 🏆 The problem is presented as a challenging physics problem, suitable for those interested in problem-solving and physics competitions.
- 🌐 The video also promotes another problem from the International Physics Olympiad related to the expansion of the universe.
Q & A
What is the main problem discussed in the script?
-The script discusses a physics problem involving a car turning left along a circular track with a cylindrical beaker inside it, and the problem is to determine the speed at which water escapes from a small hole at the highest point of the water in the beaker.
What are the two accelerations experienced by a droplet of water in the beaker?
-The droplet of water experiences centripetal acceleration, which is \( v^2 / r \), and gravitational acceleration, which is \( g \), directed vertically downwards.
How is the total acceleration of the water droplet represented in vector notation?
-The total acceleration is represented as \( a_{total} = \frac{v^2}{r} \hat{i} + g \hat{j} \), where \( \hat{i} \) and \( \hat{j} \) are unit vectors in the x and y directions, respectively.
What is the relationship between the angle theta and the accelerations?
-The angle theta is used to describe the direction of the total acceleration relative to the gravitational acceleration. The components of the total acceleration can be expressed using trigonometric functions of theta: \( g = a_t \cos(\theta) \) and \( \frac{v^2}{r} = a_t \sin(\theta) \).
How does the height of the water affect the effective height of the water in the beaker?
-The effective height of the water, \( h' \), is affected by the angle theta and is given by \( h' = h \cos(\theta) \), where h is the actual height above the hole.
What formula is used to calculate the pressure at a height h in the beaker?
-The pressure at a height h is typically given by \( P = \rho g h \), where \( \rho \) is the density of the fluid, \( g \) is the acceleration due to gravity, and h is the height.
How does the resultant acceleration affect the pressure calculation?
-The resultant acceleration changes the effective height used in the pressure calculation, which is \( h' = h \cos(\theta) \), and the pressure formula becomes \( P = \rho a_t h' \), where \( a_t = g / \cos(\theta) \).
What principle is used to relate the potential and kinetic energies in the context of the water escaping from the hole?
-Bernoulli's principle is used to relate the potential and kinetic energies, stating that the total energy per unit volume of a fluid remains constant along a streamline.
How can the speed of the escaping water be determined using Bernoulli's equation?
-By setting the potential energy \( \rho g h \) equal to the kinetic energy \( \frac{1}{2} \rho u^2 \), and solving for \( u \), we find that \( u = \sqrt{2gh} \), which is the speed of the escaping water.
What is the significance of the angle theta in the context of the problem?
-The angle theta is crucial as it determines the effective height of the water and the direction of the resultant acceleration, which in turn affects the pressure and the speed of the escaping water.
What is the final answer to the problem presented in the script?
-The final answer to the problem is that the speed of the water escaping from the hole is \( u = \sqrt{2gh} \), where \( g \) is the acceleration due to gravity and \( h \) is the height above the hole.
Outlines
🚗 Physics of Water in a Turning Car
The first paragraph of the script presents a physics problem involving a car turning left on a circular track with a cylindrical beaker inside. The beaker has a small hole, and the challenge is to determine the speed at which water escapes from the hole when the highest point in the beaker is at a height 'h' above it. The explanation involves understanding the centripetal acceleration (v^2/r) and gravitational acceleration (g), and how they combine to create a resultant acceleration. The script uses trigonometry to relate the angle of the car's turn (θ) to the effective height of the water (h' = h * cos(θ)) and the pressure at that height. It then applies Bernoulli's equation to equate potential and kinetic energy, leading to the formula for the escape speed of the water (u = sqrt(2gh)). The problem is a classic example of applying physics principles to a real-world scenario.
🌌 International Physics Olympiad: Universe Expansion
The second paragraph briefly mentions a problem from the International Physics Olympiad concerning the expansion of the universe. However, the details of the problem are not provided in the script. The mention of the Olympiad suggests that the problem likely involves complex concepts in astrophysics or cosmology, possibly related to the rate of expansion, the cosmic microwave background, or dark energy. The script indicates that the video on this topic will conclude with this problem, implying that it is a challenging and thought-provoking subject for viewers interested in advanced physics.
Mindmap
Keywords
💡Centripetal Acceleration
💡Gravity (g)
💡Resultant Acceleration
💡Vector Notation
💡Angle Theta (θ)
💡Trigonometry
💡Effective Height (h')
💡Pressure
💡Bernoulli's Equation
💡Kinetic Energy
Highlights
A car turns left along a circular track with a cylindrical beaker inside, creating a physics problem involving centripetal acceleration and gravity.
Centripetal acceleration is calculated as v^2/r, causing the car to turn left.
Gravity causes a vertical downward acceleration, labeled as 'g'.
The total acceleration is a vector sum resulting in a diagonal direction.
Acceleration components are separated into x and y directions, represented by i_hat and j_hat.
Angle theta is introduced for trigonometric analysis of the resulting forces.
Cosine of theta relates to the vertical acceleration component compared to the total acceleration.
Sine of theta is used to express the horizontal component of acceleration.
The water's effective height, h_prime, is calculated using trigonometric functions.
Pressure at height h is derived from the standard formula, adjusted for the effective height.
Bernoulli's equation is applied to relate potential and kinetic energy in fluid dynamics.
The speed of the escaping water, 'u', is determined using Bernoulli's equation.
The final answer for the water's escape speed is derived as the square root of 2 times gh.
The problem is from the American F=ma exam, indicating its complexity and educational value.
The video encourages viewers to explore more challenging physics problems, such as the International Physics Olympiad.
A related problem on the expansion of the universe is suggested for those interested in hard problem-solving.
Transcripts
Browse More Related Video
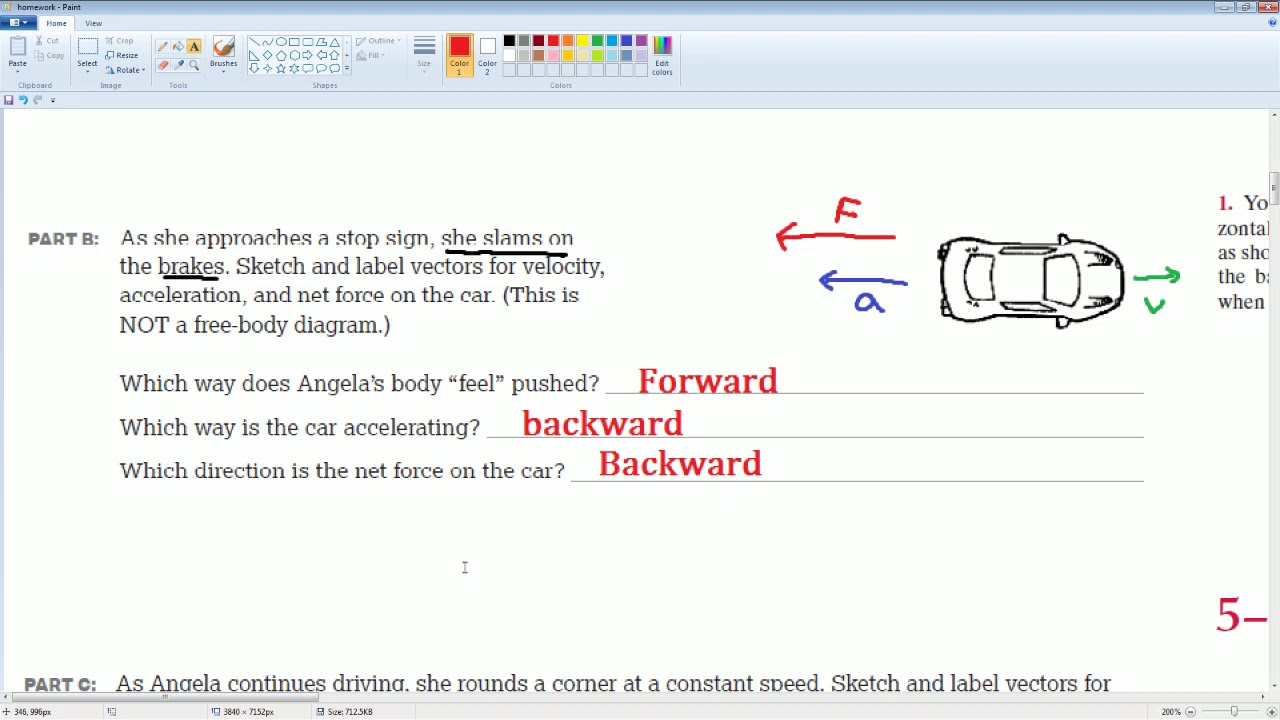
AP Physics Workbook 3.A Inertia and Acceleration

High School Physics - Centripetal Force
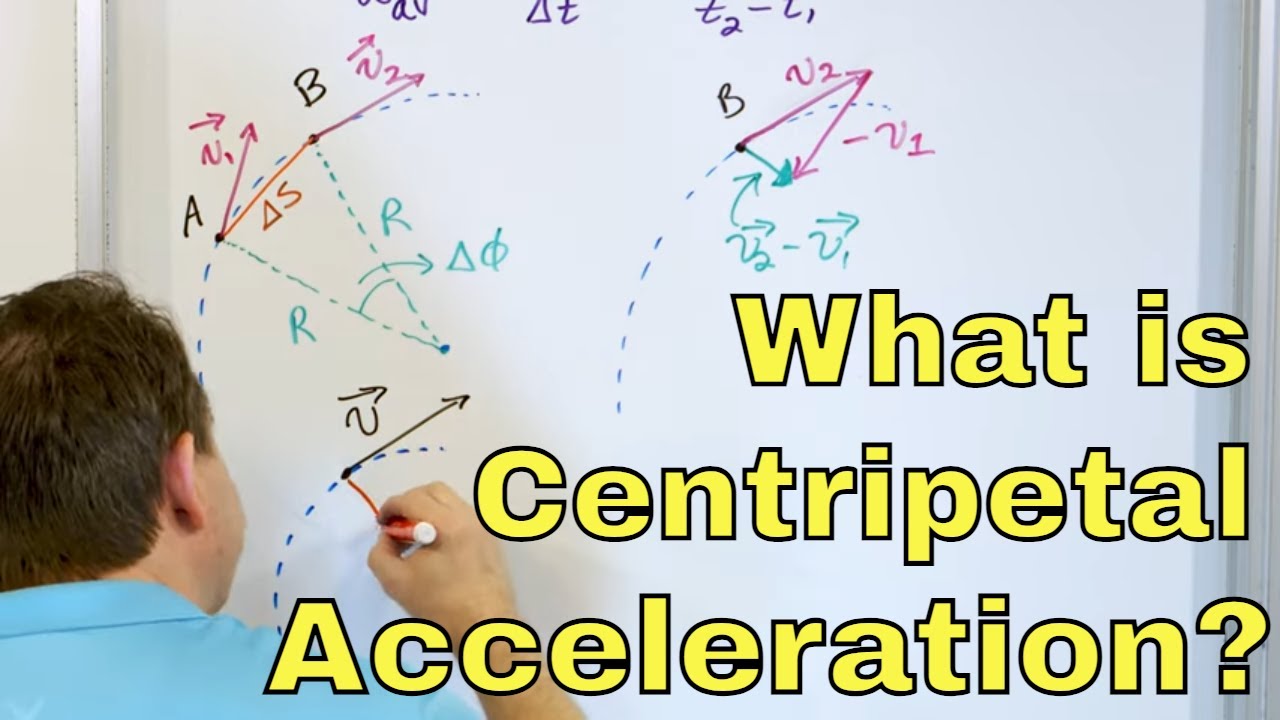
What is Circular Motion & Centripetal Acceleration in Physics? - [1-4-14]

AP Physics Workbook 3.F Horizontal Circles
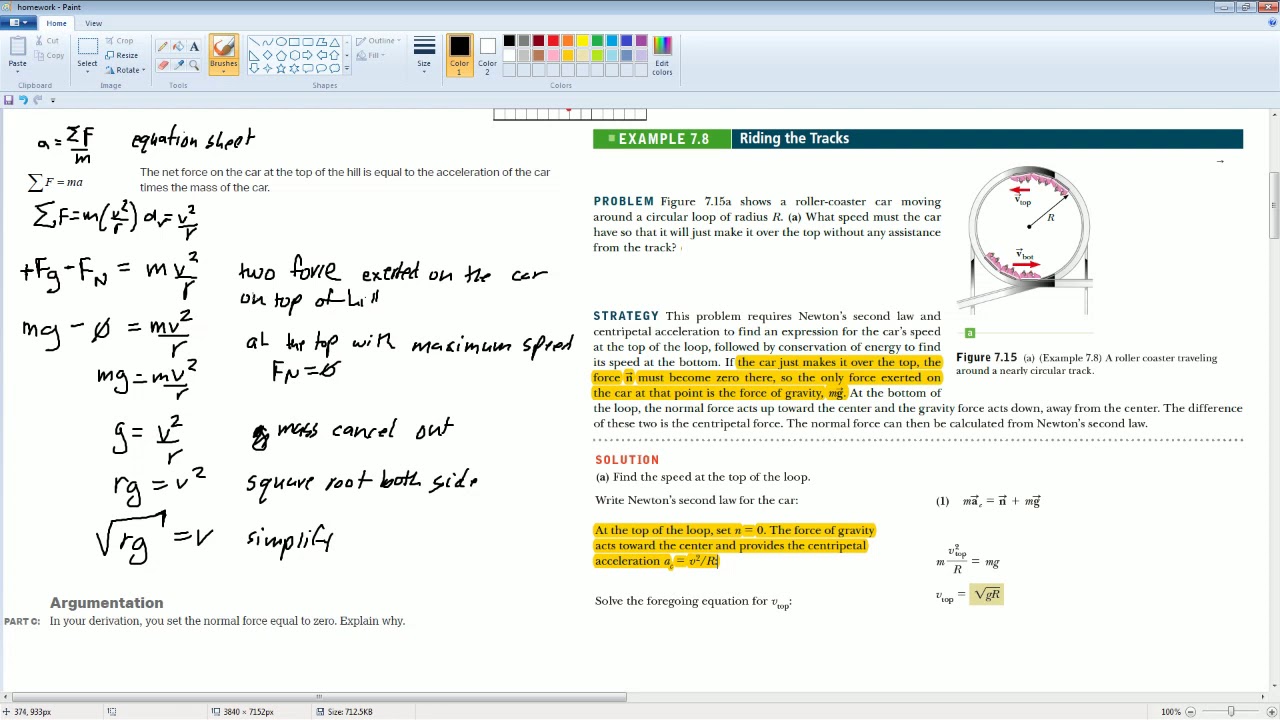
AP Physics Workbook 3.E Maximum speed over the top

2018 #1 Free Response Question - AP Physics 1 - Exam Solution
5.0 / 5 (0 votes)
Thanks for rating: