Transverse Waves Grade 10

Kevinmathscience

31 Jul 202324:08

EducationalLearning

32 Likes 10 Comments

TLDRThe video script explains the concept of waves, differentiating between pulses and waves, and focusing on transverse waves. It describes how particles move perpendicularly to the direction of wave propagation, using examples like the Mexican wave to illustrate. The lesson continues with definitions of amplitude, crest, trough, and wavelength. It clarifies the relationship between frequency and period, and how they are inversely related. The script concludes with calculations involving wave speed, frequency, wavelength, and the formula V = f * Lambda, reinforcing the concepts with practical examples.

Takeaways

💥 A transverse wave consists of a series of pulses moving together, with particles moving perpendicular to the direction of the wave's travel.
🔬 The Mexican wave is a real-world example of a transverse wave, where people move up and down while the wave travels horizontally around the stadium.
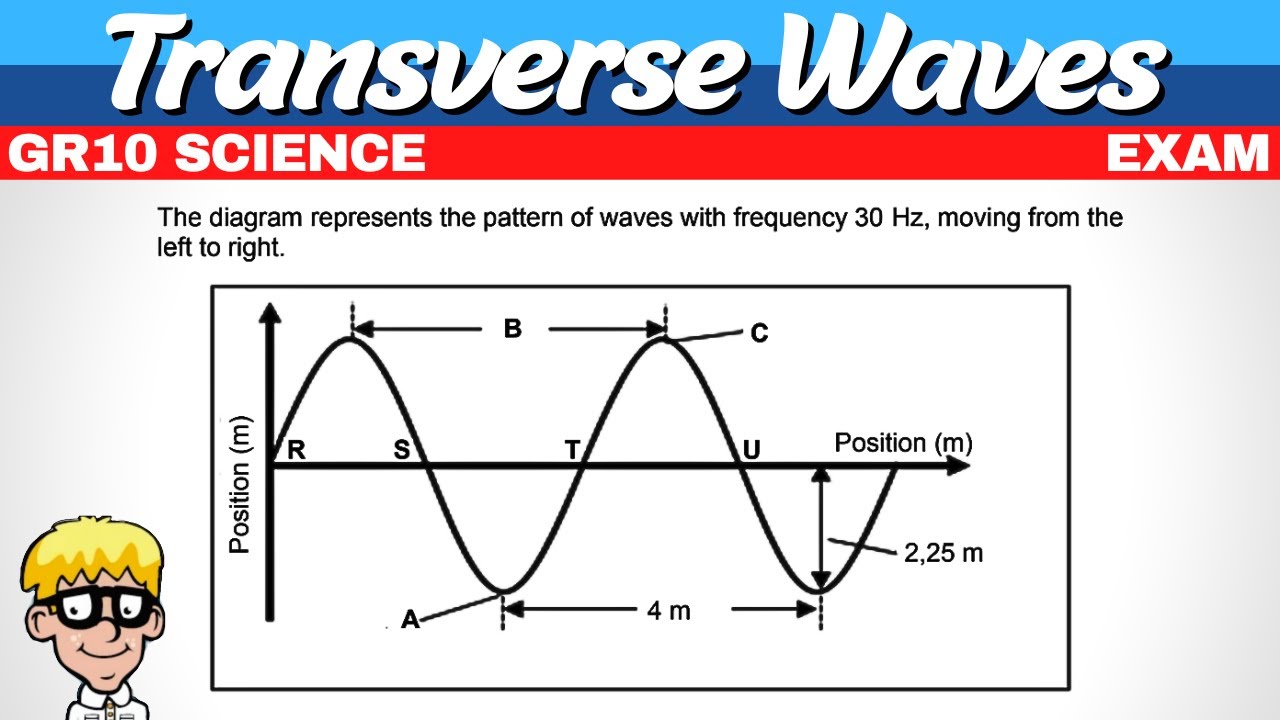
🔢 Amplitude is the distance from the middle line of a wave to its crest or trough, not the total vertical distance from crest to trough.
🚨 The crest is the highest point of a wave, and the trough is the lowest point.
📌 Wavelength is the distance between two consecutive points that are in phase (moving in the same direction and manner) on a wave.
⏳ Frequency is the number of waves that pass a point in one second, measured in Hertz (Hz), while period is the time it takes for one wave to pass, measured in seconds.
💡 Frequency and period are inversely related: frequency = 1/period and vice versa.
🚀 The speed of a wave can be calculated using the formula V = frequency (f) x wavelength (λ), integrating concepts of distance, speed, and time.
✅ Wave speed (velocity) can also be derived from the distance traveled by the wave divided by the time taken, applicable for calculating distances and times in wave motion.
🕖 Calculating wave properties involves understanding and applying formulas for frequency, period, wavelength, and wave speed, tailored to the given information about the wave.

Q & A

What is the difference between a pulse and a wave?
-A pulse is a single disturbance, whereas a wave is formed by a series of pulses. When many pulses come together, they create a wave.
What type of wave is being discussed in the script?
-The script discusses a transverse wave, where the particles move perpendicularly to the direction of the wave's travel.
How can you identify the direction of a transverse wave's movement?
-In a transverse wave, the particles move up and down, while the wave itself moves horizontally, creating a 90-degree angle between the particle motion and the wave direction.
What is an example of a transverse wave mentioned in the script?
-A Mexican wave at a sports event is an example of a transverse wave. The people in the crowd move up and down, while the wave moves around the stadium horizontally.
What is amplitude in the context of waves?
-Amplitude refers to the maximum distance from the middle line (equilibrium position) to either the crest (highest point) or the trough (lowest point) of a wave.
What are the crest and trough of a wave?
-The crest is the highest point of a wave, and the trough is the lowest point. These are the two extremities of the wave's oscillation.
How is wavelength defined in the script?
-Wavelength is the distance between any two points in the same phase of the wave, meaning two points that are doing the same thing at the same time.
What is the relationship between frequency and period in wave properties?
-Frequency is the number of waves that pass a point per second, measured in Hertz (Hz), while the period is the time it takes for one wave to pass a point, measured in seconds. They are inversely related, with frequency being the reciprocal of the period and vice versa.
What is the formula for wave speed and how is it derived?
-The formula for wave speed is V = F × Lambda, where V is the velocity of the wave, F is the frequency, and Lambda (λ) is the wavelength. This formula is derived from the basic distance-speed-time relationship, where speed equals distance over time, and for waves, distance is represented by the wavelength and time by the period.
How can you calculate the frequency of a wave if you know the period?
-If you know the period (T) of a wave, you can calculate the frequency (F) by taking the reciprocal of the period, which is F = 1/T.
What is the significance of understanding the relationship between wavelength, frequency, and wave speed?
-Understanding the relationship between wavelength, frequency, and wave speed is crucial for analyzing and manipulating wave properties in various applications, such as telecommunications, physics experiments, and engineering projects.

Outlines

00:00

🌊 Introduction to Waves

This section covers the transition from understanding a single pulse to comprehending waves as a series of pulses. It introduces the concept of a transverse wave by using an example of observing a green particle's motion, which moves up and down while the wave itself progresses horizontally. This perpendicular movement of particles to the wave's direction defines a transverse wave. An analogy is drawn with a Mexican wave at a sports event to visually illustrate how individuals (particles) move up and down, while the wave travels sideways around the stadium. This serves as a foundational understanding of what transverse waves are and how they differ from the motion of individual particles within the wave.

05:00

📐 Understanding Wave Properties

This segment delves into the fundamental properties of waves, introducing terms like crest (the top of a wave), trough (the bottom of a wave), wavelength (the distance between two similar points on consecutive waves), and phase (the alignment of points in the wave). It explains that wavelength is measured between points that are in 'phase' or aligned in their wave pattern, emphasizing the importance of selecting points doing the same thing (either both ascending or descending) for accurate measurement. This provides a crucial understanding of how to distinguish between different parts of a wave and how to measure its characteristics accurately.

10:03

⏳ Frequency and Period in Waves

Focusing on the concepts of frequency and period, this section explains frequency as the number of waves passing a point per second (measured in Hertz) and period as the time it takes for one wave to pass (measured in seconds). The inverse relationship between frequency and period is highlighted, showing how they are mathematical opposites (frequency is 1 over the period and vice versa). This fundamental relationship underpins much of wave theory and sets the stage for understanding more complex wave behavior and properties.

15:05

🔢 Calculating Wave Speed

This part transitions from theoretical concepts to practical applications, showing how to calculate the speed of a wave. It revisits basic formulas for distance, speed, and time, and relates them to wave properties by replacing distance with wavelength and time with period. The derivation of the formula V = F * Lambda (where V is velocity, F is frequency, and Lambda is wavelength) is explained, emphasizing its utility in calculating the speed of a wave. This section serves as a bridge between understanding wave properties and applying this knowledge to solve problems and make calculations regarding wave dynamics.

20:06

🔍 Practical Examples and Calculations

The final section offers practical exercises to apply the previously discussed theories and formulas, including calculating wave speed, frequency, and wavelength under various conditions. It presents several problems ranging from calculating the speed of a wave given its frequency and wavelength, to determining how far a wave will travel in a given time. This hands-on approach solidifies the understanding of wave properties and calculations, ensuring a comprehensive grasp of wave dynamics and how to manipulate and understand various wave-related formulas.

Mindmap

Keywords

💡Pulse

A pulse is a single disturbance or a burst of energy, as mentioned in the script when discussing the initial concept before waves are introduced. It is a fundamental concept that leads to the understanding of waves, as multiple pulses combined result in a wave. In the script, the pulse is used to explain the basic movement seen in a wave, with an example of a single pulse making one noticeable disturbance.

💡Wave

A wave is a series of disturbances that transfer energy through a medium, as explained in the script when it describes what happens when multiple pulses come together. Waves can be transverse or longitudinal, with the video focusing on transverse waves where particles move perpendicular to the direction of the wave's travel. The concept of a wave is central to the video's theme of exploring how energy moves through different systems.

💡Transverse Wave

A transverse wave is a type of wave where the motion of the particles in the medium is perpendicular to the direction of the wave's propagation, as explained when the script introduces the concept of particles moving up and down while the wave itself moves horizontally. This is a key concept in the video, as it differentiates transverse from longitudinal waves and helps to establish the basic principles of wave behavior.

💡Amplitude

Amplitude refers to the maximum displacement of a particle from its equilibrium position within a wave cycle, as described in the script when it talks about the distance from the middle line to the top or bottom of a wave. Amplitude is a measure of the energy or intensity of a wave, with higher amplitudes indicating more energy. It is a crucial property of waves that affects how they interact with their environment and how they are perceived.

💡Crest

The crest of a wave is the highest point in the wave cycle, as mentioned in the script when it describes the top part of the wave. It represents the point where the wave has the greatest displacement from the equilibrium position. The concept of crest is important for understanding wave dynamics and how waves transfer energy through their peaks and troughs.

💡Trough

A trough is the lowest point in a wave cycle, as explained in the script when it describes the bottom part of the wave. It is the point where the wave is the furthest from the equilibrium position in the downward direction. Understanding the concept of troughs is essential for analyzing the complete cycle of wave motion and the transfer of energy.

💡Wavelength

Wavelength is the distance between two points in a wave that are in the same phase, as defined in the script when it explains the distance between two identical parts of the wave. It is a critical property of waves that helps in characterizing the wave, including its frequency and speed. Wavelength is essential for understanding wave behavior and for making calculations related to wave motion.

💡Frequency

Frequency is the number of complete wave cycles that pass a given point in a unit of time, typically measured in Hertz (Hz), as explained in the script when it discusses the number of waves per second. It is a fundamental property of waves that is inversely related to the period of the wave. Understanding frequency is crucial for analyzing wave patterns and for calculating wave speed.

💡Period

The period of a wave is the duration of one complete wave cycle, measured in seconds, as described in the script when it talks about the number of seconds per wave. It is closely related to frequency, with the period being the inverse of the frequency. The period is a key concept for understanding the temporal aspects of wave motion and for making calculations related to wave properties.

💡Velocity

In the context of waves, velocity refers to the speed at which a wave propagates through a medium, as introduced in the script when discussing the formula for wave speed. It is determined by the product of the wave's frequency and its wavelength, expressed as V = f × λ. Understanding wave velocity is essential for analyzing the behavior of waves and for calculating other wave properties.

💡Wave Properties

Wave properties are the characteristics that define and describe the behavior of waves, including amplitude, wavelength, frequency, and velocity, as discussed throughout the script. These properties are essential for understanding how waves function and interact with their environment. The script delves into each property, explaining their significance and how they relate to one another in the context of wave behavior.

Highlights

The lesson introduces the concept of a pulse and explains how multiple pulses create a wave.

A transverse wave is defined by particles moving perpendicularly to the direction of wave propagation.

The Mexican wave example demonstrates the concept of transverse waves in a real-world scenario.

Amplitude is the distance from the middle line to the top or bottom of a wave, representing the maximum displacement of particles.

The crest and trough are respectively the highest and lowest points of a wave.

Wavelength is the distance between two points in the same phase of a wave, representing one complete cycle.

Frequency and period are inversely related, with frequency being the number of waves per second and period being the number of seconds per wave.

The formula for wave speed is V = f * Lambda, where V is velocity, f is frequency, and Lambda is wavelength.

The lesson provides practical examples and exercises to help understand the concepts of wave properties, such as amplitude, wavelength, frequency, and period.

The importance of understanding the difference between wavelength and the number of waves is emphasized to avoid common misconceptions.

The lesson explains how to calculate frequency and period based on given wave data, enhancing the understanding of wave properties.

A detailed breakdown of how to calculate wave speed using the formula V = f * Lambda is provided, with step-by-step examples.

The lesson covers the basic formulas for distance, speed, and time, and relates them to wave properties for a comprehensive understanding.

The concept of phase is introduced, explaining how it relates to the measurement of wavelength and the synchronization of wave points.

The lesson concludes with a series of practice problems that apply the learned concepts to calculate various wave properties.

The use of visual aids, such as the green particle and the Mexican wave video, enhances the learner's comprehension of wave dynamics.

The lesson emphasizes the importance of precision in calculations and the understanding of wave properties for accurate scientific analysis.

Transcripts

Browse More Related Video

Exam Transverse Waves Grade 10

Anatomy of a Wave

Transverse and Longitudinal Waves

Waves 1: Wave Characteristics

Transverse Waves on a String Problems

Wave Speed Practice Problems v2

Related Tags

Wave Mechanics Transverse Waves Amplitude Wavelength Frequency Velocity Mexican Wave Physics Education Wave Properties STEM Learning