Physics - Optics: Lenses (5 of 5) Lens Combinations - Converging Lens & Mirror

Michel van Biezen
1 May 201307:18
EducationalLearning
32 Likes 10 Comments

TLDRThis script explains the process of analyzing a lens-mirror optical system. It begins by detailing how rays of light from an object pass through a converging lens to form an image. This image then acts as an object for a mirror, which forms a second image. The script includes calculations for image distances and magnifications at each stage, using the lens formula and mirror formula. It concludes with the total magnification of the system, illustrating how a lens-mirror combination can produce inverted and real images with varying sizes.

Takeaways
  • 🔍 The video demonstrates a combination of a mirror and a converging lens to form images.
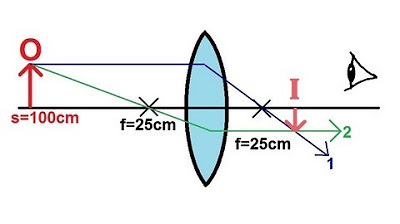
  • 🔭 When an object is placed in front of the converging lens, rays pass through it, converge, and form an image.
  • 🔄 The mirror reflects the converged rays, forming a second image.
  • 📏 To analyze the lens and mirror combination, first, the image caused by the lens is formed by ignoring the mirror.
  • 🔧 The focal length of the converging lens and the concave mirror are both positive.
  • 📐 The first image distance (S1') is calculated using the lens formula: S1' = S1 * F1 / (S1 - F1).
  • 🖼️ The magnification of the first image (M1) is negative, indicating the image is inverted and 2/3 the size of the object.
  • 🔄 The first image acts as the object for the mirror, with its distance adjusted by subtracting the lens-mirror separation.
  • 🔁 The second image distance (S2') is found using the mirror formula: S2' = S2 * F2 / (S2 - F2).
  • ⚖️ The second image is real, inverted, and formed in front of the mirror with a magnification of 0.56.
  • 📉 The total magnification (M_total) of the second image relative to the original object is -0.38.
Q & A

  • What is the main concept discussed in the video script?

    -The main concept discussed in the video script is the process of finding the image formed by a combination of a lens and a mirror, including the calculations for image distances and magnifications.

  • What type of lens is being discussed in the script?

    -The script discusses a converging lens, which is also known as a convex lens.

  • What is the role of the mirror in the lens-mirror combination?

    -The mirror in the lens-mirror combination reflects the rays that have passed through the lens to form a second image.

  • How is the image formed by the first lens found?

    -The image formed by the first lens is found by drawing rays from the object, which pass through the lens and converge to form an image on the other side.

  • What is the significance of the positive image distance (S1') found relative to the lens?

    -A positive image distance (S1') indicates that the image formed by the lens is a real image, meaning it is located on the same side of the lens as the light source.

  • What is the formula used to calculate the image distance (S1') formed by the first lens?

    -The formula used to calculate the image distance (S1') is S1' = S1 * F1 / (S1 - F1), where S1 is the object distance, and F1 is the focal length of the lens.

  • What does the negative magnification (M1) signify?

    -A negative magnification (M1) signifies that the image formed by the lens is inverted relative to the original object.

  • How is the object distance for the mirror calculated in the lens-mirror setup?

    -The object distance for the mirror is calculated by taking the distance from the first image to the mirror and subtracting the distance between the lens and the mirror.

  • What is the formula used to calculate the image distance (S2') formed by the mirror?

    -The formula used to calculate the image distance (S2') is S2' = S2 * F2 / (S2 - F2), where S2 is the object distance relative to the mirror, and F2 is the focal length of the mirror.

  • What does a positive image distance (S2') in relation to the mirror indicate?

    -A positive image distance (S2') in relation to the mirror indicates that the image is formed in front of the mirror, which is a virtual image in the context of the mirror.

  • How is the total magnification of the lens-mirror combination calculated?

    -The total magnification of the lens-mirror combination is calculated by multiplying the magnifications of the individual lens (M1) and mirror (M2).

  • What does the final total magnification (M total) represent?

    -The final total magnification (M total) represents the ratio of the size of the final image to the size of the original object, taking into account the inversions and scalings that occur through the lens-mirror system.

Outlines
00:00
🔍 Lens and Mirror Optics Combination

This paragraph introduces the concept of combining a converging lens with a mirror to form two distinct images. The process begins with the lens creating an image, which is then used as an object for the mirror to form a second image. The focal lengths of both the lens and the mirror are positive, indicating they both converge light. The explanation includes the calculation of image positions and magnifications using lens and mirror equations, resulting in a real, inverted image relative to the lens and a virtual, upright image in front of the mirror.

05:02
📐 Calculating Image Properties in a Lens-Mirror Setup

The second paragraph delves into the specifics of calculating the properties of the second image formed by the mirror in the lens-mirror combination. It explains how to determine the image distance and magnification using the mirror's focal length and the distance of the first image from the mirror. The calculation reveals a positive image distance, indicating a virtual image in front of the mirror, and a magnification that suggests the image is inverted but maintains the same orientation as the object causing it. The total magnification of the system is found by multiplying the magnifications of the lens and mirror, resulting in a significantly reduced and inverted final image relative to the original object.

Mindmap
Keywords
💡Mirror
A mirror is an object with a reflective surface that bounces light to form images. In the video, the mirror is a key component in the lens-mirror combination, used to reflect the light rays after they pass through the lens, creating a secondary image. The mirror mentioned is a converging mirror, which has a positive focal length and is used to focus the light rays to a point.
💡Lens
A lens is a transmissive optical device that focuses or disperses light. The video describes a converging lens, which is designed to bring light rays together at a focal point. The lens is crucial in the process of forming the first image before the mirror's involvement.
💡Converging Lens
A converging lens is a type of lens that bends incoming parallel light rays toward a common focal point. In the script, the converging lens is used to form an image of the object, which is then used as the object for the mirror to create a second image.
💡Focal Length
Focal length is the distance over which light rays parallel to the optical axis converge or from which they appear to diverge after passing through a lens or mirror. The script mentions the focal lengths of both the lens and the mirror, which are essential for calculating the positions of the images formed.
💡Image Formation
Image formation is the process by which an optical system like a lens or mirror creates an image of an object. The video script describes the process of forming an image with a lens, then using that image as the object for the mirror to form a second image.
💡Magnification
Magnification is the ratio of the size of the image to the size of the object, indicating how much larger or smaller the image appears compared to the original. The script calculates the magnification after the lens and then after the mirror to understand the size and orientation of the images formed.
💡Real Image
A real image is formed where light rays actually converge and can be projected onto a screen. The script mentions that the image formed by the lens is a real image, as it is located at a positive distance from the lens and can be used as an object for the mirror.
💡Inverted Image
An inverted image is one where the top and bottom of the object are reversed in the image. The script explains that the image formed by the lens is inverted and that this inversion is maintained when forming the second image with the mirror.
💡Ray Tracing
Ray tracing is a method used to predict the path of light through an optical system by drawing representative rays. The video script uses ray tracing to illustrate how light rays pass through the lens and are reflected by the mirror to form images.
💡Positive and Negative Magnification
Positive magnification indicates the image is larger than the object and is upright, while negative magnification means the image is smaller and inverted. The script calculates the magnification after the lens as negative, indicating an inverted and reduced-size image.
💡Total Magnification
Total magnification is the overall change in size from the original object to the final image through an optical system. The script calculates the total magnification by multiplying the magnifications from the lens and the mirror to find the final image size relative to the original object.
Highlights

Introduction to a lens and mirror optical system setup with a converging lens and mirror.

Explanation of how light rays pass through a converging lens and then reflect off a mirror to form images.

Methodology for analyzing lens and mirror combinations similar to lens-lens combinations.

Description of the positive focal length of both the converging lens and mirror.

Procedure for drawing rays to determine the first image formed by the lens.

Use of lens equation to calculate the image distance (S1') for the first lens.

Calculation of the first image's position at 33.3 cm from the lens.

Determination of the first image as a real, inverted image that is smaller than the object.

Process of finding the second image caused by the mirror using the first image as the new object.

Calculation of the distance from the first image to the mirror and subsequently to the mirror's focal point.

Use of mirror equation to find the second image distance (S2') with the mirror.

Result of the second image being in front of the mirror at a positive 13.1 cm distance.

M2 magnification calculation showing the second image is larger and upright compared to the first image.

Conclusion that the second image is inverted and maintains the same orientation as the object causing it.

Total magnification calculation combining both the lens and mirror effects.

Final magnification result indicating a significantly reduced image size relative to the original object.

Transcripts

Browse More Related Video

Physics - Optics: Lenses (4 of 5) Lens Combinations - Converging & Diverging Lenses

Physics - Optics: Lenses (2 of 5) Lens Combinations - Two Converging Lenses

Physics - Optics: Lenses (1 of 5) Lens Combinations - Two Converging Lenses

Multiple Two Lens System with Diverging and Converging Lens

GCSE Physics - How to Draw Ray Diagrams #70

Physics - Optics: Lenses (1 of 4) Converging Lens

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
OpticsPhysicsImage FormationLens MirrorConcave MirrorConverging LensRay TracingMagnificationReal ImageInverted Image