Calculus Chapter 3 Lecture 25 Definite Integrals
TLDRIn this calculus lecture, Professor Greist introduces the concept of the definite integral, contrasting it with the indefinite integral. He explains the definite integral as a sum of infinitesimally small quantities, using the example of summing the first 'n' natural numbers and visualizing it as the area of a triangle. The lecture delves into the formal definition of the definite integral, including the Riemann sum and the process of taking a limit as partition widths approach zero. The professor also covers properties of definite integrals, such as linearity, additiveness, and the implications of integrating odd and even functions. The lesson emphasizes the importance of understanding the integral's definition, which is foundational for future calculus concepts.
Takeaways
- 📚 The lecture introduces the concept of the definite integral, contrasting it with the indefinite integral and emphasizing its numerical nature.
- 🔍 The definite integral is defined as a limit of Riemann sums, which are sums of smaller quantities that approximate the integral as the partition widths approach zero.
- 📏 The Riemann sum is visualized as rectangles or columns sitting atop a partition of the function's domain, representing local sums that contribute to the global sum.
- 📈 The definite integral generalizes the process of summing to more complex or nonlinear functions, extending the idea of summing a series like 1+2+3... to n.
- 📉 The integral sign is a form of the letter 'S', indicating a sum, and the notation associated with it mirrors that of the Riemann sum.
- 📐 The limits of integration specify the interval over which the integral is taken, and the variable of integration can be named arbitrarily as it does not affect the value of the integral.
- 📝 An example is provided to demonstrate the computation of a definite integral, showing the process of setting up a uniform partition and taking the limit as the partition width approaches zero.
- 🔢 The properties of definite integrals are discussed, including linearity, scalar multiplication, additivity, and the orientation property which relates to the sign of the integral based on the direction of the interval.
- 📉 The concept of odd and even functions in the context of definite integrals is introduced, explaining how the symmetry of these functions affects the value of the integral over a symmetric interval.
- 📚 The importance of remembering the definition of the definite integral is highlighted, as it is foundational to understanding the computations that will be facilitated by the Fundamental Theorem of Integral Calculus.
- 🔑 The script concludes by emphasizing that while the definition of the definite integral is complex, it is crucial to grasp it before moving on to practical computation methods.
Q & A
What is the main focus of Lecture 25 by Professor Greist?
-Lecture 25 focuses on the definite integral, explaining its definition, meaning, and the process of calculating it as a numerical quantity.
How is the sum of the first n natural numbers represented geometrically in the script?
-The sum of the first n natural numbers is represented geometrically as the area of a triangle with base N and height N, discretized into squares each with side length 1.
What is the formula for the sum of the first n natural numbers, including the leftover triangles?
-The formula for the sum, including the leftover triangles, is 1/2 * n * (n + 1), which accounts for the area of the triangle plus the area of n small triangles each with area 1/2.
What is the Riemann sum and how is it visualized?
-The Riemann sum is a method to approximate the definite integral of a function by summing the product of function values at sample points and the width of the partition elements. It is visualized as columns or rectangles sitting atop the partition.
How is the definite integral defined mathematically?
-The definite integral is defined as the limit of Riemann sums as the partition elements' widths approach zero, representing the process of adding smaller and smaller local amounts to form a global sum.
What is the significance of the notation DX in the context of the definite integral?
-DX represents the differential element, which is the limit of the partition width Delta X as it approaches 0, and it is used to denote the process of taking a limit in the integral.
Why is the choice of the sampling point within each partition element not important?
-The choice of the sampling point is not important because as the partition width approaches zero, the dependence on the specific sampling point becomes less significant, and the integral value converges to the same limit.
What is an example of a definite integral that was computed in the script?
-An example given is the definite integral of X with respect to X from 0 to 1, which was computed using a uniform partition and resulted in the value of 1/2.
What are some properties of definite integrals mentioned in the script?
-Some properties mentioned are linearity, scalar multiplication, additivity, orientation, and dominance. These properties help in understanding how integrals behave under different mathematical operations.
How do odd and even functions affect the definite integral over a symmetric interval?
-For odd functions, the integral over a symmetric interval from negative L to L is always 0 due to symmetry about the origin. For even functions, the integral is twice the integral from 0 to L because of symmetry about the y-axis.
What is the fundamental theorem of integral calculus and its relevance to the definite integral?
-The fundamental theorem of integral calculus relates the definite integral to the process of differentiation. It will be discussed in the next lesson and is crucial for computing definite integrals without directly using the definition.
Outlines
📚 Introduction to Definite Integrals
Professor Greist begins Lecture 25 by transitioning from the concept of the indefinite integral to the definite integral. The definite integral is introduced as a numerical quantity, contrasting with the class of functions represented by the indefinite integral. The professor uses the sum of the first n natural numbers as a classical example to illustrate the concept of summing smaller local amounts into a global sum. This is further explained geometrically by representing each term as a square column, forming a triangle whose area represents the sum. The professor emphasizes the importance of understanding the definite integral as a limit of Riemann sums, which involves partitioning the interval, choosing sampling points, and taking the limit as the partition widths approach zero.
📘 Definite Integral Definition and Notation
In this section, the professor delves into the formal definition of the definite integral, highlighting the integral sign's relation to the concept of summation. The process of defining the definite integral involves setting up a partition of the interval from A to B, choosing sampling points within each partition, and calculating the Riemann sum. The definite integral is then defined as the limit of these Riemann sums as the partition widths approach zero. The professor also discusses the notation used in definite integrals, emphasizing the importance of understanding the limits of integration and the variable of integration. An example is provided to compute the definite integral of x dx from 0 to 1, demonstrating the application of the definition and the process of taking a limit.
📙 Properties of Definite Integrals
The professor discusses several key properties of definite integrals, starting with linearity, which states that the integral of a sum of functions is the sum of the integrals. This property is illustrated through the concept of Riemann sums and is shown to hold true in the limit. The next property is scalar multiplication, where multiplying the integrand by a constant results in the integral of the function being multiplied by the same constant. Additivity is another property, which allows for the combination of integrals over consecutive intervals into a single integral over the entire range. The orientation property is also introduced, which relates to the sign of the integral when the limits of integration are reversed. The final property discussed is dominance, which states that if a function is non-negative, its integral over an interval is also non-negative, with implications for functions where one is greater than the other.
📒 Odd and Even Functions in Definite Integrals
The final paragraph focuses on the behavior of odd and even functions within the context of definite integrals. Odd functions, which are symmetric about the origin, have the property that their integral over a symmetric interval from negative L to L is zero. This is due to the cancellation effect of positive and negative values on either side of the origin. Even functions, which are symmetric about the y-axis, have their integrals doubled over a symmetric interval, as every positive value on one side has a corresponding value on the other side. The professor also touches on the concept of Taylor series for odd and even functions and their implications for integration. The summary concludes with a reminder of the importance of the definition of the definite integral, comparing its significance to that of the derivative, and a teaser for the upcoming lesson on the fundamental theorem of integral calculus.
Mindmap
Keywords
💡Definite Integral
💡Indefinite Integral
💡Riemann Sum
💡Partition
💡Sampling Point
💡Linearity
💡Additivity
💡Orientation
💡Non-negativity
💡Odd and Even Functions
Highlights
Introduction to the concept of definite integrals as a numerical quantity, contrasting with the indefinite integral.
Explanation of how definite integrals are defined and their significance in summing smaller local amounts into a global sum.
Classical example of summing natural numbers represented geometrically as a triangle's area.
The general formula for the sum of the first n natural numbers and its relation to the area of a triangle.
Transition from local addition to the global concept of the definite integral.
Detailed definition of the definite integral involving limits, partitions, and Riemann sums.
Visualization of Riemann sums as columns or rectangles over a partition.
The importance of the integral sign notation and its relation to summation.
Clarification on the limits of integration and the variable used for integration.
Example computation of the definite integral of X with respect to X from 0 to 1.
Demonstration of the difficulty in computing definite integrals using the definition and the introduction of the fundamental theorem of integral calculus.
Properties of definite integrals, including linearity and the effect of scalar multiplication.
Additivity property of definite integrals and its geometric interpretation.
Orientation property of definite integrals and its implications for the integral of a function over a reversed interval.
Dominance property of definite integrals and its relation to the non-negativity of integrals of non-negative functions.
Computation of definite integrals of sine and cosine over symmetric intervals and the concept of odd and even functions.
Differentiation between definite and indefinite integrals and the预告 of the fundamental theorem linking them.
Transcripts
Browse More Related Video

Writing an Integral as a Limit of a Riemann Sum

Math 1325 Lecture 13 2

Lec 18 | MIT 18.01 Single Variable Calculus, Fall 2007

Calculus Chapter 3 Lecture 26 The FTIC

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus
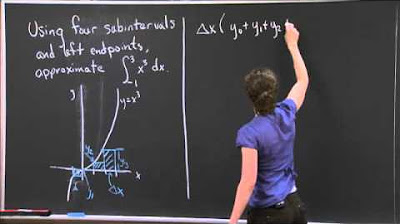
Riemann sum | MIT 18.01SC Single Variable Calculus, Fall 2010
5.0 / 5 (0 votes)
Thanks for rating: