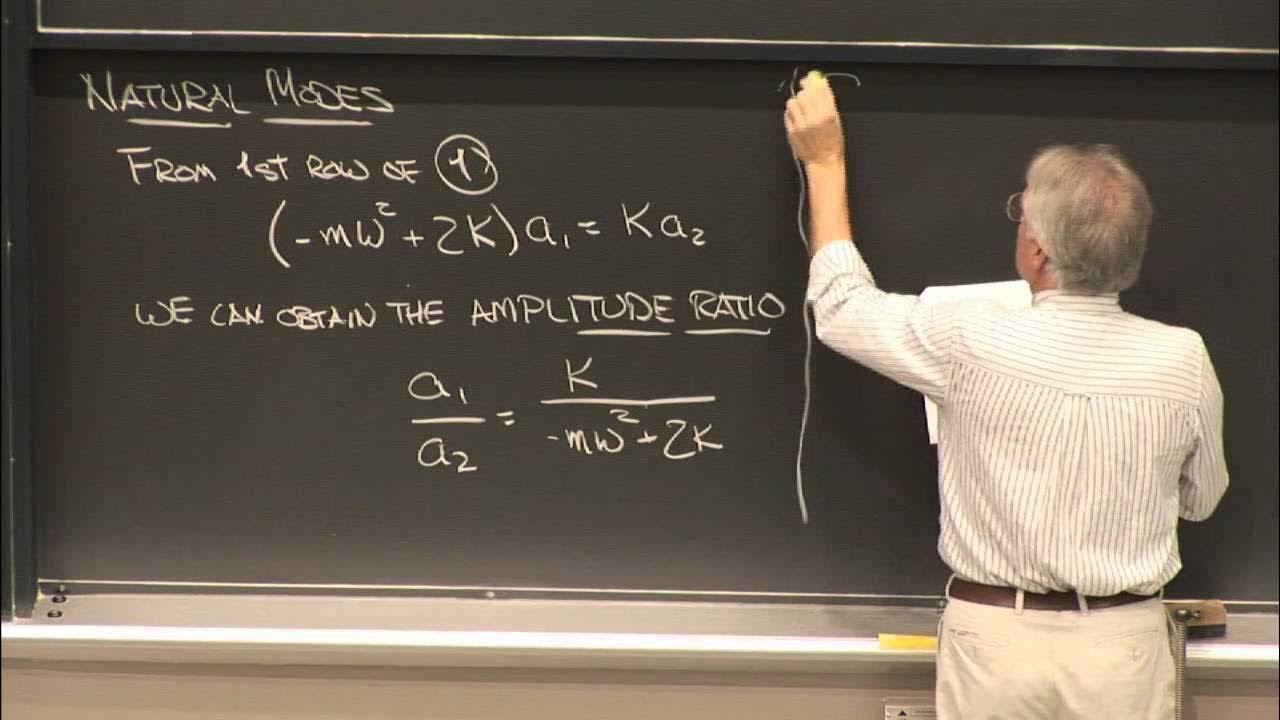Calculus Chapter 3 Lecture 20 BONUS
TLDRIn this lecture, Professor Greist explores the synchronization of coupled oscillators using metronomes on a common rolling platform. An experiment demonstrates near-synchronization but reveals a slight phase gap due to frequency discrepancies. The lecture delves into modeling this phenomenon with differential equations, showing that exact synchronization depends on identical frequencies. The discussion extends to more complex systems involving multiple oscillators and networks, highlighting the broader implications for various scientific fields and suggesting further study in dynamical systems and multivariable calculus.
Takeaways
- 🔄 The lecture discusses the synchronization of coupled oscillators, using metronomes as an example.
- 🎶 A pair of metronomes set to 184 beats per minute were coupled on a rolling platform to observe their phase difference.
- ⏳ The experiment showed a small, persistent phase difference of about 0.06 seconds, contrary to the prediction of complete synchronization.
- 🧮 The model assumed identical frequencies for the oscillators, which may not have been the case, leading to the observed phase difference.
- 📐 The revised model includes different frequencies for the oscillators (a1 and a2) and predicts a stable equilibrium at a non-zero phase.
- 🔄 The equilibrium phase difference depends on the frequency difference and coupling strength.
- ⚖️ If the coupling strength (epsilon) is too weak or the frequency difference is too large, synchronization may not occur.
- 🔍 The study of more complex systems, such as three or more oscillators, could reveal additional phenomena and behaviors.
- 🧠 This model has broader applications in fields like biology, neuroscience, and behavioral science, especially in network analysis.
- 📚 Further study in dynamical systems and multivariable calculus is necessary to understand more complex systems with many variables.
Q & A
What is the main topic of Professor Greist's lecture 20?
-The main topic of the lecture is the analysis of differential equations for a pair of coupled oscillators, with a focus on the phase difference between them and its behavior over time.
What is the purpose of the metronomes in the experiment?
-The metronomes are used to represent the oscillators in the experiment, set to the same frequency to observe if the phase difference between them decreases over time and if they synchronize.
What was the initial assumption made in the model regarding the frequencies of the oscillators?
-The initial assumption was that the frequencies of the two oscillators were identical, which is critical for the linearized solution to predict synchronization.
What was observed in the experiment that contradicted the initial prediction?
-Even after a long time, there was a small but stable gap between the clicks of the two metronomes, indicating they did not synchronize perfectly as predicted.
What might be the explanation for the observed gap in synchronization?
-The explanation could be that the frequencies of the two oscillators were not exactly the same, as they were set by hand and could have slight differences.
How does the model change when considering non-identical frequencies for the oscillators?
-The model is rewritten to include two different frequencies, a1 and a2, and the differential equation for the phase difference is re-derived, leading to a different equilibrium condition.
What is the new equilibrium condition for the phase difference when the frequencies are not identical?
-The new equilibrium condition is given by the expression arc sine of (a2 - a1) / (2 * epsilon), which predicts a phase that is close to but not exactly zero.
What are the implications of a weak coupling strength or a large frequency difference?
-If the coupling strength epsilon is very small or the frequency difference is too large, the system might fail to achieve an equilibrium, resulting in no phase-locking or synchronization.
What other phenomena could be studied by changing parameters in the model?
-By changing parameters, one could study synchronization in systems with more than two oscillators, the influence of different connection patterns, and the emergence of complex behaviors in large networks of agents.
How does the model apply to fields outside of physics, such as biology or neuroscience?
-The model is applicable in any field where a collection of agents is linked by a network, allowing for the study of synchronization and emergent behaviors in biological, neural, or social systems.
What advanced mathematical tools might be needed to study systems with a large number of variables?
-To study systems with thousands of variables, such as large networks of coupled oscillators, one would need multivariable calculus and potentially other advanced mathematical tools.
Outlines
📚 Introduction to Coupled Oscillators and Experiment
Professor Greist introduces the topic of coupled oscillators in calculus, beginning with lecture 20's bonus material. The lecture delves into the differential equations governing the phase difference between two oscillators, initially assumed to synchronize perfectly after linearization. An experiment with two metronomes set to 184 beats per minute is conducted to test this theory. The metronomes are coupled via a rolling platform to transfer impulses between them. The experiment demonstrates a phase difference that seems to decrease, suggesting synchronization. However, upon closer waveform analysis, a small phase gap remains, indicating imperfect synchronization. The professor raises questions about the assumptions made in the model, particularly regarding the identical frequencies of the oscillators, and suggests that discrepancies in the metronome settings could be a contributing factor.
🔍 Revisiting Model Assumptions and Exploring Synchronization Phenomena
The second paragraph revisits the assumptions of the model and explores the implications of non-identical frequencies in the oscillators. The professor modifies the model to accommodate two different frequencies, a1 and a2, and re-derives the differential equation for the phase difference. The analysis reveals that if the frequencies are not identical, the equilibrium phase is not zero but rather a value dependent on the difference in frequencies and the coupling strength epsilon. This adjustment in the model aligns with the experimental observation of a non-zero phase difference. The professor also discusses the limitations of weak coupling and significant frequency differences, which may prevent synchronization altogether. Furthermore, the paragraph raises the possibility of exploring more complex systems with three or more oscillators and the potential for different connection patterns to influence synchronization behavior. The discussion concludes by highlighting the broader applications of this model in various scientific fields and the need for advanced mathematical tools to analyze large networks of interacting agents.
Mindmap
Keywords
💡Calculus
💡Differential Equations
💡Coupled Oscillators
💡Phase Difference
💡Linearization
💡Metronomes
💡Synchronization
💡Equilibrium
💡Frequency
💡Coupling Strength
💡Network of Agents
Highlights
Introduction to the lecture on differential equations for coupled oscillators.
Analysis of phase angle difference between oscillators and its tendency to zero post linearization.
Experimental setup with metronomes to test the theory of phase synchronization.
Observation of phase difference reduction and stability under perturbation.
Waveform analysis revealing a persistent phase gap despite long stabilization time.
Assumption of identical frequencies in the model questioned due to the observed phase gap.
Rewriting the model to account for non-identical frequencies of oscillators.
Derivation of a new differential equation considering different frequencies.
Equilibrium analysis showing phase not necessarily zero with different frequencies.
Explanation of the observed experimental data with the adjusted model.
Discussion on the stability of equilibrium in the context of coupling strength and frequency differences.
Introduction to the concept of phase-locking and synchronization in oscillator systems.
Exploration of the impact of network topology on the behavior of coupled oscillators.
Potential applications of the model in biology, neuroscience, and behavioral science.
The necessity of multivariable calculus for analyzing systems with a large number of variables.
The possibility of equilibrium solutions generating waves in networked systems.
Invitation to study dynamical systems for a deeper understanding of complex networks.
Conclusion emphasizing the foundational role of differential equations in understanding complex systems.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: