Calculus Polar Area Limacon Example
TLDRThis video script explains the concept of calculating polar areas, using a lemon-shaped graph as an example. It emphasizes the importance of understanding the graph's limits and avoiding double counting. The script demonstrates how to find the area of the entire shape by calculating half and then doubling it, as well as how to find the area of the inner loop. It also touches on the common task of finding the area between loops by subtracting the inner loop's area from the total. The key takeaway is that mastering polar graphing is essential for accurately calculating areas in polar coordinates.
Takeaways
- 📚 The topic discussed is polar areas, specifically using a lemon-shaped example to illustrate the concept.
- 📈 The importance of carefully counting the area in polar coordinates is emphasized, especially when dealing with shapes that double back on themselves.
- 📝 It's recommended to spend time learning how to graph in polar coordinates to understand the bounds needed for polar area problems.
- 📊 The script explains how to find the area of a polar region by plotting the limits and identifying key angles where the radius equals zero.
- 🔍 Key angles identified are 0, 2π/3, π, and 4π/3, which are crucial for understanding when to start and stop the inner loop of the polar graph.
- 🔢 The method of finding half the area and then doubling it is introduced as a preferred approach for solving polar area problems.
- ⚖️ The integral from 0 to 2π/3 is used to calculate half the area, avoiding double counting by stopping before the inner loop.
- 🧮 The integral involves squaring the radius (R) and integrating with respect to theta (Dθ), with the result calculated using a calculator.
- 🔄 An alternative approach is mentioned, which involves 'backing up' to negative angles and integrating to avoid double counting.
- 🔍 The area of the inner loop is found by integrating from 2π/3 to 4π/3, using the same method of squaring the radius and integrating with respect to theta.
- 🔑 The area between the loops can be found by subtracting the inner loop area from the total enclosed area, which is a common question in polar area problems.
Q & A
What is the main topic discussed in the transcript?
-The main topic discussed in the transcript is calculating polar areas, specifically using a lemon-shaped example to illustrate the process.
Why is a lemon a good example for a polar area problem?
-A lemon is a good example for a polar area problem because it doubles back on itself, which requires careful consideration when calculating the area to avoid double-counting.
What is the importance of knowing the limits on a polar area graph?
-Knowing the limits on a polar area graph is crucial for determining the bounds of the area to be calculated and for ensuring accurate area measurements.
What are the key angles mentioned in the transcript for the polar area example?
-The key angles mentioned are 0, 2π/3, π, and 4π/3, which are important for identifying when to start and stop calculating the area within the polar graph.
What strategy does the speaker recommend for finding the area of the entire polar region?
-The speaker recommends finding half of the area first and then doubling it, which simplifies the process and helps avoid double-counting.
How does the speaker suggest avoiding double-counting the inner loop area?
-The speaker suggests stopping the area calculation at 2π/3 to avoid entering the inner loop, and then doubling the calculated area to get the total area.
What is the integral expression used to calculate the area of a polar region?
-The integral expression used is ∫(R^2 dθ), where R is the radius as a function of the angle θ.
What alternative method does the speaker mention for calculating the area?
-The speaker mentions an alternative method of starting from a negative angle (e.g., -2π/3) and integrating until a positive angle (e.g., 2π/3) to cover the entire area without double-counting.
How can you find the area of just the inner loop in a polar graph?
-To find the area of just the inner loop, you need to know when the inner loop starts and stops (e.g., from 2π/3 to 4π/3), and then integrate the appropriate expression over that range.
What is the relationship between finding the entire area and the area of the inner loop?
-The area between the loops can be found by subtracting the area of the inner loop from the entire area, which helps in understanding the composition of the polar region.
Why is it important to graph the polar curve correctly before finding the area?
-Graphing the polar curve correctly is important to understand the shape and limits of the region, which is essential for accurately calculating the area.
Outlines
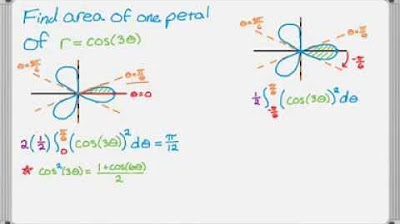
📚 Understanding Polar Area Calculations
This paragraph introduces the concept of calculating areas in polar coordinates, using a lemon-shaped graph as an example. The speaker emphasizes the importance of carefully plotting the graph to avoid double counting areas, especially when dealing with loops in the graph. Key points include identifying the limits of integration by marking angles where the radius R equals zero and the points where the graph loops back on itself. The speaker also explains the process of finding the area by integrating from 0 to 2π/3 to avoid double counting and then doubling the result to get the total area. Alternative methods of calculating the area by considering negative angles are also briefly mentioned.
🔍 Calculating Areas of Inner and Outer Loops
The second paragraph delves into the specifics of finding the area of the inner loop and the area between loops in a polar graph. The speaker explains that knowing when to start and stop the inner loop is crucial for accurate calculations. The process involves integrating from the start of the inner loop to its end, using the standard integrand R^2 dθ. The speaker also discusses alternative methods for finding the area of the inner loop, such as starting halfway through the loop or considering the area as half of the entire region and then doubling it. The paragraph concludes by noting that finding the area between loops is simply the difference between the total area and the inner loop area, highlighting the importance of graphing the polar curve for successful area calculation.
Mindmap
Keywords
💡Polar Area
💡Limits
💡Graphing in Polar
💡Theta (θ)
💡Integral
💡Inner Loop
💡Area Calculation
💡Power Reducing Formula
💡Doubling Method
💡Back Up Method
💡Area Between Loops
Highlights
Introduction to polar area problems using a lemon as an example due to its self-intersecting nature.
Importance of understanding how to graph in polar coordinates for solving polar area problems.
Identifying the limits of the polar area by plotting R=0 at 2π/3 and 4π/3.
Explanation of theta values at key points: θ=0, θ=2π/3, θ=π, θ=4π/3.
Strategy to find the area of the entire region by calculating half and then doubling it.
Using the integral from 0 to 2π/3 to find half of the area before the inner loop.
Avoiding double counting the inner loop area by stopping the integral at 2π/3.
Calculating the area using the integral of R^2 dθ from 0 to 2π/3.
Alternative method of finding the entire area by integrating from -2π/3 to 2π/3.
Using the power-reducing formula for the integrand R^2 dθ when necessary.
Finding the area of the inner loop by integrating from 2π/3 to 4π/3.
Alternative approaches to calculate the inner loop area by adjusting the limits of integration.
Calculating the area between the loops by subtracting the inner loop area from the entire area.
Emphasizing the importance of knowing when to start and stop the inner loop for area calculations.
Highlighting the common questions in polar area problems: entire area, inner loop area, and area between loops.
Providing different strategies to solve polar area problems, including doubling and backing up methods.
Encouraging practice in graphing polar curves for easier area calculations.
Concluding with the hope that the explanation is helpful and wishing good luck to the learners.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: