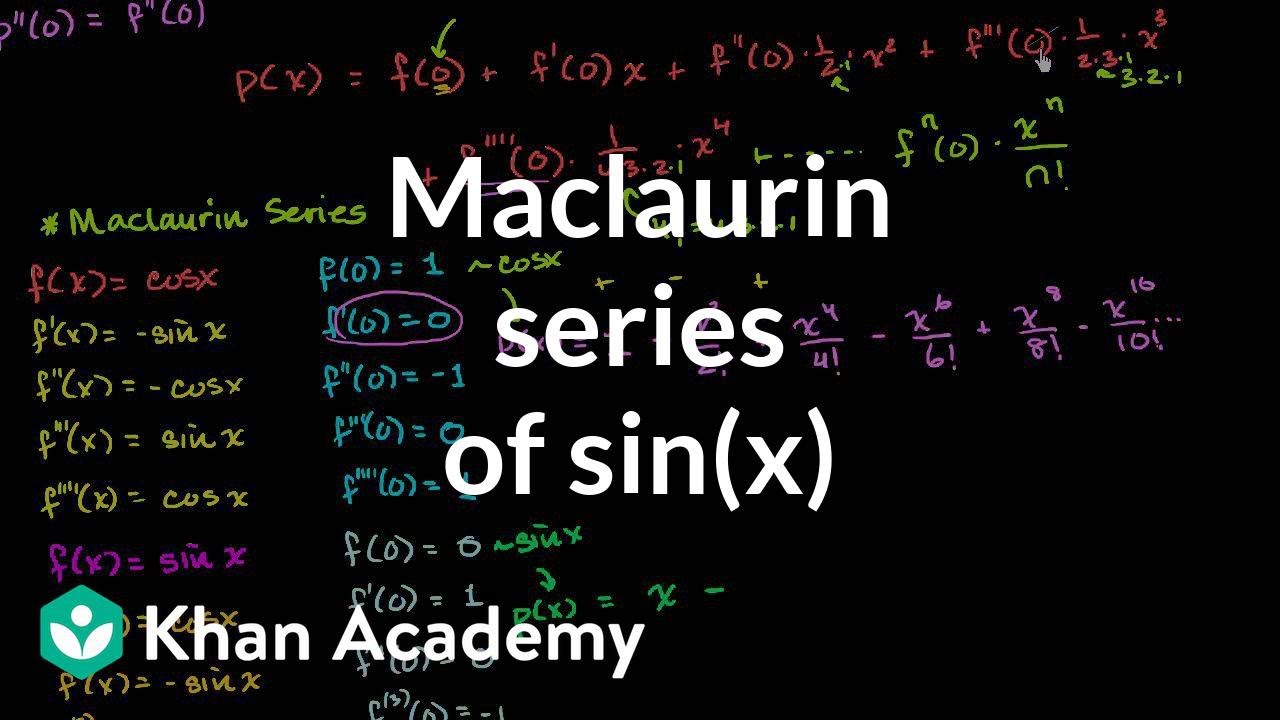Taylor Series to Memorize

turksvids
1 Nov 201407:58
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial covers four essential Taylor series to memorize: sine and cosine functions, e^x, and 1/(1-x). It explains their alternating signs, odd and even powers, and the importance of their convergence intervals. Two examples demonstrate how to apply these series by substituting variables and simplifying expressions, highlighting the practicality of memorizing these mathematical tools.

Takeaways
  • πŸ“š The video covers four essential Taylor series that need to be memorized for mathematical applications.
  • πŸ” The first series discussed is the sine function, which is represented as an alternating series of odd powers of X.
  • πŸ”„ The sine series starts with X and includes terms like (-X^3/3!), (X^5/5!), and so on, alternating in sign.
  • πŸ“‰ The cosine series is similar to sine but involves even powers of X, starting with 1 and alternating signs.
  • 🌐 The cosine series is derived from the sine series by considering cosine as an even function, emphasizing its polynomial terms.
  • πŸ”‘ The exponential function e^X is described as easier to remember, starting with 1 and including terms like (X^2/2!), (X^3/3!), and so on without alternating signs.
  • 🌐 The series for e^(iΞΈ) is mentioned as an interesting property, equating it to cos(ΞΈ) + i*sin(ΞΈ).
  • πŸ”’ The geometric series 1/(1-X) is introduced, emphasizing that it converges only when the absolute value of X is less than 1.
  • πŸ“ˆ The video provides examples of how to manipulate Taylor series, such as finding the series for sin(3X) by substituting X with 3X in the sine series.
  • πŸ“˜ An example is given for working with a complex function involving e^(-2X^3) + 2X^3 - 1, demonstrating how to add and subtract terms and divide by X^5.
Q & A

  • What are the four Taylor series discussed in the video?

    -The four Taylor series discussed in the video are sine of X, cosine of X, e to the X, and 1 over 1 minus X.

  • Why is it important to memorize the sine and cosine Taylor series?

    -Memorizing the sine and cosine Taylor series is important because they are essential for approximating these functions using polynomials, and they are often used in calculus and various mathematical applications.

  • How does the sine Taylor series differ from the cosine series?

    -The sine Taylor series consists of odd powers of X and alternates in sign, while the cosine series consists of even powers of X and also alternates in sign. This difference reflects the fact that sine is an odd function and cosine is an even function.

  • What is the general term for the sine Taylor series?

    -The general term for the sine Taylor series is (-1)^N * X^(2N+1) / (2N+1)!, where N starts from 0.

  • What is the starting term of the cosine Taylor series and why is it different from sine?

    -The cosine Taylor series starts with the term 1, which is different from the sine series because cosine is an even function and it starts with the constant term.

  • How is the Taylor series for e to the X different from the others discussed in the video?

    -The Taylor series for e to the X is different because it does not alternate in sign and includes all positive integer powers of X divided by their respective factorials.

  • What is the condition for the convergence of the Taylor series for 1 over 1 minus X?

    -The Taylor series for 1 over 1 minus X converges only when the absolute value of X is less than 1.

  • Why is it useful to know that e to the I theta equals cosine theta plus I times sine of theta?

    -Knowing that e to the I theta equals cosine theta plus I times sine of theta is useful because it connects the exponential function with the trigonometric functions, which is a fundamental concept in complex analysis.

  • How does the video demonstrate the process of finding a series for sine of 3x?

    -The video demonstrates finding a series for sine of 3x by substituting 3x for every instance of 'u' in the sine Taylor series and then simplifying the expression.

  • What is the process for working with the function e to the negative two X cubed plus 2x cubed minus 1 all over X to the fifth?

    -The process involves first writing out the Taylor series for e to the u, then substituting negative 2x cubed for 'u', simplifying the expression, and finally adding 2x cubed and subtracting 1, followed by dividing the entire series by X to the fifth.

  • Why is it important to consider the order of operations and common terms when working with Taylor series?

    -Considering the order of operations and common terms is important to ensure that the series is simplified correctly and that terms cancel out as they should, leading to the correct final expression.

Outlines
00:00
πŸ“š Memorizing Taylor Series for Basic Functions

The video script introduces the importance of memorizing four fundamental Taylor series for mathematical functions. The first series discussed is for the sine function, which is an alternating series involving odd powers of X, starting with X and continuing with terms like -X^3/3!, X^5/5!, and so on. The script emphasizes the need to remember the alternating pattern and the odd powers, which align with the sine function's odd nature. The second series is for the cosine function, which is similar but involves even powers of X, starting with 1 and including terms like -X^2/2!, X^4/4!, and so on. The script notes that cosine is an even function, hence the even powers. The third series is for e^X, which is a straightforward series starting with 1 and including terms like X^2/2!, X^3/3!, and so on, without alternation. The final series is for 1/(1-X), which is similar to e^X but without denominators, and it converges only when the absolute value of X is less than 1. The script concludes by demonstrating how to use these memorized series to quickly find series for functions like sin(3X) by substituting U with 3X in the sine series.

05:04
πŸ” Applying Taylor Series to Complex Functions

This paragraph continues the discussion by applying the memorized Taylor series to more complex functions. The script demonstrates how to find the series for e^(-2X^3) + 2X^3 - 1 divided by X^5. The process begins by substituting U with -2X^3 in the e^U series, resulting in an alternating series with terms like 1 - 2X^3 + (-2X^3)^2/2!, and so on. The script then shows how to add 2X^3 and subtract 1 to this series, leading to a simplification where the first two terms cancel out. Finally, the entire series is divided by X^5, transforming each term's power of X accordingly. The script highlights the importance of understanding pre-algebra concepts like order of operations and common terms to handle such problems effectively. The example concludes by noting that the series is only valid for X β‰  0, emphasizing the need for domain restrictions in some cases.

Mindmap
Keywords
πŸ’‘Taylor Series
The Taylor series is an infinite sum of terms calculated from the values of a function's derivatives at a single point. In the video, it is emphasized as a fundamental concept that needs to be memorized for practical applications, such as approximating functions like sine, cosine, and exponential functions.
πŸ’‘Sine of X
The sine function's Taylor series is given as an alternating series involving odd powers of x and factorial denominators. This series is crucial because it approximates the sine function using a polynomial, which is simpler to work with. The video highlights how the series alternates and is used in practice, such as finding the series for sine of 3x.
πŸ’‘Cosine of X
The cosine function's Taylor series involves even powers of x with alternating signs. It starts with 1 and includes terms like x squared over 2 factorial, minus x to the fourth over 4 factorial, and so on. The video stresses that knowing this series is essential for approximations and that its pattern is due to the even nature of the cosine function.
πŸ’‘E to the X
The exponential function's Taylor series is simpler because it doesn't alternate and includes every power of x over the corresponding factorial. The video presents this series as easier to remember and essential for various mathematical applications, such as demonstrating Euler's formula involving e^(i theta).
πŸ’‘Geometric Series
The geometric series for 1/(1-x) is introduced as another key series to memorize. It is a straightforward series without factorials, involving terms like 1, x, x squared, and so on. The video notes its importance and the condition that the absolute value of x must be less than 1 for the series to converge.
πŸ’‘Alternating Series
An alternating series is a series where the terms alternate in sign. This concept is crucial for the sine and cosine Taylor series, where the signs of the terms alternate between positive and negative. The video uses this concept to explain the structure of these series and why they approximate the respective functions effectively.
πŸ’‘Factorial
A factorial, denoted by n!, is the product of all positive integers up to n. Factorials are fundamental in the Taylor series of functions like sine, cosine, and e^x, where they appear in the denominators. The video emphasizes memorizing these series, which inherently involves understanding and using factorials.
πŸ’‘Odd and Even Functions
Odd functions have symmetry about the origin, and even functions are symmetric about the y-axis. In the video, sine is described as an odd function, leading to a Taylor series with odd powers, while cosine, an even function, has a series with even powers. This distinction helps in remembering and applying their respective series.
πŸ’‘Series Convergence
Convergence refers to the idea that as more terms are added, the series approaches a certain value. The video discusses the convergence of the geometric series for 1/(1-x), noting the condition that the absolute value of x must be less than 1. Understanding convergence is critical for correctly applying Taylor series.
πŸ’‘Term-by-Term Manipulation
Term-by-term manipulation involves modifying each term of a series individually, such as replacing variables or dividing terms. The video demonstrates this technique in examples like finding the series for sine of 3x or e^(-2x^3), showing how it simplifies working with series and adapting them to different functions.
Highlights

Introduction to running through four essential Taylor series to memorize and their application in examples.

Explanation of the Taylor series for sine of X, emphasizing its alternating nature and odd powers.

Memorization tip for sine's Taylor series by recognizing sine as an odd function.

Presentation of the Taylor series for cosine, highlighting its even powers and starting with 1.

Cosine's Taylor series as an even function leading to even powers in its series.

Description of the Taylor series for e^X, noting its non-alternating nature and factorials in the denominators.

Suggestion to explore e^(iΞΈ) and its relation to cosine and sine through power series.

Introduction of the geometric series for 1/(1-X), with a condition that |X| must be less than 1.

Emphasis on the importance of the interval of convergence for the geometric series.

Demonstration of finding a series for sine of 3X by substitution in the sine series.

Technique of simplifying the series by separating terms with their own exponents.

Example of working with e to the negative two X cubed plus 2X cubed minus 1 over X to the fifth.

Method of building the function using the Taylor series for e^u and substituting u with the given expression.

Process of simplifying the series by separating and canceling terms.

Final step of dividing the entire series by X to the fifth and adjusting the general term.

Note on the importance of order of operations and working with common terms in series manipulation.

Conclusion emphasizing the value of memorizing these Taylor series for quick problem-solving.

Transcripts

Browse More Related Video

Maclaurin series of sin(x) | Series | AP Calculus BC | Khan Academy

Polynomial approximation of functions (part 4)

Taylor Series Day 2

Calculus BC – 10.14 Finding Taylor or Maclaurin Series for a Function

AP Calculus BC Lesson 10.14 Part 2

AP Calculus BC Lesson 10.14 Part 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Taylor SeriesCalculusMathematicsEducationSine FunctionCosine Functione^xGeometric SeriesSeries ExpansionProblem SolvingMemorizability