AP Physics 1 Kinematics Free Response 8

Allen Tsao The STEM Coach
14 Nov 201805:21
EducationalLearning
32 Likes 10 Comments

TLDRIn this AP Physics 1 video, Alan from Bottle Stem Coach tackles challenging kinematic problems, focusing on a two-part projectile motion scenario involving a ball thrown onto a flat horizontal roof. He guides viewers through the process of calculating the time it takes for the ball to land on the far side, emphasizing the importance of understanding the motion in both the vertical and horizontal directions.

Takeaways
  • 🧑‍🏫 Alan from Bottle Stem Coach is presenting AP Physics 1 kinematic problems.
  • 📈 The focus is on advanced kinematic problems for thorough practice.
  • ⏸️ Alan encourages viewers to pause and attempt solving the problem themselves before watching the solution.
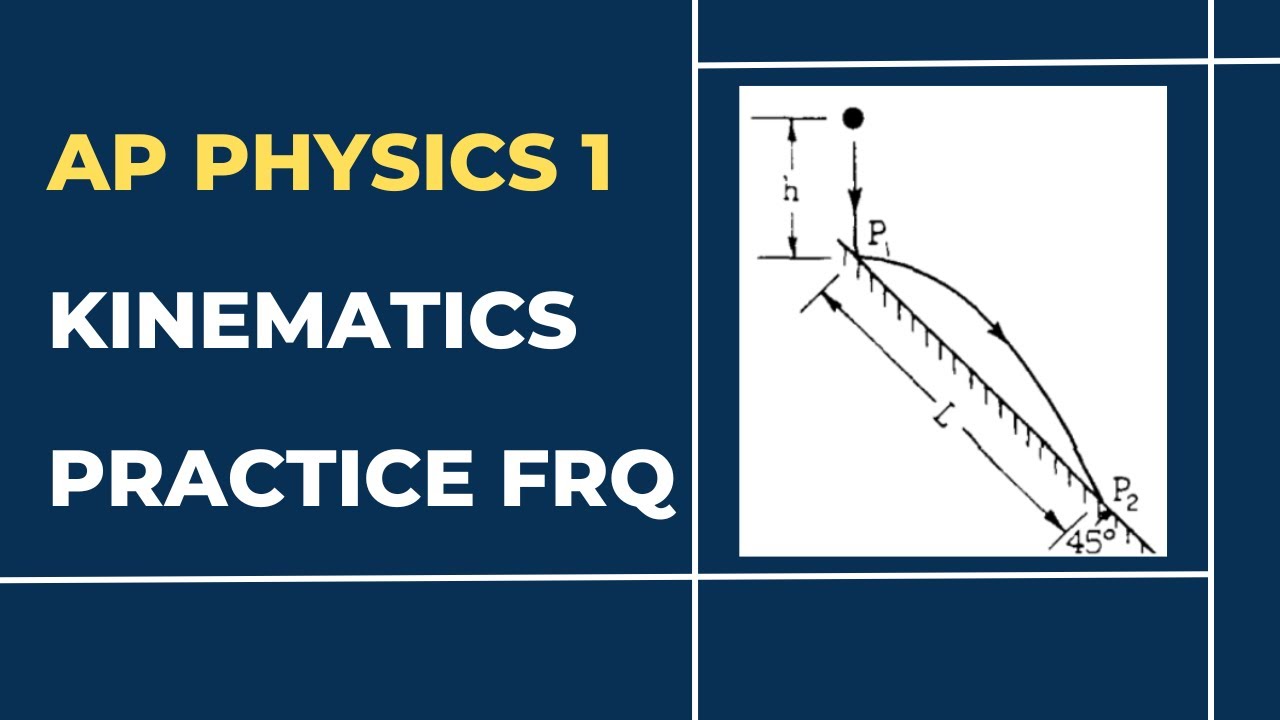
  • ⚽ The problem involves a two-part projectile motion of a ball thrown onto a flat horizontal roof.
  • 🏢 The ball lands on the roof at the highest point, rolls across the roof, and falls off the other side.
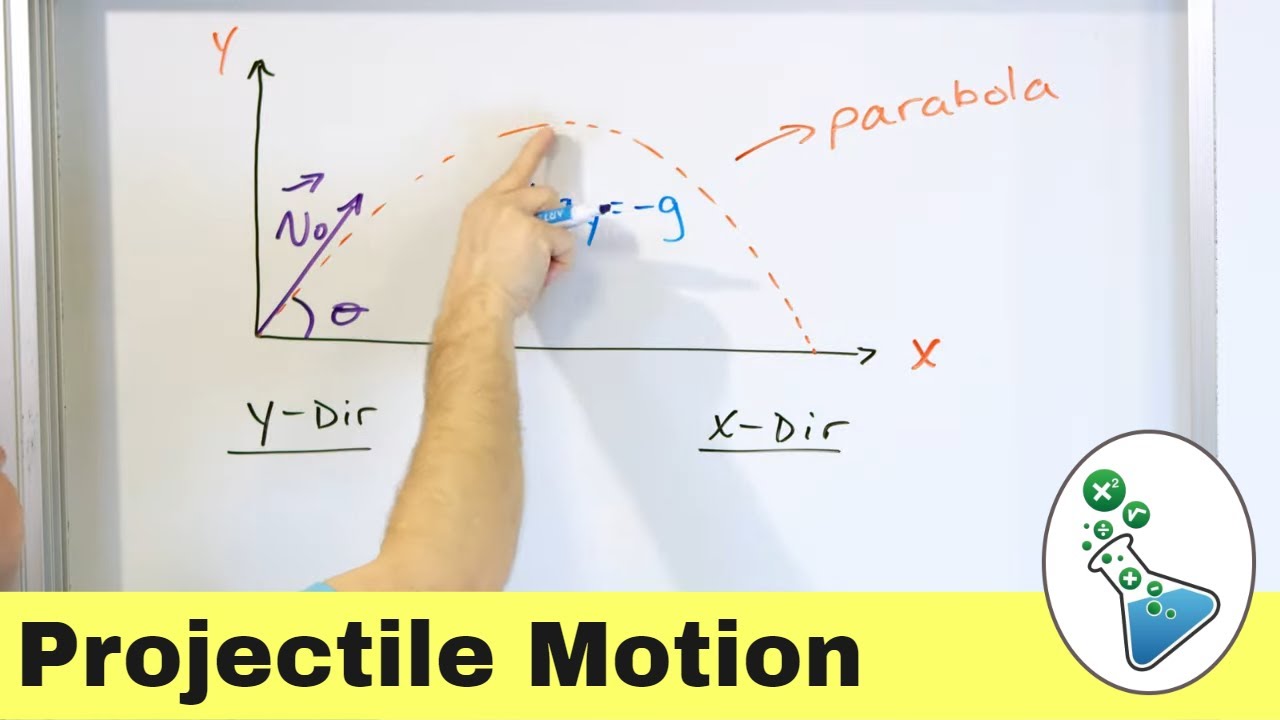
  • 📐 Variables used: initial speed (v-not), angle (theta), and length of the building (L).
  • 🔢 Time to maximum height is determined using the vertical motion equations.
  • ↕️ Time to rise to maximum height equals V naught sine theta over G, and the time to fall is the same.
  • ➡️ Time to slide across the roof is L over V naught cosine theta, considering horizontal motion with no acceleration.
  • 🕒 Total time is the sum of the times for rising, falling, and sliding across the roof: 2 V naught sine theta over G + L over V naught cosine theta.
Q & A

  • What is the context of the problem being discussed in the video?

    -The problem involves a ball being thrown onto a flat horizontal roof, landing at the highest point of its path, rolling across the roof, and then falling off the other side. The task is to find the total time from when the ball is thrown to when it lands on the far side, using given variables.

  • Which variables are given to solve the problem?

    -The given variables are the initial speed (v-not), the angle of the throw (theta), and the length of the building (L).

  • How is the time to reach the maximum height calculated?

    -The time to reach the maximum height is calculated using the equation t_{rise} = \frac{v_{not} \sin(\theta)}{g}, where g is the acceleration due to gravity.

  • Why is the time to fall the same as the time to rise?

    -The time to fall is the same as the time to rise due to the symmetry of projectile motion in the absence of air resistance.

  • What equation is used to calculate the time the ball spends sliding across the roof?

    -The equation used is L = v_{not} \cos(\theta) \cdot t, which solves to t_{slide} = \frac{L}{v_{not} \cos(\theta)}.

  • How is the total time calculated?

    -The total time is calculated by summing the time to rise, the time to fall, and the time to slide across the roof: t_{total} = 2 \frac{v_{not} \sin(\theta)}{g} + \frac{L}{v_{not} \cos(\theta)}.

  • What is the significance of ignoring friction and rotational energy in this problem?

    -Ignoring friction and rotational energy simplifies the problem to pure translational motion, making the calculations straightforward and focusing only on the kinematic aspects.

  • Why is the vertical direction chosen to find the maximum height?

    -The vertical direction is chosen because the maximum height is reached when the vertical component of the velocity (V_y) is zero.

  • How is the initial vertical velocity represented in the equations?

    -The initial vertical velocity is represented as v_{not} \sin(\theta).

  • What assumptions are made to solve this problem?

    -The assumptions include ignoring air resistance, friction, and rotational energy, and assuming constant acceleration due to gravity.

Outlines
00:00
📚 AP Physics 1 Kinematics Challenge

In this educational video, Alan from Bottle Stem Coach introduces a challenging AP Physics 1 kinematics problem involving projectile motion. The problem describes a ball thrown onto a flat horizontal roof, which lands at the highest point, rolls across, and falls off the other side without friction. Alan encourages viewers to attempt the problem before revealing the solution. The problem is broken down into three parts: the ball's ascent and descent, and its horizontal motion across the roof. The solution involves using kinematic equations to find the time it takes for the ball to complete its journey, considering the initial velocity, angle of projection, and the length of the building.

05:07
🔍 Detailed Solution to the Projectile Motion Problem

Alan proceeds to solve the problem by first addressing the vertical motion, focusing on the time to reach the maximum height, which is determined by setting the final vertical velocity to zero and using the kinematic equation involving initial velocity, acceleration due to gravity, and time. He then calculates the time for the ball to slide horizontally across the roof, using the horizontal distance and the initial horizontal velocity component. The total time for the ball's journey is found by summing the time to rise, the time to fall symmetrically, and the time to slide across the roof. The units are checked for consistency, ensuring the solution is expressed in the correct units of time and distance.

Mindmap
Keywords
💡Kinematics
Kinematics is a branch of mechanics that describes the motion of objects without considering the causes of this motion. In the video, kinematic problems involve calculating the time and distance of a ball's projectile motion, which is crucial for understanding its trajectory.
💡Projectile motion
Projectile motion refers to the motion of an object thrown or projected into the air, subject only to the acceleration of gravity. The video describes a ball's motion as it is thrown onto a roof, rolls across, and falls off, highlighting key aspects of projectile motion.
💡Initial speed (v-not)
Initial speed, denoted as v-not, is the speed of the ball when it is first thrown. It is a crucial variable in the video’s problem, used to determine the time the ball takes to reach different parts of its trajectory.
💡Angle (theta)
The angle theta is the angle at which the ball is thrown with respect to the horizontal. This angle is essential for calculating the components of the initial velocity in both vertical and horizontal directions in the video.
💡Maximum height
Maximum height is the highest point in the ball's trajectory. In the video, the maximum height is where the vertical component of the ball’s velocity becomes zero. This point is crucial for determining the time to rise and fall.
💡Symmetry
Symmetry in projectile motion refers to the property that the time to rise to the maximum height is equal to the time to fall back down to the original height. The video uses this concept to simplify the calculation of the ball’s total flight time.
💡Horizontal distance (L)
Horizontal distance, denoted as L, is the distance the ball travels across the roof. The video uses this variable to calculate the time the ball spends sliding across the frictionless roof before falling off.
💡Vertical velocity
Vertical velocity is the component of the ball's velocity in the vertical direction. In the video, it is used to determine when the ball reaches its maximum height, where the vertical velocity is zero.
💡Acceleration due to gravity (g)
Acceleration due to gravity, denoted as g, is the acceleration experienced by an object due to Earth's gravity, approximately 9.8 m/s². The video uses this constant to calculate the time it takes for the ball to rise to its maximum height and fall back down.
💡Frictionless
Frictionless describes a surface with no friction. In the video, the roof is considered frictionless, meaning the ball rolls across without any resistance, simplifying the calculations for the horizontal motion.
Highlights

Introduction to a continuation of AP Physics 1 kinematic problems.

Emphasis on tackling challenging kinematic problems beyond simple AP Physics responses.

Encouragement for viewers to attempt problems before continuing with the video.

Description of a two-part projectile motion scenario involving a ball thrown onto a flat horizontal roof.

Assumption of no friction and the use of rotational energy concepts.

Introduction of variables v-not for initial speed, theta for angle, and L for the length of the building.

Objective to find the total time from when the ball is thrown to when it lands on the far side.

Breakdown of the problem into three distinct motion parts for analysis.

Explanation of the symmetry in the time to rise and fall due to the vertical motion.

Use of the equation Vf^2 = Vi^2 + 2ad to find the time to reach maximum height.

Identification of the initial vertical velocity as v-not sine theta.

Calculation of the time to reach the maximum height using the equation Vf = Vi + at.

Determination of the time spent sliding across the roof using horizontal motion equations.

Solution for the time spent sliding across the roof using the equation Δx = V₀t + 1/2at^2.

Final calculation of total time by summing the rise, fall, and slide times.

Verification of units to ensure the correctness of the time calculation.

Conclusion and sign-off for the next video in the series.

Transcripts

Browse More Related Video

AP Physics 1 Kinematics Problem #5

Solving Projectile Motion Problems in Physics - [1-4-7]

AP Physics 1 | Unit 1: Kinematics

Projectile Motion: Shooting a Basketball Problem

Projectile Motion - AP Physics 1: Kinematics Review Supplement

Using Equations of Projectile Motion

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AP PhysicsKinematicsProjectile MotionTutorialAlan's VideoProblem SolvingPhysics EducationEducational ContentScience LearningMotion Analysis