Finding probability example 2 | Probability and Statistics | Khan Academy

Khan Academy

13 Apr 201109:56

EducationalLearning

32 Likes 10 Comments

TLDRThis video script explores three probability exercises. First, it calculates the probability of drawing a non-blue marble from a bag containing red, blue, and green marbles, resulting in a 6/7 chance. Second, it determines the likelihood of selecting a multiple of 5 from a given list, which is 7/12. Lastly, it examines the probability of a randomly chosen point from a larger circle also lying within a smaller circle inside it, concluding with a probability of 4/81. The script uses visual aids and clear explanations to simplify complex concepts.

Takeaways

🎲 The script discusses probability exercises involving marbles and circles, teaching the concept of calculating the likelihood of certain outcomes.
🛍️ A bag contains 9 red, 2 blue, and 3 green marbles, and the probability of drawing a non-blue marble is calculated by considering the total and favorable outcomes.
🔢 The total number of marbles is found by adding the red, blue, and green marbles together, which is 14 in this case.
🟥 The number of non-blue marbles is determined by subtracting the blue marbles from the total or by counting the red and green marbles directly, which equals 12.
📐 The probability of selecting a non-blue marble is simplified to 6/7 by dividing both the numerator and the denominator by their greatest common divisor, 2.
📝 The second exercise involves choosing a number from a list and determining the probability that it is a multiple of 5, which is found by identifying the multiples and dividing by the total number of options.
🔡 The multiples of 5 from the list are identified, and there are 7 such numbers, leading to a probability of 7/12 for this event.
⭕ The third exercise involves geometric probability, where a point is randomly selected within a larger circle with a smaller circle inside it.
📏 The circumference of the larger circle is given as 36π, and from this, the radius is calculated to be 18, leading to an area of 324π.
📐 The area of the smaller circle is given as 16π, and the probability that a randomly selected point from the larger circle also lies in the smaller one is the ratio of their areas.
🗂 The final probability is simplified to 4/81 by dividing both the numerator and the denominator by their greatest common divisor, 4.

Q & A

What is the total number of marbles in the bag described in the script?
-There are 14 marbles in total: 9 red marbles, 2 blue marbles, and 3 green marbles.
What is the probability of selecting a non-blue marble from the bag?
-The probability of selecting a non-blue marble is 6/7. This is calculated by dividing the number of non-blue marbles (12) by the total number of marbles (14).
How many non-blue marbles are there in the bag?
-There are 12 non-blue marbles in the bag, which includes 9 red marbles and 3 green marbles.
What is the total number of numbers to choose from in the second exercise?
-There are 12 numbers to choose from in the list provided in the second exercise.
What is the probability that a randomly chosen number from the list is a multiple of 5?
-The probability that a randomly chosen number is a multiple of 5 is 7/12. This is because there are 7 multiples of 5 in the list of 12 numbers.
How many multiples of 5 are there in the list of numbers?
-There are 7 multiples of 5 in the list, which are numbers ending in 0 or 5.
What is the circumference of the larger circle described in the script?
-The circumference of the larger circle is 36π.
What is the area of the smaller circle contained within the larger circle?
-The area of the smaller circle is 16π.
What is the radius of the larger circle with a circumference of 36π?
-The radius of the larger circle is 18, calculated by dividing the circumference (36π) by 2π.
What is the area of the larger circle with a radius of 18?
-The area of the larger circle is 324π, calculated by squaring the radius (18^2) and multiplying by π.
What is the probability that a point randomly selected from the larger circle also lies in the smaller circle?
-The probability is 4/81. This is the ratio of the area of the smaller circle (16π) to the area of the larger circle (324π).

Outlines

00:00

🎲 Calculating Probability of Selecting a Non-Blue Marble

This paragraph discusses a probability exercise involving a bag containing 9 red marbles, 2 blue marbles, and 3 green marbles. The task is to determine the probability of randomly selecting a non-blue marble from the bag. The solution involves calculating the total number of marbles (14) and identifying the number of non-blue marbles (12). The probability is then simplified by dividing both the numerator and the denominator by 2, resulting in a 6/7 chance of selecting a non-blue marble. Additionally, the paragraph introduces another probability problem involving selecting a multiple of 5 from a list of numbers, concluding with a 7/12 probability for that scenario.

05:00

📏 Probability of a Point Being in a Smaller Circle

This paragraph explores the probability of randomly selecting a point from a larger circle that also lies within a smaller circle contained within it. The larger circle has a circumference of 36 pi, and the smaller circle has an area of 16 pi. The solution involves calculating the radius of the larger circle (18) and its area (324 pi). The probability of the point being in the smaller circle is determined by the ratio of the areas of the smaller and larger circles, which simplifies to 4/81. This reflects the percentage of the larger circle's area that is occupied by the smaller circle.

Mindmap

Keywords

💡Probability

Probability refers to the measure of the likelihood that an event will occur. It is calculated as the ratio of the number of favorable outcomes to the total number of possible outcomes. In the video, probability is the central theme, as it is used to determine the likelihood of selecting a non-blue marble, a multiple of 5, and a point within a smaller circle within a larger circle.

💡Marbles

Marbles in this context are the objects in a bag used to demonstrate probability calculations. They are categorized by color, with specific quantities of red, blue, and green marbles mentioned. The script uses marbles to illustrate the concept of calculating the probability of selecting a non-blue marble.

💡Non-blue Marble

A non-blue marble represents any marble that is not blue. In the script, the probability of randomly selecting a non-blue marble from a bag containing red, blue, and green marbles is calculated. This concept is used to explain the fraction of marbles that meet a certain condition (not being blue) out of the total number of marbles.

💡Multiple of 5

A multiple of 5 is any number that can be divided by 5 without leaving a remainder. The script discusses the probability of randomly selecting a number from a list that is a multiple of 5, which is an example of using probability to find the likelihood of an event (choosing a number divisible by 5) out of a set of possibilities.

💡Circumference

Circumference is the measurement of the distance around a circle. In the script, the circumference of a larger circle is given as 36 pi, which is then used to find the radius and subsequently the area of the circle, important for calculating the probability of a point falling within a smaller circle inside it.

💡Area

Area is a measure of the space inside a two-dimensional shape. The script discusses the area of both a larger and a smaller circle, using the formula for the area of a circle (pi times the radius squared) to calculate the probability that a randomly selected point within the larger circle also lies within the smaller circle.

💡Radius

The radius of a circle is the distance from its center to any point on its edge. In the video, the radius is derived from the given circumference of the larger circle (36 pi) to find the area, which is necessary for calculating the probability of a point being within the smaller circle.

💡Random Selection

Random selection implies choosing an item from a set without any bias or preference. The script uses this concept to describe the process of picking a marble from a bag or a point from a circle, where each item has an equal chance of being selected, which is fundamental to calculating probabilities.

💡Constraint

A constraint in the context of the script refers to a condition that must be met for an outcome to be considered successful. For example, selecting a non-blue marble or a multiple of 5 is a constraint that defines the set of successful outcomes from the total possible outcomes.

💡Favorable Outcomes

Favorable outcomes are those outcomes that meet the criteria set by the constraint in a probability problem. In the script, the number of non-blue marbles and multiples of 5 are examples of favorable outcomes, as they are the successful outcomes that fulfill the condition of the problem.

💡Total Possibilities

Total possibilities refer to the entire set of outcomes that can occur in a probability scenario. The script mentions the total number of marbles and numbers as the total possibilities from which favorable outcomes are selected to calculate probabilities.

Highlights

Introduction to probability exercises involving marbles and circles.

A bag contains 9 red marbles, 2 blue marbles, and 3 green marbles.

The probability of selecting a non-blue marble is calculated by considering the total number of marbles.

Total marbles in the bag are 14, calculated by adding red, blue, and green marbles.

Non-blue marbles are either red or green, totaling 12.

The probability of selecting a non-blue marble is simplified to 6/7.

A list of numbers is presented to determine the probability of selecting a multiple of 5.

There are 12 numbers in the list, each with an equal chance of being selected.

Multiples of 5 are identified by looking for numbers ending in 5 or 0.

Seven numbers in the list are multiples of 5.

The probability of selecting a multiple of 5 is 7/12.

A circle with a circumference of 36 pi is introduced, with a smaller circle inside.

The smaller circle has an area of 16 pi.

The probability of a randomly selected point from the larger circle also lying in the smaller circle is explored.

The radius of the larger circle is calculated from its circumference, found to be 18.

The area of the larger circle is calculated as 324 pi.

The probability of a point being in the smaller circle is the ratio of their areas, simplified to 4/81.

Transcripts

Browse More Related Video

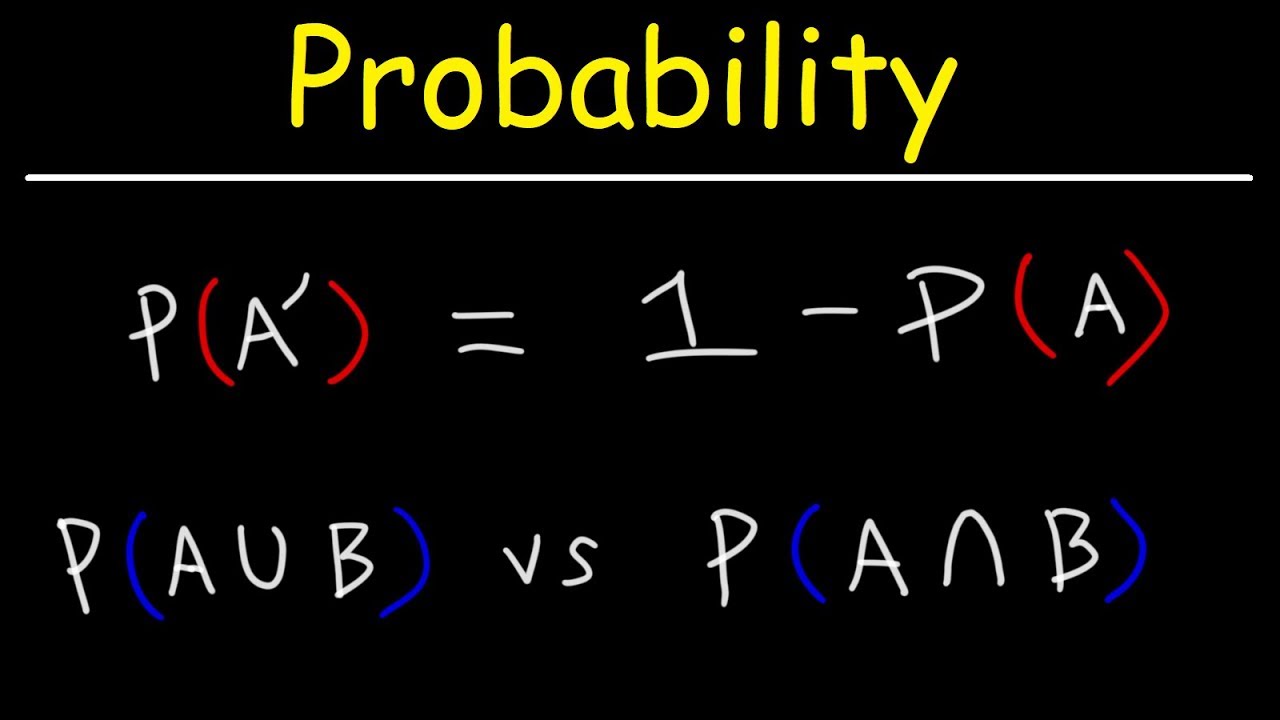
Probability of Complementary Events & Sample Space

Math 119 Chapter 4 part 2

Average x-Coordinate in a Region | MIT 18.01SC Single Variable Calculus, Fall 2010

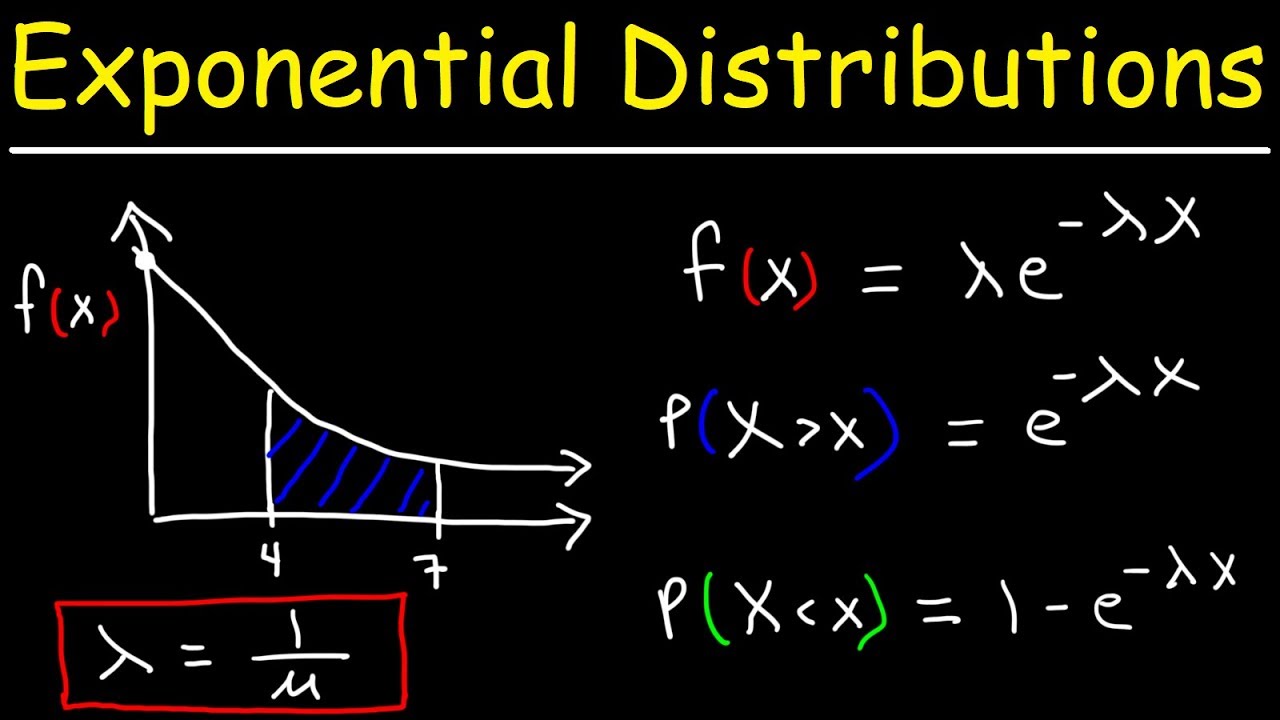
Probability Exponential Distribution Problems

2 | FRQ (Part A: Probability) | Practice Sessions | AP Statistics

Probability of making 2 shots in 6 attempts | Probability and Statistics | Khan Academy

Finding probability example 2 | Probability and Statistics | Khan Academy

Takeaways

Q & A

What is the total number of marbles in the bag described in the script?

What is the probability of selecting a non-blue marble from the bag?

How many non-blue marbles are there in the bag?

What is the total number of numbers to choose from in the second exercise?

What is the probability that a randomly chosen number from the list is a multiple of 5?

How many multiples of 5 are there in the list of numbers?

What is the circumference of the larger circle described in the script?

What is the area of the smaller circle contained within the larger circle?

What is the radius of the larger circle with a circumference of 36π?

What is the area of the larger circle with a radius of 18?

What is the probability that a point randomly selected from the larger circle also lies in the smaller circle?