2 | FRQ (Part A: Probability) | Practice Sessions | AP Statistics

Advanced Placement
17 Apr 202313:56
EducationalLearning
32 Likes 10 Comments

TLDRIn this AP Stats tutorial, Luke Wilcox explores probability through two free response questions. The first involves a shampoo filling machine with a normal distribution, calculating the probability of under-filled bottles and the likelihood of crate rejection due to under-filled shampoo bottles. The second question examines the performance of a new igniter for model rockets, assessing the success rate and failure probability over multiple tests. Wilcox's session concludes with a discussion on statistical evidence suggesting a lower failure rate for the new igniter, providing a comprehensive review of probability concepts.

Takeaways
  • 📚 The video is an AP Stats practice session led by Luke Wilcox, an AP Stats teacher at East Kentwood High School in Michigan.
  • 📝 Students are encouraged to download and attempt the probability questions before watching the video for self-assessment.
  • 🧴 The first problem involves a shampoo filling machine with a normal distribution of shampoo amounts, aiming to find the probability of underfilling.
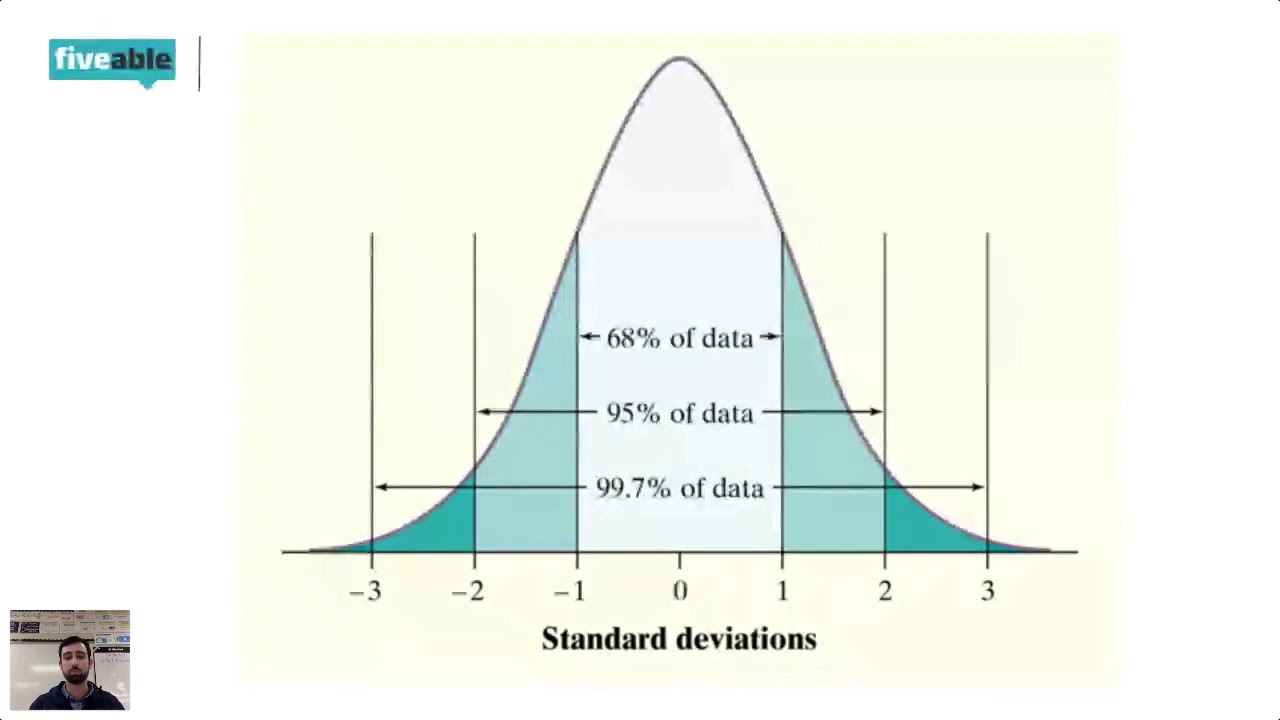
  • 📉 The normal distribution of shampoo amounts is characterized by a mean of 0.6 liters and a standard deviation of 0.04 liters.
  • 🔢 The z-score calculation for the underfilling threshold (0.5 liters) results in -2.5, indicating 2.5 standard deviations below the mean.
  • 📊 Using the z-table, the probability of a bottle being underfilled is approximately 0.0062 or 0.062.
  • 📦 The second scenario involves shipping crates of shampoo bottles, where crates may be rejected if a random box contains two or more underfilled bottles.
  • 🎯 The probability of crate rejection is calculated using the binomial distribution, considering the independence of bottle fillings.
  • 📈 Adjusting the machine's programming to a mean of 0.56 liters and a standard deviation of 0.03 actually increases the underfill probability to 0.02275, not improving the situation.
  • 🚀 The second question discusses the testing of a new 'super igniter' for model rockets with a given 15% failure rate.
  • 🔄 The testing process for super igniters involves launching rockets sequentially until a failure occurs or 32 successful launches happen.
  • 🤔 Given the success of the first 30 super igniters, a statistical analysis suggests that it may be reasonable to believe the failure rate is less than 15% based on a very low p-value.
Q & A

  • Who is the speaker in the video session?

    -The speaker is Luke Wilcox, an AP Stats teacher at East Kentwood High School in Kentwood, Michigan.

  • What is the main topic of the video session?

    -The main topic of the video session is to go through two free response questions related to probability.

  • What is the recommendation for students before watching the video?

    -The recommendation is for students to download the questions first and try them on their own before watching the video.

  • What is the normal distribution of the shampoo amount in the bottles according to the script?

    -The shampoo amount in the bottles is normally distributed with a mean of 0.6 liters and a standard deviation of 0.04 liters.

  • What is considered as an under filled bottle in the script?

    -A bottle is considered to be under filled if it has less than 0.5 liter of shampoo.

  • What is the calculated probability that a randomly selected bottle of shampoo will be under filled?

    -The calculated probability that a randomly selected bottle of shampoo will be under filled is about 0.062 or 6.2%.

  • What is the distribution of the random variable X, which represents the number of under filled bottles in a box of 10 bottles?

    -The random variable X is distributed as a binomial random variable with a fixed number of trials n equal to 10 and the probability of each bottle being under filled is 0.062.

  • What is the condition for a crate to be rejected by the beauty supply warehouse manager?

    -A crate will be rejected if two or more bottles in the randomly selected box are under filled.

  • What is the calculated probability that a crate will be rejected by the warehouse manager?

    -The calculated probability that a crate will be rejected by the warehouse manager is 0.0017 or 0.17%.

  • What is the company considering to reduce the number of crates rejected by the beauty supply warehouse manager?

    -The company is considering adjusting the program of the filling machine so that the amount of shampoo in each bottle is normally distributed with a mean of 0.56 and a standard deviation of 0.03.

  • What is the recommendation for the manufacturing company regarding the original vs. adjusted programming of the filling machine?

    -The recommendation is to continue using the original programming of the filling machine because the probability of an under filled bottle is lower with the original programming (0.062) compared to the adjusted programming (0.02275).

  • What is the failure rate of the igniters in the company's model rockets?

    -The company estimates that the overall failure rate of the igniters is 15%.

  • What is the significance of the testing process for the super igniters?

    -The testing process is to determine the performance of the super igniters by launching rockets with them until a failure occurs or 32 successful launches have been achieved.

  • What is the probability that the first 30 super igniters selected using the testing process successfully launch rockets?

    -The probability that the first 30 super igniters successfully launch rockets is 0.0076 or 0.76%.

  • Given that the first 30 super igniters successfully launch rockets, what is the probability that the first failure occurs on the 31st or 32nd super igniter tested?

    -The probability that the first failure occurs on the 31st or 32nd super igniter tested, given that the first 30 were successful, is 0.2775 or 27.75%.

  • Is it reasonable to believe that the failure rate of the super igniters is less than 15% given the first 30 successfully launched rockets?

    -Yes, it is reasonable to believe that the failure rate is less than 15% because the probability of 30 consecutive successful launches with a 15% failure rate is very low (0.0076), which is strong evidence against the null hypothesis of a 15% failure rate.

Outlines
00:00
📚 AP Statistics Practice Session Introduction

Luke Wilcox, an AP Stats teacher at East Kentwood High School, introduces an AP Daily practice session focused on probability. He encourages students to download and attempt two free response questions before watching the video. The first question involves a machine that fills shampoo bottles with a normal distribution of mean 0.6 liters and standard deviation 0.04 liters. The task is to determine the probability of underfilling, defined as less than 0.5 liters. Wilcox advises using a normal distribution graph to visualize the problem and calculate the z-score for the boundary value, leading to a probability of underfilling of approximately 0.062. The second scenario involves boxes of shampoo bottles and the probability of crate rejection based on underfilled bottles.

05:02
🔢 Calculating Probability of Crate Rejection

The session continues with the second part of the free response question, which concerns the probability of a crate being rejected due to underfilled shampoo bottles. The random variable X represents the number of under filled bottles in a box of 10, following a binomial distribution with n=10 and a probability of underfilling per bottle of 0.062. To find the probability of crate rejection, which requires two or more under filled bottles, Wilcox uses the complement rule, subtracting the probability of 0 or 1 under filled bottle from 1. Using the binomial formula, he calculates the probability of rejection to be very low at 0.0017. The company then considers adjusting the filling machine's settings to a new mean of 0.56 liters and standard deviation of 0.03 liters. After comparing the probabilities of underfilling for both settings, Wilcox recommends maintaining the original programming due to a lower probability of underfilled bottles.

10:05
🚀 Testing the Performance of Super Igniters

The video script addresses a different scenario involving the testing of a new type of igniter for model rockets, aiming to reduce the failure rate from an estimated 15%. The testing process involves launching rockets with super igniters until a failure occurs or 32 successful launches are achieved. For part A, the probability of the first 30 super igniters all being successful is calculated by raising the success probability (0.85) to the power of 30, resulting in 0.0076. In part B, given the first 30 igniters are successful, the probability of the first failure occurring on the 31st or 32nd igniter is found by considering the two mutually exclusive events and adding their probabilities, yielding 0.2775. Part C poses a question about the reasonableness of believing the failure rate is less than 15% based on the successful launches. Wilcox suggests treating this as a significance test, with the null hypothesis being a 15% failure rate. The low probability of 0.0076 under the null hypothesis provides convincing evidence that the failure rate is indeed less than 15%. The session concludes with a preview of the next video, which will cover data collection.

Mindmap
Keywords
💡AP Stats
AP Stats refers to the Advanced Placement Statistics course, which is a college-level curriculum taught in high school. In the video, Luke Wilcox is an AP Stats teacher, and the content of the video is tailored to students preparing for the AP Statistics exam, focusing on probability-related questions.
💡Normal Distribution
A normal distribution, also known as a Gaussian distribution, is a type of continuous probability distribution that is characterized by its symmetrical bell-shaped curve. In the script, the amount of shampoo filled in bottles is described as having a normal distribution with a mean of 0.6 liters and a standard deviation of 0.04 liters, which is used to calculate probabilities of underfilling.
💡Mean
The mean, often referred to as the average, is a measure of central tendency in statistics. It is calculated by summing all the values in a data set and then dividing by the number of values. In the context of the video, the mean is used to describe the average amount of shampoo in the bottles, which is 0.6 liters.
💡Standard Deviation
Standard deviation is a measure that indicates the amount of variation or dispersion in a set of values. A low standard deviation indicates that the values tend to be close to the mean, while a high standard deviation indicates that the values are spread out over a wider range. In the script, the standard deviation of 0.04 liters is used to describe the spread of the shampoo amounts in the bottles.
💡Z-Score
A z-score represents the number of standard deviations a data point is from the mean. It is used in standardizing the data and is particularly useful in normal distributions. In the video, the z-score is calculated to determine the probability of a bottle being underfilled, with a z-score of negative 2.5 indicating 2.5 standard deviations below the mean.
💡Binomial Distribution
A binomial distribution is a discrete probability distribution of the number of successes in a fixed number of independent Bernoulli trials, each with the same probability of success. In the script, the binomial distribution is used to model the number of underfilled bottles in a box of 10, given the probability of an individual bottle being underfilled.
💡Complement Rule
The complement rule in probability states that the probability of the complement of an event is 1 minus the probability of the event itself. In the script, the complement rule is used to calculate the probability that a crate will be rejected by considering the probability that fewer than two bottles in a box are underfilled and subtracting this from 1.
💡Failure Rate
Failure rate is a measure of the probability that a device will fail during a specified time period. In the context of the video, the failure rate of the igniters is estimated to be 15%, meaning that 15% of all igniters fail to operate correctly. This is a key parameter in assessing the performance of the new 'super igniters'.
💡Significance Test
A significance test is a statistical method used to determine whether the results of a study are statistically significant. It involves setting up null and alternative hypotheses and calculating a p-value to decide whether to reject the null hypothesis. In the script, the concept of a significance test is discussed in the context of whether the failure rate of the super igniters is convincingly less than 15%.
💡P-Value
A p-value is the probability that a statistical model or hypothesis is true, given the data. A small p-value (typically ≤ 0.05) indicates strong evidence against the null hypothesis, suggesting that the observed results are unlikely to have occurred by chance. In the script, the p-value is used to argue that there is convincing evidence that the failure rate of the super igniters is less than 15%.
Highlights

Introduction to AP Daily practice sessions by Luke Wilcox, an AP Stats teacher.

Recommendation to try probability questions before watching the video for better understanding.

Explanation of a normal distribution problem involving a shampoo filling machine with a mean of 0.6 liters and standard deviation of 0.04 liters.

Calculation of the probability of under-filled shampoo bottles using the z-score method.

Use of the normal distribution table to find the area corresponding to the z-score for probability calculation.

Introduction of a binomial distribution problem regarding the shipping of shampoo bottles in crates.

Explanation of the binomial distribution and its application to calculate the probability of crate rejection.

Use of the complement rule to simplify the calculation of the probability of crate rejection.

Binomial probability calculation for the number of under-filled bottles in a box of 10.

Discussion on the manufacturing company's consideration to adjust the filling machine's programming.

Statistical justification for choosing between the original and adjusted machine programming based on under-fill probability.

Introduction to the second free response question about model rockets and super igniters.

Testing process for super igniters and its impact on the failure rate calculation.

Calculation of the probability of the first 30 super igniters successfully launching rockets.

Probability calculation for the first failure occurring on the 31st or 32nd super igniter.

Hypothesis testing approach to determine if the super igniter's failure rate is less than 15%.

Significance of the P-value in providing evidence for a lower failure rate than initially estimated.

Conclusion and invitation to the next session on collecting data in AP Stats.

Transcripts

Browse More Related Video

2022 Live Review 2 | AP Statistics | Making Sense of Probability & Random Variables

4 | FRQ (Part A: Multi-Focus) | Practice Sessions | AP Statistics

Generalizing k scores in n attempts | Probability and Statistics | Khan Academy

3 | FRQ (Part A: Sampling) | Practice Sessions | AP Statistics

AP Stats - Cram Review (2019)

1 | MCQ | Practice Sessions | AP Statistics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AP StatsProbabilityEducationPracticeVideosShampoo BottlesBinomial DistributionManufacturingTesting ProcessIgnitersStatistical Analysis