Directional derivative, formal definition
TLDRThis video script delves into the concept of partial and directional derivatives for two-variable functions. It explains the formal definition of the partial derivative with respect to 'X', using a visual approach to illustrate how a function is influenced by a slight nudge in the 'X' direction. The script then transitions to the directional derivative, emphasizing the use of vector notation to represent the direction of the nudge. It discusses the importance of considering the magnitude and direction of the vector in calculating the rate of change of the function, highlighting the potential impact of scaling the vector on the derivative's value.
Takeaways
- 📚 The video discusses the formal definition of the partial derivative of a two-variable function with respect to X.
- 📐 It introduces the concept of the directional derivative, which extends the idea of the partial derivative to any direction in the input space.
- 📈 The partial derivative is visualized as the rate of change of the function when 'nudging' the input in the X direction.
- 📍 The script uses a diagram to illustrate the concept of input space (X Y plane) and output space (real number line).
- 🔍 The partial derivative is defined as the limit of the ratio of the change in the function to the change in the input variable as the change approaches zero.
- 📝 The script introduces vector notation to express the partial derivative, emphasizing the role of the unit vector in the X direction.
- 🧭 The directional derivative is defined as the limit of the ratio of the change in the function to the scaled change in the input vector in a given direction.
- 📉 The importance of the direction of the vector V in the directional derivative is highlighted, as it determines the direction of the 'nudge' in the input space.
- 🔄 The script mentions that scaling the vector V by a factor affects both the initial nudge and the resulting change in the output, which is a key aspect of the directional derivative.
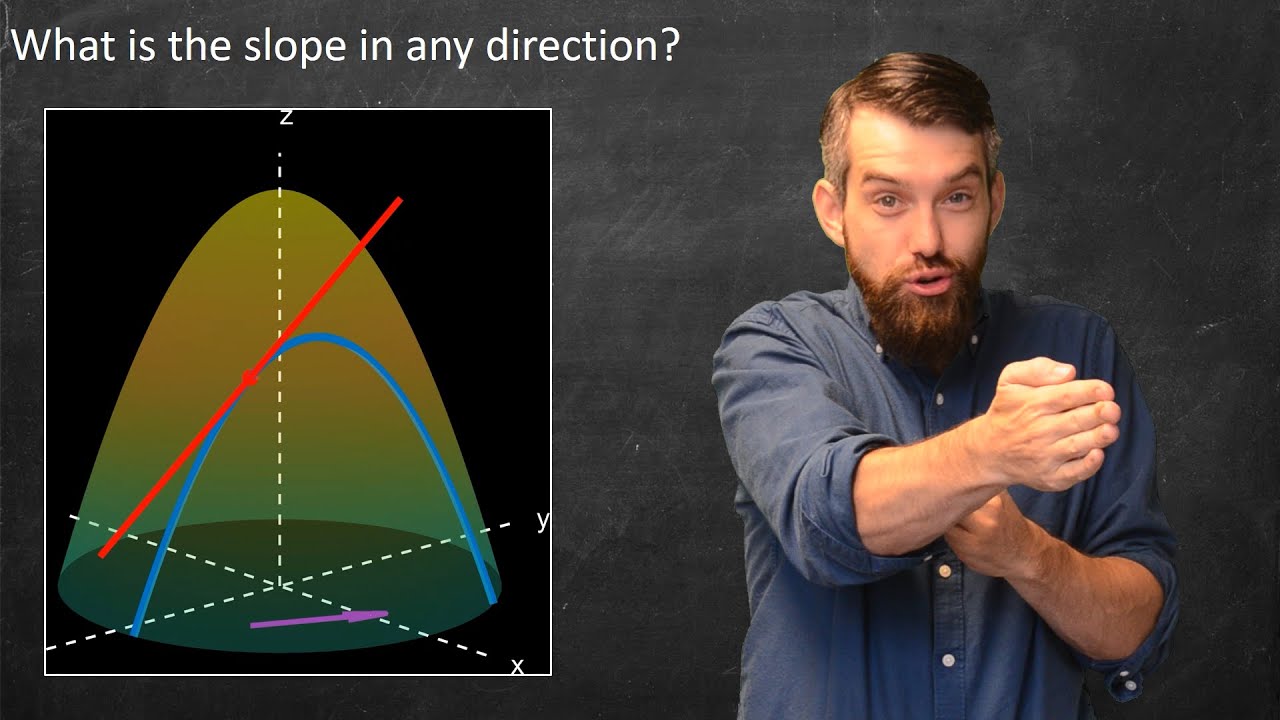
- 🤔 The video hints at the interpretation of the directional derivative as the slope of a graph, which will be discussed in a subsequent video.
- 📝 Some authors modify the definition of the directional derivative to include the absolute value of the vector to focus solely on direction, but the script suggests that the current definition has its merits.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is the formal definition of the partial derivative of a two-variable function and the introduction to the concept of the directional derivative.
What does the video script define as the input space?
-The input space is defined as the X-Y plane, which is the plane where the function's input variables lie.
How does the script describe the process of taking the partial derivative?
-The script describes taking the partial derivative by nudging the function slightly in the X direction and observing how it influences the function's output.
What is the role of variable 'H' in the formal definition of the partial derivative?
-In the formal definition, 'H' represents the change in the input space, which is used to measure the influence on the function when only the X component is altered.
What does the script suggest using to represent the unit vector in the X direction?
-The script suggests using a hat over the letter 'I' (i.e., \(\hat{i}\)) to represent the unit vector in the X direction.
How is the concept of the directional derivative introduced in the script?
-The directional derivative is introduced as an extension of the partial derivative, where the function's change is considered in the direction of any vector, not just along the X or Y axis.
What is the significance of the vector 'V' in the context of the directional derivative?
-The vector 'V' represents the direction in which the directional derivative is being evaluated. It captures the direction of the nudge in the input space that influences the function's output.
How does the script explain the scaling of vector 'V' in the directional derivative?
-The script explains that if you scale the vector 'V' by a certain value, it will double the initial nudge and the resulting nudge in the output, thus doubling the size of the derivative.
What is the role of the limit in the formal definition of the directional derivative?
-The limit in the formal definition of the directional derivative is used to consider the behavior of the function as the nudge (H) approaches zero, which helps in finding the rate of change at that point.
Why might some authors add the absolute value of the original vector in the definition of the directional derivative?
-Some authors add the absolute value of the original vector to ensure that scaling the vector does not influence the derivative's magnitude, focusing only on the direction.
What does the script suggest about the interpretation of the directional derivative?
-The script suggests that the directional derivative can be interpreted as the slope of a graph, but cautions that care must be taken in this interpretation, especially when considering the scaling of the vector 'V'.
Outlines
📚 Introduction to Partial Derivatives and Directional Derivatives
The script begins with an introduction to the formal definition of the partial derivative of a two-variable function with respect to one variable, X. It aims to lead into the concept of the directional derivative, which measures the rate of change of a function in a specific direction. The explanation includes a visual representation of the input space (X-Y plane) and output space (real number line), and how the partial derivative is calculated by considering a small change in the X direction. The script also introduces vector notation to describe the change in the function, using the unit vector in the X direction, represented by a hat over 'i'. The transition to the directional derivative is explained, highlighting how the direction of change is captured by a vector, and the formal definition of the directional derivative is presented, emphasizing the use of vector notation for clarity.
🔍 Exploring the Directional Derivative and Its Interpretation
In the second paragraph, the script delves deeper into the concept of the directional derivative, discussing its calculation and interpretation. It explains how the directional derivative is the ratio of the change in the output to the change in the input direction, and how this ratio is taken as the input nudge approaches zero. The script also touches on the potential confusion that arises when scaling the direction vector, as it affects both the input and output changes, which could be addressed by adjusting the definition to include the absolute value of the vector. However, the speaker prefers the original definition and plans to explore the implications of vector scaling in subsequent videos. The paragraph concludes with a promise to discuss the interpretation of the directional derivative as the slope of a graph in the next video.
Mindmap
Keywords
💡Partial Derivative
💡Directional Derivative
💡Input Space
💡Output Space
💡Unit Vector
💡Vector Notation
💡Limit
💡Nudge
💡Slope
💡Magnitude
💡Vector V
Highlights
Introduction to the formal definition of the partial derivative of a two-variable function with respect to X.
Building up to the formal definition of the directional derivative in the direction of a vector V.
Explanation of the input space as the X Y plane and the output space as the real number line.
Visualizing the partial derivative by nudging a point in the X direction and observing the function's influence.
The concept of partial F and partial X in the context of a slight nudge in the input space.
Use of variable H to represent the change in the input space and its effect on the function.
Vector notation is introduced to rewrite the definition of the partial derivative.
Clarification of the unit vector in the X direction represented by the hat notation.
The idea of extending the concept to moving in different directions using vector addition.
Formal definition of the directional derivative in terms of vector notation.
Interpretation of the directional derivative as the slope of a graph.
The impact of scaling the vector V on the directional derivative and the resulting nudge to the output.
Discussion on the potential modification of the definition to include the absolute value of the vector V.
Preference for the current definition of the directional derivative and its implications.
Anticipation of further discussion on the interpretation of the directional derivative in subsequent videos.
Final summary of the formal definition for the directional derivative to be considered.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: