Vector Addition and Resultants (in 3 dimensions)

Houston Math Prep
17 Jan 202107:59
EducationalLearning
32 Likes 10 Comments

TLDRThis video from Houston Math Prep delves into the concept of vector addition and resultants, particularly in 3D space. It explains how to add vectors component-wise and visualizes the process by moving one vector's starting point to the other's endpoint. The video demonstrates the commutative property of vector addition through examples, including finding the resultant of two vectors given their components. It also addresses the resultant of multiple forces and introduces the concept of equilibrium, showing how to calculate an additional force needed to achieve a zero resultant vector.

Takeaways
  • 📚 Vector addition involves moving the initial point of one vector to the endpoint of another.
  • 🔍 In 3D space, vectors have three components: v1, v2, v3 for vector v and w1, w2, w3 for vector w.
  • ➕ Vector addition is done component-wise: add the corresponding components of each vector.
  • 🎯 The resultant vector represents the sum of two or more vectors.
  • 🧮 Example: Adding vectors v = (-5, 2, -6) and w = (3, 8, -3) results in v + w = (-2, 10, -9).
  • 🔄 Vector addition is commutative: v + w = w + v.
  • 📐 When vectors are added, the result can be visualized as a diagonal in a parallelogram formed by the vectors.
  • 🔢 In vector addition, the order of addition does not affect the resultant vector.
  • ⚙️ Example with three vectors: Adding forces f1 = (3, 6, 0), f2 = (-9, 0, 12), and f3 = (-3, 18, 9) results in (-9, 24, 21).
  • ⚖️ To achieve equilibrium (zero vector), an additional force equal and opposite to the resultant vector must be added.
Q & A

  • What is the main topic discussed in the video?

    -The main topic of the video is vector addition and resultant vectors in 3D space, also known as R3.

  • How are the components of vector V labeled in the video?

    -The components of vector V are labeled as V1, V2, and V3.

  • What does the video suggest to do when adding vector W to vector V?

    -The video suggests moving the initial point of vector W to the end of vector V to visually interpret the result of vector addition.

  • What is the resultant vector?

    -The resultant vector is the vector that represents the sum of two or more vectors, showing the total amount of units traveled in each direction.

  • How is the resultant vector found when given two vectors V and W?

    -The resultant vector is found by adding the corresponding components of vectors V and W together component-wise.

  • What is the example given in the video for finding the resultant of two vectors?

    -The example given is finding the resultant of vector V = (-5, 2, -6) and vector W = (3, 8, -3) by adding their components to get the resultant vector (-2, 10, -9).

  • Why is the order of addition not important when adding vectors?

    -The order of addition is not important because adding real numbers, and by extension vector components, is a commutative operation, meaning V + W is the same as W + V.

  • What does the video illustrate about the addition of vectors using a parallelogram?

    -The video illustrates that regardless of the order in which vectors are added, the resultant vector runs diagonally across a parallelogram formed by the two vectors, leading to the same point.

  • What is the concept of equilibrium in the context of the video?

    -Equilibrium in the context of the video refers to a state where the resultant of all forces or vectors is zero, indicating no net change or movement.

  • How does the video solve for an additional force to achieve equilibrium?

    -The video solves for an additional force by finding a vector that, when added to the existing resultant, zeros out each component, resulting in a zero vector.

  • What is the final problem presented in the video involving more than two vectors?

    -The final problem involves finding the resultant of three force vectors F1 = (3, 6, 0), F2 = (-9, 0, 12), and F3 = (-3, 18, 9) and determining an additional force to achieve equilibrium.

Outlines
00:00
📚 Introduction to Vector Addition in 3D Space

This paragraph introduces the concept of vector addition in three-dimensional space (R3). It explains how to add two vectors, V and W, by moving the initial point of one vector to the end of the other and then summing their components to get a resultant vector. The video provides a step-by-step example of adding two vectors with components (-5, 2, -6) and (3, 8, -3), resulting in a new vector (-2, 10, -9). The paragraph also discusses the commutative property of vector addition, meaning the order of addition does not affect the resultant vector.

05:02
🔍 Calculating the Resultant of Multiple Vectors

This paragraph delves into the process of finding the resultant of more than two vectors, using forces as an example. It outlines how to sum three given force vectors with components (3, 6, 0), (-9, 0, 12), and (-3, 18, 9) to obtain a resultant force vector of (-9, 24, 21). The video then poses a question about achieving equilibrium by adding an additional force vector that, when combined with the previous three, results in a zero vector. The solution involves adding a vector with components (9, -24, -21) to cancel out the existing resultant, thus achieving equilibrium. The paragraph concludes with a summary of the key points and a prompt for further learning.

Mindmap
Keywords
💡Vector Addition
Vector addition is the process of combining two or more vectors to produce a resultant vector. In the context of the video, it's described as traveling along one vector and then continuing along another, resulting in a single path that represents the total displacement. The script illustrates this with the example of adding vector v and vector w in 3D space, where the components of each vector are summed to find the components of the resultant vector.
💡3D Space (R3)
3D space, or R3, refers to a geometric setting where every point can be defined by three coordinates, commonly referred to as the x, y, and z axes. The video uses this concept to explain how vectors with three components, such as v and w, are added together. The script demonstrates that the addition of these vectors in R3 results in a new vector that encapsulates the total movement in all three dimensions.
💡Components
In the context of vectors, components are the individual elements of a vector that correspond to its position along each axis in space. The script breaks down vector v into components v1, v2, and v3, and vector w into w1, w2, and w3. These components are crucial for understanding how vector addition works, as they are the values that are directly added together to find the resultant vector's components.
💡Resultant
A resultant is the outcome of adding two or more vectors together. It represents the combined effect of the original vectors. In the video, the term is used to describe the vector that is obtained after adding vectors v and w. The script provides an example where the resultant of adding vectors v and w is calculated by adding their respective components, yielding a new vector that represents the total displacement.
💡Commutative Operation
A commutative operation is one where the order of the operands does not affect the result. In the script, it is explained that vector addition is commutative, meaning that the resultant vector remains the same whether you add vector v to w or w to v. This property is demonstrated through the visual representation of the parallelogram that results from adding vectors in either order.
💡Parallelogram
In the context of vectors, a parallelogram is formed when two vectors are placed tail to head, with the resultant vector running diagonally across it. The script uses the parallelogram to visually demonstrate the commutative property of vector addition, showing that regardless of the order in which vectors are added, the diagonal (resultant vector) remains the same.
💡Equilibrium
Equilibrium in the context of forces and vectors means a state where the net effect is zero, resulting in no change in motion. The script discusses finding an additional force that, when added to a set of forces, would result in a zero resultant vector, indicating a state of equilibrium. This is illustrated by finding a vector that would counteract the resultant of three given forces, leading to a net force of zero.
💡Force Vectors
Force vectors are representations of physical forces as vectors, where the direction of the vector indicates the direction of the force, and its magnitude represents the strength of the force. In the script, three forces are given as vectors f1, f2, and f3, and the task is to find their resultant to understand the overall effect of these forces.
💡Component-wise Addition
Component-wise addition refers to the process of adding corresponding components of two or more vectors to find the components of the resultant vector. The script demonstrates this by adding the x, y, and z components of vectors v and w to find the components of the resultant vector v + w.
💡Displacement
Displacement is the change in position of an object and is represented by a vector whose magnitude is the distance between the starting and ending points, and direction is from the start to the end. In the video, displacement is discussed in the context of vector addition, where the resultant vector represents the total displacement after traveling along the individual vectors.
Highlights

Introduction to vector addition and resultants in 3D space.

Explanation of vector components for v and w in R3 space.

Visual representation of vector addition by moving vector w to the end of vector v.

Interpretation of the resultant vector as a straight line path.

Component-wise addition method for combining vectors in 3D space.

Definition of the resultant vector as the sum of two vectors.

Example calculation of vector addition for vectors v and w.

Illustration of the commutative property of vector addition.

Demonstration of vector addition order and its effect on the resultant.

Parallelogram representation of vector addition.

Explanation of equilibrium in the context of vector addition.

Calculation of an additional force to achieve equilibrium.

Concept of resultant force as a vector with all zero components.

Final problem involving the addition of multiple force vectors.

Method for finding the resultant of three given force vectors.

Discussion on the commutative nature of vector addition operations.

Conclusion and encouragement for further study on vector addition and resultants.

Transcripts

Browse More Related Video

Resultant vector | Vector addition: Grade 10 Physics

Displacement Vectors and Adding Multiple Vectors | Physics with Professor Matt Anderson | M3-04

How To Find The Resultant of Two Vectors

13 - Adding Two Vectors Graphically in Physics (Vector Sum & Resultant Vectors)

Vectors Full Topic -Physics

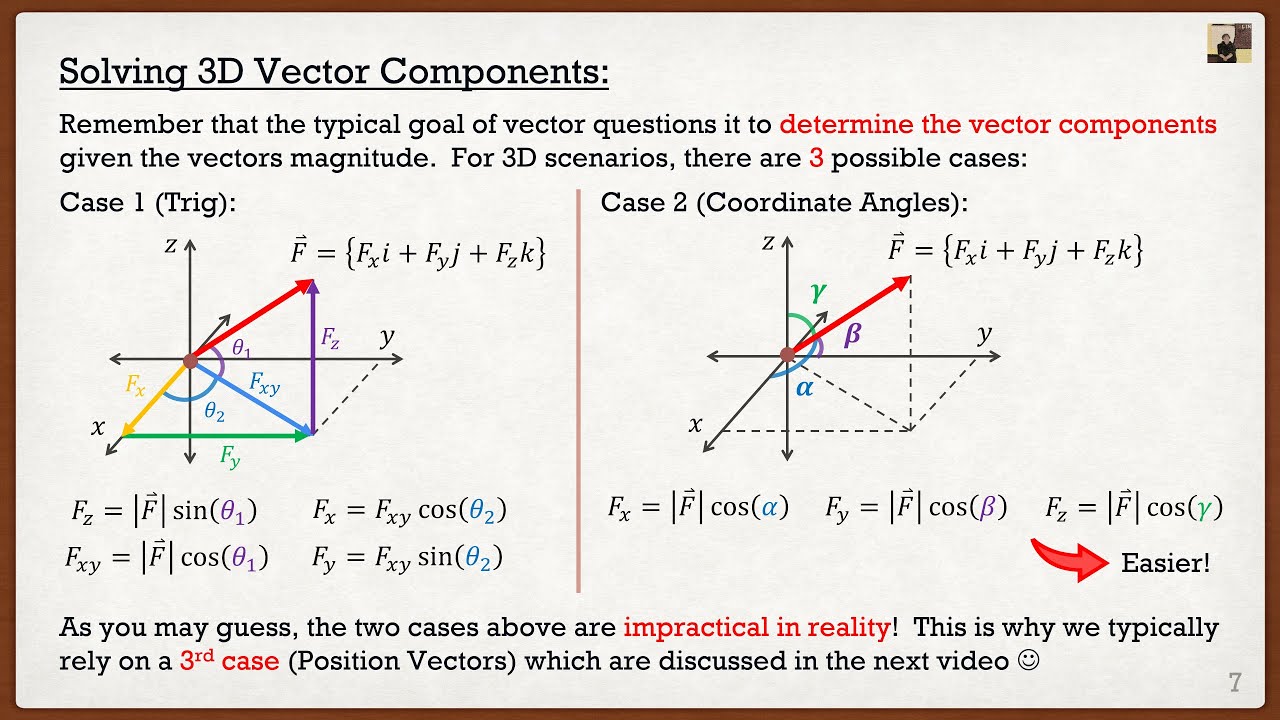
Engineering Mechanics: Statics Lecture 4 | Cartesian Vectors in 3D

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Vector Addition3D SpaceResultantsMath PrepEducationalVectorsComponent-wiseCommutativeEquilibriumForce Vectors