Calculus 3: Tangent Planes and Linear Approximation (Video #14) | Math with Professor V
TLDRThis calculus lecture delves into tangent planes and linear approximations for functions of multiple variables. It explains how tangent planes, like tangent lines in single-variable calculus, provide the best local fit to a surface at a given point. The process of finding a tangent plane's equation involves taking partial derivatives and using them as components of the normal vector. The lecture further explores linear approximations, which simplify complex functions into linear ones for ease of use, and demonstrates their application with examples. It concludes with a discussion on differentiability, highlighting that the existence of partial derivatives alone isn't sufficient, but their continuity at a point is what ensures differentiability.
Takeaways
- 📚 The primary use of derivatives for single-variable functions is to find the equation of the tangent line at a point on a curve, which provides close approximations to function values nearby.
- 📈 Tangent planes for surfaces serve a similar role as tangent lines for curves, fitting the surface well at the point of tangency and providing close approximations for nearby points.
- 🔍 To find the equation of a tangent plane, start by considering the surface's equation and the point of tangency with coordinates (X₀, Y₀, Z₀).
- 📐 The process involves finding the partial derivatives of the surface with respect to X and Y, which help determine the direction vectors of the tangent lines on the surface at the point of tangency.
- 🤖 The normal vector to the tangent plane is found by taking the cross product of the direction vectors of the tangent lines, evaluated at the point of tangency.
- 🧩 The equation of the tangent plane is then constructed using the normal vector components and the point of tangency, typically put into standard form.
- 📝 Differentials are used to approximate changes in function values for single-variable functions, and this concept is extended to multiple variables using the tangent plane.
- 📉 Linear approximations are useful for estimating function values when dealing with small changes in the input variables, and they rely on the tangent plane for functions of multiple variables.
- 🔢 The accuracy of linear approximations depends on the application and how sensitive the function is to changes in variables, with smaller changes generally leading to more accurate approximations.
- 🔍 To show differentiability for functions of two variables, one must express the change in the function (ΔZ) in terms of the partial derivatives and small changes in the input variables (ΔX, ΔY), along with error terms that approach zero.
- 📘 If the partial derivatives of a function exist and are continuous at a point, the function is differentiable at that point, simplifying the process of showing differentiability compared to using the limit definition.
Q & A
What is the primary purpose of tangent planes in the context of surfaces?
-Tangent planes serve a similar role to tangent lines for curves in functions of a single variable. They fit the surface near the point of tangency, providing close approximations to the function values near that point.
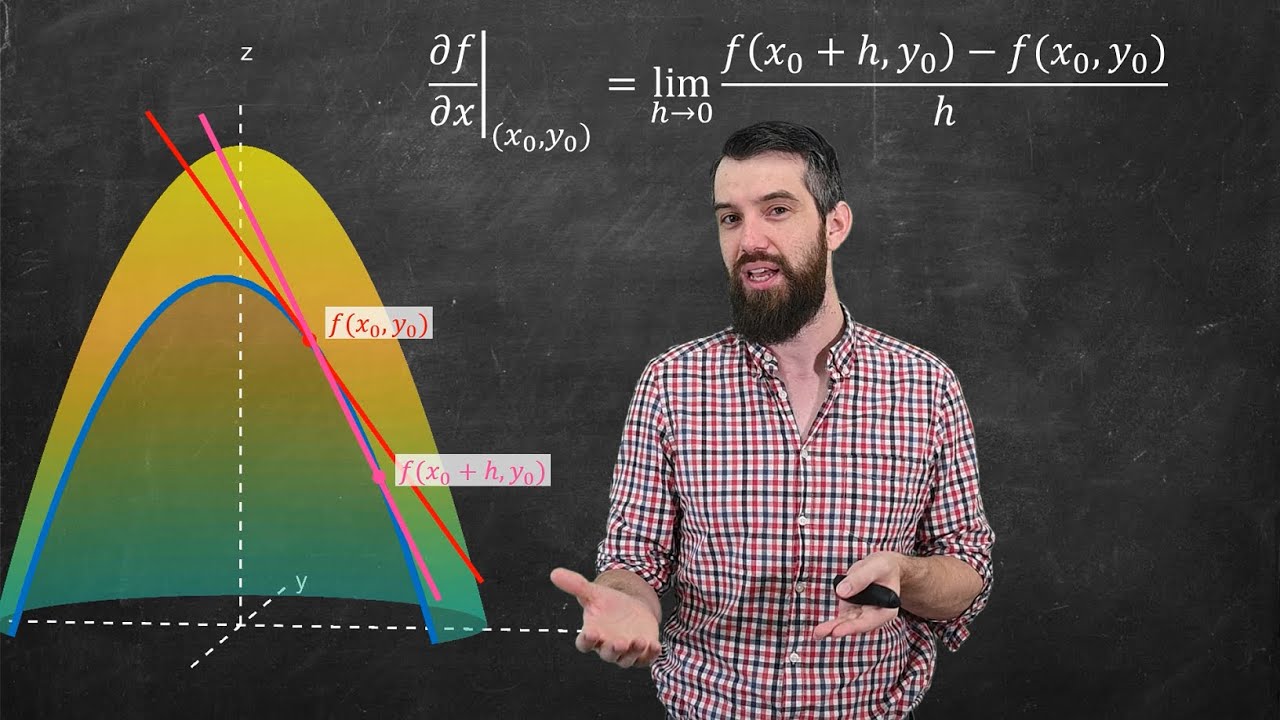
How does the tangent plane equation relate to the surface and its partial derivatives?
-The equation of the tangent plane is derived from the partial derivatives of the surface function with respect to x and y, evaluated at the point of tangency. It represents the best linear approximation of the surface at that point.
What is the process for finding the equation of a tangent plane to a given surface at a specific point?
-The process involves finding the partial derivatives of the surface function with respect to x and y at the point of tangency, using these to form a normal vector, and then using the normal vector to derive the equation of the tangent plane.
Can you explain the concept of linear approximation using the tangent plane?
-Linear approximation using the tangent plane involves using the tangent plane as a linear function to approximate the values of the original function of multiple variables near the point of tangency.
How is the differential of a function of multiple variables, denoted as DZ, related to the partial derivatives of the function?
-The differential DZ is the total change in the function and is approximated by the sum of the partial derivatives of the function with respect to each variable, multiplied by the respective increments in those variables (DX and DY).
What is the formula for the linearization L(X, Y) of a function F(X, Y) at a point (a, b)?
-The linearization L(X, Y) at a point (a, b) is given by F(a, b) + (∂F/∂X)(a, b) * (X - a) + (∂F/∂Y)(a, b) * (Y - b), where ∂F/∂X and ∂F/∂Y are the partial derivatives of F with respect to X and Y, respectively, evaluated at the point (a, b).
What does it mean for a function of two variables to be differentiable at a point?
-A function of two variables is differentiable at a point if the change in the function (ΔZ) can be expressed as the sum of its partial derivatives times the changes in the variables (ΔX and ΔY), plus higher-order terms that approach zero as ΔX and ΔY approach zero.
How can you show that a function is differentiable using its partial derivatives?
-A function is differentiable at a point if its partial derivatives with respect to all variables exist and are continuous at that point. This ensures that the function has a well-defined tangent plane, and the linear approximation is valid.
What is the significance of the error terms ε1 and ε2 in the definition of differentiability for functions of two variables?
-The error terms ε1 and ε2 represent the deviations of the actual change in the function (ΔZ) from the linear approximation. They are multiplied by the changes in the variables (ΔX and ΔY), and their significance lies in the fact that they approach zero as ΔX and ΔY approach zero, indicating that the linear approximation becomes more accurate.
Can you provide an example of how to use the linearization of a function to approximate a value?
-Sure. Given a function F(X, Y) = X^2 - 2XY + 3Y, to approximate F(1.03, 1.99), you would first find the linearization at a nearby point, say (1, 2), calculate the total differential DZ, and then add this to F(1, 2) to get the approximation. The result would be an estimate of the function's value at the new point.
Outlines
📚 Introduction to Tangent Planes and Linear Approximations
This paragraph introduces the concept of tangent planes and linear approximations in the context of calculus for functions of multiple variables. It compares the role of tangent lines for single-variable functions to tangent planes for surfaces. The paragraph explains how tangent planes provide close approximations of function values near a point of tangency, similar to how tangent lines do for curves. It sets the stage for deriving the equation of a tangent plane to a surface at a given point, using the partial derivatives of the function and the coordinates of the point of tangency.
🔍 Deriving the Equation of a Tangent Plane
The paragraph details the process of finding the equation of a tangent plane to a surface at a specific point. It begins by considering the surface defined by a function Z=f(X,Y) and a point of tangency P with coordinates (X₀, Y₀, Z₀). The method involves holding one variable constant to define curves on the surface, calculating the slopes of these curves at point P, and using these slopes as direction vectors for the tangent plane. The normal vector to the tangent plane is found by crossing these direction vectors, and the equation of the tangent plane is then formulated using this normal vector and the point of tangency.
📈 Linear Approximation and Differentials
This section discusses the application of linear approximation and differentials to functions of single and multiple variables. It starts by reviewing the use of differentials (dy) as an approximation for changes in a function's value (ΔY) for single-variable functions. The concept is then extended to functions of two variables, where the tangent plane is used to approximate the change in Z (ΔZ), and the total differential (DZ) is introduced as an approximation tool. An example is provided to demonstrate the use of differentials in approximating the square root of a number, highlighting the accuracy of the approximation when the change in the input variable is small.
📘 Extending Linear Approximations to Multivariable Functions
The paragraph extends the concept of linear approximation to functions of multiple variables. It explains how to use the tangent plane to approximate values on a surface near a given point. The linear approximation is expressed in different forms, emphasizing the components of the partial derivatives at the point of tangency. The paragraph also introduces the notation for the increment of Z (ΔZ) and the total differential (DZ), showing how they are used in approximations. An example is given to illustrate the process of approximating a function value using the total differential, which involves evaluating the function at a nearby point and adjusting based on the differential.
🔧 Linearization and Approximation Example
This paragraph provides an example of linearization and approximation for a function of two variables. It involves a function f(X,Y) and the process of finding its linearization at a specific point (a,b). The linearization is derived by evaluating the function and its partial derivatives at the point, and then using these to form the equation of the tangent plane, which serves as the linear approximation. The example concludes with using the linearization to approximate the function's value at a point close to the original point of linearization, demonstrating the application of the concept.
📉 Differentiability of Functions of Two Variables
The paragraph explores the concept of differentiability for functions of two variables. It starts by recalling the definition of differentiability for single-variable functions and then presents the analogous definition for functions of two variables. The function is differentiable at a point if the change in the function's value (ΔZ) can be expressed as a sum of the partial derivatives times the changes in the variables (ΔX, ΔY), plus error terms that approach zero as ΔX and ΔY approach zero. The paragraph includes an example that demonstrates showing differentiability by manipulating the function's change to match the required form, and then introduces a theorem that simplifies the process by stating that if the partial derivatives exist and are continuous at a point, the function is differentiable at that point.
📚 Summary of Differentiability and Continuity
The final paragraph summarizes the lesson on differentiability and continuity for functions of two variables. It emphasizes the cumbersome nature of proving differentiability using the limit definition and introduces a theorem that simplifies the process by stating that the existence and continuity of partial derivatives at a point are sufficient for differentiability. The theorem is applied to the previously discussed function to demonstrate its use. The paragraph concludes the lesson with a confirmation of the function's differentiability over the entire domain of R², given the continuity of polynomials.
Mindmap
Keywords
💡Tangent Plane
💡Partial Derivatives
💡Linear Approximation
💡Differentials
💡Normal Vector
💡Differentiability
💡Continuity
💡Error Bounds
💡Total Differential
💡Linearization
Highlights
Tangent planes to a surface serve a similar purpose to tangent lines to a curve, providing the best fit near the point of tangency.
The process to find the equation of a tangent plane involves holding one variable constant to find the slope of the tangent line on the surface.
Direction vectors for curves on the surface are key to finding the normal vector of the tangent plane.
The equation of the tangent plane is derived from the normal vector components evaluated at the point of tangency.
Differentials are used to approximate changes in functions, simplifying complex equations into linear approximations.
Linear approximations are particularly useful when dealing with small changes near the point of tangency.
The accuracy of linear approximations depends on the application and how sensitive the study is to errors.
Differentiability of a function of two variables requires the existence of partial derivatives and their continuity at a point.
A function is differentiable if the change in the function can be expressed as the sum of its partial derivatives times the changes in variables plus an error term that approaches zero.
The theorem that partial derivatives existing and being continuous at a point implies differentiability simplifies the process of proving differentiability.
Polynomial functions are continuous on their entire domain, which is crucial for proving differentiability.
The concept of linearization extends the idea of linear approximation from single-variable to multi-variable functions.
Linearization provides an approximation of a function's value at a point near a given point using the tangent plane.
The total differential, DZ, is used to approximate changes in multi-variable functions and is central to linearization.
The process of finding the equation of a tangent plane and linear approximation involves algebraic manipulation and understanding of partial derivatives.
Examples provided in the lecture demonstrate the step-by-step process of finding tangent planes and linear approximations.
The lecture concludes with practical applications and a deeper understanding of the theoretical concepts discussed.
Transcripts
Browse More Related Video

How to find the TANGENT PLANE | Linear approximation of multi-variable functions

Local linearization
What is a tangent plane

Calculus AB Homework 3.1 Derivative Rules

Calculus 3: Directional Derivatives and the Gradient Vector (Video #16) | Math with Professor V
What is differentiability for multivariable functions??
5.0 / 5 (0 votes)
Thanks for rating: