The Mode – The Most Frequently Occurring Measure of Central Tendency (5-2)

Research By Design
18 Aug 201605:21
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the concept of mode as a measure of central tendency, particularly suited for nominal data and unaffected by outliers. It contrasts unimodal and bimodal distributions, illustrating with examples like fishing peaks and overlapping IQ scores. The mode's advantage lies in its resistance to outliers, but it can be misleading if it doesn't represent the majority of data points. The script advises using the mode for nominal data, in bimodal distributions, or as an initial analysis tool for ordinal or scale variables.

Takeaways
  • 📊 The mode is the score that occurs most frequently in a data set and represents the central tendency for nominal level data.
  • 🔍 A unimodal distribution has one mode, indicating a single peak of frequency.
  • 📈 A bimodal distribution has two modes, suggesting two distinct peaks of frequency, which can occur naturally or due to overlapping distributions.
  • 🐟 An example of a natural bimodal distribution is the number of fish caught at dawn and dusk, with fewer caught at midday.
  • 📚 When comparing distributions, like average IQ scores from different schools, a bimodal result may indicate the need to analyze separate groups.
  • 🍦 The mode is particularly useful for nominal data, such as the most popular ice cream flavor in a survey.
  • 🔢 Calculating the mode often involves arranging scores in ascending order to identify the most frequent score(s).
  • 🔄 SPSS reports the smallest mode in a bimodal distribution and warns of multiple modes.
  • 🚫 The mode is unaffected by outliers, which is an advantage but also a limitation as it can be misleading if the mode does not represent the majority of the data.
  • 💯 The median is a more representative measure of central tendency when the mode is not indicative of the overall data distribution.
  • 📝 The mode should be reported when a single score dominates the distribution, especially with nominal data or in bimodal situations where the mean or median would be unrepresentative.
  • 📉 The mode is less useful when the most common score is zero, as it may not reflect the behavior of interest in the data set.
Q & A

  • What are the three measures of central tendency mentioned in the script?

    -The three measures of central tendency mentioned are the mean, the median, and the mode.

  • What is the mode in statistics?

    -The mode is the most frequently occurring score in a data set, representing the value with the highest frequency.

  • What is a unimodal distribution?

    -A unimodal distribution is one that has a single most frequently occurring score, indicating there is only one major peak in the data.

  • What is a bimodal distribution?

    -A bimodal distribution has two major peaks, meaning there are two modes, which are the two most frequently occurring scores.

  • Why is the mode commonly used with nominal level data?

    -The mode is commonly used with nominal level data because it is effective for identifying the most popular category or the most frequently chosen option in categorical data.

  • What are the two reasons for a bimodal distribution according to the script?

    -The two reasons for a bimodal distribution are: 1) There may genuinely be two modes in the data, such as different peaks for activities at different times of the day. 2) Two different distributions may be overlapping each other, such as comparing average scores from two distinct groups.

  • How does SPSS handle a bimodal distribution when calculating the mode?

    -When SPSS encounters a bimodal distribution, it reports the smallest mode and gives a warning that multiple modes exist.

  • What is the advantage of the mode over the mean in terms of outliers?

    -The mode is not susceptible to outliers at all, meaning any outlier, no matter how large, has no effect on the mode.

  • What is a disadvantage of the mode in terms of data representation?

    -A disadvantage of the mode is that it ignores most of the data by not considering scores other than the most frequent, which can lead to a mode that is not representative of the overall data set.

  • When is it appropriate to report the mode instead of the mean or median?

    -The mode should be reported when there is one particular score that dominates the distribution, when the data is nominal, or when the distribution is bimodal and the mean or median would be non-representative.

  • Why is the mode not useful when the most common score is 0?

    -The mode is not useful when the most common score is 0 because it doesn't provide information about the behavior of interest, such as the number of cigars smoked in a month, where the mode of 0 would not reflect the actions of those who have smoked.

  • How can the mode be used as an initial approach to ordinal or scale variables in a dataset?

    -The mode can be a quick and easy way to get a sense of ordinal or scale variables when first approaching a dataset, providing an initial insight into the most common values present.

Outlines
00:00
📊 Understanding Modes in Data Analysis

This paragraph introduces the concept of the mode as a measure of central tendency, which is the value that appears most frequently in a data set. It explains the distinction between unimodal and bimodal distributions, with examples like fish catching patterns and overlapping IQ score distributions. The mode is highlighted as particularly useful for nominal data, such as ice cream flavor preferences, and its calculation involves arranging data to identify the most frequent score. The paragraph also discusses the limitations of the mode, such as its insensitivity to outliers and its tendency to ignore most of the data. It concludes by advising when to use the mode, such as with nominal data or when the distribution is bimodal, and notes its advantages and disadvantages.

05:03
📘 Initial Exploration with Ordinal and Scale Variables

The second paragraph discusses the use of the mode for initial exploration of ordinal or scale variables in a dataset. It suggests that the mode can provide a quick and easy insight into the data, especially when first approaching a set. This is useful for gaining a preliminary understanding of the data distribution and identifying the most common scores or categories. The paragraph implies that while the mode may not be the final measure of central tendency for these types of data, it serves as a valuable initial tool in the analysis process.

Mindmap
Keywords
💡Mode
The mode is defined as the value that appears most frequently in a data set. It is a measure of central tendency that is particularly useful for nominal data, such as the most popular ice cream flavor in a class poll. In the script, the mode is used to illustrate how to identify the most common score or category in a distribution. The concept is further explored through examples of unimodal and bimodal distributions, where the script explains that a bimodal distribution has two modes, such as the peaks of fish caught at dawn and dusk, or potentially overlapping distributions like average IQ scores from different schools.
💡Central Tendency
Central tendency refers to a central or typical value for a set of data. The script introduces three measures of central tendency: mean, median, and mode. The concept is central to understanding data distribution and is used to summarize and describe the center of the data set. The video script discusses the mode as one of these measures, emphasizing its unique properties and appropriate use cases.
💡Unimodal Distribution
A unimodal distribution is characterized by having a single peak, where one score or value occurs more frequently than any other in the data set. The script mentions that this distribution has one mode, which is the most frequently occurring score. It is used to describe a typical distribution where there is a clear majority or most common element, such as a single popular ice cream flavor in a poll.
💡Bimodal Distribution
A bimodal distribution is one that has two peaks, indicating that there are two modes in the data set. The script uses the example of fish caught at dawn and dusk to illustrate a true bimodal distribution. It also discusses the possibility of a bimodal distribution occurring when two different groups' data overlap, such as average IQ scores from a state university and a community college.
💡Nominal Level Data
Nominal level data refers to categorical data where the variables are names or labels without any intrinsic order. The script explains that the mode is most commonly used with nominal data, such as determining the most popular ice cream flavor in a class. This type of data is analyzed using nonparametric tests, and the mode serves as an appropriate measure of central tendency for such data.
💡Outliers
Outliers are data points that are significantly different from other observations in the data set, often being much higher or lower. The script points out that the mode is not affected by outliers, as it only considers the most frequently occurring score. This is an advantage of the mode, but it can also be a disadvantage if the mode does not accurately represent the majority of the data.
💡Median
The median is another measure of central tendency that represents the middle value of a data set when it is ordered from least to greatest. The script contrasts the mode with the median, noting that the median can provide a more representative central value when the mode is misleading due to the presence of outliers or a skewed distribution.
💡Mean
The mean, or average, is the sum of all values in a data set divided by the number of values. The script discusses the mean as a measure of central tendency but also notes that it can be heavily influenced by outliers. In contrast to the mode, the mean considers all data points, which can be both an advantage and a disadvantage depending on the context.
💡Nonparametric Test
Nonparametric tests are statistical tests that do not assume a specific distribution of the data. The script mentions that nominal level variables are analyzed using nonparametric tests, which are appropriate for data that do not meet the assumptions required for parametric tests, such as normal distribution or equal variance.
💡Ordinal Data
Ordinal data is a type of data that has a natural order but does not provide information about the differences in magnitude between values. The script suggests that the mode can be a quick way to get a sense of ordinal or scale variables when first approaching a dataset, indicating that the mode can provide a basic understanding of the most common category or score.
💡Data Set
A data set refers to a collection of data, typically used for analysis and interpretation. The script uses the term 'data set' to refer to the group of scores or categories being analyzed, such as the ice cream flavors in a class poll or the number of fish caught by fishermen. The mode is calculated from the data set to identify the most frequent value.
Highlights

Three measures of central tendency are discussed: mean, median, and mode.

Mode is the most frequently occurring score in a dataset.

A unimodal distribution has only one major peak, indicating one mode.

A bimodal distribution has two major peaks, suggesting two modes.

Mode is commonly used with nominal level data for the most popular category.

Bimodal distributions can occur due to two distinct peaks or overlapping distributions.

Example of a true bimodal distribution: number of fish caught at dawn and dusk.

Bimodal distribution can result from overlapping distributions, like average IQ scores from different schools.

SPSS reports the smallest mode in a bimodal distribution and warns of multiple modes.

Mode is not affected by outliers, unlike mean and median.

Mode can be misleading if it does not represent the majority of the data.

Mode is less useful when the most common score is 0, like in the case of smoking behavior.

Mode should be reported when one score dominates the distribution, especially with nominal data.

Mode is the preferred measure of central tendency for nominal level variables and nonparametric tests.

Mode can provide a quick sense of ordinal or scale variables when first exploring a dataset.

Choosing the appropriate measure of central tendency depends on the nature of the data.

Mode is the central tendency measure of choice when variables are measured at the nominal level.

Transcripts

Browse More Related Video

Mean, Median and Mode - Measures of Central Tendency

Mean, Median, Mode, and Outliers: Measures of Central Tendency

Understanding Central Tendency

Data and the frequency distribution

SPSS: Understand Ordinal, Nominal & Scale (aka Level of measurment)

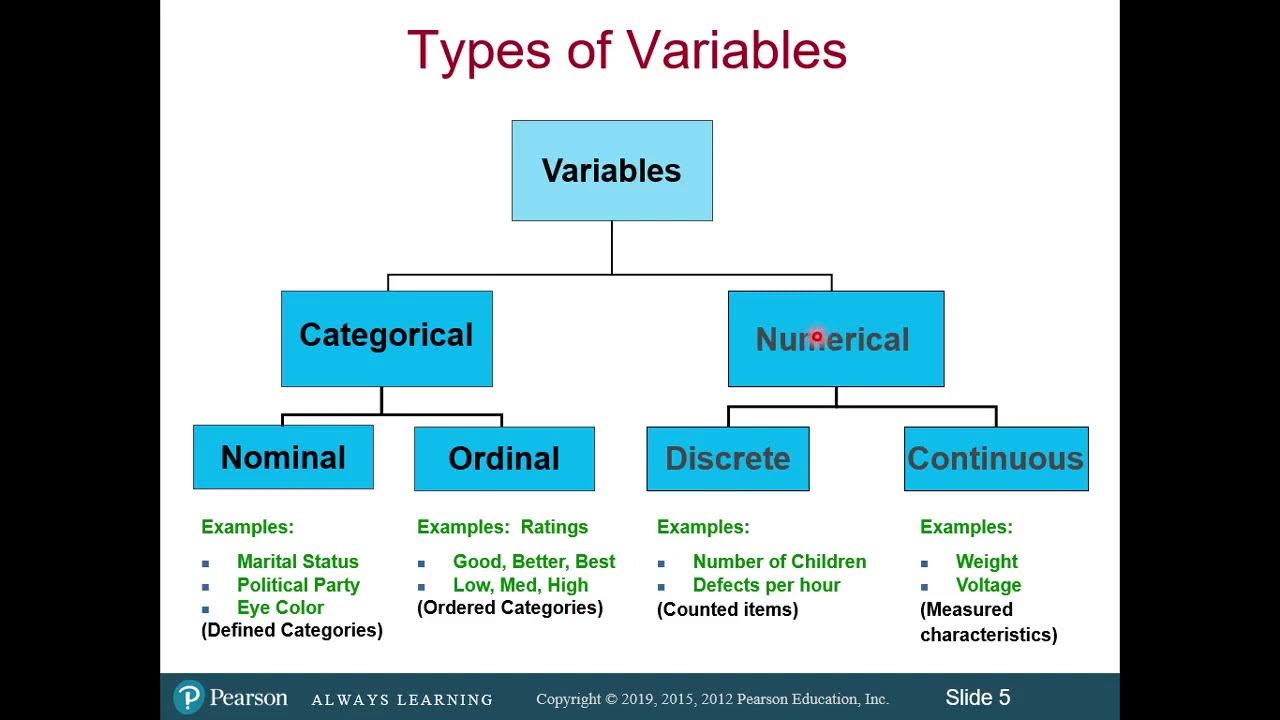
Classification of Variables and Types of Measurement Scales

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Mode AnalysisCentral TendencyData DistributionUnimodalBimodalOutliersStatistical MeasuresNominal DataDescriptive StatisticsData InterpretationSPSS Mode