How To Find The Median Of A Data Set

mrmaisonet
20 Mar 202003:47
EducationalLearning
32 Likes 10 Comments

TLDRThis instructional video teaches viewers how to determine the median of a data set, whether it's odd or even. For an odd set, the median is the single middle number; for an even set, it's the average of the two central numbers. The video demonstrates this with examples, showing how to order the data and calculate the median by averaging the central numbers if necessary. The presenter encourages viewers to subscribe and follow for more educational content.

Takeaways
  • 📊 The video discusses how to find the median of a data set, explaining the process for both odd and even number of data points.
  • 🔢 The example of an odd data set is given with seven numbers, which is ordered from least to greatest to find the median.
  • 🏁 The median of an odd data set is the single middle number, which in the example is six, as it has three numbers on either side.
  • 📈 The video then moves to an even data set, also ordered from least to greatest, with a pair of numbers in the middle.
  • 🔄 For an even data set, the median is found by taking the average of the two middle numbers, which in the example are seven and nine.
  • 🧮 The process of averaging is demonstrated by adding the two middle numbers together and dividing by two, resulting in eight as the median.
  • 📐 The video emphasizes that this method works regardless of the size of the numbers, as long as you add and divide by two.
  • 👉 The importance of ordering the data set before finding the median is highlighted as the first step in the process.
  • 📝 The video provides a clear explanation of the median concept, making it accessible for viewers to understand and apply.
  • 🌐 The presenter encourages viewers to follow them on social media, using the name 'Odinson', and to subscribe to their channel for more content.
  • 🎶 Background music is used throughout the video to engage the audience and maintain interest.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is teaching how to find the median of a data set, both when the data set is odd and even.

  • Why is the first data set in the video considered odd?

    -The first data set is considered odd because it contains seven numbers, and seven is an odd number.

  • What is the first step in finding the median of a data set?

    -The first step in finding the median is to order the data set from least to greatest.

  • How is the median determined for an odd data set?

    -For an odd data set, the median is the single middle number once the data set is ordered.

  • What is the median of the first data set in the video?

    -The median of the first data set is six, as it is the middle number after ordering the data set.

  • How does the process of finding the median change for an even data set?

    -For an even data set, you take the two middle numbers, calculate their average, and that average is the median.

  • What is the median of the second data set in the video?

    -The median of the second data set is eight, which is the average of the two middle numbers, seven and nine.

  • What is the method to find the median when the data set has two middle numbers?

    -To find the median in such cases, you add the two middle numbers together and divide the sum by two.

  • Why is the method of adding and dividing by two always correct for finding the median of two numbers?

    -This method is always correct because it mathematically finds the central point between the two numbers, regardless of their size.

  • What does the video suggest at the end for viewers to do?

    -The video suggests that viewers subscribe to the channel and follow the creator on social media under the name 'Odinson'.

Outlines
00:00
📊 Finding the Median of Odd and Even Data Sets

The video script introduces the concept of finding the median in a data set, emphasizing the difference between odd and even data sets. For an odd data set, exemplified by a set of seven numbers, the median is the middle number after arranging the data from least to greatest. The script demonstrates this with the number six, which is the balancing point with three numbers on either side. In contrast, for an even data set, there is no single middle number. The median is determined by averaging the two central numbers. The script illustrates this with a data set that, when ordered, has the numbers seven and nine as the middle pair, and their average, eight, is the median. The presenter also explains that this averaging method works regardless of the size of the numbers involved. The video concludes with a call to action for viewers to subscribe and follow the presenter on social media.

Mindmap
Keywords
💡Median
The median is the middle value in a data set when it is ordered from least to greatest. It is a measure of central tendency and is particularly useful when the data set is skewed. In the video, the concept of median is central to understanding how to find the central value in both odd and even data sets. For the odd data set, the median is the single middle number, which in the example given is six. For the even data set, the median is calculated by averaging the two middle numbers, which in the example is the average of seven and nine, resulting in eight.
💡Data Set
A data set is a collection of data points or values, which can be numbers, words, or observations. In the context of the video, the data sets are collections of numbers that are used to demonstrate how to find the median. The video provides examples of both odd and even data sets, highlighting the different methods required to find the median in each case.
💡Odd Data Set
An odd data set refers to a collection of numbers where the total count is an odd number. In the video, an example of an odd data set is given with seven numbers. The odd data set has a unique characteristic where the median is always the single middle number after the set is ordered. The video illustrates this with the number six being the median of the odd data set.
💡Even Data Set
An even data set is a collection of numbers with an even total count. The video explains that when dealing with an even data set, there is no single middle number. Instead, the median is found by averaging the two middle numbers after the data set is ordered. The example in the video shows an even data set with the numbers two, three, five, seven, nine, and ten, where the median is calculated as the average of seven and nine, which is eight.
💡Ordering
Ordering in the context of the video refers to arranging the numbers in a data set from the smallest to the largest value. This is a necessary step before finding the median, as the position of each number determines whether it is the median or part of the calculation for the median in even data sets. The video demonstrates this process with both the odd and even data sets provided.
💡Balancing Point
The term 'balancing point' in the video is used metaphorically to describe the median of an odd data set. It refers to the single middle number that has an equal number of values on either side. The video uses the example of the number six, which is the balancing point of the odd data set with seven numbers.
💡Middle Number
The middle number is the value that is exactly in the center of a data set when it is ordered. For an odd data set, there is one middle number that is the median. The video explains that for the odd data set example, the middle number, and thus the median, is six.
💡Averaging
Averaging is the process of finding the mean of two or more numbers by adding them together and then dividing by the count of the numbers. In the context of the video, averaging is used to find the median of an even data set. The two middle numbers of the data set are added together and then divided by two to find the median, as demonstrated with the numbers seven and nine resulting in eight.
💡Central Tendency
Central tendency refers to a measure that represents a typical or central value of a data set. The median is one such measure, and it is particularly useful when the data set is not symmetrically distributed. The video's theme revolves around finding the median, which is a central tendency measure, to understand the central value of different data sets.
💡Skewed Data
Skewed data refers to a data set where the distribution of values is not symmetrical, often with a longer tail on one side. While the video does not explicitly mention skewed data, the concept of the median as a measure of central tendency is relevant because the median is less affected by outliers or skewness in the data compared to other measures like the mean.
💡Outliers
Outliers are data points that are significantly different from other values in the data set, often causing skewness. The video does not directly discuss outliers, but understanding the median as a robust measure of central tendency is important because it is less influenced by outliers compared to the mean, making it a reliable indicator of the data's central value.
Highlights

Introduction to the concept of finding the median in a data set.

Explanation of an odd data set with seven numbers as an example.

Step-by-step process of ordering a data set from least to greatest.

Identifying the median in an odd data set as the middle number.

Demonstration of finding the median in an odd data set with the number six.

Transition to understanding the median in an even data set.

Ordering an even data set and identifying the lack of a single middle number.

Method of finding the median in an even data set by averaging the two middle numbers.

Example of calculating the median with the numbers seven and nine.

General formula for finding the median in an even data set: (number1 + number2) / 2.

Emphasizing the universality of the median calculation method regardless of number size.

Illustration of the median calculation with a practical example.

Reinforcement of the median calculation method's accuracy and reliability.

Encouragement to subscribe to the channel for more educational content.

Invitation to follow the creator on social media for updates and interaction.

Closing remarks with thanks for watching the video on median calculation.

Transcripts

Browse More Related Video

Finding the Median | Math with Mr. J

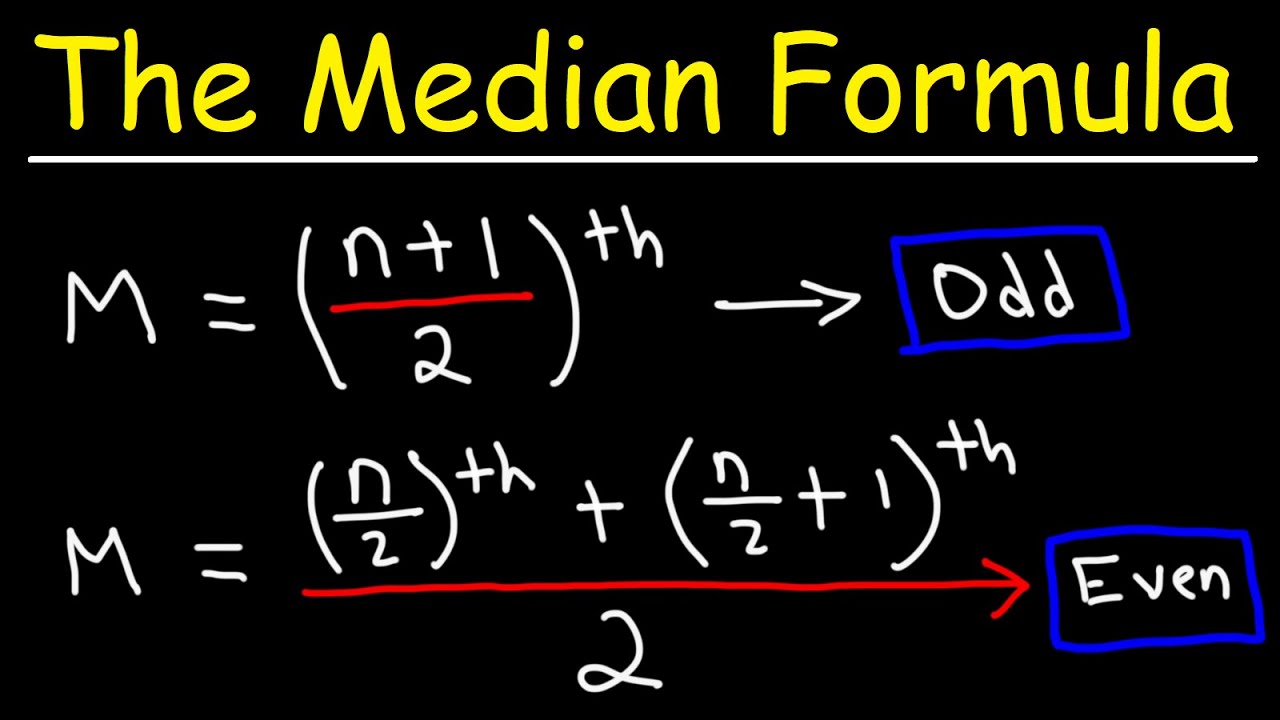
How To Calculate The Median Using a Formula - Statistics

How do we Find the Median? | Don't Memorise

How to calculate interquartile range IQR | Data and statistics | 6th grade | Khan Academy

How to Find the Median | Math with Mr. J

Finding mean, median, and mode | Descriptive statistics | Probability and Statistics | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Median CalculationData AnalysisOdd SetEven SetStatisticsEducationalMathematicsTutorialNumerical DataOdison