How do we Find the Median? | Don't Memorise

Infinity Learn NEET
19 Dec 201403:03
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explains the concept of the median in a set of numbers. It clarifies that the median is the middle value, which can be easily identified when the set is arranged in ascending order. For an odd number of terms, the median is the central term, exemplified with a set of seven numbers where the fourth term is the median. For an even number of terms, the median is the average of the two central values, as demonstrated with a set of eight numbers where the median is calculated as the average of the fourth and fifth terms. The script also highlights the general formula for finding the median position in terms of the total number of terms, n.

Takeaways
  • 🔢 The median is the middle or central value in a set of numbers, found after arranging the numbers in ascending order.
  • 📈 To identify the median, the numbers must first be ordered from least to greatest.
  • 🔑 If there is an odd number of terms, the median is the middle term, as illustrated by the set with seven terms, where the fourth term is the median.
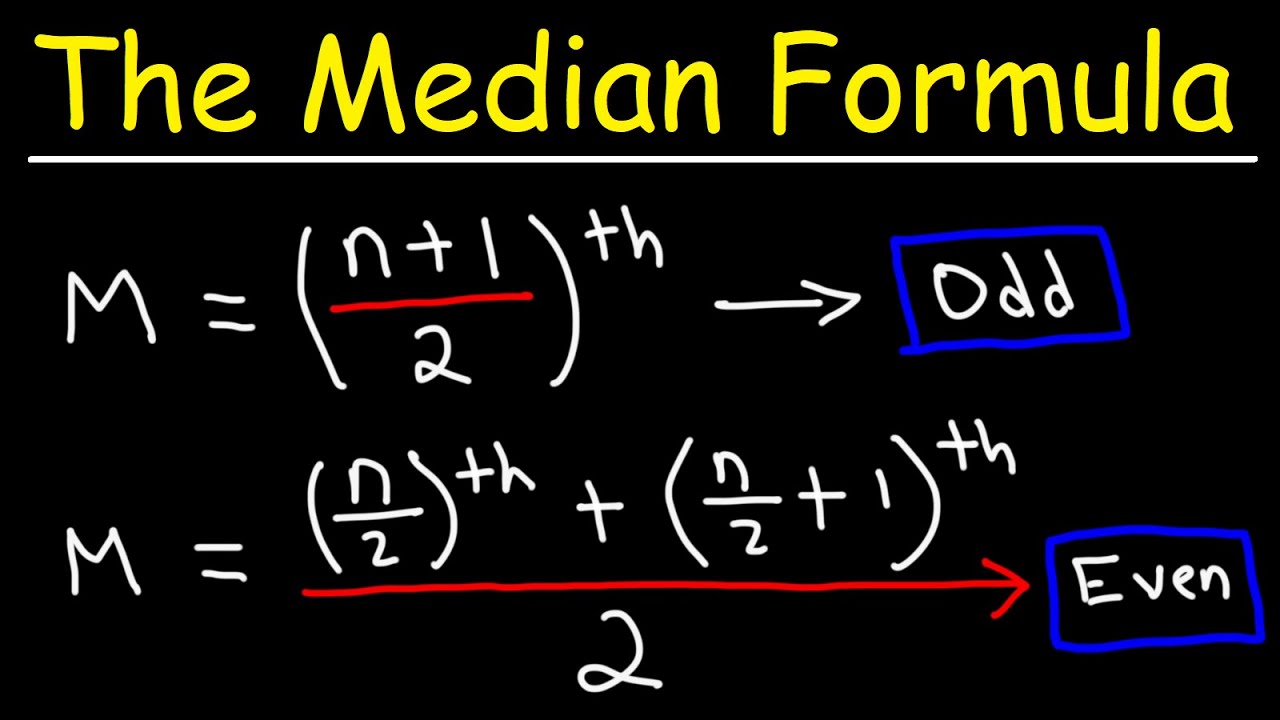
  • 🔢 The formula for finding the median position in an odd-numbered set is n + 1 / 2, where n is the total number of terms.
  • 🤔 The formula for the median position only applies to sets with an odd number of terms.
  • 📊 For even-numbered sets, the median is the average of the two central terms, as shown with the set of eight terms where the median is the average of 15 and 17.
  • 🔍 In an even-numbered set, the median is not a single term but the mean of the n/2th and (n/2 + 1)th terms.
  • 📐 The script provides a clear example of how to calculate the median for both odd and even sets of numbers.
  • 📝 The importance of arranging numbers in ascending order before finding the median is emphasized throughout the script.
  • 📈 The script explains the concept of the median in a step-by-step manner, making it easy to understand for viewers.
  • 📚 The transcript serves as a teaching tool for understanding the concept of medians in statistics.
Q & A

  • What is the median in a set of numbers?

    -The median is the middle value in a set of numbers that have been arranged in ascending order. It represents the central tendency of the data set.

  • How do you find the median of a set with an odd number of terms?

    -For a set with an odd number of terms, the median is the value that has an equal number of terms on either side of it when the set is arranged in ascending order.

  • What is the median of the number set 2, 4, 6, 12, 12, 16, and 18?

    -The median of this set is 12, as it is the fourth term when the numbers are arranged in ascending order, which has three terms on each side.

  • How can you determine the position of the median in a set with an odd number of terms?

    -In a set with an odd number of terms, the median is the (n+1)/2 term, where n is the total number of terms in the set.

  • What happens to the median when the number of terms in the set is even?

    -When the number of terms is even, the median is the average of the two middle terms in the set after arranging the numbers in ascending order.

  • Can the formula for finding the median position be applied to sets with an even number of terms?

    -No, the formula (n+1)/2 only works for sets with an odd number of terms. For even-numbered sets, the median is the average of the two central terms.

  • What is the median of the number set 7, 7, 14, 15, 17, 24, 29, and 42?

    -The median is 16, which is the average of the two middle terms, 15 and 17, in the set arranged in ascending order.

  • How do you find the positions of the two middle terms in a set with an even number of terms?

    -In a set with an even number of terms, the two middle terms are the (n/2) and (n/2 + 1) terms, where n is the total number of terms in the set.

  • Why is the median considered a better measure of central tendency than the mean in some cases?

    -The median is less affected by outliers or extreme values in the data set, making it a more robust measure of central tendency when the data is skewed.

  • What is the significance of arranging numbers in ascending order before finding the median?

    -Arranging numbers in ascending order ensures that the terms are in a sequence that allows for the identification of the middle value or the average of the two middle values, which is essential for calculating the median.

Outlines
00:00
🔢 Finding the Median of a Set of Numbers

To find the median of a set of numbers, first arrange the numbers in ascending order. The median is the middle value in the ordered set. For a set with an odd number of terms, the median is the middle term. In this example, with the set 2, 4, 6, 12, 12, 16, and 18, the median is the fourth term, which is 12. The position of the median can be found using the formula (n + 1) / 2, where n is the number of terms in the set. This formula only works for sets with an odd number of terms.

⚖️ Finding the Median in Even Sets

For sets with an even number of terms, finding the median involves averaging the two central terms. After arranging the numbers in ascending order, for example, 7, 7, 14, 15, 17, 24, 29, and 42, the two central terms are 15 and 17. The median is the average of these terms, calculated as (15 + 17) / 2, resulting in 16. The general formula to find the median in an even set is to take the average of the n/2 term and the (n/2 + 1) term.

Mindmap
Keywords
💡Median
The median is a statistical measure that represents the middle value of a dataset when it is ordered from least to greatest. In the video, the median is the central concept, as it is used to explain how to find the middle value in both odd and even sets of numbers. For instance, in the set with seven numbers, the median is the fourth term, which is 12, as it has an equal number of terms on either side.
💡Ascending Order
Ascending order refers to the arrangement of numbers from the smallest to the largest. In the context of the video, arranging the numbers in ascending order is a prerequisite for finding the median. This is exemplified when the set of numbers 2, 4, 6, 12, 12, 16, and 18 is ordered to find the median, which is 12.
💡Central Value
The central value is another term for the median in a dataset. It is the value that divides the data into two equal halves. The video emphasizes the importance of the central value in understanding the distribution of data, as it is the middle term in an ordered set with an odd number of terms.
💡Odd Number of Terms
When a dataset contains an odd number of terms, the median is the exact middle value. The video script uses the example of a set with seven terms to illustrate this, where the fourth term, 12, is the median because seven is an odd number.
💡Even Number of Terms
In contrast to an odd number of terms, when a dataset has an even number of terms, the median is calculated as the average of the two central values. The video script demonstrates this with a set of eight terms, where the median is the average of the fourth and fifth terms, 15 and 17, resulting in 16.
💡Average
The average, or mean, is calculated by adding all the values in a dataset and then dividing by the number of values. In the context of the video, the average is used to find the median when the dataset has an even number of terms, as seen with the set where the median is the average of 15 and 17.
💡Data Distribution
Data distribution refers to the way in which values in a dataset are spread out. The video discusses how the median helps understand the distribution, especially when the data is ordered, as it provides insight into the central tendency of the dataset.
💡Term
In the context of the video, a term refers to an individual value within a dataset. The script explains how to identify the median by referring to the position of terms in an ordered set, such as the 'fourth term' or 'fifth term' being the median in different scenarios.
💡Statistical Measure
A statistical measure is a numerical value that represents some aspect of a dataset. The median is one such measure, and the video focuses on it as a way to summarize and understand the central tendency of a dataset.
💡Ordered Set
An ordered set is a collection of numbers arranged in a specific sequence, such as ascending or descending order. The video emphasizes the importance of ordering a set to determine the median accurately.
💡Central Tendency
Central tendency is a measure that describes the center point of a dataset. The median is a central tendency measure, and the video explains how it represents the middle of the data, which is crucial for understanding the overall distribution.
Highlights

The median is the middle or central value in a set of numbers.

To find the median, numbers must be arranged in ascending order.

In a set with an odd number of terms, the median is the middle term.

The example set 2, 4, 6, 12, 12, 16, and 18 has 12 as the median.

The formula for finding the median in an odd set is the (n+1)/2 term.

For an even number of terms, the median is the average of the two central terms.

The set with eight terms, 7, 7, 14, 15, 17, 24, 29, and 42, has a median of 16.

The median in an even set is the average of the n/2 and n/2 + 1 terms.

The median is not always a single term but can be the average of two terms.

The concept of the median is important for understanding central tendencies in data sets.

Arranging numbers in ascending order is a prerequisite for median calculation.

The median can be found using a simple formula based on the number of terms in the set.

The median is a measure of central tendency that is robust to outliers.

Understanding the median helps in summarizing and interpreting data effectively.

The median is a key statistical measure used in various fields of study.

The example sets illustrate the process of finding the median in both odd and even number of terms.

The median calculation method varies depending on whether the set has an odd or even number of terms.

The median is a fundamental concept in statistics with practical applications.

Transcripts

Browse More Related Video

How To Calculate The Median Using a Formula - Statistics

Finding the Median | Math with Mr. J

How To Find The Median Of A Data Set

Finding mean, median, and mode | Descriptive statistics | Probability and Statistics | Khan Academy

How to calculate interquartile range IQR | Data and statistics | 6th grade | Khan Academy

Why do we Need the Median? - Example | Don't Memorise

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Median CalculationStatistical AnalysisData ArrangementOdd SetEven SetCentral ValueMathematics TutorialNumber OrderingAverage MedianEducational Content