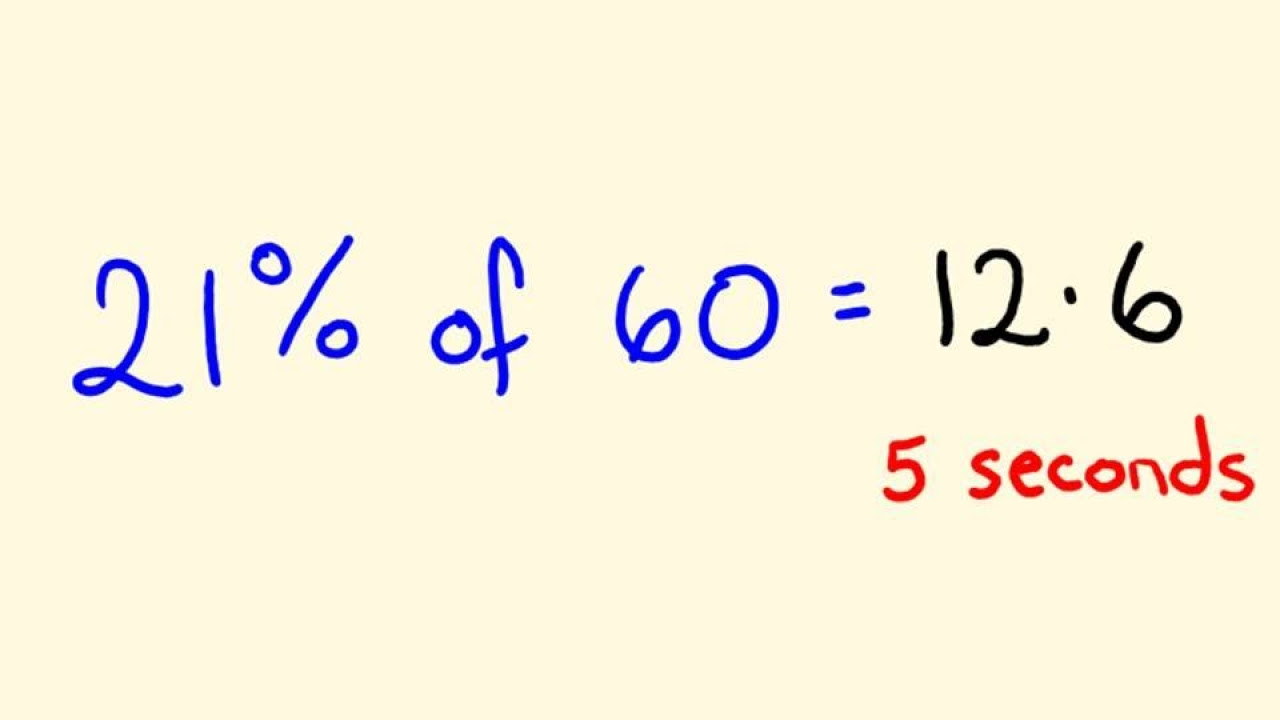Percentage Trick - Solve precentages mentally - percentages made easy with the cool math trick!
TLDRThis Tech Math video offers a straightforward method for calculating percentages mentally, starting with simple percentages like 15%, 25%, 50%, 75%, and 95%. The host explains that percentages are out of 100, and demonstrates quick calculations using 100%, 50%, 10%, and 5% of a number. By breaking down percentages into these components and practicing mental math, viewers can rapidly find answers like 15% of 80 or 95% of 720. The video encourages practice and promises a follow-up to tackle more complex percentages.
Takeaways
- 📚 The video is an introduction to calculating percentages mentally, starting with simple percentages like 15%, 25%, 50%, 75%, and 95%.
- 🔢 A percentage is a number out of 100, with 'per' meaning 'out of' and 'cent' meaning '100'.
- 💡 To calculate a percentage of a number, understand that 100% of a number is the number itself, 50% is half, 10% is one-tenth, and 5% is half of 10%.
- 🧠 The method involves breaking down percentages into 10% and 5% portions to simplify mental calculations.
- 📈 For example, calculating 15% of a number involves finding 10% and then adding 5% of the same number.
- 📉 Similarly, for a percentage like 95%, you calculate 100% and then subtract 5%.
- ✅ The video provides examples, such as calculating 15% of 80, which is 12, by adding 10% (8) and 5% (4) of 80.
- 📝 Practice is encouraged to improve mental calculation skills, with viewers prompted to try examples like 60% of 42 or 95% of 720.
- 🤔 The script suggests that some percentages, like 85%, may be more challenging to calculate mentally due to the complexity of the numbers involved.
- 🔄 The process involves both addition and subtraction, depending on whether you're calculating a percentage of a number or subtracting a percentage from a whole.
- 👨🏫 The presenter offers further guidance in subsequent videos for more complex percentages, such as 22%, 53%, and 87%.
Q & A
What is the main topic of the video?
-The main topic of the video is to teach viewers how to calculate percentages mentally, starting with simple percentages like 15%, 25%, 50%, 75%, and 95%.
What does the word 'percentage' literally mean?
-The word 'percentage' literally means 'per hundred', with 'per' meaning 'out of' and 'cent' meaning 'hundred'.
How do you calculate 100% of a number?
-100% of a number is the actual number itself. For example, 100% of 80 is 80.
What is 50% of a number?
-50% of a number is half of the number. For instance, 50% of 80 is 40.
How can you find 10% of a number?
-10% of a number is found by dividing the number by 10. For example, 10% of 80 is 8.
What is 5% of a number in relation to 10% of the same number?
-5% of a number is half of 10% of the same number. So, if 10% of a number is X, then 5% of the number is X/2.
How can you mentally calculate 15% of a number?
-To mentally calculate 15% of a number, you can find 10% of the number and then add 5% of the same number to it.
In the video, what method is used to calculate 60% of 42?
-The method used to calculate 60% of 42 in the video is by finding 50% (which is half of 42) and then adding 10% of 42 to it.
How is 95% of a number calculated in the video?
-95% of a number is calculated by taking 100% of the number and then subtracting 5% from it.
What is the hint given for calculating 25% of a number?
-The hint given for calculating 25% of a number is that 25% is the same as dividing the number by four, since 25% is a quarter of the whole.
What is the next step after learning to calculate simple percentages mentally?
-The next step, as mentioned in the video, is to learn how to calculate more complex percentages, such as 22%, 53%, or 87%, which are not as easily divisible by five.
Outlines
📚 Introduction to Mental Percentage Calculation
The video begins with an introduction to the concept of percentages and mental calculation techniques. The host explains that percentages are numbers out of 100 and introduces the idea of calculating simple percentages like 15%, 25%, 50%, 75%, and 95% of a number. The method involves breaking down the percentage into parts of 100%, 50%, 10%, and 5%, and then mentally adding or subtracting these parts to find the result. The host demonstrates this with examples like calculating 15% of 80 and 60% of 42, emphasizing the importance of understanding the basic components of percentages.
🧠 Advanced Mental Calculation Techniques
This paragraph delves into more complex mental calculation techniques for percentages. The host challenges viewers with examples such as calculating 95% of 720, which involves understanding 100% of a number and then subtracting a percentage from it. The method of moving the decimal place to find 10% of a number and then halving it to find 5% is reiterated. The host provides additional practice problems, such as calculating 55% of 360, 25% of 860, and 85% of 620, encouraging viewers to attempt these mentally and then check their answers. The emphasis is on practice to improve mental calculation skills for various percentages.
🚀 Moving Beyond Basic Percentages
In the final paragraph, the host looks forward to the next video, where the focus will shift from basic percentages divisible by fives and tens to more complex percentages like 22%, 53%, and 87%. The host acknowledges that some numbers are trickier to calculate mentally and that practice is key to mastering these skills. The video concludes with an invitation for viewers to continue learning and improving their mental math abilities, with a promise of more advanced techniques in upcoming videos.
Mindmap
Keywords
💡Percentage
💡Mental Calculation
💡100%
💡50%
💡10%
💡5%
💡Addition
💡Subtraction
💡Divisibility
💡Practice
💡Complex Percentages
Highlights
Introduction to a mental math technique for calculating percentages with ease.
Explanation of the meaning of 'percentage', which is a number out of 100.
Method for quickly calculating simple percentages like 15%, 25%, 50%, 75%, and 95%.
Understanding that 100% of a number is the number itself.
How to calculate 50% of a number, which is half of the original number.
Technique for finding 10% of a number by dividing by 10.
Calculating 5% of a number by taking half of 10%.
Combining 10% and 5% to find 15% of a number.
Example calculation: 15% of 80 results in 12.
Method for calculating 60% of a number by using 50% and 10%.
How to calculate 95% of a number by subtracting 5% from 100%.
Practical examples provided for mental math practice.
Encouragement to practice mental math calculations on paper and then compare with the provided method.
Additional practice problems presented for viewers to try: 55% of 360, 25% of 860, and 85% of 620.
Explanation of how to calculate 55%, 25%, and 85% using the taught method.
The importance of practice in mastering mental math calculations.
Upcoming video teaser on tackling more complex percentages beyond those divisible by five.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: