4.4.0 Counting - Lesson Overview, Learning Outcomes, and Key Concepts
TLDRThis video offers an overview of Lesson 4.4, focusing on combinatorics, a branch of mathematics that deals with counting. The lesson is based on slides provided by Pearson and modified by the instructor for the course. It covers five counting methods: the multiplication rule, factorial rule, permutations, permutations with identical objects, and combinations. The video emphasizes the importance of accurate counting for calculating probabilities, using the classical approach. The instructor assures students that while formulas will be provided, understanding the principles behind them is crucial for real-world applications.
Takeaways
- 📚 The video provides an overview of Lesson 4.4, which focuses on counting techniques, often referred to as combinatorics.
- 📑 The slides for this lesson are based on Pearson's materials but have been modified by the speaker for their course.
- 🕒 Due to time constraints, only the first two sections of Chapter 4 are covered, which include basic probability concepts and the addition and multiplication rules.
- 🔢 Section 4.4 specifically discusses counting the number of outcomes, which is crucial for applying the ideas from previous sections.
- 📘 The lesson is from the 'Essentials of Statistics' textbook, 6th edition, by Mario Triola.
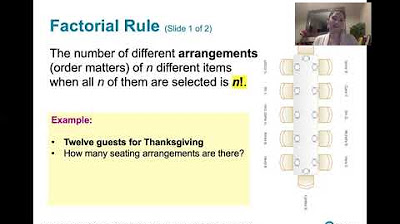
- 📝 Learning outcomes include understanding five counting methods: the multiplication counting rule, factorial rule, permutations, permutations with identical objects, and combinations.
- 🎯 The probability of an event is calculated by dividing the number of ways the event can occur by the total number of possible outcomes.
- 📈 To apply probability formulas accurately, one must be able to count the number of outcomes for both the numerator and the denominator.
- 📚 The section involves more formulas than previous ones, but students are not expected to memorize them as they will be provided during exams.
- 🤓 The speaker emphasizes understanding the counting principles behind the formulas rather than just memorizing them.
- 🎓 The speaker mentions having taken a combinatorics course as an undergraduate, noting its difficulty and the importance of not overcounting.
- 🌐 The lesson aims to teach students how to count outcomes accurately for real-world applications and probability calculations.
Q & A
What is the main topic of Lesson 4.4 in the video?
-The main topic of Lesson 4.4 is counting, also known as combinatorics, which focuses on the methods for counting the number of outcomes in various scenarios.
What are the five methods for counting that are covered in the video?
-The five methods for counting covered in the video are the multiplication counting rule, the factorial rule, permutations, permutations with identical objects, and combinations.
Why is counting the number of outcomes important in probability?
-Counting the number of outcomes is important in probability because it allows for the calculation of the probability of an event by dividing the number of ways an event can occur by the total number of possible outcomes.
What is the source of the slides used in the video?
-The slides used in the video are based on slides provided by Pearson but have been modified by the instructor for their course.
Which textbook does the video refer to for its content?
-The video refers to the textbook 'Essentials of Statistics' by Mario Triola, specifically the sixth edition.
What is the instructor's approach to teaching the counting formulas in the video?
-The instructor does not expect students to memorize the counting formulas, as they will be provided during the exam. Instead, the focus is on understanding the counting principles behind the formulas.
What is the significance of understanding the counting principles behind the formulas?
-Understanding the counting principles behind the formulas helps students to know where the formulas come from, which enables them to select the appropriate formula for a given problem without blindly applying it.
Why does the instructor emphasize not wanting students to blindly apply formulas?
-The instructor emphasizes this to ensure that students have a deeper understanding of the concepts, which allows for better problem-solving and application of knowledge in various situations.
What is the relationship between permutations and combinations in the context of this video?
-Permutations and combinations are the core counting methods discussed in the video. Understanding these concepts is key to solving most real-world counting problems related to probability.
What is a special case of permutations discussed in the video?
-A special case of permutations discussed in the video is permutations with identical objects, where the order of arrangement matters but some objects are indistinguishable from each other.
How does the instructor plan to help students avoid common counting mistakes like counting things twice?
-The instructor plans to teach the counting principles and methods thoroughly, enabling students to understand and apply the correct formulas, thus reducing the chances of counting errors.
Outlines
📚 Introduction to Lesson 4.4 on Counting
This paragraph introduces Lesson 4.4, which focuses on counting, also known as combinatorics. The content is based on slides provided by Pearson and modified by the speaker for their course. The lesson is part of the last section in Chapter Four, and due to time constraints, only the first two sections are covered, including basic probability concepts and the addition and multiplication rules. The main goal of this section is to teach students how to count the number of outcomes, which is essential for applying the ideas from previous sections. The learning outcomes include five counting methods, one of which is a special case of another. The speaker emphasizes the importance of understanding the counting principles behind the formulas rather than memorizing them, aiming for students to apply the formulas correctly and not blindly.
Mindmap
Keywords
💡Combinatorics
💡Permutations
💡Multiplication Rule
💡Factorial
💡Identical Objects
💡Combinations
💡Probability
💡Essentials of Statistics
💡Learning Outcomes
💡Tear Out Card
💡Classical Approach
Highlights
Lesson 4.4 provides an overview of counting, also known as combinatorics.
The slides are based on Pearson's materials and modified for the course.
Only the first two sections of Chapter 4 are covered due to time constraints.
Section 4.4 focuses on counting the number of outcomes, a crucial aspect of applying probability concepts.
The text is from 'Essentials of Statistics' by Mario Triola, 6th edition.
Learning outcomes include five methods for counting, with one being a special case.
Students will learn the multiplication counting rule, factorial rule, and permutation concepts.
Permutations with identical objects and combinations are also covered.
Counting outcomes is essential for calculating probabilities using the classical approach.
The video series will explore the five counting methods in detail.
The counting methods involve more formulas than previous sections.
Formulas will be provided during exams, so memorization is not required.
Understanding the counting principles behind the formulas is encouraged.
The instructor emphasizes the importance of not blindly applying formulas.
The course includes intuitive reasons behind the formulas for better understanding.
The instructor took a challenging combinatorics course as an undergraduate.
The section covers a couple of counting methods, focusing on permutations and combinations.
Understanding permutations and combinations is key to most real-world counting applications.
The lesson aims to teach accurate counting for probability calculations.
The overview concludes with an introduction to the next video in the series.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: