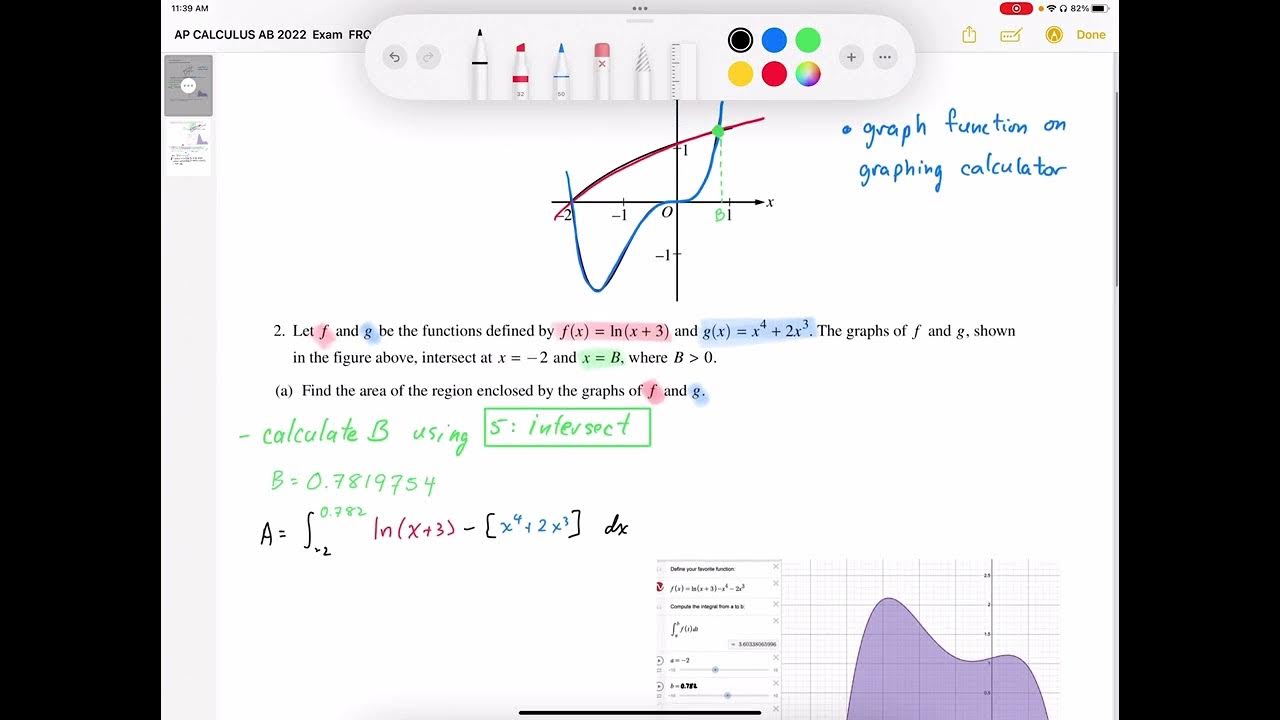
AP Precalculus Practice Exam Question 24

NumWorks
23 May 202304:09
EducationalLearning
32 Likes 10 Comments

TLDRThe video script details the process of finding the intersection points of two logarithmic functions, f(x) and g(x). The presenter starts by setting the functions equal and using the product property of logarithms to combine them. After simplifying, they obtain a quadratic equation, which they solve to find potential solutions. However, they emphasize the importance of checking the domain of logarithmic functions, discarding the extraneous solution of x = -4, and concluding that the valid intersection point is at x = 3.

Takeaways
  • 📚 The problem involves finding the intersection points of two functions, f(x) and g(x), which are both logarithmic with different bases and arguments.
  • 🔍 To find the intersection points, the script sets f(x) equal to g(x) and uses the properties of logarithms to simplify the equation.
  • 📘 The product property of logarithms is applied to combine the two logs on the left side of the equation into a single logarithmic expression.
  • 📙 The script multiplies the arguments of the combined logarithm, resulting in an expression that is equivalent to the logarithm of the sum of x and 9.
  • 📗 The multiplication of (x - 1) and (x + 3) is expanded to form a quadratic expression inside the logarithm.
  • 📒 The script then raises both sides of the equation to the base 10 to eliminate the logarithm, resulting in a quadratic equation.
  • 📕 The quadratic equation is simplified to x^2 + 4x - 12, which is then factored into (x + 3)(x - 4).
  • 📔 The solutions to the quadratic equation are x = 3 and x = -4, but the latter is an extraneous solution due to the domain restrictions of logarithmic functions.
  • 📓 The domain of logarithmic functions requires the argument to be positive, thus x = -4 is discarded as it would result in a negative argument for the logarithm.
  • 📔 The only valid solution for the intersection point is when x = 3, as it satisfies the domain requirements of both f(x) and g(x).
  • 📘 The process highlights the importance of checking the domain of logarithmic functions when solving equations to avoid extraneous solutions.
Q & A

  • What is the given function for f(x) in the transcript?

    -The function f(x) is defined as the logarithm base 10 of (x - 1) plus the logarithm base 10 of (x + 3).

  • What is the given function for g(x) in the transcript?

    -The function g(x) is defined as the logarithm base 10 of (x + 9).

  • What is the first step in finding the intersection points of the graphs of f and g?

    -The first step is to set the two functions equal to each other, meaning f(x) = g(x).

  • How does the speaker combine the two logarithms on the left side of the equation?

    -The speaker uses the product property of logarithms to combine the two logarithms by multiplying the insides of the logs.

  • What is the result of multiplying (x - 1) and (x + 3)?

    -The multiplication results in x^2 + 3x - x - 3, which simplifies to x^2 + 2x - 3.

  • What does the speaker do to eliminate the logarithm after multiplying the insides of the logs?

    -The speaker raises both sides of the equation to the power of 10, which is the base of the logarithm, to remove the logarithm.

  • What type of equation does the speaker end up with after removing the logarithm?

    -The speaker ends up with a quadratic equation, x^2 + 2x - 3.

  • How does the speaker simplify the quadratic equation to solve for x?

    -The speaker rearranges the equation to have zero on one side and then factors the quadratic to (x + 3)(x - 4) = 0.

  • What are the potential solutions for x from the factored equation?

    -The potential solutions are x = -3 and x = 4.

  • Why is x = -4 considered an extraneous solution?

    -x = -4 is considered an extraneous solution because it results in a negative value inside the logarithm, which is not within the domain of a logarithmic function.

  • What is the final solution for x that the speaker concludes is valid?

    -The final valid solution for x is 3, as it does not result in a negative value inside the logarithm.

Outlines
00:00
📚 Intersection Points of Logarithmic Functions

This paragraph discusses the process of finding the intersection points of two logarithmic functions, f(x) and g(x), defined with different bases and arguments. The speaker begins by setting the two functions equal to each other to find the points where their values are the same. Using the product property of logarithms, the equation is simplified to a quadratic form inside a logarithm. The speaker then exponentiates both sides with base 10 to remove the logarithm, resulting in a quadratic equation. After rearranging the terms and factoring, the solutions to the quadratic equation are found to be x = 3 and x = -4. However, the domain of the logarithmic functions restricts the solutions to positive values, thus x = -4 is discarded as an extraneous solution. The valid intersection point is therefore x = 3.

Mindmap
Keywords
💡Logarithm
A logarithm is the inverse operation to exponentiation, expressing the power to which a base number must be raised to produce a given value. In the video, logarithms with base 10 are used to define the functions f and g, which are essential for determining the points of intersection on the XY plane. The script uses logarithms to set up the equation for finding where the two functions are equal.
💡Function
A function in mathematics is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. The video discusses two specific functions, f(x) and g(x), which are used to find their intersection points by setting them equal to each other.
💡Intersection Points
Intersection points are the points where two or more geometric shapes, such as lines or curves, meet. In the context of the video, the intersection points are the solutions to the equation set up by equating the two given functions, which represent the points where their graphs meet on the XY plane.
💡Product Property
The product property of logarithms states that the logarithm of a product is equal to the sum of the logarithms of the factors. In the script, this property is used to combine two logarithmic expressions into a single logarithm of a product, simplifying the equation for further analysis.
💡Quadratic Equation
A quadratic equation is a polynomial equation of degree two, typically in the form ax^2 + bx + c = 0. The video script involves transforming the equation derived from the logarithmic expressions into a quadratic equation to find the values of x that satisfy the equation, which are the x-coordinates of the intersection points.
💡Factoring
Factoring is the process of breaking down a polynomial into a product of its factors. In the script, the quadratic equation x^2 + x - 12 is factored into (x + 3)(x - 4) to simplify the process of finding the roots, which are the x-values that make the equation equal to zero.
💡Roots
Roots of an equation are the values of the variable that make the equation true. In the context of the video, the roots of the quadratic equation are the x-values that, when substituted into the equation, result in zero, indicating the intersection points of the functions f and g.
💡Extraneous Solution
An extraneous solution is a solution that, although it satisfies the algebraic manipulation of an equation, does not satisfy the original equation's domain restrictions. In the video, the script identifies -4 as an extraneous solution because it would result in a negative value inside a logarithm, which is not allowed.
💡Domain
The domain of a function is the set of all possible input values (x-values) for which the function is defined. Logarithmic functions have a restricted domain, as they are only defined for positive arguments. The video script emphasizes the importance of checking the solutions against the domain of the original logarithmic functions.
💡XY Plane
The XY plane, also known as the Cartesian plane, is a two-dimensional plane that provides a framework for graphing functions and equations. In the video, the XY plane is the visual space where the graphs of the functions f and g are analyzed to find their intersection points.
💡Equation
An equation is a statement that asserts the equality of two expressions. In the video, the script involves setting up an equation by equating the two given functions, f(x) and g(x), to find the points where their graphs intersect on the XY plane.
Highlights

The problem involves finding the intersection points of two logarithmic functions, f(x) and g(x).

Function f(x) is defined as the sum of two logarithms with different bases and arguments.

Function g(x) is defined with a single logarithm with a base of 10 and a different argument.

The process begins by setting f(x) equal to g(x) to find the intersection points.

The product property of logarithms is used to combine the two logarithms in f(x).

The multiplication of the arguments (x - 1) and (x + 3) is performed to simplify the equation.

The equation is transformed into a single logarithm equivalent to the original g(x).

The multiplication results in a quadratic expression inside the logarithm.

The logarithm is eliminated by raising both sides as powers of 10.

A quadratic equation is derived from the simplified expression.

The quadratic equation is rearranged to set it equal to zero for factoring.

The quadratic expression factors into (x - 3)(x + 4).

Solving the factored equation yields two potential solutions, x = 3 and x = -4.

The domain of logarithmic functions restricts the possible solutions.

The solution x = -4 is identified as extraneous due to the domain restriction.

The valid solution for the intersection point is x = 3, as it falls within the domain of the logarithmic functions.

Transcripts

Browse More Related Video

AP CALCULUS AB 2022 Exam Full Solution FRQ#2a

Finding Intersections of Functions (Precaluclus - College Algebra 22)

Exponential Logarithmic Equations

2022 AP Calculus AB Free Response #2

Area Between Two Curves

Function Operations

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Logarithmic FunctionsIntersection PointsQuadratic EquationDomain AnalysisMath ProblemEducational ContentAlgebraic SolutionLogarithmic PropertiesExtraneous SolutionMathematical Analysis