AP Calculus BC 2023 Taylor Series FRQs: What to Look For?
TLDRThis educational video script discusses the importance of understanding Taylor series, focusing on recurring patterns in exam questions from 2018 to 2022. It emphasizes the need to master the ratio test, interval of convergence, and error bounds, particularly for alternating series. The speaker provides a detailed walkthrough of a 2022 problem, demonstrating how to apply the ratio test and alternating series test to determine convergence and calculate error bounds, highlighting the significance of careful study and targeted preparation.
Takeaways
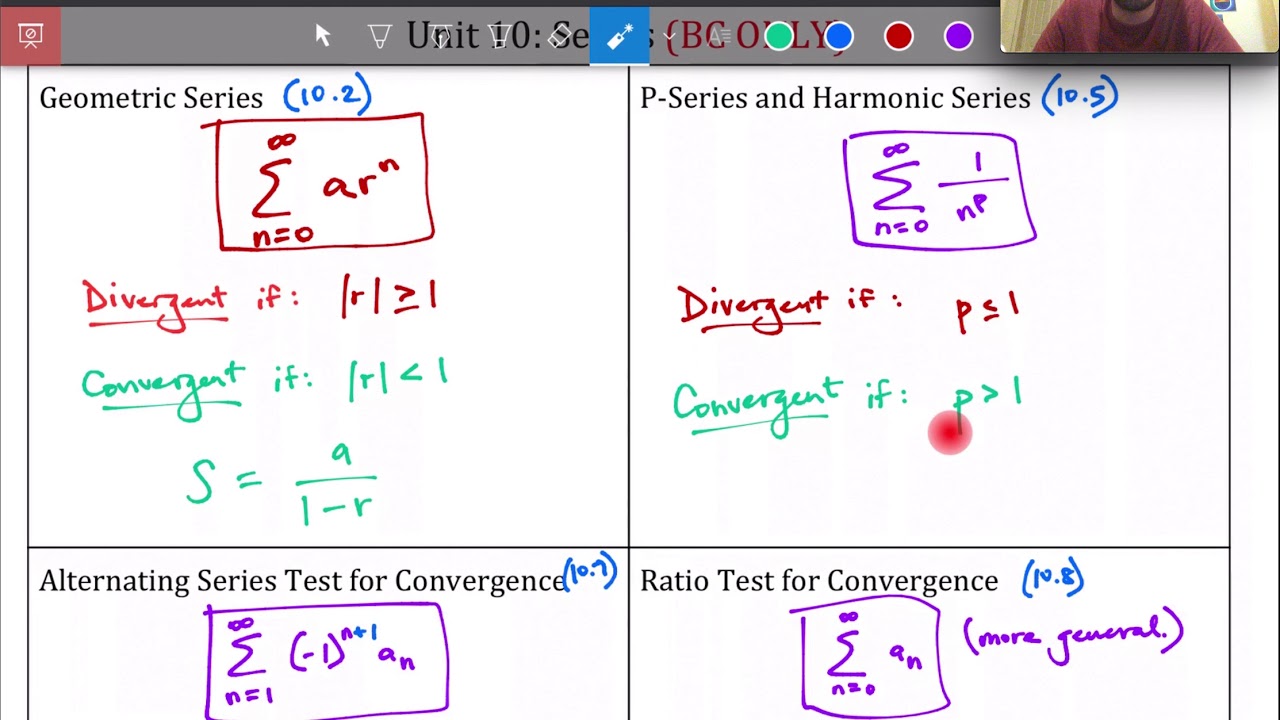
- 📚 The video is focused on analyzing Taylor series and understanding common patterns in exam questions related to power series.
- 🔍 The presenter highlights the importance of recognizing patterns in past exam questions to focus study efforts effectively.
- 📉 The script discusses the use of the Ratio Test to determine the interval of convergence for power series, a recurring theme in exam questions.
- 📌 The presenter emphasizes checking endpoints when determining the interval of convergence, as seen in the 2018 and 2022 exam questions.
- ⚠️ The need to apply the Alternating Series Test to ensure convergence at endpoints is stressed, especially when dealing with alternating series.
- 📝 The importance of stating that a series is an alternating series with terms decreasing in absolute value to zero is mentioned for clarity in exam responses.
- 📈 The script provides an example of using the Ratio Test to find the interval of convergence, illustrating the process step by step.
- 📊 The presenter shows how to calculate the error bound for an alternating series, using the formula and providing a numerical example.
- 🔢 The script touches on finding the first four non-zero terms of a power series, which is a common request in exam questions.
- 📐 The derivative of a power series and its representation as an infinite series are discussed, with an example calculation provided.
- 🔑 The video concludes with advice on studying smarter, not harder, and focusing on important topics like the Ratio Test, Alternating Series Test, and error bounds.
Q & A
What is the main topic of the video?
-The main topic of the video is to analyze Taylor series, focusing on determining the interval of convergence and error bounds using the alternating series test.
Why did the speaker exclude the year 2020 from the analysis?
-The speaker excluded the year 2020 because it was described as a 'funky year,' implying that it was an outlier or did not follow the patterns seen in the other years.
What is the significance of the 'ratio test' mentioned in the video?
-The ratio test is significant in determining the interval of convergence for a power series. It helps in establishing whether the series converges, diverges, or has an undetermined status.
What is the 'alternating series test' used for in the context of the video?
-The alternating series test is used to determine the convergence of a series where the terms alternate in sign and decrease in absolute value to zero.
What pattern did the speaker notice in the exam questions from 2018 to 2022?
-The speaker noticed a recurring pattern where questions about power series, interval of convergence, error bounds, and the alternating series test were frequently asked.
What is the interval of convergence for the power series discussed in the video?
-The interval of convergence for the power series discussed is between -1 and 1, inclusive of the endpoints, as determined by the ratio test and the alternating series test.
What is the error bound for the approximation found in the video?
-The error bound for the approximation is less than 1/10, as calculated using the alternating series error bound after plugging in the value of x as 1/2.
What is the significance of the 'integral test' and 'limit comparison test' mentioned in the video?
-The integral test and limit comparison test are significant as they are methods used to determine the convergence of series, especially in the context of infinite series.
How does the speaker approach the problem of finding the first four non-zero terms of a power series?
-The speaker approaches this by taking the derivative of the original power series and then identifying the first four non-zero terms of the resulting series.
What is the value of f'(1/6) as discussed in the video?
-The value of f'(1/6) is found by plugging in x = 1/6 into the derived series for f'(x), which results in a geometric series with a ratio of -(1/6)^2, leading to a value of 37/36.
Outlines
📚 Analyzing Taylor Series and Exam Patterns
The speaker begins by addressing the audience and expressing the intent to discuss Taylor Series, specifically focusing on a question related to the analysis of the series for BC. They provide an overview of the types of questions that have appeared in exams over the last four years, excluding 2020 due to its unusual circumstances. The speaker highlights the recurring themes in the exam questions, such as determining the interval of convergence and the error bound using the alternating series test. They also note the importance of understanding the ratio test and the interval of convergence for power series. The speaker's goal is to help the audience identify patterns in the exam questions to focus their study efforts more effectively.
🔍 Deep Dive into Power Series and Error Bounds
This paragraph delves deeper into the specifics of power series, alternating series tests, and error bounds. The speaker provides a step-by-step explanation of how to use the ratio test to find the interval of convergence for a given power series. They emphasize the importance of checking endpoints and apply the alternating series test to confirm convergence. The speaker then moves on to explain how to calculate the error bound for an alternating series, using a specific example where the series starts at one half. They stress the need to state the conditions for the alternating series test and to be careful with the signs in the calculations. The summary also includes a brief mention of finding the first four non-zero terms of a power series and the general term of the infinite series representing the derivative of a function.
📘 Final Thoughts on Study Strategies and Series Tests
In the final paragraph, the speaker wraps up the discussion by encouraging the audience to fine-tune their study focus based on the patterns observed in the exam questions. They stress the importance of working smarter, not harder, and using the insights gained from the video to concentrate on the most relevant topics. The speaker also provides a brief example of how to use the result from a previous part to find the value of a derivative at a specific point, highlighting the need to be cautious with the signs in the series. The paragraph ends with a call to action for the audience to like and subscribe to the video to help others benefit from the information as well.
Mindmap
Keywords
💡Taylor Series
💡Power Series
💡Interval of Convergence
💡Alternating Series Error Bound
💡Ratio Test
💡Integral Test
💡Limit Comparison Test
💡Geometric Series
💡F Prime (f')
💡Error Bound
Highlights
Introduction to analyzing Taylor series for BC calculus, focusing on patterns in past exam questions.
Review of 2018 BC exam question on determining the interval of convergence and error bound using the alternating series.
Observation of recurring themes in exam questions from 2018 to 2022, including power series, ratio test, and error bounds.
Emphasis on the importance of studying the ratio test and interval of convergence for power series.
Explanation of the alternating series error bound and its application in exam questions.
Demonstration of using the ratio test to find the interval of convergence for a given power series.
Process of checking endpoints to ensure convergence of the power series.
Explanation of the alternating series test for convergence at endpoints.
Calculation of the error bound for a specific value using the alternating series error bound.
Importance of stating the conditions for an alternating series when calculating error bounds.
Writing the first four non-zero terms of a power series as a common exam question.
Derivation of the power series representation of the derivative of a function.
Identification of the general term for the infinite series representing the derivative of a function.
Use of the result from part C to find the value of the derivative at a specific point.
Recognition of the geometric series in the derivative and its properties.
Advice on focusing study efforts on important topics based on exam patterns.
Encouragement to work smarter, not harder, when preparing for exams.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: